Dynamic fingerprint of fractionalized excitations in single-crystalline Cu₃Zn(OH)₆FBr

Abstract

Beyond the absence of long-range magnetic orders, the most prominent feature of the elusive quantum spin liquid (QSL) state is the existence of fractionalized spin excitations, i.e., spinons. When the system orders, the spin-wave excitation appears as the bound state of the spinon-antispinon pair. Although scarcely reported, a direct comparison between similar compounds illustrates the evolution from spinon to magnon. Here, we perform the Raman scattering on single crystals of two quantum kagome antiferromagnets, of which one is the kagome QSL candidate Cu₃Zn(OH)₆FBr, and another is an antiferromagnetically ordered compound EuCu₃(OH)₆Cl₃. In Cu₃Zn(OH)₆FBr, we identify a unique one spinon-antispinon pair component in the E_2g magnetic Raman continuum, providing strong evidence for deconfined spinon excitations. In contrast, a sharp magnon peak emerges from the one-pair spinon continuum in the E_g magnetic Raman response once EuCu₃(OH)₆Cl₃ undergoes the antiferromagnetic order transition. From the comparative Raman studies, we can regard the magnon mode as the spinon-antispinon bound state, and the spinon confinement drives the magnetic ordering.

Introduction

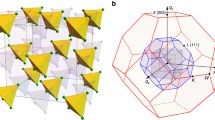
Quantum spin liquid (QSL) represents a new class of condensed matter states characterized by the long-range many-body entanglement of topological orders^{1,2,3,4,5,6,7,8,9}. The lattice of the spin-1/2 kagome network is a long-sought platform for antiferromagnetically interacting spins to host a QSL ground state^{10,11,12,13,14,15,16}. However, a structurally ideal realization of the kagome lattice in experiments is rare. Herbersmithite [ZnCu₃(OH)₆Cl₂] is the first promising kagome QSL candidate^{3,16,17,18,19,20,21,22,23}, in which no long-range magnetic order was detected down to low temperature^17,18, and inelastic neutron scattering revealed a magnetic continuum, as a hallmark of fractionalized spin excitations^20,22. Up to date, most, if not all, experimental information on the nature of kagome QSL relies on a single compound of Herbertsmithite. Considering the fact that a lattice distortion has recently been confirmed in Herbersmithite^24,25, which stimulates investigations on the subtle magneto-elastic effect in the kagome materials^26,27, an alternative realization of the QSL compound with the ideal kagome lattice is still in urgent need. Zn-Barlowite [Cu₃Zn(OH)₆FBr] is another candidate for a kagome QSL ground state^{28,29,30,31,32,33,34,35,36,37,38} with no lattice distortion being reported yet. Measurements on the powder samples didn’t detect the long-range magnetic order down to temperatures of 0.02 K, four orders of magnitude lower than the Curie–Weiss temperature^30,32. Besides the lack of magnetic order, the fractionalized spin excitations, i.e., spinons, is essential evidence for the long-range entanglement pattern in QSL. However, spectroscopic evidence for the deconfined spinon excitations in Zn-Barlowite is still lacking, in part due to unavailable single-crystal samples.

Raman scattering is sensitive to the local symmetries depending on the light polarization^39,40, and also capable of detecting magnetic excitations ranging from the spin-wave magnon excitation to deconfined spionons^{41,42,43,44,45,46,47,48,49,50}. Raman scattering has previously been reported for Herbertsmithite and revealed the multiple spinon scattering process¹⁹. In recent years, the atacamite family ReCu₃(OH)₆Cl₃ (Re=Y, Eu, Sm, and Nd) with the perfect kagome lattice has been synthesized and a chiral 120^∘ antiferromagnetic (AFM) order with the wave vector q = 0 is identified in the ground state^{51,52,53,54,55}. The kagome spin systems can be described by the kagome Heisenberg model with the Dzyaloshinski–Moriya (DM) interaction

$$H=J\mathop{\sum}\limits_{\langle ij\rangle }({{\bf{S}}}_{i}\cdot {{\bf{S}}}_{j})+D\hat{z}\cdot \mathop{\sum}\limits_{\langle ij\rangle }{{\bf{S}}}_{i}\times {{\bf{S}}}_{j},$$

(1)

where summation runs over nearest-neighbor bonds 〈ij〉, and J and D are the nearest-neighbor exchange and the DM interaction constants, respectively, for the spins S_i,j on the i- and j-th sites. We ignore the in-plane DM interactions regarding to the previous electron paramagnetic resonance measurements in the related kagome systems^55,56. A DM interaction larger than the critical value of (D/J)_c ~ 0.08 induces a chiral 120° AFM order from the QSL state^57,58,59. By the first-principle calculations (Supplementary Note 1), Zn-Barlowite and EuCu₃(OH)₆Cl₃ have D/J values of 0.05 and 0.3, resulting in QSL and AFM ground states, respectively, consistent with the experimental identification of the ground states^30,54. While the elementary spin excitation of the kagome QSL is the deconfined spinon, the low energy excitation in the kagome AFM ordered states is the magnon. A direct comparison by the magnetic Raman scattering can reveal the evolution from deconfined spinons in Zn-Barlowite to magnons in EuCu₃(OH)₆Cl₃, but has not been performed yet.

In this work, we exclude the kagome lattice distortion by angle-resolved polarized Raman (ARPR) scattering and second-harmonic-generation (SHG), and reveal the spin dynamics of spinon excitations on the single-crystalline Cu_3.18Zn_0.82(OH)₆FBr. We observe a remarkable E_2g magnetic Raman continuum, which can be decomposed into one spinon–antispinon pair (one-pair (1P)) and two spinon–antispinon pair (two-pair (2P)) components of spinon excitations, in line with theoretical studies of the kagome QSL⁶⁰. The one-pair continuum is unique, serving as the fingerprint of spinons. In a control experiment, beside the two-magnon (2M) magnetic Raman continuum, we probe a sharp one-magnon (1M) Raman peak in EuCu₃(OH)₆Cl₃ below the AFM transition temperature. The magnon peak emerges from the 1P continuum in the magnetic Raman scattering, can be regarded as the bound state of the spinon–antispinon excitations. As schematically summarized in Fig. 1, our comparative Raman study demonstrates the spinon deconfinement and confinement in the kagome QSL compound and ordered antiferromagnet, respectively. The AFM order transition can be thought to be driven by the spinon confinement.

Fig. 1: Schematical comparative Raman responses for the AFM and QSL states.

With a large DM interaction D, the kagome antiferromagnet develops a chiral 120° AFM ground state. Increasing J/D, the fluctuation of the kagome system increases, driving the system into the QSL state. By increasing the temperature, the thermal fluctuation melts the magnetic order and turns the system into the classic paramagnetic state at high temperatures. Cu₃Zn and EuCu₃ have the QSL and AFM ground states, and allow spinon and magnon excitations, respectively. Magnetic Raman scattering measures different elementary excited states in the two different ground states. Here 1P and 2P denote the one-pair and two-pair spinon excitations, respectively. 1M and 2M in AFM ordered state denote the one- and two-magnon excitations, respectively. The 1M Raman peak in AFM measures the magnon while the 1P Raman continuum in QSL probes the spinon excitations. The shadow background of the 1M peak, marked as `1P', denotes the continuum above T_N in EuCu₃, mimicking the 1P continuum in the QSL state.

Results

We grown single crystals of Barlowite Cu₄(OH)₆FBr, Zn-Barlowite Cu_3.18Zn_0.82(OH)₆FBr, and EuCu₃(OH)₆Cl₃ (we use the short-hand notation Cu₄, Cu₃Zn, and EuCu₃, respectively) with high quality (“Methods” and Supplementary Note 2). The interlayer Cu²⁺ concentration (18%) is comparable to that (15%) in Herbertsmithite⁶¹. We estimate the superexchange strength for the kagome spins in Cu₃Zn as J ≃ 13 meV by the Curie–Weiss temperature Θ_CW = −220 K (Supplementary Note 2)⁶². The superexchange interaction for EuCu₃ is about J ≃ 7 meV^53,54,55. Note the electronic ground state of Eu³⁺ in EuCu₃ is the non-magnetic ⁷F₀ configuration.

Figure 2a presents the temperature evolution of Raman spectra in Cu₃Zn with sharp phonon modes superimposing on the magnetic continuum background. With the help of first-principles calculations, we assign the symmetry representations for phonon modes in Supplementary Note 3. No structural phase transition is observed in Cu₃Zn down to 4 K. We tracked the Raman spectral evolution of the crystal structures from Cu₄ to Cu₃Zn (Supplementary Note 4). Cu₃Zn has no Raman-active mode related to the kagome Cu²⁺ vibrations, indicating the kagome layer remains intact. Cu₄ has distorted kagome layers at 200 K, signaled by an extra phonon mode at 62 cm⁻¹ corresponding to the kagome Cu²⁺ vibration. The previous SHG study revealed the parity symmetry in Barlowite 2 [Cu₄(OH)₆FBr] and Zn-Barlowite [Cu_3.66Zn_0.33(OH)₆FBr]²⁵. We confirmed the inversion symmetry by SHG in our single crystals of Cu₃Zn (Supplementary Note 6).

Fig. 2: Temperature dependent and ARPR spectra in Cu₃Zn.

a Temperature evolution of unpolarized Raman spectra in Cu₃Zn. The inset is the photo of single crystals. ARPR intensity for low-energy continua (b), the Br⁻E_2g phonon (75 cm⁻¹) (c), and the O²⁻A_1g phonon (429 cm⁻¹) (d). The dash-dotted lines are the corresponding theoretical curves based on the C₃ rotation symmetry.

Figures 2b–d are the ARPR responses of Cu₃Zn in three different polarization configurations (“Methods” and Supplementary Note 5). In the XX (XY) configuration, the incoming and outgoing light polarizations are parallel (perpendicular) and rotated simultaneously. In the X-only configuration, the outgoing polarization is fixed and only incoming light is rotated. Theoretically, the Raman cross section of a Kagome QSL ground state does not depend on the polarization of the incoming or outgoing light³⁹ and keeps invariant against rotating light polarization in the XX, XY, and X-only configurations. Figure 2b is the ARPR response for the magnetic continua at low frequency with the integrated Raman susceptibility $\chi ^{\prime} =\frac{2}{\pi }\mathop{\int}\nolimits_{10{\text{cm}}^{-1}}^{60{\text{cm}}^{-1}}\frac{\chi ^{\prime\prime} (\omega )}{\omega }d\omega$, where the susceptibility is related to the Raman intensity I(ω) = (1 + n(ω))χ″(ω) with the bosonic temperature factor n(ω). Figure 2c and d are the corresponding results of the Br⁻E_2g phonon, and O²⁻A_1g phonon modes, respectively. For threefold rotation symmetry, the A_1g mode response follows the ${\cos }^{2}(\theta )$ function of the rotation angle θ in X-only configuration, keeps constant in XX polarization, and vanishes in XY configuration; the E_2g mode is isotropic in all the three configurations. The magnetic continuum contains both A_1g and E_2g channels at high temperature (290 K), and only the E_2g channel at low temperature (4 K). The experimental ARPR responses agree well with the theoretical dash-dotted curves, confirming the threefold rotational symmetry in the magnetic excitations (Fig. 2b) and lattice vibrations (Figs. 2c, d). We notice that in Herbertsmithite, although it was not discussed, the lattice distortion was evident by the anisotropic ARPR responses¹⁹ and may account for the difference from our results.

Having established the structurally ideal realization of the kagome lattice by SHG and ARPR scattering, and the absence of the thermodynamic anomaly, we now present our spectroscopic results of spin dynamics in Cu₃Zn with subtracting phonon contributions. Figure 3a–c are the magnetic continuum of Cu₃Zn in the A_1g channel, which is activated only at high temperatures, and disappears at low temperatures. The integrated Raman susceptibility in Fig. 3b fits the thermally activated function, $\chi ^{\prime} (T)\propto {{\rm{e}}}^{-{\omega }^{* }/T}$ with ω* = 53 cm⁻¹. The result suggests the A_1g continuum measures the thermal fluctuation of the interacting kagome spins^41,63,64. Different from the A_1g channel, the pronounced E_2g magnetic Raman continuum persists down to 4 K (Fig. 3d–f), indicating the intrinsic quantum fluctuation of the kagome spins. The substantial low energy component has a non-monotonic temperature dependence. It increases with the temperature decreasing from 290 K to 50 K, but decreases with further temperature reducing as shown in Fig. 3d–f. The E_2g magnetic Raman susceptibility χ″(ω, T) distributes the main spectral weight among the frequency region less than 400 cm⁻¹, and reaches the maximum at around 150 cm⁻¹ and 50 K, as shown in Fig. 3f.

Fig. 3: Temperature dependent magnetic Raman continua in Cu₃Zn.

a The A_1g Raman susceptibility ${\chi }_{{A}_{\text{1g}}}^{^{\prime\prime} }={\chi }_{{\rm{XX}}}^{^{\prime\prime} }-{\chi }_{{\rm{XY}}}^{^{\prime\prime} }$. The solid lines are guides to the eye. b Temperature dependence of the A_1g static Raman susceptibility ${\chi }_{{A}_{\text{1g}}}^{\prime} (T)=\frac{2}{\pi }\mathop{\int}\nolimits_{10\,{\text{cm}}^{-1}}^{400\,{\text{cm}}^{-1}}\frac{{\chi }_{{A}_{\text{1g}}}^{^{\prime\prime} }(\omega )}{\omega }d\omega$. The solid line is a thermally activated function. c Color map of ${\chi }_{{A}_{\text{1g}}}^{^{\prime\prime} }(\omega ,T)$. d The E_2g Raman response function ${\chi }_{{E}_{\text{2g}}}^{^{\prime\prime} }={\chi }_{{\rm{XY}}}^{\prime}$. The solid lines are guides to the eye. The light green and pink shadow marked as “1P” and “2P” represent the one-pair and two-pair components of Raman continuum. e Temperature dependence of the E_2g static Raman susceptibility $\chi_{{E}_{\text{2g}}} ^{\prime} (T)=\frac{2}{\pi }\mathop{\int}\nolimits_{10\,{\text{cm}}^{-1}}^{780\,{\text{cm}}^{-1}}\frac{{\chi }_{{E}_{\text{2g}}}^{^{\prime\prime} }(\omega )}{\omega }d\omega$. The solid line is a guide to the eye. f Color map of ${\chi }_{{E}_{\text{2g}}}^{^{\prime\prime} }(\omega ,T)$.

The low-energy E_2g Raman continuum is crucial as it has an origin of the spinon excitation in the kagome QSL from the theoretical perspective⁶⁰. In the XY configuration for the E_2g channel, the Raman tensor on the kagome lattice is written in terms of spin-pair operators^39,60,65,66

$${\tau }_{R}\propto \mathop{\sum}\limits_{R}{{\bf{S}}}_{R3}\cdot ({{\bf{S}}}_{R1}+{{\bf{S}}}_{R+{{\bf{a}}}_{2}1}-{{\bf{S}}}_{R2}-{{\bf{S}}}_{R-{{\bf{a}}}_{1}+{{\bf{a}}}_{2}2}),$$

(2)

where S_R1,2,3 are spin operators on three sites of the R-th kagome unit cell and a_1,2 are the lattice vectors. The spin operator has the spinon f_iσ representation ${S}_{i}^{\alpha }={\sum }_{\sigma \sigma ^{\prime} }{f}_{i\sigma }^{\dagger }{\tau }_{\sigma \sigma ^{\prime} }^{\alpha }{f}_{i\sigma ^{\prime} }/2$ where τ^α is the α-th Pauli matrix. The spin-pair is ${{\bf{S}}}_{i}\cdot {{\bf{S}}}_{j}=-\frac{1}{2}{\hat{\chi }}_{ij}^{\dagger }{\hat{\chi }}_{ij}$ with ${\hat{\chi }}_{ij}={\sum }_{\sigma }{f}_{i\sigma }^{\dagger }{f}_{j\sigma }$. In the mean field theory, the spinon hopping amplitude $\chi =\langle {\hat{\chi }}_{ij}\rangle$ is non-zero. So we have 1P and 2P components in the Raman tensor⁶⁰

$${\tau }_{R}^{{\rm{1P}}}\propto \chi \mathop{\sum}\limits_{R}({\hat{\chi }}_{R3,R1}+\,\text{h.c.}\,)+\cdots \ ,$$

(3)

$${\tau }_{R}^{{\rm{2P}}}\propto \mathop{\sum}\limits_{R}{\hat{\chi }}_{R3,R1}^{\dagger }{\hat{\chi }}_{R3,R1}+\cdots \ ,$$

(4)

where ⋯ denotes omitted terms in Eq. (2) for the notation simplicity. While the 2P component is analogous to the 2M scattering, the 1P contribution is a unique prediction for spinon excitations in the kagome QSL. In Fig. 3d, we schematically decompose the E_2g Raman continuum into 1P and 2P components of spinon–antispinon excitations. The 1P component has the maximum at 150 cm⁻¹ (1.4J), and extends up to 400 cm⁻¹ (3.8J) at low temperatures. The 2P component has the maximum at 400 cm⁻¹ (3.8J) and the cut-off around 750 cm⁻¹ (6.7J). The mentioned features (maxima and cut-offs) of 1P and 2P excitations in the E_2g Raman response agree well with the theoretical prediction for the kagome QSL state⁶⁰.

In more detail, the 1P component dominates the E_2g magnetic Raman continuum at low frequency. It displays the power-law behavior up to 70 cm⁻¹, with a significantly nonmonotonic temperature dependence, as shown in Fig. 4. The low-energy continuum evolves from a sublinear behavior T^α with α < 1 to a superlinear one T^α with α > 1 as reducing the temperature. A central question for the kagome QSL is whether a spin gap exists. Previous results on the powder samples of Cu₃Zn suggest a small spin gap^30,32. If such a gap exists, the power-law behavior of the E_2g magnetic Raman continua sets an upper bound for the spin gap of 2 meV.

Fig. 4: Power-law behavior for E_2g magnetic Raman continua at low frequency in Cu₃Zn.

a, b are power-law fitting of ${\chi }_{{E}_{\text{2g}}}^{^{\prime\prime} }(\omega )\propto {\omega }^{\alpha }$ at low and high temperatures, respectively. c Temperature-dependent exponent α for the power-law fitting.

The theoretical calculation for kagome Dirac spin liquid (DSL) predicts the power-law behavior for the Raman susceptibility in the E_2g channel at low frequency⁶⁰. The 1P spinon excitation in DSL gives the linear density of state (DOS) ${{\mathcal{D}}}_{\text{1P}}\propto \omega$. The matrix element turns out to be exactly zero for all 1P excitations with ω = 0 in the mean field Dirac Hamiltonian. As a result a Raman spectrum that scales as ω³ was predicted. However, the vanishing of the matrix element is somewhat accidental and depends on the assumption of a DSL in an ideal kagome Heisenberg model. Any deviation from the ideal DSL state, e.g., a small gap in the ground state^30,32, DM interactions, or other effects of perturbations^26,67, changes the wave functions and may result in a constant matrix element. In that case, the Raman spectrum will be simply proportional to the DOS of the 1P component ${{\mathcal{D}}}_{\text{1P}}$ which is linear in ω. From our fitting for Cu₃Zn in Fig. 4, we find that α = 1.3 when approaching zero temperature. The existence of a small gap in the spinon spectrum may explain the discrepancy.

Considering the interlayer Cu²⁺ concentration (18%) in Cu₃Zn, we make a remark here about the disorder effect in the magnetic Raman scattering. The temperature-dependent E_2g static magnetic susceptibility ${\chi }_{{E}_{\text{2g}}}^{\prime}(T)$ of Cu₃Zn in Fig. 3 exhibit the maximal spin fluctuations at 50 K. The non-monotonic T-dependence deviating from the Curie–Weiss behavior is associated with the enhancement of nearest-neighbor spin correlations at low temperatures⁶⁷. However, such significant deviation from Curie–Weiss behavior is masked by the interlayer Cu²⁺ moments in the bulk thermodynamic measurements, e.g., heat capacity and bulk magnetization³⁰. In contrast to a significant energy dependent magnetic Raman susceptibility ${\chi }_{{E}_{\text{2g}}}^{^{\prime\prime} }(\omega )$ at 4 K in Cu₃Zn, the scattered neutron signal ${\chi }_{\,\text{INS}\,}^{^{\prime\prime} }(\omega )$ in Herbertsmithite is overall insensitive to energy transfer, rather flat above 1.5 meV, but increases significantly with reducing energy below 1.5 meV due to the interlayer Cu²⁺ ions^20,22. So Raman scattering singles out the kagome magnetic excitations and remains unmasked in the presence of the interlayer Cu²⁺ due to the matrix element effect as explained below. The Raman scattering measures the nearest-neighbor spin-pair τ_R ∝ S_i ⋅ S_j dynamics, but the spin pairs associated with the interlayer Cu²⁺ ions are weaker than the singlet pairs for the kagome spins. As the light polarization in our Raman measurements is in the kagome ab plane, and the projected factor of the spin-pairs associated with the interlayer Cu²⁺ ions, $({{\bf{r}}}_{ij}\cdot {\hat{{\bf{e}}}}_{\text{in}})({{\bf{r}}}_{ij}\cdot {\hat{{\bf{e}}}}_{\text{out}})$, is small, as the related pair bond vector r_ij has the angle around 52° with respect to the kagome plane. As a result, the interlayer Cu²⁺ ions contribute a negligible Raman matrix element and we ignore their effect in the discussions about the Raman experiments. Moreover, the inelastic neutron scattering in Herbertsmithite measures the magnetic continuum up to 3J²⁰, the same energy range as the 1P Raman component in Cu₃Zn. These results suggest that the magnetic Raman continuum originates from the kagome spins, and the 1P component has an origin of spinon excitations.

Figure 5 presents a control Raman study on the magnetic ordered kagome antiferromagnet EuCu₃, which has the antiferromagnetic superexchange strength J ≃ 7 meV. In Supplementary Note 7, the ARPR scattering on EuCu₃ confirms the threefold rotational symmetry. Above the ordering temperature T_N = 17 K, the magnetic Raman continuum in the E_g channel displays the extended continuum, similar to the E_2g magnetic continuum at 4 K in Cu₃Zn. Below T_N, a sharp peak, i.e., 1M peak as discussed below, is observed on top of the magnetic continuum. The integrated Raman susceptibility ${\chi }_{{E}_{\text{g}}}^{\prime}(T)$ monotonically increases as lowering the temperature as shown in Fig. 5b, different from non-monotonic behavior in ${\chi }_{{E}_{\text{2g}}}^{\prime}(T)$ of Cu₃Zn in Fig. 3e. The magnetic Raman susceptibility χ″(ω, T) in EuCu₃ distributes the main spectral weight among the frequency region less than 400 cm⁻¹, and the magnon peak locates at 72 cm⁻¹ below 17 K, as shown in Fig. 5c.

Fig. 5: Temperature dependent E_g magnetic Raman continua in EuCu₃.

a The E_g Raman susceptibility ${\chi }_{{E}_{\text{g}}}^{^{\prime\prime} }={\chi }_{{\rm{XY}}}^{^{\prime\prime} }$. The solid lines are guides to the eye. A sharp magnon peak appears in the E_g magnetic Raman continuum below the magnetic transition temperature T_N = 17 K. b Temperature dependence of the static Raman susceptibility in the E_g channel $\chi_{{E}_{\text{g}}} ^{\prime} (T)=\frac{2}{\pi }\mathop{\int}\nolimits_{10\,{\text{cm}}^{-1}}^{780\,{\text{cm}}^{-1}}\frac{{\chi }_{{E}_{\text{g}}}^{^{\prime\prime} }(\omega ,T)}{\omega }d\omega$. The solid line is a guide to the eye. c Color map of ${\chi }_{{E}_{\text{g}}}^{^{\prime\prime} }(\omega ,T)$.

To directly compare the 1P spinon continuum in Cu₃Zn and the 1M peak in EuCu₃, we plot the E_g Raman response in EuCu₃ at selected temperatures in Fig. 6. The E_2g Raman continuum in Cu₃Zn at 4 K is also plotted with the proper scale for the Raman frequency. Above T_N = 17 K, EuCu₃ has the substantial magnetic continuum with the profile similar to that in Cu₃Zn at 4 K. There are less pronounced low-energy continuum excitations in EuCu₃ than those in Cu₃Zn, probably due to the large DM interaction which suppresses the low-energy quantum fluctuations. Below T_N, a sharp magnon peak at 72 cm⁻¹ appears in EuCu₃ with the corresponding energy scale of the 1P continuum maximum in Cu₃Zn. We stress that the magnon Raman peak is direct spectroscopic evidence for the q = 0 120° non-collinear AFM spin configurations, and invisible in the $\sqrt{3}\times \sqrt{3}$ structure of the 120^∘ AFM (“Methods”).

Fig. 6: Comparative Raman studies of EuCu₃ and Cu₃Zn.

We select the E_g magnetic Raman continua in EuCu₃ at several temperatures. For a comparison, we also plot the E_2g magnetic Raman continuum in Cu₃Zn at 4 K with the Raman shift scaled by the superexchange energy ratio of 1.9.

For the AFM order state, the low-energy excitation is the spin-wave magnon which can be described in the spin-wave theory⁶⁸. In the local spin basis ${\tilde{{\bf{S}}}}_{i}$ of the AFM order, we have the Raman tensors in the XY configuration of the E_g channel for 1M and 2M components as following

$${\tau }_{R}^{{\rm{1M}}}\propto \mathop{\sum}\limits_{R}({\tilde{{\bf{S}}}}_{R1}^{y}+{\tilde{{\bf{S}}}}_{R2}^{y}-{\tilde{{\bf{S}}}}_{R3}^{y}),$$

(5)

$${\tau }_{R}^{{\rm{2M}}}\propto \mathop{\sum}\limits_{R}{\tilde{{\bf{S}}}}_{R3}\odot ({\tilde{{\bf{S}}}}_{R1}+{\tilde{{\bf{S}}}}_{R+{{\bf{a}}}_{2}1}-{\tilde{{\bf{S}}}}_{R2}-{\tilde{{\bf{S}}}}_{R-{{\bf{a}}}_{1}+{{\bf{a}}}_{2}2}),$$

(6)

with the 2M spin-pair operator ${\tilde{{\bf{S}}}}_{i}\odot {\tilde{{\bf{S}}}}_{j}={\tilde{S}}_{i}^{x}{\tilde{S}}_{j}^{x}+({\tilde{S}}_{i}^{y}{\tilde{S}}_{j}^{y}+{\tilde{S}}_{i}^{z}{\tilde{S}}_{j}^{z})/2$. For the details, please refer to the “Methods” section. Therefore, the E_g Raman scattering in the AFM order state measures 1M and 2M excitations as demonstrated in Fig. 1. Thus, the magnon excitation emerges from the 1P continuum and can be regarded as the bound state of the spinon–antispinon excitations.

Discussion

Deconfined spinons yield to the magnetic continuum, however, the Raman continuum does not necessarily imply the spin fractionalization. Only 2M excitation itself gives rise to a Raman continuum in the ordered antiferromagnet⁴². In this work, the comparative Raman study in Cu₃Zn and EuCu₃ resolves this uncertainty. Guided by the theoretical prediction⁶⁰, the E_2g Raman continuum can be decomposed into 1P and 2P components of the spinon–antispinon excitations. While the 2P component has the maximum at 3.8J, resembling the 2M broad peak⁴², the 1P continuum in Raman is unique for QSL. Its maximum and extended range have the same energy scale as the spin-wave magnon peak in EuCu₃ and the inelastic neutron continuum cutoff (up to 3J) in the Herbertsmithite, respectively.

The 1P component of Raman continuum reveals fractionalized spin excitations, providing strong evidence for the kagome QSL ground state in Cu₃Zn. Our comparative Raman studies explicitly show the evolution from the deconfined spinon excitation in the kagome quantum spin liquid compound Cu₃Zn to the conventional magnon in the kagome ordered antiferromagnet EuCu₃. On the material side, Zn-Barlowite is an ideal structural realization of the kagome lattice. Along with Herbertsmithite, the single-crystalline Zn-Barlowite stands able to single out the intrinsic properties of the kagome QSL.

Methods

Sample preparation and characterization

High qualified single crystals of Zn-Barlowite was grown by a hydrothermal method similar to crystal growth of herbertsmithite^69,70. CuO (0.6 g), ZnBr₂ (3 g), and NH₄F (0.5 g) and 18 ml deionized water were sealed in a quartz tube and heated between 200 °C and 140 °C by a two-zone furnace. After 3 months, we obtained millimeter-sized single crystal samples. The value of x in Cu_4−xZn_x(OH)₆FBr has been determined as 0.82 by Inductively Coupled Plasma-Atomic Emission Spectroscopy (ICP-AES). The single-crystal X-ray diffraction has been carried out at room temperature by using Cu source radiation (λ = 1.54178 Å) and solved by the Olex2.PC suite programs⁷¹. The structure and cell parameters of Cu_4−xZn_x(OH)₆FBr are in coincidence with the previous report on polycrystalline samples^30,32. For Barlowite(Cu₄(OH)₆FBr), the mixture of CuO (0.6 g), MgBr₂ (1.2 g), and NH₄F (0.5 g) was transferred into Teflon-lined autoclave with 10 ml water. The autoclave was heated up to 260 °C and cooled to 140 °C after 2 weeks. A similar growth condition to Barlowite was applied for the growth of EuCu₃(OH)₆Cl₃ with staring materials of EuCl₃ ⋅ 6H₂O (2 g) and CuO (0.6 g).

Measurement methods

Our thermodynamical measurements were carried out on the Physical Properties Measurement System (PPMS, Quantum Design) and the Magnetic Property Measurement System (MPMS3, Quantum Design).

The temperature-dependent Raman spectra are measured in a backscattering geometry using a home-modified Jobin-Yvon HR800 Raman system equipped with an electron-multiplying charged-coupled detector (CCD) and a ×50 objective with long working distance and numerical aperture of 0.45. The laser excitation wavelength is 514 nm from an Ar⁺ laser. The laser-plasma lines are removed using a BragGrate bandpass filter (OptiGrate Corp.), while the Rayleigh line is suppressed using three BragGrate notch filters (BNFs) with an optical density 4 and a spectral bandwidth ~ 5–10 cm^−1 72. Thus, Raman signal down to 5 cm⁻¹ can be measured⁷³. The 1800 lines/mm grating enables each CCD pixel to cover 0.6 cm⁻¹. The samples are cooled down to 30 K using a Montana cryostat system under a vacuum of 0.4 mTorr and down to 4 K using an attoDRY 1000 cryogenic system. All the measurements are performed with a laser power below 1 mW to avoid sample heating. The temperature is calibrated by the Stokes-anti-Stokes relation for the magnetic Raman continuum and phonon peaks. The intensities in two cryostat systems are matched by the Raman susceptibility. The ARPR measurements⁴⁰ with light polarized in the ab kagome plane of samples were performed in parallel (XX), perpendicular (XY), and X-only polarization configurations (Supplementary Note 5).

SHG measurements were performed using a homemade confocal microscope in a backscattering geometry. A fundamental wave centered at 800 nm was used as excitation source, which was generated from a Ti-sapphire oscillator (Chameleon Ultra II) with an 80 MHz repetition frequency and a 150 fs pulse width. After passing through a ×50 objective, the pump beam was focused on the sample with a diameter of 2 μm. The scattering SHG signals at 400 nm were collected by the same objective and led to the entrance slit of a spectrometer equipped with a thermoelectrically cooled CCD. Two shortpass filters were employed to cut the fundamental wave.

Magnon Raman peak in kagome AFM ordered state

With a large DM interaction D, the kagome antiferromagnet in Eq. (1) devoleps a q = 0 type 120° AFM order at low temperature in EuCu₃^{53,54,55,57,58,59}. In terms of the local basis for the AFM order, we rewrite the Hamiltonian as

$$H=J\mathop{\sum}\limits_{\langle ij\rangle }{\tilde{{\bf{S}}}}_{i}\odot {\tilde{{\bf{S}}}}_{j}+D\mathop{\sum}\limits_{\langle ij\rangle }{\tilde{{\bf{S}}}}_{i}\otimes {\tilde{{\bf{S}}}}_{j},$$

(7)

with

$${\tilde{{\bf{S}}}}_{i}\odot {\tilde{{\bf{S}}}}_{j}={S}_{i}^{x}{S}_{j}^{x}+\cos ({\theta }_{ij})({S}_{i}^{y}{S}_{j}^{y}+{S}_{i}^{z}{S}_{j}^{z})+\sin ({\theta }_{ij})({S}_{i}^{z}{S}_{j}^{y}-{S}_{i}^{y}{S}_{j}^{z}),$$

(8)

$${\tilde{{\bf{S}}}}_{i}\otimes {\tilde{{\bf{S}}}}_{j}=\sin ({\theta }_{ij})({S}_{i}^{y}{S}_{j}^{y}+{S}_{i}^{z}{S}_{j}^{z})+\cos ({\theta }_{ij})({S}_{i}^{y}{S}_{j}^{z}-{S}_{i}^{z}{S}_{j}^{y}),$$

(9)

where θ_ij is an angle between two neighboring spins and ${S}_{i}^{x,y,z}$ below denotes the local basis of the AFM order. The effective linear spin wave Hamiltonian is given as

$${{\mathcal{H}}}_{\text{eff}}=J\mathop{\sum}\limits_{\langle ij\rangle }[{S}_{i}^{x}{S}_{j}^{x}+(\cos {\theta }_{ij}+\sin {\theta }_{ij}D/J)\times ({S}_{i}^{y}{S}_{j}^{y}+{S}_{i}^{z}{S}_{j}^{z})],$$

(10)

for which the Holstein-Primakoff representation for spin operators in the local basis was applied and the energy dispersion was obtained in ref. ⁶⁸.

In the local spin basis, we have the Raman tensor in the XY configuration is given as

$${\tau }_{R}^{{\rm{XY}}}=\frac{\sqrt{3}}{4}\mathop{\sum}\limits_{R}{\tilde{{\bf{S}}}}_{R3}\odot ({\tilde{{\bf{S}}}}_{R1}+{\tilde{{\bf{S}}}}_{R+{{\bf{a}}}_{2}1}-{\tilde{{\bf{S}}}}_{R2}-{\tilde{{\bf{S}}}}_{R-{{\bf{a}}}_{1}+{{\bf{a}}}_{2}2}).$$

(11)

In the spin-pair operator ${\tilde{{\bf{S}}}}_{i}\odot {\tilde{{\bf{S}}}}_{j}$ in Eq. (8), there are two-magnon contribution in terms of ${S}_{i}^{x}{S}_{j}^{x}+\cos ({\theta }_{ij})({S}_{i}^{y}{S}_{j}^{y}+{S}_{i}^{z}{S}_{j}^{z})$, and one- and three-magnon contributions in terms of $\sin ({\theta }_{ij})({S}_{i}^{z}{S}_{j}^{y}-{S}_{i}^{y}{S}_{j}^{z})$. For the q = 0 spin configuration, we find that ${\tau }_{R}^{{\rm{XY}}}$ in Eq. (11) has the non-vanished one magnon contributions. For the $\sqrt{3}\times \sqrt{3}$ AFM state, ${\tau }_{R}^{{\rm{XY}}}$ has no one-magnon contribution. Therefore, the observed one-magnon peak in the E_g channel in EuCu₃ provides evidence for the q = 0 spin ordering at low temperatures. In the linear spin-wave theory, we take S^z in the local basis as a constant, ${S}_{i}^{z}=\langle {S}^{z}\rangle =1/2$, and the Raman tensor in XY configuration is given as

$${\tau }_{R}^{{\rm{XY}}}=\frac{3}{8}\mathop{\sum}\limits_{R}({S}_{R1}^{y}+{S}_{R2}^{y}-2{S}_{R3}^{y}),$$

(12)

in terms of the local basis, directly measuring the one magnon excitation.

For EuCu₃, the exchange interaction parameters are estimated as J = 7 meV, D/J = 0.3, leading to the magnon peak position of Δ_sw = 1.1J = 77 cm⁻¹, very close to the measured value 72 cm⁻¹ in our Raman measurement of the one-magnon peak.