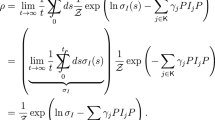Skip to main content
SpringerLink
Log in

Finite Precision Representation of the Conley Decomposition

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

We present a theoretical basis for a novel way of studying and representing the long-time behavior of finite-dimensional maps. It is based on a finite representation of ε-pseudo orbits of a map by the sample paths of a suitable Markov chain based on a finite partition of phase space. The use of stationary states of the chain and the corresponding partition elements in approximating the attractors of maps and differential equations was demonstrated in Refs. 7 and 3 and proved for a class of stable attracting sets in Ref. 8. Here we explore the relationship between the communication classes of the Markov chain and basic sets of the Conley decomposition of a dynamical system. We give sufficient conditions for the existence of a chain transitive set and show that basic sets are isolated from each other by neighborhoods associated with closed communication classes of the chain. A partition element approximation of an isolating block is introduced that is easy to express in terms of sample paths. Finally, we show that when the map supports an SBR measure there is a unique closed communication class and the Markov chain restricted to those states is irreducible.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

REFERENCES

  1. Akin, E. (1993). The General Topology of Dynamical Systems, American Mathematical Society, Providence, RI.

    Google Scholar 

  2. Bowen, R. (1975). Equilibrium States and the Theory of Axiom A Diffeomorphisms, Lecture Notes in Mathematics, Vol. 470, Springer Verlag, New York.

    Google Scholar 

  3. Dellnitz, M., and Junge, O. (1998). On the approximation of complicated dynamical behavior. SIAM J. Num. Anal. (in press).

  4. Dellnitz, M., Hohmann, A., Junge, O., and Rumpf, M. (1997). Exploring invariant sets and invariant measures. Chaos 7(2), 221-228.

    Google Scholar 

  5. Easton, R. W. (1989). Isolating blocks and epsilon chains for maps. Physica D 39(1), 95-110.

    Google Scholar 

  6. Easton, R. W. (1993). Transport of phase space volume near isolated invariant sets. J. Dynam. Diff. Eq. 5(3), 529-536

    Google Scholar 

  7. Hsu, C. S. (1987). Cell to Cell Mapping: A Method of Global Analysis for Nonlinear Systems, Springer Verlag, New York.

    Google Scholar 

  8. Hunt, F. Y. (1998). Unique ergodicity and the approximation of attractors and their invariant measures using Ulam's method. Nonlinearity 11, 307-317.

    Google Scholar 

  9. Hunt, F. Y. (1996). Approximating the invariant measures of randomly perturbed dissipative maps. J. Math. Anal. Appl. 198, 534-551.

    Google Scholar 

  10. Hunt, F. Y., and Miller, W. M. (1992). On the approximation of invariant measures. J. Stat. Phys. 66, 535-548.

    Google Scholar 

  11. Kifer, Y. (1988). Random Perturbations of Dynamical Systems, Birkhauser, Boston.

    Google Scholar 

  12. McGehee, R. (1988). Some metric properties of attractors with applications to computer simulations of dynamical systems. Preprint.

  13. Easton, R. W., and Meiss, J. D. (1993). Exit times and transport for symplectic twist maps. Chaos 4(2), 153-165.

    Google Scholar 

  14. Meyn, S., and Tweedie, R. (1993). Markov Chains and Stochastic Stability, Communications and Control Engineering Series, Springer Verlag London, London.

    Google Scholar 

  15. Miller, W. M. (1994). Stability and approximation of invariant measures for a class of nonexpanding transformations. Nonlin. Anal. Theory Methods Appl. 23, 1013-1025.

    Google Scholar 

  16. Miller, W. M., and Akin, E. (1999). Invariant measures for set valued dynamical systems. Trans. Am. Math. Soc. 351, 1203-1255.

    Google Scholar 

  17. Mischaikow, R., and Mrozek, M. (1998). Chaos in the Lorenz equations: A computer assisted proof. II. Details. Math. Comp. 67(223), 1023-1046.

    Google Scholar 

  18. Osipenko, G. Construction of Attractors and Filtrations, Banach Center Publications, 47, Polish Academy of Sciences, Warszawa, pp. 173-192.

  19. Resnick, S. J. (1992). Adventures in Stochastic Processes, Birkhauser, Boston, Basel, Berlin.

    Google Scholar 

  20. Robinson, C. (1995). Dynamical Systems: Stability, Symbolic Dynamics, and Chaos, CRC Press, Boca Raton, FL.

    Google Scholar 

  21. Shub, M., Tischler, D., and Williams, R. (1988). The Newtonian graph of a complex polynomial. SIAM J. Math. Anal. 19, 246-256.

    Google Scholar 

  22. Stuart, A. M., and Humphries, A. R. (1996). Dynamical Systems and Numerical Analysis, Cambridge University Press, New York.

    Google Scholar 

  23. Conley, C. (1978). Isolated Invariant Sets and the Morse Index, CBMS 38, American Mathematical Society.

Download references

Author information

Authors and Affiliations

  1. Mathematics and Computational Sciences Division, National Institute of Standards and Technology, Gaithersburg, Maryland, 20899

    Fern Y. Hunt

Authors
  1. Fern Y. Hunt
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Hunt, F.Y. Finite Precision Representation of the Conley Decomposition. Journal of Dynamics and Differential Equations 13, 87–105 (2001). https://doi.org/10.1023/A:1009092431497

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1023/A:1009092431497

Search

Navigation