Recent progresses on graphene-based artificial nanostructures: a perspective from scanning tunneling microscopy

Abstract

Graphene, a Dirac semimetal, exhibits the simplest lattice configuration and band structure in the world of two-dimensional materials. Due to its remarkable brevity and tunability, graphene becomes an ideal platform for studying the fundamental physics arising from the linear dispersion around the Dirac point, as well as for exploring symmetry-breaking orders in the flat band through playing with various artificial structures and external fields. In this review, we provide an overview of the nanoscale graphene model structures such as defects, quantum dots, strains, and superlattices in scanning tunneling microscopy measurements. Utilizing nanostructures in diverse dimensions, we present some behaviors of electrons near singularities of density of states from the perspective of scanning tunneling microscopy.

1 Introduction

Graphene is the starting point of two-dimensional (2D) materials, possessing spin, valley and sublattice degrees of freedom in an extremely simple lattice structure. Its stability and tunability enable us to play with various intrinsic degrees of freedom, understand interactions between them, and further investigate topology, magnetism and superconductivity. The Hamiltonian matrix of monolayer graphene in low energy can be written as [1, 2]:

$$\begin{aligned} H \simeq \begin{pmatrix} 0 & v_{F} \xi q e^{i\xi \theta _{\mathbf{q}}}\\ v_{F} \xi q e^{- i\xi \theta _{\mathbf{q}}} & 0 \end{pmatrix} . \end{aligned}$$

(1)

ξ is the valley index. $v_{F}$ is about $10^{6}\ \mathrm{m}/\mathrm{s}$. The eigenvectors are given by:

$$\begin{aligned} \begin{aligned}&\psi _{\pm, \mathbf{K}} ( \mathbf{k} ) = \frac{1}{\sqrt{2}} \begin{pmatrix} e^{- \frac{i \theta _{\mathbf{k}}}{2}}\\ \pm e^{\frac{i \theta _{\mathbf{k}}}{2}} \end{pmatrix}, \\ &\psi _{\pm, \mathbf{K}'} ( \mathbf{k} ) = \frac{1}{ \sqrt{2}} \begin{pmatrix} e^{\frac{i \theta _{\mathbf{k}}}{2}}\\ \pm e^{- \frac{i \theta _{\mathbf{k}}}{2}} \end{pmatrix}, \end{aligned} \end{aligned}$$

(2)

which is on the basis of A and B sublattices. ± corresponds to $\pi ^{*}$ and π bands. The linear dispersion and the pseudospin degrees of freedom are two essential features that give rise to many interesting phenomena, including the half-integral quantum Hall effect and Klein tunneling [3, 4].

The behavior of electrons can be modulated by a large number of nanostructures or periodic lattices, resulting in distinct responses in microscale measurements. However, what are the electronic properties of a single structure, or how does the local density of states (LDOS) varies spatially at the nanoscale? Scanning Tunneling Microscopy (STM) and Scanning Tunneling Spectroscopy (STS) can provide answers to these. STM and STS are precise tools for visualizing electron distribution at the atomic level with high energy resolution. Additionally, it provides the opportunity to construct atomic-scale structures and devices through tip-sample interactions. In this article, we focus on the different structures from the atomic level to hundreds of nanometers with the help of STM and discuss their electronic properties near singularities.

2 Atomic-scale defects

Graphene is generally non-magnetic, however, by introducing artificial defects through the electron or ion irradiation, magnetism can be induced in the sp electron system, which can extend several nanometers compared with the strongly localized d and f elements [5]. Two fundamental types of single-carbon defects are hydrogen chemisorption and single-atom vacancy. When a defect is created in the A sublattice, i.e., removing a $p_{z}$ orbital from the π graphene system, the $p_{z}$ orbitals of carbon atoms in the B sublattice will contribute to the quasilocalized states. The ground state of this spin system is given by $S =1/2\times \vert N_{A} - N_{B} \vert$, $N_{A}$ and $N_{B}$ are the number of $p_{z}$ orbital removed from each sublattice [6].

The electrons the in $p_{z}$ orbital of hydrogen chemisorption defects can result in strong Stoner ferromagnetism, with a magnetic moment of $1 u_{B}$ per defect. There is only a slight protrusion of the hydrogenated atom and a small displacement of the neighboring carbon atoms, thus the spin-density projection still remains symmetrical (Fig. 1(a)). Through STM experiments, it has been experimentally confirmed that the magnetic moment featured by 20 millielectron volt spin-split is visible at the Dirac point around the Fermi energy. The spin-polarized state is located on the sublattice opposite to the sublattice where the hydrogen atom is chemisorbed (Fig. 1(b)). By tuning the energy position of the impurity level in comparison to the Fermi level, it is feasible to switch it from a magnetic state to a nonmagnetic state (Fig. 1(c)) [7, 8].

Figure 1

Magnetism in the flat energy level. (a) DOS (left panel) and spin projection (right panel) of hydrogen chemisorption defects [5]. (b) Left panel: STM topography of a single H atom on monolayer graphene. Right panel: $dI / dV$ spectra measured on and off a H atom. The defect state shows spin splitting about 20 meV [7]. (c) $dI / dV$ spectra and DFT calculation of DOS on n-doped graphene. The splitting vanishes and transfers to a nonmagnetic state when it is far away from the Fermi energy [7]. (d) The DOS (left panel) and spin projection (right panel) of a single vacancy defect [5]. (e) The STM image of a single carbon vacancy defect and the corresponding STS spectrum. The spin-up and spin down are marked on the spectrum [10]. (f) Basic phase diagram of pseudo-gap Kondo effects as a function of chemical potential and Kondo coupling strength. The critical point separates subcritical and asymmetric strong-coupling regimes [11]

The concentration-dependent magnetic moment in vacancy defects system is about $1.15 u_{B}$ per defect. The localized $s p^{2}$ orbital (labeled by 1 in Fig. 1(d)), which results in dangling σ bonds, contributes $1 u_{B}$. The extended $p_{z}$ orbitals, which forms π band, around the dangling bond (labeled by 1′ in Fig. 1(d)) contribute fractional magnetic moments (Fig. 1(d)). The threefold symmetric states are broken as a result of the Jahn–Teller distortion brought by the reconstruction of two dangling bonds (Fig. 1(d) and (e)). In contrast to hydrogenated defects, the spin splitting in vacancy defects can still be observed above the Fermi energy (Fig. 1(e)), which is likely a consequence of the interaction between the non-orthogonal σ and π bands in an imperfect planar configuration. The dual contribution of magnetic moments of single vacancy defects offer the potential to manipulate the magnetism by changing the curvature of structures (Fig. 1(f)) [9–11].

STM is not only able to precisely identify the LDOS around defects but it can also be utilized to shape the nanostructures and carry out further in-situ investigations of nanostructures. By using an STM tip, hydrogen chemisorption defects can be precisely placed or removed from the A or B sublattices, thus enabling further studies about lattice-dependent physics, interactions between defects, as well as the formation of diverse patterns [12]. For a single vacancy defect, the van der Waals force between the tip and sample can tune the local curvature of the defect, resulting in various magnetic states such as ferromagnetic and quenched antiferromagnetic states [10]. Furthermore, the same idea is also applicable to the rough $\mathrm{SiO}_{2}$ substrates. Moreover, the increased LDOS beyond the Dirac point can screen the local moments induced by defects (Fig. 1(f)), thus resulting in the emergence of a Kondo peak in pseudo-gap systems [11, 13, 14]. With the help of tip manipulation, substrate engineering, and tunable carrier density, it is feasible to control and image different types of magnetic states at the atomic scale.

In addition to the magnetism induced by signal atom defects, the quasiparticle interference (QPI) around defects can offer an insight into the band structure and its topology, thus providing a complementary story to the LDOS [15]. Figure 2(a) illustrates the inter- and intravalley scattering processes in the momentum space for monolayer graphene system, wherein the momentum transfer vector at the iso-energy contour is $\boldsymbol{q}_{k} - \boldsymbol{q}_{k '} = \boldsymbol{q}_{k} - (-\boldsymbol{q}_{k} )$ [16]. Thus, the momentum transfer in this case is 2q, which is equivalent to the radius of the ring in Fourier transform (FFT) images [17, 18]. In this process, the transfer between opposite q for the same valley is destructive, as the π rotation of the pseudospin causes a π/2 phase shift in the wave function, thus leading to $\langle u_{\pm} ( K - q ) | u_{\pm} ( K + q ) \rangle =0$. As a result, the disorder-free system is only characterized by the intervalley scattering pattern (Fig. 2(b-g)), without any intravalley scattering information (Fig. 2(d)) [19–21]. From the relation between $E = e V_{b}$ versus q, the linear dispersion can be extracted as shown in Fig. 2(h).

Figure 2

Band structure and pseudospin imaged by QPI. (a) The intra-valley backscattering rotates the pseudospin by π. The inter-valley backscattering between q and −q momentum leads to the rotation of $2 \theta _{\boldsymbol{q}}$ of the pseudospin. (b) Monolayer graphene and its schematic diagram of 2D fast FFT. (c) The FFT of 100 × 100 nm² STM image taken at −4 mV with the number of pixels: 2048 × 2048 [17]. (d)–(g) The central region and three outer rings without and with a radius of $2 \boldsymbol{q}_{\boldsymbol{F}}$ [17]. (i) The relation between STM tip (magenta dot) and pseudospin rotation in the processes of inver-valley scattering [17]. (j) The theoretical density modulation of the phase image. (k)–(l) The density modulation on A and B sublattices. (a) and (i)–(l) are adapted from [2]

Recently, QPI has been investigating topological characteristics of the band structure by projecting phase-induced wave dislocations around a defect [2]. The Dirac point in monolayer graphene is a phase singularity, being the vertex center for the rotation of the pseudospin. As the wave function progresses through an adiabatic cycle that includes a Dirac point, it accumulates a gauge-invariant geometrical and topological phase, i.e., Berry phase:

$$\begin{aligned} \gamma = i {\varoint {}} {}_{\mathcal{C}_{\mathbf{K}}} \langle u_{\pm} | \nabla _{\mathbf{q}} u_{\pm} \rangle \cdot d \mathbf{q} = \frac{1}{2} {\varoint {}} {}_{\mathcal{C}_{\mathbf{K}}} \,\mathrm{d} ( \xi \theta _{\mathbf{q}} ) = \pi W_{\mathbf{K}}. \end{aligned}$$

(3)

The Berry curvature integral around a loop in momentum space is associated with the rotation of momentum and pseudospin winding $W_{\mathbf{K}}$ around the singularity. As depicted in Fig. 2(i), the STM tip, represented by the magenta dot with the position of cylindrical axis $( r, \theta _{r} )$, injects electrons into the system and these electrons are reflected by the potential from the defect. The direction of incoming electrons is $\theta _{\boldsymbol{q}} = \theta _{\boldsymbol{r}} - \pi$, and the direction of reflected electrons is $\theta _{-\boldsymbol{q}} = \theta _{\boldsymbol{r}} = \theta _{\boldsymbol{q}} + \pi$. So, by rotating a circle around a defect with an angle of $\theta _{\boldsymbol{r}} =2 \pi$ in real space, the momentum wave vector also shifts by $\theta _{\boldsymbol{q}} = \theta _{\boldsymbol{r}} =2 \pi$. The lock-in relation $\theta _{\mathrm{pseudo}} =2 \theta _{\boldsymbol{q}}$ between the pseudospin and the wave vector leads to the pseudospin rotating by $\theta _{\mathrm{pseudo}} =-2 \theta _{\boldsymbol{q}} =-2 \theta _{\boldsymbol{r}} = 4 \pi$, when the tip goes around the defect (Fig. 2(j)). One wavefront, which is the standing wave filtered from K and $K'$ points of the FFT image, corresponds to the phase of 2π. Therefore, this process can induce two additional wavefronts in real space. The two wavefronts represent the 4π rotation of pseudospin, 2π rotation of the momentum and π Berry phase of the system. Thus, it enables one to visualize the pseudospin rotation and directly measure the Berry phase without magnetic fields.

For the defect located on the A sublattice, the scattering process from the A sublattice around the tip to the A sublattice around the defect does not present a phase difference. No rotation of pseudospin is shown in the wave function thus no additional wavefront is visible in Fig. 2(k). When the scattering occurs between the A sublattice surrounding the tip and the B sublattice surrounding the defect, the phase difference between two sublattices (see Eq. (2)), characterized by the rotation of the pseudospin, leads to changes of the wavefront around the defect (Fig. 2(l)). Based on Green functions, the total density can be described by:

$$\begin{aligned} \delta \rho ={}& \delta \rho _{A} ( r, V_{b} ) \cos ( \Delta \mathbf{K} \cdot \mathbf{r} ) \\ &{}+ \xi \xi ' \delta \rho _{B} ( r, V_{b} ) \cos \bigl( \Delta \boldsymbol{K} \cdot \boldsymbol{r} - \bigl( \xi - \xi ' \bigr) \theta _{\boldsymbol{r}} \bigr) . \end{aligned}$$

(4)

$V_{b}$ is the sample bias and ξ is the index of each valley. Thus, from dislocations around the defect, one can obtain the pseudospin winding and the Berry phase in the graphene system through the QPI at a zero magnetic field [22, 23].

3 Nano-scale quantum dot

To form a functional qubit for the further quantum computer, the system must meet the DiVincenzo criteria [24], which includes the ability of initialization, manipulation and read out [25]. Electrons confined within a small island, i.e., quantum dots, can be initialized, manipulated, gated and measured with precision, thus satisfying all criteria. Artificial quantum dots have been well studied for spin and charge qubits in the pioneer GaAs semiconductor [26–28]. Nowadays, Si has become the widely used workhorse material, and graphene has been identified as a future material due to its weaker spin-orbit coupling and hyperfine interaction, which can reduce the decoherence caused by these interactions [29].

Several approaches for constructing nanoscale graphene quantum dots (GQDs) have been demonstrated in STM experiments, e.g., substrate engineering, tip potential, and tip-assisted substrate engineering. Through substrate engineering, quantum dots as small as 10 nm can be created. During high-temperature growth processes, the formation of GQDs can be attributed to the presence of Cu vacancy islands or self-organized sulfur islands (Fig. 3(a)) [30–32]. For this potential barrier formed by GQD, massless Dirac fermions can totally traverse the barrier at the normal angle, called Klein tunneling. If the electrons cross the potential at certain angles, they can be temporarily trapped and do the cyclotron motions as the electron around the nucleus, resulting in quasibound states in the STS spectra [30–32]. The energy difference of these quasibound states is equal and follows the equation: $E = \alpha \hslash v_{F} / R$. For monolayer graphene on hBN, it is difficult to shield the external electric field from the tip, thus resulting in the bending of the local band and the formation of an edge-free potential junction with a diameter of approximately 100 nm beneath the tip (Fig. 3(b)) [33]. The band bending changes depending on the sample bias and gate voltage, as a consequence of the varying screening effects around $\varepsilon _{\mathrm{n}} = \mu _{0} + e V_{\mathrm{b}}$ and external electric fields (Fig. 3(b)). Furthermore, by using STM tip pulses, the strong electric field penetrates the gated graphene into the insulator layer, causing field-induced ionization and charge diffusion in the hBN insulator [34–36]. A smooth junction with a diameter of about 200 nm, featuring by the well-defined radial quantum number and angular momentum quantum number can be created in this way (Fig. 3(c)) [37].

Figure 3

Generation and tuning of quasibound states in GQDs. (a) STS spectra taken at different locations on and off the dot. The first resonance $N_{1}$ is maximum at the center of the dot, whereas the subsequent high intensity peaks are shifted towards the edge. The final peak $N_{4}$ is maximum around the edge, thus exhibiting a douat distribution around the edge [30]. (b) Left panel: $dI / dV$map as the function of gate voltage. Right panel: $dI / dV$ spectra taken along the lines in the map at $V_{g}= 16$ V (red) and $V_{g}=-11$ V (blue). The peaks labeled 1”, 2”, 3” with smaller spacings correspond to WGMs at the energy $E = \mu _{0} + e V_{b}$, while the peaks labeled 1’, 2’, 3’, are the same WGMs probed at the Fermi level $e = \mu _{0}$. The resonance spacing of 40 mV is indicative of a cavity radius of 50 nm [33]. (c) Left panel: an illustration of the potential profile of a circular graphene resonator in a parabolic potential, scale bars, 100 nm. Right panel: the differential conductance map, obtained from graphene resonator, displaying $m = 1/2$ states at the center, while states with higher angular momentum are located away from the center [37]. (d) Left panel: a graphene and WSe₂ heterostructure quantum dot with a height of 0.8 nm and a width of 2.2 nm. Right panel: the calculated space-energy LDOS map for the dot with $\beta = 2.4, r_{0} = 9$ nm [40]. (e) Left panel: dual-gate configurations for graphene. The wave function distribution of the p-doped junction with an angular momentum of $m = 10$ is depicted on a blue silicon substrate. Right panel: the backgate voltage-dependent tip-induced potentials display the Coulomb-like potential, which is the source of ACSs (top panel), and a cavity-like potential, which is the cause of WGMs (bottom panel) [39]

Another type of confinement state in graphene is atomic collapse states (ACSs), which arises from a Coulomb-like potential with a large local charge Z [38–40]. The fine structure constant of the atom is $\alpha = \frac{e^{2}}{\hslash c} \approx \frac{1}{137}$, where c is the speed of light, ℏ is the reduced Planck constant. In graphene, the Fermi velocity is $v_{\mathrm{F}} = \frac{c}{300}$. Therefore, the fine structure constant is larger with $\frac{e^{2}}{\hslash v_{F}} \approx 2.5$. When the interaction strength, $\beta \equiv Z \alpha _{\mathrm{g}}$ (here $\alpha _{\mathrm{g}}$ is $\alpha ( \frac{c}{\kappa v_{\mathrm{F}}} )$,and κ is effective dielectric constant) is larger than the critical number 0.5, electrons tend to “collapse” into the nucleus due to the large charge attraction so that confinement states follow the exponential function: $E_{n} = \frac{\hslash v_{F} \beta}{ r_{0}} e^{- \frac{\pi}{7} n} + E_{D}$, where $\gamma = \sqrt{\beta ^{2} -( m +1/2 )^{2}}$, m is the orbital states, and $E_{D}$ is Dirac point.

This type of confinement can be achieved through substrate engineering and tip-induced band bending, similar to the one mentioned previously. For the electrostatic potential:

$$\begin{aligned} V_{\beta} ( r ) = \textstyle\begin{cases} \hslash v_{F} \frac{\beta}{r_{0}}, & r \leq r_{0},\\ \hslash v_{F} \frac{\beta}{r}, & r > r_{0}, \end{cases}\displaystyle \end{aligned}$$

(5)

with large cutoff radius $r_{0}$, the higher angular momentum states are hard to collapse into the center by the Coulomb potential, thus forming whispering gallery modes (WGMs) around the edge of dots. ACSs in the center of the island and the WGMs near the edge can coexist in GQDs (Fig. 3(d)). The Au-coated tip can create a large work function difference between the tip and graphene sample, generating a point charge-like deep and narrow potential (Fig. 3(e)). With a large ΔW, the tip-induced effective charge presents the effective interaction strength: $\beta _{\mathrm{eff}} = ( 1/ \hslash v_{\mathrm{F}} ) \sqrt{ ( 2 \int _{r_{r}}^{n} V ( r )^{2} r \, \mathrm{d} r / ( 1+2\ln ( R / r_{0} ) ) )}$, which can determine the ACSs or WGMs in this system. By changing the backgate voltage and filling electrons up to the charge neutrality point, the screening length is decreased and the upper part of the well is broadened, driving ACSs transform into WGMs in certain doping windows.

The confinement behaviors of GQDs are strongly related to the band topography, which can also demonstrate topological characteristics of the band structure. In monolayer graphene, the intrinsic singularity, i.e., Dirac point, allows one to tune the “static” Berry phase of graphene, which is characterized by the jump of ±m orbital states of confinement states (Fig. 4(a)) [37]. Intuitively, the Bohr–Sommerfeld quantization rule, associated with the energy level $E_{n}$, provides a basic understanding of novel resonances observed at low magnetic fields: $\frac{1}{\hslash} \varoint {}_{C_{R} ( E_{n} )} \boldsymbol{p} \cdot d \boldsymbol{q} =2 \pi ( n + \gamma - \frac{\varphi _{\mathrm{B}}}{2 \pi} )$, where q is the canonical coordinate; p is the momentum; ℏ is Planck’s constant divided by $2 \pi; \gamma$ is Maslov index; $\varphi _{\mathrm{B}}$ is the Berry phase accumulated in the loop. Orbital states can be derived from the semiclassical Hamiltonian $H = E_{n} = \boldsymbol{v}_{\mathrm{F}} \Pi + U ( r )$, wherein $U ( r )$ is the confinement potential and $\Pi = \boldsymbol{p} - e \boldsymbol{A}$ is the kinetic momentum. By changing the phase $\varphi _{\mathrm{B}}$, the energy level $E_{n}$ and orbital states will be changed correspondingly.

Figure 4

Band-related quantum confinement in GQDs. (a) Top panel: electron trajectories projected on the Dirac cone. Above the critical magnetic field $B_{c}$, the contour encloses the Dirac point, which induces the π Berry phase (red line). Below the critical point, it does not include the Dirac point, then the spectra do not change (blue line). The critical magnetic field can be expressed as $B_{c} = \frac{2 \hslash}{ \epsilon} \frac{m\kappa}{\varepsilon}$. Here, ε is the energy of the orbit, and $\varepsilon = 65$ mV is the energy relative to the position of Dirac point which corresponds to the $m =1/2, n =1$ state. κ is obtained from the potential $U ( r )= \kappa r^{2}$, which is around 10 $\mathrm{eV}/ \mathrm{m}^{2}$. Bottom panel: Berry phase induced jumping of $m =\pm 1/2$ states for the $n =4$ resonance level [37]. (b) Top panel: Berry curvature in momentum space for $K'$ valley in a gapped bilayer graphene system. The contours are the electron trajectories in different magnetic fields. Bottom panel: valley splitting of ($K, n, m$) in a gapped bilayer GQD. The energy levels are $( K,0,1), ( K',0,-1 )$ and ($K,1,1$), respectively. Solid spheres are experimental data and colored lines are theoretical results [49, 50]. (c) Top panel: the low-energy band structure of the ABA trilayer graphene in the absence of external electric fields. The blue bands represent effective monolayer bands. The semitransparent red bands represent effective bilayer bands. The yellow arrows indicate the orientations of self-rotating wave packets, while the white arrows indicate directions of topological magnetic moments from self-rotating wave packets. Bottom panel: large $g_{v}$ factor as the function of the monolayer graphene gap. The value of the gap obtained from the energy spacing of $LL_{+}$ and $LL_{-}$ in 0.8 T [51]

Without magnetic fields, the momentum space trajectory does not enclose the Dirac point, so the Berry phase $\varphi _{\mathrm{B}}$ is 0 and ±m orbital states remain as time-reversal states. When the magnetic field is activated, the momentum space trajectory encloses the Dirac point, and Berry phase $\varphi _{\mathrm{B}}$ changes from 0 to π. The +m and −m orbitals experience distinct Lorentz forces, thereby resulting in the lifting of orbital degeneracy in GQDs. More rigorously, the Einstein–Brillouin Keller quantization (EBK) enables the visualization of trajectories along orbits. This is done by considering $\varoint {} \boldsymbol{p} \cdot d \boldsymbol{q}$ along two distinct loops on a torus, namely $C_{R}$ and $C_{\theta}$. The trajectory along $C_{\theta}$ yields the half-integer quantization of angular momentum, while the movement along $C_{R}$ determines the energy level for a given angular momentum. The phase term is determined by the winding number Π around the Dirac point. If it does not enclose the origin, the Berry phase will be zero. Above the $B_{c}$, the magnetic field shifts the trajectory, resulting in the sign change for Π along the $C_{R}$, which consequently leads to a Berry phase change to π, thus shifting the energy level in GQDs (Fig. 4(a)) [41].

Bilayer graphene offers more tunable parameters, such as a displacement field-dependent bandgap and the valley degree of freedom, allowing for the better confinement of electrons and the generation of valley qubits with longer lifetime [42–46]. We focus on the $C_{R}$ term of the trajectory in following discussions. Different from the monolayer graphene, Berry curvature of gapped bilayer graphene is characterized by a ring-shaped distribution around the saddle point in each valley (top panel of Fig. 4 (b)), rather than a single singularity. The movement of electrons results in continuous changes of the integral of Berry curvature along the loop, i.e., the Berry phase. Moreover, two valleys exhibit the opposite Berry curvature, and the effective Lorentz force is in opposite directions for ±m in K and $K'$ valleys, respectively. This leads to the reversed evolution of $( K, n, m )$ and $( K', n, m' )$ states under magnetic fields, so the ground states $( K,0,1 ), ( K',0,-1 )$ gradually separate in STS spectra (bottom panel of Fig. 4(b)). This Berry phase-induced valley polarization has also been reported in higher confinement energy levels under small magnetic fields [47]. Similarly, the self-rotation of the wave packet shows topological magnetic moments in the effective gapped monolayer band in trilayer ABA system. Magnetic moments of electrons in two valleys $\boldsymbol{M} = \boldsymbol{z} \tau M_{z}$ will couple with magnetic fields by $2 | \boldsymbol{B} \cdot \boldsymbol{M} |$, giving rise to a large valley splitting with $g_{\nu}$ about 1050 (Fig. 4(c)). From above, we can know that the singularity of band structures, Berry curvature, orbital magnetic moments, and Berry phase can be revealed by confinement states in p-n junctions, thereby providing the potential for future long-lifetime qubits based on orbitals and valleys [48].

In quantum dot systems, the interaction of wave functions is highly important and leads to a plethora of unexpected results [52–56]. For instance, for relativistic artificial molecules in a double GQD system (Fig. 5(a)), the lowest angular momentum state (low energy) at smaller oblique incidence angles exhibits a maximum in the center of GQDs. It is not confined as effectively as higher angular momentum states. Consequently, it will propagate outside the dot and interact with other quantum dots nearby, resulting in strong coupling states as demonstrated in the right panel of Fig. 5(a). The coupled confined states split into a bonding orbital with lower energy and an antibonding orbital with higher energy, which decreases with the increasing of angular momentum or energy due to better confinements [57]. Analogously, in an elliptical GQD, two ACSs also can be viewed as a bonding state ($N_{1}$) and an antibonding state ($N_{2}$), as shown in Fig. 5(b). The bonding state is localized at the center of the dot, while the antibonding state exists at the end of the potential. With the increasing of λ, i.e., the potential profile, the $N_{1}$ ACS evolves into the first ACS of the elliptical dot, while the $N_{2}$ ACS transforms into the WGM of the elliptical dot. ACSs, corresponding to relatively small orbital quantum numbers, tend to be drawn inward towards the center of the GQD before being released. On the other hand, WGMs are quasi-trapped due to oblique incidences. Thus, despite the different origins of ACSs and WGMs, i.e., Klein tunneling and scattering, there is a deep and close relationship between them that can lead to one type evolving into another.

Figure 5

Visualizing interactions of the electron wave function assisted by GQDs. (a) Left panel: the STM image of double GQDs structures. Right panel: LDOS map of the first quasibound state along the dashed line of the left panel [57]. (b) Calculated LDOS maps along Y axis of an elliptical Coulomb potential. Middle and bottom panels are quasibound states $N_{1}$ and $N_{2}$ [61]. (c) A sketch of a tip-induced movable quantum dot under magnetic fields. (d) STS spectra taken at different stacking regions. The shaded parts are in the same orbital and blue dots indicate valley transitions. The energy spacing here is estimated by $\Delta V = E_{\mathrm{C}} / ( e \alpha _{\mathrm{tip}} )$. $E_{\mathrm{C}} = e^{2} / C_{\Sigma}, \alpha _{\mathrm{tip}} = C_{\mathrm{tip}} / C_{\Sigma}$, where$C_{\Sigma}$ includes the capacitance between the tip and sample $C_{\mathrm{tip}}$, the capacitance between the dot and the backgate, and the capacitance between the dot and surrounding graphene. (e) Maps of valley and spin gaps deduced by $E_{\mathrm{add}}^{n} ( \boldsymbol{r} )= e\eta \mathrm{V}_{P_{n} +1} ( \boldsymbol{r} )- V_{P_{n}} ( \boldsymbol{r} )$. Here, η is the coefficient of the tip gating, and $V_{P_{n}}$ is the peak position in STS spectra. (c)-(e) are adapted from [59]. (f) Top panel: $dI / dV$ map as a function of $V_{b}$ at 4 T. Bottom panel: the x-y slice at $V_{b} = 6$ mV, which shows the compressible disk from different LLs. (g) Simulated LDOS map with or without the e-e interaction at 3 T. The e-e interaction is given by $V_{\mathrm{ee}} ( r )= \frac{\hat{e}^{2}}{r}, \hat{e}^{2} = \frac{e^{2}}{4 \pi \epsilon _{0} \kappa _{\mathrm{ave}}}$ with $\kappa _{\mathrm{ave}}$ an average dielectric constant and $\epsilon _{0}$ the vacuum permittivity. (f)–(g) are adapted from [60]

Under external magnetic fields, the Coulomb interaction in GQDs can help one see the spin and valley splitting in the quantum Hall regime (Fig. 5(c)). By utilizing the electric field of STM tips, the Landau Level (LLs) underneath the tip can be displaced into the gaps of surrounding LLs. Thereby it creates an edge-free, movable quantum dot that can protect the spin and valley symmetries without requiring physical edges. In the graphene-hBN alignment system, GQDs exhibit a large Coulomb interaction $E_{C} = e^{2} / C_{\Sigma}$ due to the small capacitance. The quadruplets of charging peaks in a single orbital quantum number can be divided into two doublets (Fig. 5(d)), which is attributed to the lattice potential-induced valley splitting in this system. By tracking the additional energy maps, the distribution of spin and valley splitting can be imaged at the superlattice scale (Fig. 5(e)) [58, 59]. Furthermore, with the ionized impurities in hBN, a position-fixed quantum dot can be created (Fig. 5(g)). When the quantum Hall regime is turned on, the LL plateaus form and show up as bright rings on the map (Fig. 5(f)). The wedding cake-like distribution is owing to the interplay between LLs and electron interactions. The Coulomb interaction causes the LLs to move to a lower energy level and become flattened in the central region, as shown in Fig. 5(g) [60]. The magnetic field-related interaction provides a new platform for exploring novel quantum phases in GQD systems.

4 Single-to-periodic strained structures

Non-uniform strain can produce pseudo-magnetic fields (PMFs), which provide a new approach to mimic real magnetic fields and generate Landau quantization at the zero magnetic field (Fig. 6(a) and (b)) [62–66]. The two-dimensional strain field $u_{ij} ( x, y )$ can create a gauge field [63]:

$$\begin{aligned} \boldsymbol{A}_{\mathrm{ps}} = \frac{\beta}{a} \begin{pmatrix} u_{xx} - u_{yy}\\ -2 u_{xy} \end{pmatrix}, \end{aligned}$$

(6)

where a is the lattice constant, $\beta =-\partial \ln t /\partial \ln a \approx 2$ and t is the nearest-neighbour hopping parameter. Using polar coordinates, gauge field can be written as:

$$\begin{aligned} \begin{aligned} A_{r} ={}& \frac{\beta}{a} \biggl[ \biggl( \frac{\partial u_{r}}{\partial r} - \frac{u_{r}}{r} - \frac{1}{r} \frac{\partial u_{\theta}}{\partial \theta} \biggr) \mathrm{co} \mathrm{s} 3 \theta \\ &{}+ \biggl( - \frac{1}{r} \frac{\partial u_{r}}{\partial \theta} + \frac{u_{\theta}}{r} - \frac{\partial u_{\theta}}{\partial r} \biggr) \mathrm{si} \mathrm{n} 3 \theta \biggr], \\ A_{\theta} ={}& \frac{\beta}{a} \biggl[ \biggl( - \frac{\partial u_{\theta}}{ \partial r} + \frac{u_{\theta}}{r} - \frac{1}{r} \frac{\partial u_{r}}{ \partial \theta} \biggr) \mathrm{co} \mathrm{s} 3 \theta \\ &{}+ \biggl( \frac{1}{r} \frac{\partial u_{\theta}}{\partial \theta} + \frac{u_{r}}{r} - \frac{\partial u_{r}}{\partial r} \biggr) \mathrm{si} \mathrm{n} 3 \theta \biggr]. \end{aligned} \end{aligned}$$

(7)

Figure 6

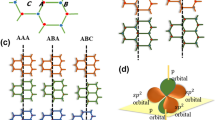
Traditional strain engineering techniques of zero-dimensional, 1D and 2D structures. (a) Top panel: the deformed graphene (red) which can generate uniform PMFs. The blue one is the original graphene film. Bottom panel: graphene lattice orientations with respect to the strain [63]. (b) Top panel: distribution of PMFs in stretching graphene. Bottom panel: DOS of PMF at 7 T (red), real LLs at 10 T (blue). The black curve is the DOS with no field and no strain. The peak at zero energy is come from the zigzag edge [63]. (c) Top panel: monolayer graphene on Pt (111) substrate. Bottom panel: zoom in STM topography of a triangular nanobubble [67]. (d) STS spectra taken on the top of the bubble with 8 different points. The PLLs are marked with blue dots [67]. (e) Top panel: quasi-periodic ripples formed in the 1D channel and its corresponding STS spectra taken at different positions of the strain. Bottom panel: STS maps around zero energy peaks [68]. (f) Top panel: the topography of periodic ripples around the edge. Bottom panel: stretching of the bond in real space and PMFs distribution near the interface. The valley-polarized snake states are marked. It comes from the valley-dependent PMFs, which associate different directions of electron trajectories under opposite PMFs [69]. (g) Top panel: shear components of TBGs with angles of 1.03^∘ and 0.14^∘. The red and blue arrows are two shear components respectively [73]. Bottom panel: a STM image of the TBG with angle of 0.48^∘. STS spectra are taken at AA, AB and BA regions and marked in the corresponding areas in the STM image [72]

The PMF is then described by:

$$\begin{aligned} B_{\mathrm{S}} = \frac{\partial A_{y}}{\partial x} - \frac{\partial A_{x}}{ \partial y} = \frac{1}{r} \frac{\partial A_{r}}{\partial \theta} - \frac{\partial A_{\theta}}{\partial r} - \frac{A_{\theta}}{r} . \end{aligned}$$

(8)

Therefore, the uniform$B_{\mathrm{S}} =8 \beta c / a$ (given in units $\hslash / e \equiv 1$, c is a constant) can be generated by the displacements:

$$\begin{aligned} u_{r} = c r^{2} \mathrm{si} \mathrm{n} 3 \theta, \qquad u_{\theta} = c r^{2} \mathrm{co} \mathrm{s} 3 \theta. \end{aligned}$$

(9)

From above, we can see that PMFs can only be created by non-uniform shear strain which can result in a non-zero $\nabla \times \boldsymbol{A}_{\mathrm{ps}}$ and the isotropic strain or uniform strain yields the zero PMF.

PMFs generated by nanoscale strain structures can up to hundreds of Tesla beyond the laboratory limit, offering an approach to explore the carrier density at inaccessible high magnetic fields. Therefore, many studies have developed a variety of methods to construct local nanostructures and explored them by using STM. Initially, graphene grown on platinum (111) exhibits plenty of intrinsic triangular nanobubbles (Fig. 6(c)), ranging from 4 nm to 10 nm, in accordance with the $C_{3 v}$ lattice symmetry of graphene (Fig. 6(c)). The spectra presented LLs-like peaks can be fitted by (Fig. 6(d)):

$$\begin{aligned} E_{n} = \operatorname{sgn} ( n ) \hslash \omega _{\mathrm{c}} \sqrt{n} + E_{\mathrm{D}},\quad \omega _{\mathrm{c}} = \sqrt {2 e\hslash v_{\mathrm{F}}^{2} B_{\mathrm{s}}} , \end{aligned}$$

(10)

where $E_{n}$ is the energy of the nth LL with respect to the Fermi level, ω is the cyclotron resonance frequency, and $v_{F}$ is the Fermi velocity. The magnetic flux in one bubble can be described by the relation: $\Phi = ( \beta h^{2} // a ) \Phi _{0}$, where h is the height, l is the width, a is the C-C bond length, and $\Phi _{0}$ is the quantum of flux. $\beta =\partial \log ( t )/\partial \log ( a )$ is about 2 to 3, which is related to the change of the hopping magnitude between the nearest neighbor carbon atoms [67]. When bubbles are arranged into an array, zeroth Pseudo Landau Levels (PLLs) in quasi-periodic ripples can couple with each other, resulting in the splitting of zeroth PLLs and a serpentine LDOS pattern along the one-dimensional (1D) channel (Fig. 6 (e)) [68]. Substrate engineering can be used to create periodic ripples as depicted in Fig. 6(f). This strain deforms the hexagonal lattice in both real and reciprocal space, resulting in the shift of the Dirac cones at K and $K'$ points into opposite directions ($\delta \mathrm{k}\equiv \mathrm{eA}/\mathrm{c}$), which causes the PMFs have opposite signs in two valleys (Fig. 6(f)). Moreover, due to incomplete screening of the pseudo-electric field, there is a redistribution of electrons and an in-plane electric field is formed, resulting in a new manifestation of pseudo-(E, B) effects [69]. The uniform periodic strain can be expanded into 2D form, i.e., a moiré pattern (Fig. 6 (g)). In the twisted bilayer graphene (TBG) with small twist angle, the strain is uniform and strong enough to confine massive Dirac electrons into circularly localized PLLs [70–72]. The PMFs are opposite in two valleys, resulting in clockwise and counterclockwise valley vertex current around the AA regions. Thus, low-angle TBG can be a suitable platform for quantum anomalous Hall effect and valley Hall effect at the moiré scale [72].

After this, more and more techniques have been developed to control strained structures at the nanoscale. For instance, tip-pulse induced bubbles on Ge substrate [74], van der Waals force induced graphene lifting [75], specific shape arrays with lithography [76, 77], etc. When graphene is grown on Ge (110) by chemical vapor deposition, the surface is terminated with Ge-H bond but with low Ge-H bond energy. Thus, the hydrogen atoms readily desorb when a local negative bias stimulus is applied. As can be seen in Fig. 7(a) (top panel), after the AFM/STM tip negative bias, bubbles ranging from tens to hundreds of nanometers emerge (middle panel). Furthermore, STS spectra reveal an unusual six-fold $B_{s}$ because the PMFs with three-fold symmetry have the sign alternating between two consecutive maxima (bottom panel of Fig. 7(a)) [74, 78]. By positioning the STM tip very close to the sample, the van der Waals force between them can drag the sample up, thus allowing for the creation of hundreds to thousands of PMFs at the atomic scale, as illustrated in Fig. 7(b) [75]. Utilizing lithography, well-defined arrays can be managed and tuned, and the spatial distribution of PMFs is studied precisely with STS (Fig. 7(c)) [76]. By placing a van der Waals heterostructure on these arrays, the distorted moiré pattern of graphene and hBN acts as an effective magnifying glass, magnifying the strain-induced lattice distortion by a factor of 20 (Fig. 7(d)). From the above, we can see that techniques are transitioning to controllable approaches and going ahead into devices gradually.

Figure 7

Techniques development in strain engineering. (a) Top panel: schematic of AFM tip-induced bubbles. Middle panel: corral patterns created by local stimulus with negative bias of AFM tip. Bottom panel: three-fold symmetric PMFs as a function of rotational angles taken from the STS data [74]. (b) Top left panel: the attractive van der Waals force between the STM tip and sample results in a Gaussian deformation of the graphene on SiO₂. Top right panel: STM images of the same graphene area with different tunneling currents. Bottom panel: LDOS contrast as a function of lifting amplitude and its corresponding PMFs $B_{s}$ [75]. (c) Top panel: an AFM image of two closed Pd tetrahedrons with graphene on top of it. Middle panel: the PMF map calculated from the topography with the same region of the top panel. Bottom panel: SEM image of monolayer graphene on rectangular arrays on SiO₂/Si substrate [76]. (d) Top panel: schematic of the transfer processes of graphene on Au pillars. Bottom panel: strain induced distorted moiré pattern and its corresponding FFT image with the same region [77]

The remarkable feature of PMFs is not only the large and tunable magnetic fields, but also its potential to serve as a valley filter and the correlation system. The strain-induced PMF preserves the time-reversal symmetry and the directions are reversed at two distinct Dirac points [79, 80], which can lead to a series of phenomena, such as valley-polarized propagation, valley-polarized LLs, and sublattice-polarized states, etc. For example, the opposite sign of PMFs in two valleys can be used as a valley filter and propagator in the device with a periodic design (Fig. 8(a)). When Dirac fermions are incident perpendicularly to the parallel channels with PMFs, the electrons in K and $K'$ valley can be spatially separated due to opposite electron trajectories under opposite PMFs, i.e., moving electrons in clockwise and counterclockwise circles in the momentum space. The lateral separation can increase as the number of wrinkles according to the simulation (top panel of Fig. 8(a)). Similarly, PMFs can also be used as a topological channel to collimate fermions with some specific angles (bottom panel of Fig. 8(a)) [76]. By combining real magnetic fields and PMFs, valley polarization can be distinctly identified and tuned (Fig. 8(b)) [81]. The effective magnetic field for K valley is $B - B_{S}$, and for $K'$ valley is $B + B_{S}$ [79, 80], and the energy spacing of two valleys for the nth LL is:

$$\begin{aligned} \begin{aligned} &\Delta _{n} = \sqrt{2 e \hslash v_{F}^{2} \mathrm{n} ( B + B_{S} )} - \sqrt{2 e\hslash v_{F}^{2} \mathrm{n} ( B - B_{S} )}, \\ &\quad n =\ldots -2,-1,1,2,\ldots. \end{aligned} \end{aligned}$$

(11)

Figure 8

Valley polarization and emergence of correlated states in strained structures. (a) Top panel: trajectories of K and $K'$ electrons for an incident angle of with respect to strain-induced parallel PMFs. Bottom panel: trajectories of K valley electrons incident at an angle parallel to the channels. Parallel wrinkles act as a valley propagator, showing the collimation of valley-polarized states [72]. (b) Top panel: Landau quantization in the monolayer graphene under both PMFs and external magnetic fields. Here the valley degeneracy of the $L L_{-1}$ is lifted, showing valley-polarized LLs [80]. Bottom panel: tunneling spectra obtained from three different positions of the right panel. The LLs index for each spectrum are identified. The STM strain structure with a transition region marked with the dashed line. Around this line, the valley splitting and the PLL is zero [85]. (c) Top panel: electron transmission is influenced by inhomogeneous magnetic fields B, inhomogeneous pseudomagnetic fields $B_{S}$, and the combination of inhomogeneous PMF and homogeneous external magnetic fields. PMFs host opposite signs in the two valleys, and the magnetic length in two valleys under PMFs is denoted as $l_{B}$ ($l_{B'}$). Bottom panel: the experimental and calculated maps of pseudomagnetic confinement in relation to magnetic fields. The black and red dashed lines signify the bound states for K and $K '$ valleys, while the green dashed lines represent the emergent $LL_{0}$. Additionally, the dark blue lines indicate the two lowest bound states near the Dirac point [82]. (d) STM images of buckled graphene on NbSe₂ and hBN substrate. (e) Top panel: STS spectra taken at crest and trough regions. Bottom panel: simulated LDOS of energy spectra versus PMFs at A and B lattices. (f) Top panel: LDOS spectra as a function of positions. Bottom panel: the changes of $B_{\mathrm{PMF}} / B$ at the same region with the top panel. (g) STS spectra as a function of gate voltage, showing pseudo-gaps around the partially filled bands. (d)–(g) are adapted from [83]

The schematic of the valley splitting is shown in the top panel of Fig. 8(b). Therefore, in STS spectra, one can observe the valley splitting of LL₋₁ under magnetic fields (bottom panel of Fig. 8(b)). By tuning the strain from compressive to strain-free and then to compressive region, the valley splitting of LL₋₁ becomes zero and splits again along the strain (Fig. 8(b)), demonstrating a valley and PMFs switch generated by the strain structure. Furthermore, inhomogeneous PMFs in strained graphene can introduce valley-polarized confinement states of massless Dirac fermions under real magnetic fields (top panel of Fig. 8(c)) [82]. By combining real magnetic fields and PMFs, the total effective magnetic fields in two valleys become imbalanced; the K valley has a higher effective magnetic field, which leads to a shorter effective magnetic confined length and a larger energy spacing, and the $K'$ valley has a lower magnetic field, resulting in a longer effective magnetic confined length and a smaller energy spacing, thus causing a valley splitting in pseudomagnetic confinement regions (bottom panel of Fig. 8(c)). PMFs also play an important role in pseudospin degree of freedom. Taking STS spectra on a 2D periodic heterostructure (Fig. 8(d)), the $dI/dV$ spectra show spatial variation and sublattice polarization, as shown in Fig. 8(e). The sublattice polarization can be understood by squaring the Dirac Hamiltonian:

$$\begin{aligned} \begin{aligned} & E^{2} \Psi ^{\mathrm{A}} = v_{\mathrm{F}}^{2} \bigl[ \boldsymbol{\pi}^{2} + e\hslash B_{\mathrm{ps}} \bigr] \Psi ^{\mathrm{A}}, \\ & E^{2} \Psi ^{\mathrm{B}} = v_{\mathrm{F}}^{2} \bigl[ \boldsymbol{\pi}^{2} - e\hslash B_{\mathrm{ps}} \bigr] \Psi ^{\mathrm{B}}, \end{aligned} \end{aligned}$$

(12)

where $\Psi ^{\mathrm{A}/\mathrm{B}}$ is the wave function amplitude on A and B sublattices,$\boldsymbol{\pi} = ( \boldsymbol{p} \pm e \boldsymbol{A}_{\mathrm{ps}} )$ is the canonical momentum, $B_{\mathrm{ps}} = ( \nabla \times \boldsymbol{A}_{\mathrm{ps}} )$ is PMF, and ± denotes two different valleys. The coupling of PMFs to the graphene sublattice pseudospin shifts the energy of states on the A and B sublattices, resulting in sublattice polarization in real space (bottom panel of Fig. 8(e)) [75]. In this system, PMFs are changed with respect to positions and the gaps between PLLs lead to magnetic confinement states, featuring equal energy spacings between PLLs (Fig. 8(f)). More interestingly, the $dI / dV$ spectra show pseudo-gaps and the flat bands around the Fermi energy, which is a signal of correlation in this strain system (Fig. 8(g)) [83]. Therefore, PMFs in strained structures can be a promising direction for exploring the valley degree of freedom and electron correlation. Symmetry-breaking states, valley Hall effects, and superconductivity may become next steps to be investigated in well-defined strain structures [84].

5 Conclusion and outlook

In this paper, we introduce the electronic properties of nanostructures studied in STM measurements. These nanostructures present extraordinary properties from their various energy states, e.g., flat energy levels of vacancies or hydrogen absorptions, quasibound states in GDQs, and zero-modes in strained structures, which can interact with the other nanostructures, external fields and reflect the intrinsic band topology of graphene. These nanostructures are simple and can be easily studied, thus providing well-defined model systems to investigate the fundamental physics.

The emergence of 2D twistronics has provided a broader avenue for exploring the electronic characteristics of nanostructures. The combination of different physics, such as correlated states, superconductivity, and magnetism in one material, has captured the attention of researchers to work on it. These nanostructures also play an important role in the twisted system, influencing their electronic properties and reflecting their ground states. The development of novel scanning probe techniques is also on the rise. Scanning probe tip has the capacity to detect not only current but also a range of other physical quantities, such as force, magnetic field, electric field, temperature, capacitance and so on. The scanning probe can perform a variety of functions, including monitoring, nano-fabrication, and serving as a platform for the nanodevice placed on it. The integration of various physical quantities and materials on the probe represents a promising direction that is expected to yield a wealth of new intriguing information about materials. More studies and techniques are needed to be developed to answer questions about the mechanism of different electronic properties in graphene nanostructures and whether they can eventually facilitate the realization of quantum computing in certain forms in the future.