1 Introduction

Optical micromanipulation techniques, invented by Ashkin, have been widely applied to capture, transport, and analyze tiny objects in various research fields [1,2,3]. In principle, when target objects of optical manipulation are illuminated with parallel light, they exhibit linear translational motions because that light carries linear momenta; microparticles will be blown off by dissipative force (the sum of scattering and absorption forces) along the propagation direction of the light [4, 5]. On the other hand, when laser light is tightly focused to a dielectric microparticle using a microscope objective with a high numerical aperture, optical force (gradient force) originating from the momentum change of photons via the refraction at the surface of the particle pushes the particle to the focal point of the laser light. Consequently, the particle is stably trapped at the focal point by the gradient force; this is commonly known as optical tweezers [6]. Thus, fundamental motions in the optical manipulation are (i) pushing along the propagation axis by dissipative force and (ii) trapping at a specific location by gradient force. In other words, catching or one-way transportation of microparticles are mainly achieved using these two forces and, reciprocal or round-trip motions are not so common in optical manipulation using steady-state light field. In our previous papers, we have demonstrated one-dimensionally reciprocal motion of an optically trapped single-polymer particle through the switching of absorption force using photochromic reactions of organic compounds incorporated in the particle [7, 8]. In line with these previous studies, we aim to realize orbital motions of microparticles in stationary optical field in the present work. The two-dimensionally orbital rotation will provide a new degree of freedom in the optical manipulation because only linear motions have mainly been induced by the linear momenta of photons; the achievement will greatly assist further development of sophisticated optical manipulation techniques.

In the present work, we achieved the orbital rotation of microparticles using (i) non-coaxially counter-propagating optical field and (ii) environmental disturbance (i.e., Brownian motion). We constructed a non-coaxial counter-propagating optical tweezers with an axis-to-axis interval of a few micrometers (Scheme 1); the beam 1 propagates from the left to the right while the beam 2 propagates from the right to the left as shown in Scheme 1a. The two lasers possess orthogonally linear polarizations. One may guess that a microparticle exhibit orbital rotation with this setup because the scattering force of the beam 1 pushes the microparticle from the left to the right, and the scattering force of the beam 2 can send it back from the right to the left; however, no orbital rotation occurs only with this setup. Due to the symmetry in the optical field of the counter-propagating lasers, the microparticle will be stably trapped at some specific locations where the sum of total optical forces (gradient and dissipative forces) is zero.

Scheme 1
scheme 1

A Microparticle escaping from an optical-trapping potential of non-coaxially counter-propagating laser beams with the help of thermal fluctuation

Full size image

The environmental disturbance plays a major role for realization of the orbital rotation. The optical forces acting on tiny objects are essentially small; they are in the order of femtonewton to piconewton [9,10,11]. These forces are comparable with thermal fluctuation in solution at room temperatures. As already described above, a microparticle is stably trapped at some local minima in the trapping potential, when only the optical field is considered (i.e. optical manipulation in vacuum). In the solution, however, microparticles exhibit continuous orbital rotation in the circular optical field, because they can climb over the shallow local potential minima thanks to the Brownian motion in solution at the room temperature, as represented in Scheme 1b. We have demonstrated in the present report the above idea, “Brownian motion-mediated orbital rotation of microparticles in the stationary and the symmetry trapping potential”, both in numerical simulations and experiments. This idea differs from optical manipulation methods for orbital rotation of tiny objects using spatial light modulators [12] or angular momenta of photons [13, 14], because only linearly polarized stationary Gaussian beams have been used.

1.1 Numerical simulations on Brownian dynamics

To verify our idea for the orbital rotation, we performed numerical simulations on Brownian motion in the presence of the optical forces for a single microparticle in solution using Brownian dynamics simulation (BDS) developed by Ermak and McCammon [15]. The simulations are based on the following equations:

$${\varvec{r}}\left(t+\mathrm{d}t\right)={\varvec{r}}\left(t\right)+\frac{1}{\xi }\mathrm{d}t\bullet {\varvec{f}}\left({\varvec{r}}(t)\right)+\Delta {{\varvec{r}}}^{B}$$
(1)

Here, \({\varvec{r}}\left(t\right)\) is the position of a particle at time \(t\), \(dt\) is a short time-step, \(\xi\) is the viscous drag expressed by \(\xi =6\pi \eta a\) with the Stokes radius of particle, \(a\), and the viscosity of medium, \(\eta\), \({\varvec{f}}\left({\varvec{r}}(t)\right)\) is the external force acting on the particle at time \(t\) and position \({\varvec{r}}\), and \(\Delta {{\varvec{r}}}^{B}\) is displacement during \(dt\) by Brownian motion. Each component of the random displacement \((\Delta {x}^{B},\Delta {y}^{B},\Delta {z}^{B})\) at a temperature \(T\) satisfies the following relations:

$$\left\langle \Delta {x}^{B}\right\rangle =\left\langle \Delta {y}^{B}\right\rangle =\left\langle \Delta {z}^{B}\right\rangle =0$$
(2)
$$\left\langle {\left(\Delta {x}^{B}\right)}^{2}\right\rangle = \left\langle {\left(\Delta {y}^{B}\right)}^{2}\right\rangle = \left\langle \Delta {\left(\Delta {z}^{B}\right)}^{2}\right\rangle =\frac{2{\mathrm{k}}_{\mathrm{B}}\mathrm{Td}t}{\upxi }$$
(3)

Here, \({k}_{B}\) is the Boltzmann constant. In the BDS each component of the random displacement, \(\Delta {i}^{B} (i=x, y, or z)\), obeys the following normal distribution:

$$\rho \left(\Delta {i}^{B}\right)=\sqrt{\frac{\xi }{4\pi {k}_{B}T\mathrm{d}t}} \mathrm{exp}\left\{-\frac{\xi }{4{k}_{B}T\mathrm{d}t}{\left(\Delta {i}^{B}\right)}^{2}\right\}$$
(4)

The light intensity distributions of the two non-coaxially counter-propagating beams along the z-axis, \({I}_{\pm }\left(x, y, z\right)\), are expressed by Eq. 5, where the subscripts + and – correspond to beams 1 and 2 in Fig. 1a, respectively:

$${I}_{\pm }\left(x,y, z\right)=\frac{2P}{\pi {w\left(z\right)}^{2}}\mathrm{exp}\left\{-\frac{{\left(x\pm h\right)}^{2}+{y}^{2}}{{w\left(z\right)}^{2}}\right\}$$
(5)
$$w\left(z\right)={w}_{0}\sqrt{1+{\left(\frac{\lambda z}{\pi {{w}_{0}}^{2}}\right)}^{2}}$$
(6)

Here, \(P\) is the total incident laser power, \({w}_{0}\) and \(\lambda\) are, respectively, the beam waist and wavelength of the incident laser, \(h\) is the half distance between the optical axis of two counter-propagating laser beams on the xz-plane. To calculate the optical forces acting on the microparticle, we assumed an optical field created by the non-coaxial counter-propagating laser beams separated by \(2h\) between the beam axes, as shown in Fig. 1a. One can clearly see the symmetric optical fields created by the two counter-propagating Gaussian beams.

Fig. 1
figure 1

a Schematic illustration of the optical setup for non-coaxially counter-propagating laser beams used in the present study. b The detailed optical configuration under the up-light optical microscope for the non-coaxially counter-propagating laser manipulation. A photo of the actual setup is also shown

Full size image

We simulated the lateral Brownian motion of a target particle with time-interval \(dt\) based on the above equations, where the optical gradient (\({{\varvec{F}}}_{\mathrm{grad}}\)) and scattering (\({{\varvec{F}}}_{\mathrm{scat}}\)) forces were incorporated as the external force, \({\varvec{f}}\left({\varvec{r}}\right)\), \(({\varvec{r}}=(x,y,z))\) as shown by Eqs. 7–9. Note that the optical forces do not depend on time in the present simulation considering steady-state CW laser irradiation:

$${\varvec{f}}\left({\varvec{r}}\right)={{\varvec{F}}}_{\mathrm{grad}}\left({\varvec{r}}\right)+{{\varvec{F}}}_{\mathrm{scat}}\left({\varvec{r}}\right)$$
(7)
$${{\varvec{F}}}_{\mathrm{grad}}\left({\varvec{r}}\right)=\frac{1}{2}{\epsilon }_{m}\alpha \left(\nabla {\left|{{\varvec{E}}}_{+}({\varvec{r}})\right|}^{2}+\nabla {\left|{{\varvec{E}}}_{-}({\varvec{r}})\right|}^{2}\right)=\frac{{n}_{m}\alpha }{{\epsilon }_{0}c}\left\{\nabla \left({I}_{-}\left({\varvec{r}}\right)\right)+\nabla \left({I}_{+}\left({\varvec{r}}\right)\right)\right\}$$
(8)
$$\left(\alpha =4\pi {a}^{3}\frac{{\epsilon }_{p}-{\epsilon }_{m}}{{\epsilon }_{p}+2{\epsilon }_{m}}\right)$$
$$F_{{{\text{scat}}}} \left( r \right) = \frac{{n_{m} }}{c}C_{{{\text{scat}}}} \left( {I_{ - } \left( r \right) - I_{ + } \left( r \right)} \right) \cdot \mathop{z}\limits^{\rightharpoonup}$$
(9)
$$\left({C}_{\mathrm{scat}}=\frac{{k}_{m}}{6\pi }{\alpha }^{2},{k}_{m}=\frac{2\pi }{\lambda }\right)$$

Here, \({\epsilon }_{m}\) and \({\epsilon }_{p}\) are the permittivity of the surrounding medium and particle, respectively, \(\alpha\) is the polarizability of the particle derived under dipole approximation, \({{\varvec{E}}}_{\pm }({\varvec{r}})\) and \({I}_{\pm }\left({\varvec{r}}\right)\) are, respectively, the electric field and light intensity of the counter-propagating laser beams, \({n}_{m}\) is the refractive index of the surrounding medium, \({C}_{scat}\) is the scattering cross-section of the particle, \(\lambda\) and \({k}_{m}\) are the wavelength and wavenumber in the surrounding medium of the laser. In this simulation, we employed particles much smaller than the wavelength of light so that the gradient and scattering forces can approximately be expressed by the simple Eqs. (8 and 9).

1.2 Optical setup, sample, and experimental procedure

To demonstrate the idea above, we performed experiments on the orbital rotation with an optical setup shown in Fig. 1. A linearly polarized continuous-wave (CW) diode-pumped solid state (DPSS) laser oscillating at a wavelength of 640 nm (RLK40500, LASOS) was used for the optical manipulation. The beam diameter of the CW laser was triply expanded using a beam-expander and its polarization was converted into circular polarization using a quarter wave-plate. The CW red laser beam was split into two beams with orthogonally linear polarizations by a polarizing beam splitter. The two beams (beam 1 and beam 2 in Fig. 1a) were individually steered to prepare a counter-propagating setup as shown in Fig. 1a. Both laser beams were individually focused using a microscope objective (MPlanFL N 10X, NA = 0.3, Olympus).

As a target of the optical manipulation, an aqueous colloid of fluorescent polystyrene microparticle (FluoSpheres™ Carboxylate-Modified Microspheres FF8816, ThermoFisher, 4.2 × 1010 particles/mL) with a diameter of 1.0 µm was chosen. The colloidal solution was diluted by ca. 5000–10000 times with ultrapure water. A custom-made tiny quartz cuvette was filled with the colloidal solution thus diluted at an appropriate particle concentration. To observe the optical manipulation of the particles around the two beam waists, we employed an upright optical microscope with a water-immersion microscope objective (LUMPlanFL N 40X, NA = 0.8, Olympus) and a CCD camera (INFINITY 3–1 URM, Lumenera). The distance (\(\Delta h\) in Fig. 1b) between the axes of the two lasers was tuned from 0 µm to ca. 6 µm. The position of the axis of each beam was determined by detecting fluorescence intensity of an aqueous solution of Atto 647 (ATTO TECH gmbh) in the tiny cuvette. The absorption band of the fluorophore in the microparticles shows the maximum peak at 625 nm and its fluorescence peak locates at 645 nm. The 640 nm laser could excite the fluorophores in the microparticles although the wavelength locates in the red-edge of the absorption band. By detecting the fluorescence of the microparticles in a wavelength range > 650 nm, the motion of the microparticles in the counter-propagating optical tweezers was monitored. All experiments were performed at a room temperature of 294 K under atmospheric conditions.

2 Results and discussion

2.1 Simulations

We first simulated the lateral motion of a microparticle only with the optical forces under the assumption that the random displacement due to thermal fluctuation is zero (without Brownian motion). The light intensity distribution is shown in Fig. 2a; the laser power of each beam is 500 mW, the distance between the axes of the two lasers, \(2h\) (= \(\Delta h\) in the experiment), is 2.5 µm, and the wavelength (\(\lambda\)) and beam waist (\({w}_{0}\)) for each beam are 640 nm and 1 µm, respectively. It should be noted that in the series of simulation, we considered a simple condition for extracting the essence of the rotational motions; we employed a smaller particle size (75 nm in radius) compared with the experiments for approximating that the particle experiences almost uniform light intensity expressed by Eq. (5), that is the light intensity distribution over the volume of the particle is ignored. This size reduction of target particle increased the displacement in a unit time by the Brownian motion and, the trajectory of the rotational motion was much scattered. To decrease the scattering in the trajectory of a particle, we simulated the motion of the particle under a little more viscous (as ca. twice viscosity as water, 2 cP) solution. Since the condition above is not so far from the actual experimental condition and the essence of the rotational motion driven by optical force can be numerically investigated.

Fig. 2
figure 2

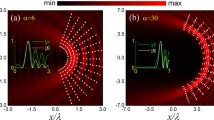
a Calculated light intensity distribution of the non-coaxially counter-propagating laser beams based on Eqs. 5 and 6. The wavelength (\(\lambda\)), beam waist (\({w}_{0}\)), and incident power (\(P\)) for each beam are 640 nm, 1 µm, and 500 mW, respectively. The distance between the optical axes of the two beams (\(2h\)) is 2.5 µm. b, c Trajectories of the lateral motion of a dielectric particle with a radius of 75 nm and a refractive index of 1.6 in the presence of scattering and gradient forces due to the two beams shown in a, b without and c in the presence of thermal (Brownian) motion at 298 K. The refractive index and viscosity of the medium is 1.4 and 2.0 cP, respectively. d Overlapped trajectories of the particle in b and c. e, f Trajectories of the lateral motion of the dielectric particle at e 10 K and f 50 K; simulation condition is the same as that of c except for temperature. g–i Trajectories of the lateral motion of the dielectric particle at 298 K at laser intensities of g 20, h 400, and i 2000 times higher than the simulation (c)

Full size image

In Fig. 2b, the red solid line superimposed on the light intensity distribution shows a trajectory, thus calculated of the small dielectric particle. As already described above, the beam 1 propagates from the left to the right and, the beam 2 from the right to the left. At the beginning of the simulation, the microparticle was placed at x = 1.25 µm and z = 0 µm. With the passage of time, the microparticle was pushed from the left to the right along the beam 1 mainly by its scattering force. Around the position (x, z) = (1.25 µm, 9 µm), the microparticle started to move towards the bottom because the overlapped two beams exert the optical gradient force on the particle. Because the spatial distribution of light intensity has a local maximum at ca. (x, z) = (0 µm, 11 µm), the point (trap site 2 in Fig. 2b) is a local potential minimum of optical trap, and the particle fell into the local (trapping) potential minimum while showing a spiral trajectory. Finally, the particle was stably trapped at the local minimum of the potential where the sum of the total optical forces is zero; it took ca. 11 s from the start of the motion to the trapping by the potential. As clearly shown in Fig. 2b, the particle did not exhibit any orbital rotation in the counter-propagating optical tweezers because of the symmetry in the optical field.

Next, we calculated the trajectory of the same particle in the same medium in the presence of thermal fluctuation (Brownian motion) at 298 K; the numerical parameters of optical forces are also the same as those in Fig. 2b. Figure 2c shows a trajectory of the particle simulated under the above-mentioned condition. At the beginning, the microparticle was placed at x = 1.25 µm and z = 0 µm as the initial position of the simulation. Then the microparticle started Brownian motion in the presence of the optical forces. The particle was pushed to the right by the scattering force of the beam 1 and turned to the bottom around the point of (x, z) = (1.25 µm, 9 µm) in the similar manner with Fig. 2b. As opposed to the result shown in Fig. 2b, the microparticle was not trapped at any specific location and successively transported from the right to the left by the scattering force of the beam 2. After reaching the left part in Fig. 2c (z = ca. − 12 µm), the microparticle transferred from the beam 2 to the beam 1 by the assistance of thermal fluctuation in the similar manner. After the transfer to the beam 1, the microparticle was pushed to the right, and a series of the above motions continued. As a result, the microparticle exhibited the clockwise orbital rotation as shown in Fig. 2c.

To clearly visualize the difference between Fig. 2b and c, the trajectories with and without the Brownian motion are displayed in Fig. 2d. In the absence of thermal fluctuation, the microparticle exposed to the optical field in the counter-propagating two lasers will be trapped at one of the local potential minima because the sum of the gradient and dissipative forces is zero in those positions. In contrast, the Brownian motion allowed the microparticle to climb over the shallow potential formed in the area where the two beams overlapped (trap site 1 and 2 in Fig. 2b). Therefore, the microparticle was permitted to continue the orbital rotation in the counter-propagating two lasers separated each other by 2.5 µm. These interpretations are clearly supported by the following two behaviors in Fig. 2d. First, one can see ballistic motions of the microparticle at around the beam waists. This is because the well-defined directions of the dissipative forces along the light propagations [4, 5]. Second, with the Brownian motion, the trajectory in the area where the two beams overlap is scattered compared to the ballistic motions at the beam waists (Fig. 2c). This scattered behavior clearly indicates that the potential depth of the trapping sites 1 and 2 is shallow enough for a microparticle with thermal energy at room temperature. These numerical results expect that the orbital rotation of the microparticle will be possible with the thermal fluctuation in the symmetric optical field consisting of two slightly focused CW laser beams. The inset of Fig. 2d shows the time course of the X position of the rotational motion with Brownian motion. The rotational frequency is in the order of 0.5–1 Hz. The values are comparable to the experimental values for microparticles the data of which is shown in Figs. 3 and 4, indicating that the simulation can be used for investigating the essential mechanism of the rotational motion under similar condition with the experiment.

Fig. 3
figure 3

a Time-course fluorescence images of a 1-µm particle trapped by the coaxially counter-propagating laser beams. b Trajectory of the particle shown in a in the trapping process. The inset of b shows the time course of the fluorescence intensity of the particle during the transportation and trapping. c Time-course fluorescence images of single orbital rotation of a 1-µm microparticle under photo-irradiation with the non-coaxially counter-propagating laser beams. d–f Trajectories of orbital rotation of 1 µm particles for different inter-beam distance, \(\Delta h\); each value of \(\Delta h\) are shown in the figures

Full size image
Fig. 4
figure 4

Rotational speed of the 1-µm particles as a function of incident laser power of beam 1 and 2. Note that the laser power of the two beams were identical within < 1% error as shown in figure SM1

Full size image

Figure 2e and f shows simulated trajectories of the microparticle at temperatures of 10 K and 50 K, respectively. Even at the very low temperature (10 K), the particle showed orbital motion as shown in Fig. 2e while being sometimes trapped by the shallow trapping potentials as the trajectory in Fig. 2b, which can be confirmed by the spiral trajectory indicated by a black arrow in the left part of Fig. 2e. On the other hand, at 50 K, the particle showed similar orbital motion to that at 298 K although the scattering of trajectory is smaller. These simulations indicate that the depth of the trapping potential is comparable to the averaged thermal energy of the particle in the temperature range around 10 K and, the result implies a very high light intensity, > > 30 times, is required for trapping of the particle by this optical configuration at room temperature (298 K) even under the condition that neither particle nor solvent does not absorb incident laser. To investigate this expectation, we conducted a series of simulation at very high laser intensities. Figure 2g–i shows trajectories of the microparticle at much higher (20-, 400-, and 2000-times) light intensities than that in Fig. 2c. It should be noted here that we employed different time intervals of the simulation, \(dt\), for precisely track the motions of the particle, because the transportation speed of the particle due to the optical forces increases with increasing light intensity. The particle was not trapped in the trap sites 1 nor 2 at the 20-times light intensity (Fig. 2g), while at the 400-times light intensity (Fig. 2h), the particle was partially trapped in the trap sites and, at much higher intensity of 2000-times (Fig. 2i), the particle was almost trapped and sometimes went out the trapping potential with a low probability. From these results, we can conclude that the trapping effect of the two trap sites is negligible under the actual experimental condition where the incident light intensity is in the range from several to several tens of mW (Fig. 4).

To demonstrate the above approach for the orbital rotation, we performed a series of optical manipulation experiments as a function of the distance (\(\Delta h\) in Fig. 1b) between the two laser beams. First, we trapped one of the fluorescent microparticles by the counter-propagating laser beams at \(\Delta h=0\) µm. In this experiment the two laser beams are coaxially overlapped, and the microparticle was stably trapped at the beam waist. Figure 3a shows the time course of fluorescence images of this trapping process. The microparticle first (at 0 s) accidentally entered the right part of the photo-irradiation area by Brownian motion. The particle was transported to the left (from 0.25 to 0.75 s) by the gradient force of the laser although the scattering and absorption forces of the two beams balanced each other. Finally, the particle was stably trapped at the beam waist of the laser beams. The corresponding trajectory of the microparticle is shown in Fig. 3b. The apparent size of the particle in the figure changes with time owing to the decrease in the fluorescence intensity due to the photodegradation of the dyes contained in the particle by the 640-nm irradiation as shown in the inset of Fig. 3b that exhibits the time trace of the fluorescence intensity of the particle during the trapping.

Separating the two beams by several micrometers led to orbital motion of a microparticle. Figure 3c shows a series of fluorescence images of single orbital rotation of the microparticle induced by the non-coaxially counter-propagating laser beams separated by 2.7 µm. First the microparticle is moving from the right to the left by the dissipative force of the beam 2 (0–99.6 ms). After reaching the turning point in the left at 133 ms, the particle starts turning motion and its moving direction is completely reversed at 199 ms, then the particle goes back to the right by the dissipative force of the beam 1. The particle repeated this orbital motion at least until the fluorescence disappeared due to photodegradation of the fluorophore by the 640-nm irradiation. Figure 3e shows the whole trajectory of the orbital rotations single rotation of which is exhibited in Fig. 3c.

The size and shape of the track of the rotation depends on the distance between the two laser beams. With an increase in the value of \(\Delta h\), the rotation orbit expanded both for the X and Y axes, as shown in Fig. 3d–f. Thus, it has been demonstrated that the microparticles can exhibit continuous orbital rotations with the help of the Brownian motion in the steady-state, symmetric optical field of counter-propagating two laser beams.

To gain a deeper insight into the orbital rotation, we examined laser power dependence of the rotational velocity of the microparticle; the velocity was evaluated as revolutions per minutes (RPM). For this purpose, we observed the orbital rotation of the microparticle as a function of the laser power at the distance between the two lasers of 5.8 µm, as shown in Fig. 4. These RPMs were determined by the trajectories of the orbital rotations shown in Supplementary Materials, Fig. SM1. It should be noted here that the shape of every trajectory was almost identical at each laser power, allowing us to fairly compare the RPMs. As shown in Fig. 4, the RPM linearly increased with laser power. This linear behavior can be ascribed to linear dependences of the optical gradient and the dissipative forces on the incident light intensity [6] and negligible trapping potential of the trap site 1 and 2 (see Fig. 2b). As already discussed, the trapping potential will become effective at much higher laser intensity at the room temperature (298 K), where the rotational frequency will be affected by partial trapping by the trap sites. The linear dependence of rotational frequency on laser power in Fig. 4 indicates the averaged thermal energy of the particle at 298 K is enough large to easily escape from the trapping potential in the examined power range of the incident laser.

3 Summary

We have demonstrated the orbital rotation of the microparticle in the non-coaxially counter-propagating optical tweezers both in the numerical simulations and the experiments. In the symmetry optical fields without the Brownian motion, the microparticle was initially transported by the optical forces and, with the passage of time, it was stably trapped at some locations where the sum of the optical forces is zero (i.e., a local minimum in the trapping potential). In contrast, with the Brownian motion, the microparticle exhibited the continuous clockwise orbital rotation in the counter-propagating optical tweezers in water at the room temperature. The behavior of the orbital rotations was dependent on the spatial displacement between the two laser beams and the incident laser power. These results clearly show that the orbital rotations were mainly driven by the optical forces, although the Brownian motion plays a major role to climb over the shallow trapping potential wells. From the results obtained in the report, we foresee that the environmental disturbance will be able to provide a wide range of approaches to assist further development of advanced optical manipulation techniques.