1 Introduction

Ultra-high-performance concrete (UHPC) exceeds the performance of normal or high-strength concrete, allowing for the construction of more slender and efficient reinforced concrete (RC) structures. This is achieved using ultra-fine constituents and reactive powders which improve the mechanical properties and durability, yet increases the brittleness [1, 2]. For enhancement of the ductility properties, steel fibres are particularly attractive as concrete constituents [2,3,4]. These also can contribute to reducing the amount and congestion of conventional reinforcement in RC elements [4, 5] and are widely used in practice in tunnel linings, industrial floor slabs and aircraft runways. UHPC is a low water-to-binder ratio cementitious composite that has compressive strengths in the range of 130–160 MPa, under ambient curing and typical mixing technology. It has a low porosity due to improved homogeneity and enhanced packing density due to the use of relatively fine and ultra-fine materials such as micro- or nano-silica [6]. In UHPC, the coarse aggregates are replaced by fine sand and quartz powders, as in high-strength cement pastes, the skeleton formed by the conventional aggregates can become the weakest component [7].

High-strength mixes provided with micro-silica tend to have a denser microstructure in comparison with conventional mixes due to the formation of the new gel as a result of the secondary hydration between the calcium hydroxide and silica [1, 6]. Under complex and controlled curing regimes and production techniques, such UHPC can reach strengths above 450 MPa [8]. Silica fume, in ranges up to 25–30% of the cement mass along with heat treatment, increases C–S–H production through accelerating the cement hydration, as well as acts as a micro-filler of concrete voids to reduce the porosity [9]. Aiming at reducing the environmental impact of UHPC, which uses at least twofold of the cement used in normal-strength concrete, recent studies substituted cement up to about 45% by weight by less-energy-intensive cementitious additives such as fly ash, ground granulated blast-furnace slag, limestone powder or metakaolin [2, 10].

Steel fibre-reinforced concrete (SFRC) is a composite material that is characterised by an enhanced ductility and post-cracking tensile residual strength or toughness, due to the reinforcement mechanisms provided by fibres bridging the crack surfaces [11, 12]. The mechanical performance of UHPSFRC of cement-based composites is strongly affected by the fibre volume, fibre geometry and orientation within the matrix. Tensile, bending and compressive cracking stress, peak stress, strain at peak stress, toughness and residual strength under cyclic loading are significantly improved with the increase in volumetric fibre content [11, 13]. For the same fibre content, twisted steel fibres lead to a better mechanical performance than hooked-end fibres [14], and implicitly hooked fibres are more effective than the straight geometries. Besides enhanced ductility and spalling properties, there is a significant benefit of using steel fibres in concrete structures where shear or punching shear is a governing consideration in design [15, 16]. The results showed that incorporating a high dosage of fibres (0.75–1.25% in volume) into concrete significantly improves the structural behaviour of SFRC beams; this provides bending hardening, considerably limits crack opening in service and gives a ductile behaviour for ultimate conditions [4]. An increase in steel fibre volume fraction from 0.5 to 0.75% increased the shear capacities by 13% and 30%, respectively, and the diagonal cracking strength by 35% on average [17]. Even a relatively low amount of fibres, below 0.7%, can contribute to the shear strength and ductility of concrete beams without transverse reinforcement [5].

UHPSFRC experimental member studies typically focused on the flexural response of rectangular beams provided with conventional longitudinal reinforcement in low or high amounts and typically incorporating transverse reinforcement [18,19,20,21]. In terms of benefits, the presence of fibres had a similar effect as for normal or high-strength concrete. An increase in steel fibre content generally produces lower shear crack widths and a less brittle behaviour [22]. A moderate number of stirrups can effectively restrain shear cracks and allow more parallel diagonal shear cracks to develop and propagate thoroughly within the shear span [23,24,25]. Shear performance of UHPSRFC was addressed through a limited number of push-off tests [22], T-beams [23, 24] and I-beams with and without openings within the shear span [25]. Numerical simulations of UHPFRC behaviour using nonlinear procedures are limited to modelling the material response [26,27,28] or the flexural response RC beams incorporating UHPFC materials [19, 29]. Although shear behaviour was successfully represented using three-dimensional models for conventional RC beams and slabs [e.g. 30], models incorporating UHPSFRC seem to be lacking.

This paper presents a study into the response of UHPSFRC materials and elements through a series of compressive and flexural tests on cubic and prismatic samples, respectively, as well as on medium-scale I-beams provided with conventional longitudinal reinforcement and various steel fibre contents. After describing the experimental methodology and test results, nonlinear numerical validation procedures and parametric studies are carried out against flexural samples and structural beams, using concrete damage plasticity models. An inverse analysis is carried out to assess the tensile stress–strain curve of UHPSFRC, which is used for modelling the structural members. The results from the numerical simulations along with those from the tests in this paper and from a collated database are used for the modification of a shear strength assessment method for UHPSFRC members with particular focus on beams loaded within low values of shear span-to-depth ratios. The proposed method is shown to offer a reliable and practical approach for shear strength assessment of structural UHPSFRC members.

2 Experimental assessment

2.1 Materials and mix designs

The concrete mixes incorporated cement silica fume, quartz, admixtures and tap water. The binders include CEM I 52.5 R cement with high initial strength conforming to BS EN 197-1 [31] and a high SiO2 content silica fume. The cement composition included on around 95% main clinker components (tricalcium silicate C3S, dicalcium silicate C2S, tricalcium aluminate C3A and tetracalcium aluminoferrite C4AF), < 4% sulphates SO3, < 0.10% chlorides Cl, < 0.9% alkali Eq Na2O. The silica fume was added to improve internal cohesion and water retention in the fresh concrete, as well as to facilitate particle packing for increasing the cement matrix density and strength due to the formation of additional hydration products in hardened concrete [32]. Quartz sand particles with sizes up to 1.2 mm and with a specific gravity of 2.65 were used as mineral aggregates. A polycarboxylate ether superplasticiser was used to improve workability and ensure suitable flow ability whilst maintaining low water-to-binder ratio in the range of w/b ≈ 0.13 [33]. Its main effect is accelerating cement hydration through an earlier development of hydration heat and rapid development of hydration products.

The concrete mixes investigated in this paper incorporated two fibres with two relative configurations. Longer hooked-end fibres with a length of lf = 25 mm and a diameter of df = 0.4 mm were used stand-alone or mixed in a 50/50 ratio with shorter straight fibres with a length of lf = 6 mm and a diameter of df = 0.175. The benefit of adding two types of fibres in the cementitious matrix is that these act at two different scales that would enhance the mechanical properties and post-cracking performance of SFRC. The longer fibres were made of stainless steel and had nominal tensile strengths above 1450 MPa and elastic modulus around 210 GPa [34]. The shorter fibres were relatively stronger with a tensile strength above 2800 MPa and a similar elastic modulus as the longer fibres. These were made of steel with a brass coating of about 8.5 g/m2 [35]. These fibres were mixed in the cementitious matrix comprising the above materials in three fibre percentages by volume Vf: 1.5%, 2.0% and 2.55%). The mixes incorporating the longer fibres (lf/df = 62.5) are noted with L, whilst those which include both long (lf/df = 62.5) and short (lf/df = 34.3) fibres are referred to as hybrid and noted with H.

The cement, silica fume and quartz sand, in the ratios listed in Table 1, were mixed together in an 85-L rotary mixer, until a homogenous dry mix was obtained. Following, the water combined with the admixture was mixed together until an acceptable level of workability was reached. Ultimately, the fibres (L) or the fibre blend (H) were integrated in the wet mix and mixed together. Each concrete batch required up to 10–15 min total mixing time to be completed. Concrete fresh properties were assessed by means of a flow cone, and it was shown that steel fibres did not influence significantly the flow, which was around 120 mm for all mixes. Cubic samples of 50, 70, 100 mm, along with 100 × 100 × 400 mm prismatic samples, were prepared to assess the compressive and flexural strength of the investigated concrete materials. The material strengths are listed in Table 2. The concrete poured in the larger specimens prepared for structural shear testing was produced in the same manner as for the samples for material testing.

Table 1 Concrete mixes
Full size table
Table 2 Specimen details
Full size table

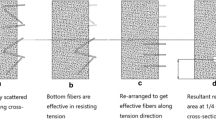
The concrete prisms and structural members were cast horizontally with the bottom fibre on the bed of the formworks. After casting, all concrete elements were covered with a thin oil film and plastic sheets to prevent evaporation and ensure appropriate hydration. The specimens were removed from forms 24 h after casting and further subjected to a steam curing regime at 90 °C and 40% relative humidity for a period of 5 days. The structural members were provided with Grade S500 longitudinal reinforcement as described in the following section. The material properties of the steel reinforcement materials, determined from a minimum of three steel coupons, were the yield strength fy = 512 MPa, the ultimate strength ft = 690 MPa and the corresponding ultimate strain of εu = 0.13 mm/mm.

2.2 Specimens and testing arrangement

The parameters directly investigated in the testing programme were the fibre types, long or hybrid, as well as the steel fibre content (1.5%, 2.0% and 2.55%). Besides the cubic and prismatic samples cast for material strength assessment, six 1600 mm long structural members were tested. An I-shaped cross section with a maximum flange width of bf = 120 mm and beam height of h = 240 mm was considered. The beam web had a thickness of tw = 50 mm and a web height of hw = 60 mm. The specimens were longitudinally reinforced with 2 × Ø14 mm bars in tension and 2 × Ø10 mm in compression. Except for 2 × Ø6 bar used for fixing the longitudinal bars, at each of the supports, no transverse reinforcement was provided. The members were subjected to monotonic three-point loading with a shear span-to-depth ratio (a/d) of 1.25 and were tested in two stages.

In the first Stage (1), the beams were supported at a moment span of ls = 1400 mm with the load applied at a/d = 1.25 from the left support. After the ultimate state was reached, the undamaged side of the beam was supported at ls = 560 mm and tested by applying the load mid-way between the supports (Stage 2). The specimen references depicted in Table 2 adopt the format Cxyy/z in which x indicates the type of fibres (L for long and H for hybrid fibre blend), yy depicts the fibre volume (15 for 1.5%, 20 for 2.0% and 25 for 2.55%), and z is for the moment span configuration (1 for ls = 1400 mm and 2 for ls = 560 mm). For example, CH25/1 indicates a beam provided with hybrid fibre blend with a fibre content of 2.55% that had a moment span of 1400 mm. The concrete cover to the tension reinforcement was c = 30 mm. It is worth noting that, prior to carrying out tests in Stage 2, all specimens have been visually inspected within the short moment span ls = 560 mm (Fig. 1b) using a crack width microscope. Except for CH20/2 which had some cracks within that region after being tested in Stage 1, all other specimens had no visible damage. Moreover, a few of the specimens (i.e. CH25/1, CH20/1, CL25/1 and CL20/1) had duplicates, yet for consistency, only one specimen per material type is reported in detail in this paper.

Fig. 1
figure 1

Testing arrangement: a Stage 1 tests—ls = 1400 mm, b Stage 2 tests—ls = 560 mm

Full size image

The test set-up was designed to simulate loading conditions characteristic for precast reinforced concrete (RC) beams under concentrated load with relatively high shear action. A schematic outline of the rig and instrumentation details are given in Fig. 1a, b. The load was applied through a hinge to the member by means of a hydraulic actuator that was provided with an HBM load cell with a capacity of 5 MN. The load was applied gradually in steps of 10 kN, and the data was recorded continuously throughout the test with a digital logger. Two steel rollers acting as boundary conditions were positioned on a stiff support frame which was connected directly to the laboratory floor. Displacements were measured at a minimum of three locations along with the moment span of the beam with transducers with a precision of 0.1 mm. The surface deformations were recorded at five locations by means of digital dial gauges within the shear span. These were positioned on the tension flange, web and compression flange of each specimen. Crack patterns and crack widths were recorded in all tests up to values of wmax = 0.4 mm. The mechanical properties of concrete were tested using a servo-hydraulic controlled testing machine type Advantest-9 with a capacity of 3000 kN, whilst those for assessment of the steel material properties through a testing machine provided with and 100 kN HBM load cell and HBM displacement measurement devices.

2.3 Test results

The tests described in this section focused on assessing the influence of fibre content and type to the shear performance of UHPSFRC elements. As mentioned before, concrete materials with relatively longer fibres with lf/df = 62.5 or a fibre blend of long (lf/df = 62.5) and short (lf/df = 34.3) elements, with volumetric contents Vf of 1.5%, 2.0% and 2.55%, were used in the structural members. It is worth noting that a previous study on members subjected to shear action that incorporated similar concrete materials with 0% and 2.0% fibres showed that the presence of fibres increased the ultimate capacity of the fibrous concrete (UHPFRC) with up to twofold, and the corresponding ultimate displacement threefold in comparison the counterpart without fibres (UHPC) [36]. The same study indicated that the presence of 2.0% fibres increases the flexural strength with up to 150% of the non-fibre-reinforced concrete, whilst enhancing the post-peak response.

As shown in Fig. 2, the specimens tested in this paper had a shear-governed response, primarily due to the relatively short shear span-to-depth ratio. The test results depicted in Table 3 indicate a gradual increase in ultimate capacity with an increase in fibre content. For the members provided with hybrid fibre blend (CH), shear cracking was observed between 40 and 60 kN, corresponding to about 23–30% of the ultimate load, whilst flexural cracking initiated around 70 kN which is in the range of expected cracking force based on the tensile concrete strengths obtained from material testing. Moreover, the specimen with the highest hybrid fibre blend (CH25/1) seems to have developed delayed cracking in comparison to the members with lower hybrid fibre blends (CH20/1 and CH15/1). Similarly, for members with long steel fibres (CL), the shear cracking occurred between 40 and 71 kN which corresponds to 17–40% of the peak capacity. Flexural cracking was noted around 60 kN. It is worth noting that these values are based on a visual inspection and the crack widths corresponding to the force values mentioned above were in the range of w = 0.02 mm.

Fig. 2
figure 2

Test crack patterns

Full size image
Table 3 Test and numerical results
Full size table

In terms of ultimate capacity, an increase in hybrid fibre blend content (Vf) from 1.5 to 2.0% showed an enhancement in strength 17% (CH15/1 vs. CH20/1), whilst an increase in Vf from 1.5 to 2.55% showed an increase in strength of about 24% (CH15/1 vs. CH25/1). An increase of 15% was observed between the CH15 and CH25 members with Vf = 1.5% and Vf = 2.55% subjected to concentrated load and short moment span (ls = 560 mm). Based on the results from Table 3, it is noted that the hybrid fibre blend had a more beneficial effect in enhancing the ultimate capacity. Moreover, an increase in fibre volume from 1.5 to 2.0% showed an increase in capacity of about 34% (CL15/1 vs. CL20/1), whilst an increase in Vf from 1.5 to 2.55% showed a strength enhancement of about 46% (CL15/1 vs. CL25/1). For cases tested with short moment span (ls = 560 mm), the strength enhancement was 27% and 36% for CL15/2 versus CL20/2, and CL15/2 and CL25/2, respectively.

As mentioned in Sect. 2.2, CH25/1, CH20/1, CL25/1 and CL20/1 had duplicates which were tested in the loading arrangement illustrated in Fig. 1a. The standard deviation of the test strength of the two duplicates was 3.1%, 3.0%, 18%, 5.9% for CH25/1, CH20/1, CL25/1, CL20/1, respectively. Except for the strengths of the duplicates of CL25/1 which had relatively large standard variation, all results are within reliable limits.

More importantly, some increase in ultimate deformation was observed with an increase in fibre content. The beams provided with a fibre volume of 2.55% and 2.0% showed a flexurally governed shear failure, whilst those with 1.5% failed with limited or no sign of flexure. The increase in ductility is primarily attributed to the crack bridging effect provided by the fibres. This effect was clearly observed in the four point-bending tests on notched prismatic samples without longitudinal reinforcement, prepared for flexural strength assessment. Prisms with both long (CL) or hybrid (CH) fibre content of 2.55% had an increase of around 5.5% in strength in comparison with their counterparts with 1.5% fibre content (Table 2). Based on the experimental observations, the distribution of the two fibre types was relatively even through the failure surface, hence, contributing to a similar extent to the response. By contrast, non-homogenous distributions, either by localisation of the fibres depending on the casting direction or by bulging, would have an impact to the member capacity and would affect significantly the crack kinematics and crack bridging behaviour. The test results on prismatic samples are further used for inverse finite element analysis to obtain the uniaxial tensile constitutive response of UHPFRC. Such methods to assess the stress–stress response of concrete materials have been used before [e.g. 26, 37, 38].

3 Numerical modelling

3.1 Concrete damage plasticity

The ‘concrete damaged plasticity’ model (CDP), incorporated in the commercial finite element programme Abaqus 6.14 [39], was employed to represent the triaxial behaviour of concrete. For this, the potential yield function is controlled by the effective stress values, the biaxial behaviour of concrete (fb0/fc = 1.16) and Kc = 2/3 that governs the shape of the deviatoric plane. The CDP model accounts for a non-associated potential plastic flow, in which the plastic volume expansion is not proportional to the increase in stresses. This is represented in the plastic potential flow function by the dilation angle φ measured in the p–q plane at high confining pressure and the surface eccentricity (ϵ = 0.1). The constitutive model requires uniaxial stress (σ)–strain (ε)/crack displacement (w)–damage (di) relationships for compression and tension behaviour. In this study, a plastic stiffness degradation scheme, typical for coupled damage-plastic concrete constitutive models was adopted.

Before crushing in compression or cracking in tension, no degradation occurs, and the plastic strains are equal to the inelastic strains. Beyond these points, the stiffness reduction enables the development of irreversible plastic strains that are directly proportional to the stress decrease [30]. The damage in compression dc and damage in tension dt parameters are also part of the variable field output of the FE environment. They allow direct interpretation of the stress state within different regions of the members, illustrated by compression and tension damage patterns, and member stiffness degradation. Established on a continuum approach, in which no physical separation is created in the model mesh, tension damage mesh regions provide an effective way to illustrate the kinematic aspects of crack development. The input constitutive parameters have been determined based on the tests described in Sect. 2 and are presented next.

3.2 Uniaxial constitutive properties

As mentioned before, compression and four-point bending tests were performed to obtain the mechanical properties of the UHPFRC materials reported in this paper. The compression stress–strain curve obtained from tests was used as direct input in the numerical model, whilst the four-point bending tests were used to determine the tensile constitutive response. Assessment of direct tensile properties of steel fibre concrete materials requires a relatively complex testing arrangement. An alternative to assess the tensile stress–strain is through an indirect finite element analysis from three- or four-point bending tests. The procedure involves modelling the flexural test and carefully varying the key material parameters such as the tensile strength, fracture energy or post-peak representations and crack bandwidth to obtain close agreement between test and numerical force–crack mouth opening displacement curves [26, 37, 38]. It is assumed that a single straight crack transfers all stresses through the interface and the crack width varies linearly with its depth from mouth to root. The root of the crack with w = 0 represents the rotation point of two discrete bodies.

To simulate reliably the response of the flexural prismatic specimens, the full member geometry was accounted for in modelling. The models of 100 × 100 × 400 mm samples constructed to undertake an inverse analysis for assessment of the tensile stress–strain curves of UHPFRC were made of 2D planar deformable shells incorporating four-node bilinear plane stress quadrilateral elements with reduced integration (CPS4R). Both 3D models using solid elements (C3D8R) and 2D models using the element types mentioned before have been built. Close inspection of the results indicated that both modelling procedures offer nearly identical predictions and modelling prismatic members with square cross section can be regarded as plain stress problem. As a significant number of sensitivity studies need to be carried in order to obtain the tensile stress–strain from bending tests, a much lower computational time is obtained by employing shell models. This has been shown in past studies which indicated that both 3D solid and 2D shell modelling closely estimate the experimental load–deformation response of concrete members, with 2D modelling offering savings in the computational time of about 14-fold [40].

Additionally, besides the implicit computational time savings, this is also corroborated with the fact that a very fine mesh needs to be adopted in order to capture reliably the softening behaviour of concrete, as described below. Hence, shell models were used to address this particular problem. In the rectangular shell models, boundary conditions and load application points were made of circular discrete rigid wires connected to reference points and tied to the deformable shell component. The Newton–Raphson method was used for the integration procedure, and a displacement control procedure was considered in which a vertical displacement δ was applied to the relevant reference points.

The tensile strength of fibre-reinforced concrete is determined from the flexural strength obtained from four-point bending tests using an established model and a tensile-to-flexural strength conversion factor around αfl ≈ 0.6 [41] (Eqs. 1–3). This parameter depends on the type of the stress–strain curve and implicitly type of concrete modelled [29]; hence, λfl = 0.065. In modelling, a linear-elastic behaviour was considered up to cracking fct, followed by a nonlinear tension softening law (Eq. 4) [42]. It is worth noting that the original softening law constitutive parameters (c1 = 3, c2 = 6.93, wmax = 160 μm) were calibrated against tests on normal concrete with tensile strengths around 3.2 MPa. A sensitivity analysis was carried out for the UHPSFRC materials investigated in this paper by modifying the c1 and wmax parameters from Eq. 4 (Fig. 3). The c1 parameter controls the shape of the descending branch, whilst wmax represents the crack opening at zero stress.

Fig. 3
figure 3

Sensitivity analysis for the tensile stress–strain input curve: a influence of the ultimate crack width wmax, b influence of c1 parameter

Full size image

As mentioned before, the presence of steel fibres has a significant effect in enhancing the post-peak fracture energy, represented by the area under the tensile σ–ε curve. Implicitly, wmax for UHPSFRC depends on the fibre content and type, and it takes larger values than for normal concrete. In order to calibrate the nonlinear tension softening law, wmax of normal concrete (i.e. 160 μm) was multiplied with factors between 4.00 and 5.05, depending on the type of fibre and content (long fibres—L or hybrid blend—H; Vf = 1.5–2.55%) (Fig. 3a). On the other hand, the c1 parameter was varied from 1 to 3. For the same wmax, a lower value of c1 indicates lower fracture energy in comparison with a higher c1 value (Fig. 3b).

The sensitivity study showed that a value of c1 = 1 captures reliably the test response. For the numerical input stress (σ), crack mouth displacement wi–damage (dt) relationships were considered. In the uniaxial post-peak tension regime, the damage is assumed to grow linearly from 0 to 0.9 which corresponds to w = 0 and wmax, respectively (Eq. 5). Such ranges were also employed in other studies from the literature [42]. Beyond this value, the damage scalar is subsequently held constant to preserve a residual stiffness and strength [43].

$$f_{\text{ct,fl}} = \frac{PL}{{bh^{2} }}$$
(1)
$$f_{\text{ct}} = \alpha_{\text{fl}} \times f_{\text{ct,fl}}$$
(2)
$$\alpha_{\text{fl}} = \frac{{\lambda_{\text{fl}} \times h_{\text{b}}^{0.7} }}{{1 + \lambda_{\text{fl}} \times h_{\text{b}}^{ 0 {. 7}} }}$$
(3)
$$\frac{\sigma }{{f_{\text{ct,num}} }} = \left\{ {1 + \left( {c_{1} \frac{w}{{w_{ \hbox{max} } }}} \right)^{3} } \right\} \exp \left( { - c_{2} \frac{w}{{w_{{\mathrm{max} }} }}} \right) - \frac{w}{{w_{ \hbox{max} } }}\left( {1 + c_{1}^{3} } \right) \exp \left( { - c_{2} } \right)$$
(4)
$$d_{i} = \left\{ \begin{array}{l} 0 \to \varepsilon < \varepsilon_{i} \hfill \\ 1 - \sigma_{i} /f_{ci} \hfill \\ \end{array} \right.\quad \text{where}\quad i = c, t.$$
(5)

An additional mesh sensitivity study was carried out to investigate the mesh size influence on the numerical response. Figure 4 shows the load–crack opening p–w curves for the models of the prismatic samples provided with four mesh sizes: 1.5 mm, 3 mm, 6 mm and 12 mm and 1.5% hybrid fibres (H1.5%). The crack width from numerical simulations has been obtained by subtracting the horizontal displacements at nodal points situated at mid-depth of the two notch faces. This corresponds to the location of the displacement transducers attached to the test specimens. As observed, the mesh sizes equal to or higher than 3 mm offer lower strength and unreliable post-peak response, whilst the 1.5 mm mesh shows good agreement between the test and numerical response.

Fig. 4
figure 4

Mesh sensitivity analysis for the inverse finite element analysis, a load–crack opening curves, b mesh sizes for 2D models, c 3D model

Full size image

After validating the modelling procedures for the H1.5% concrete, the same approach was employed to the models with L1.5%, L2.55% and H2.55% concrete. For comparison purposes only, a three-dimensional model of H1.5% using solid elements (C3D8R) has been constructed (Fig. 4c). To reduce the computational time, only the crack front was meshed with 1.5 mm element seeds, whilst for the remaining model the mesh size was increased gradually. Even with this modelling strategy, the ratio between the computational time of the 3D model and the 2D model with lm = 1.5 mm was higher by a factor of 127. As expected, both modelling techniques offer nearly identical results in terms of strength predictions.

The comparative test and numerical p–w curves are illustrated in Fig. 5, indicating a reliable modelling approach and that the tensile σ–ε obtained from the inverse analysis can be used for modelling of structural UHPFRC members. It is worth noting that for the intermediate fibre contents (L2.0% and H2.0%) the input tensile σ–ε was obtained from direct interpolation of σ–ε for materials with higher and lower fibre content. The compressive stress–strain σ–ε relationship was determined from material tests on cubic samples described in Sect. 2.1. A factor of 1.00 was used to convert 100 mm cube fc,cube to cylinder fc compressive strength of UPHC, as suggested in studies from the literature [44]. Both structural and reinforcement steels were modelled using bilinear elastoplastic properties with hardening using the material properties obtained from material tests (Table 4).

Fig. 5
figure 5

Comparative assessment between test and numerical results for flexural tests: a H1.5%, b H2.55%, c L1.5%, d L2.55% concrete; e schematic representation of the test prisms

Full size image
Table 4 Material parameters considered in numerical modelling
Full size table

3.3 Numerical validation

The concrete parts of the UHPFRC beams were modelled using eight-node solid elements with reduced integration (C3D8R), whilst the longitudinal reinforcement with 3D truss elements (T3D2) [39]. The truss elements were embedded in the eight-node solid elements, and perfect bond was assumed. In contrast to the prismatic elements which had a square cross section and the numerical problem can be reduced to a plane stress case, the UHPFRC beams had an I-shaped cross section. Thus, a three-dimensional modelling was considered, primarily to capture reliably the damage propagation within the variable width of the cross section.

The 1600 mm beams were connected through two 50-mm-diameter rollers made of solid elements, representing the test boundary conditions (Fig. 6). The loading strip and the boundary rollers were tied to reference points through multi-point constraints. To simulate the test conditions, surface-to-surface interaction with tangential behaviour defined by a friction coefficient of 0.5 was assigned between rollers and concrete element. The friction confection between steel and concrete varies between 0.2 and 0.8, depending on the loading mechanism and test set-up, and values around 0.5 have been used to reliably simulate experimental tests similar to those described in this paper [e.g. 30]. The supports were assigned with elastic steel material properties, as they were overdesigned to remain in the elastic regime. As for the prismatic models, the Newton–Raphson method was used for the integration procedure and a displacement control procedure was considered in which a vertical displacement δ was applied to the relevant reference points. The gravity load was included prior to applying the vertical displacement.

Fig. 6
figure 6

Representation of the 3D beam models, a schematic view, b view of the shear span, c cross section

Full size image

The p–δ load–displacement responses obtained from the numerical simulations on the three-dimensional beam geometry illustrated in Fig. 6, for the specimens described in Sect. 2.3, are shown in Figs. 7 and 8, whilst the main parameters are given in Table 3. The displacement values shown in the figures are those measured on the opposite face to the load application point, which corresponds to notation D1 in Fig. 1. Good agreement was obtained between the predictions and test strengths with a Ptest/Pnum = 1.01 and coefficient of variation COV of 0.12 for the cases with ls = 1400 mm moment span.

Fig. 7
figure 7

Comparative assessment between the load–displacement curves for Stage 1 configurations, a CH15/1, b CH20/1, c CH25/1, d CL15/1, e CL20/1, f CL25/1

Full size image
Fig. 8
figure 8

Comparative assessment between the load–displacement curves for Stage 2 configurations, a CH15/1, b CH20/1 (only numerical results), c CH25/1, d CL15/2, e CL20/2, f CL25/2

Full size image

Using the same material properties, it is shown that the numerical strengths are slightly larger than those from the second set of tests (ls = 560 mm) with a test-to-numerical average of 0.92, particularly since the test beams might have suffered micro-cracking from Stage 1 tests (ls = 1400 mm). It is worth noting that steel fibres have minimal influence on the compressive strength of the material in comparison with their influence on the tensile strength. Unsurprisingly, as indicated in Table 3, the changes in strength between the results obtained from modelling are relatively small. For low shear span-to-depth ratios, the shear capacity of reinforced concrete members is governed by the strut capacity and implicitly sensitive primarily to the compressive strength of the material.

The predicted strengths and tension damage patterns offer a clear insight into the governing ultimate behaviour. The P–δ plots show similar stiffnesses and sudden drops in capacity after reaching ultimate for most of the specimens, whilst the crack patterns in Fig. 2 are resembling the inclined tension plastic strain fields from Fig. 9. It is worth mentioning that such results were achieved with a relatively fine mesh within the shear span, in the range of lm = 10 mm that corresponds to a lm/h ratio of 0.04 and a mesh-independent uniaxial σ–ε input (Fig. 6).

Fig. 9
figure 9

Distribution of equivalent plastic strains at ultimate for: a Stage 1 loading scheme, b Stage 2 loading scheme

Full size image

The crack band model with constant fracture energy, in which the strain is a function of crack width and a characteristic element length le, was considered for the beam models [45]. For beam models, the numerical input was converted into inelastic cracking strain εct,i using the crack width values used for modelling the four-point bending tests. Following the numerical validation of the structural beams and prismatic members, a series of parametric investigations accounting for varying reinforcement ratios ρl and shear span-to-depth ratios a/d were conducted using the CDP constitutive parameters described above and listed in Table 4. An identical three-dimensional model having the same geometry and mesh as the beam models used for validations was employed for the parametric studies.

3.4 Parametric investigations

A total of 40 models incorporating four different longitudinal reinforcement ratios ρl = 0.85, 1.45, 2.00 and 2.50%, variations of the shear span-to-depth ratio a/d = 0.75, 1.25, 1.75, 2.25 and concrete constitutive properties of H15, H20 and H25 concrete were constructed. Model name adopts the format Cx–yyy–zzz in which x is the type of the fibre (H or L), yyy represents the shear span (e.g. 075 for a/d = 0.75), and zzz is for the reinforcement ratio (e.g. 145 for ρl = 1.45%). As shown in Fig. 10a, for the reduction in a/d ratio from 2.25 to 0.75, an increase in member capacity is observed, particularly due to the increase in the direct strut effect. For cases a/d < 1.0, it is typically assumed that the load is completely transferred to the support through the direct strut.

Fig. 10
figure 10

Numerical results: a influence of a/d ratio, b influence of reinforcement ratio to member capacity (P)

Full size image

On the other hand, as shown in Fig. 10b, a decrease in reinforcement ratio from ρl = 2.50 to ρl = 0.85% shows a reduction in ultimate capacity and as expected a relatively more flexible response. Unsurprisingly, a low reinforcement ratio (ρl = 0.85%) combined with a high shear span-to-depth ratio a/d = 2.25 resulted in flexural failure. This behaviour is also captured by the tension damage fields depicted in Fig. 11. By contrast, models with ρl > 2.00% and a/d < 1.25 resulted in shear-governed failures, whilst for combinations of ρl and a/d within the above ranges developed mixed-mode failures. In terms of concrete type, it is shown that the influence in members strength is directly proportional to the input strength properties. The results and observations obtained from parametric investigations are used for the design considerations proposed in the following section, noting that only models with shear failures and 1.00 < a/d < 2.50, representative for short a/d ratios, are considered.

Fig. 11
figure 11

Tension damage distributions at ultimate for selected models from the parametric investigations

Full size image

4 Design considerations

The following section introduces a method to assess the shear strength of UHPSFRC members subjected to concentrated loads in the vicinity of the support with shear span-to-depth ratios below a/d < 2.5, using the results and observations from the tests described in Sect. 2 and numerical simulations in Sect. 3, as well as the results of a collated data set tests from the literature [46,47,48,49,50,51]. The method employs a cumulative contribution of the concrete matrix (Vc) and that of the fibres (Vf) to the total shear capacity V (Eq. 6). The contribution of the concrete matrix represented through Eq. 7 [52] is dependent on the reinforcement strain at the intersection with the critical shear crack ε, a size effect factor which depends on the maximum aggregate size (d/ddg), as well as a k = 0.019 coefficient for the failure criterion [52]. It is worth noting, that due to the direct strut effect for members loaded within a/d < 2.5, strut-and-tie modelling can be considered in design [40, 53].

As mentioned before, the configurations investigated in this paper cover tests and numerical models with a/d < 2.5, whilst the concrete beam shear model was validated for cases in which a/d > 2.5, particularly since the theoretical strut carries a significant amount of total shear force. To account for the loads taken to the support through the direct strut, in the proposed method, the shear resistance is modified through an enhancement factor λβ that is based on typical assumptions in codified procedures, which consider an inverse reduction β factor applied to the design shear force [40, 53]. A detailed analysis of the results of a database with simply supported beams without shear reinforcement subjected to concentrated loads showed that enhancement factors around 2.5 × d/a would be appropriate and would always offer safe or overly conservative estimates, whilst 3.5 × d/a would fit the average representation [54].

Additionally, current shear models were typically validated to concrete strengths below those investigated in this paper. It is also known that for concrete under uniaxial compression, the deviation from elastic stiffness occurs later for high-strength concrete materials in comparison with normal or low strength, and this effect becomes more visible for UHPC. The latter behaves practically elastic nearly until crushing (σ ≈ 0.95 × fc). This response would enhance the capacity of load-carrying struts that can be characterised by undisturbed uniaxial compression states. Considering the above comments, the factor employed to account for the shear enhancement due to direct strut effect near the supports is λβ = 3.5 (Eq. 7b).

In terms of shear transfer actions, in conventional reinforced concrete, the forces are transferred though the compression zone, dowel action, residual stresses at the crack tip and aggregate interlock [55]. The aggregate interlock contribution is dependent on the roughness of the crack interface, aggregate type, their embedment depth in the cement paste, the magnitude of the slip and the opening of the two interfaces [16]. Several studies have shown that aggregate interlock plays a significant role as a shear carrying mechanism at low concrete compressive strengths, yet for high-strength concrete or fine powder concretes that do not contain any coarse aggregates, any contribution along cracked interfaces due to aggregate interlock, and/or shear friction, is greatly reduced [56, 57]. This is also supported by experimental evidence which showed that relatively straight cracks are characterised by smooth fracture planes and that the cracked interfaces typically pass through the aggregate particles [58]. Implicitly, in UHPC beams in shear, the aggregate interlock effect is limited or non-existent. To capture this effect, in analytical modelling the aggregate size dg is typically taken as zero for such members [59]. The strain in the reinforcement εs is calculated considering the acting bending moment MS at half shear span (x = a/2) from the load application point and accounts for the contribution of fibres to the moment capacity (Eqs. 7c, d). The contribution of fibres to bending capacity is averaged across the failure surface Af,b, whilst ff is the tensile strength provided by fibres as a product of the global orientation factor Kf,max, fibre aspect ratios αf, fibre volume ρf and the bond performance of embedded fibres τb, described below.

$$V = V_{\text{c}} + V_{\text{f}}$$
(6)
$$V_{\text{c}} = \frac{{\lambda_{\beta } kb_{\text{w}} d\sqrt {f_{\text{c}} } }}{{\sqrt {\varepsilon_{\text{s}} d/\left( {16 + d_{\text{g}} } \right)} }}$$
(7a)
$$\text{where}\quad \lambda_{\beta } = \left| \begin{aligned} 1.0 \to a_{\text{v}} /d > 2.5 \hfill \\ 3.5 \times \left( {d/a_{\text{v}} } \right) \to 1.0 \le a_{\text{v}} /d \le 2.5 \hfill \\ \end{aligned} \right.$$
(7b)
$$\varepsilon_{\text{s}} = \frac{{M_{\text{S}} - A_{\text{f,b}} f_{\text{f}} \left( {h/2} \right)}}{{A_{\text{s}} E_{\text{s}} \left( {d - c/2} \right)}}$$
(7c)
$$c = \frac{{A_{\text{s}} f_{\text{y}} + A_{\text{f,b}} f_{\text{f}} }}{{\lambda f_{\text{c}} b_{\text{w}} }}.$$
(7d)

In SFRC, besides the above mechanisms, the fibres bridge the crack interfaces acting as an additional strength enhancement mechanism. The shear capacity of high-performance fibre-reinforced concrete strongly depends on the amount of fibre in the concrete mixture [60]. Hence, the fibre contribution of the shear capacity depends on the fibre type, geometry, bond properties as well as the fibre orientation in the fractured plane (Eq. 8a), considering that the contribution of fibres is averaged over the failure surface [61]. The inclination of the fracture plane can be determined with Eq. 8b [16]. The global orientation factor Kf,max is determined from the assumption that all fibres are pulled out from the matrix using a crack width dependent formulation [62]. In design, for typical fibre ranges with aspect ratios αf = 20–120 and diameters df = 0.15–0.90 mm, Kf,max can be determined with Eq. 8c [57]. As expected, the bond performance τb of embedded fibres depends on their geometry, and as shown in Eq. 8e, it is a function of the square root of concrete strength. For hooked-end fibres, the λf parameter is in the range of λf = 1.0–1.5, whilst for straight fibres varies between λf = 0.6–0.9 [57, 62]. For the assessments in this paper, a nonlinear representation of λf, as a function of Vf is considered, in which the polynomial parameters of Eq. 8f are relevant for hooked-end fibres.

$$V_{\text{f}} = K_{\text{f,max}} \alpha_{\text{f}} \rho_{\text{f}} \tau_{\text{b}} b_{\text{w}} z\cot \theta$$
(8a)
$${\text{where}}\quad \tan \theta = 0.6 + \rho_{\text{l}} \frac{{{\text{f}}_{\text{ys}} }}{{f_{\text{c}} }}\left( {\frac{d}{1600}} \right)^{0.5}$$
(8b)
$$K_{\text{f,max}} = 0.5 - \left( {0.645/\alpha_{\text{f}}^{0.45} } \right)$$
(8c)
$$\alpha_{\text{f}} = l_{\text{f}} /d_{\text{f}}$$
(8d)
$$\tau_{\text{b}} = \lambda_{\text{f}} \sqrt {f_{\text{c}} }$$
(8e)
$$\lambda_{\text{f}} = 1 - 0.05 \times V_{\text{f}}^{2} .$$
(8f)

Estimates of shear strength V using Eqs. 6–8 are plotted in Fig. 12a against the shear strength Vtest of members tests from this paper and those from the collated database (Table 5) as well as the shear strength Vnum of models from the parametric investigations in Sect. 3.3. As observed from the figure, the estimates of the proposed constitutive equations are in reasonably good agreement with the test and numerical results with an overall average of test-to-predicted Vcalc/Vtest ratio of 1.15 and a coefficient of variance of 0.17. The comparative results between tests in this paper and database versus predictions show a Vcalc/Vtest ratio of 1.21, whilst between numerical results and predictions a Vcalc/Vtest of 1.10, respectively. Close inspection into the predictions offered by Eqs. (6–8) and those available in the literature indicates that the former are superior to the latter which typically offer either unconservative or overly conservative estimates. As shown in Fig. 12b, predictions of the test strengths using the model available in Model Code 2010 [41], which employs at its basis the Eurocode 2 [52] fundamentals with due account for the presence of the fibres, show a Vcalc/Vtest ratio of 1.52 and a COV in the range of 0.25.

Fig. 12
figure 12

Strength predictions: a Eqs. (6–8), b model code 2010 [41]

Full size image
Table 5 Database and predictions
Full size table

It is hence shown that the proposed assessment method results in relatively conservative estimates, particularly when compared with the test database and can be directly used in practice. However, it is worth noting that the predictions of the proposed expressions are limited to the ranges of the data set. For the shear span-to-depth ratios investigated in this paper, more refined results could be obtained by strut-and-tie design, provided that detailed information regarding the size of the support and load transfer plates is available. In practical design, the shear strength of a beam member is taken as the minimum between that of the sectional shear that can be assessed with Eqs. (6–8) and of that attributable to arching action can be predicted using strut-and-tie models [63].

5 Concluding remarks

The performance of ultra-high-performance steel fibre-reinforced concrete (UHPSFRC) subjected to loads at relatively low shear span-to-depth ratios was investigated in this study. The results from six UHPSFRC structural members provided with longitudinal reinforcement only, as well as corresponding material tests were reported and discussed in detail. Nonlinear numerical simulations were also carried out, and their results were compared to those from tests. The numerical models were used to carry out sensitivity studies with particular focus on the tensile constitutive response as well as the influence of key structural parameters to shear strength. The test results combined with those from numerical simulations and from a collated database from previous experimental studies enabled the development of a series of design expressions to estimate shear strength of UHPSFRC beams.

The experimental and numerical results showed that the shear strength and failure mode of such members is largely dependent on the fibre content, flexural reinforcement ratio and location of loading. An increase in hybrid fibre blend content from 1.5 to 2.0% indicated an enhancement in strength 17%, whilst an increase in fibre content from 1.5 to 2.55% showed a higher strength with about 24%, whilst previous studies indicated that from 0 to 2% the strength enhancement was twofold. More importantly, the members with higher fibre volume showed a flexurally governed shear failure, whilst those with 1.5% failed with limited or no sign of flexure. It is worth noting that similar trends were obtained from tests on flexural prisms.

The numerical simulations indicate that nonlinear models can be used to assess the tensile stress–strain curve of UHPSFRC from flexural tests as well as the strength and failure mode of structural members, provided that a refined mesh and characteristic constitutive parameters are accounted for. These showed that for the reduction in shear span-to-depth ratio from 2.25 to 0.75, an increase in capacity is observed due to the direct strut effect, whilst a reduction in the flexural reinforcement ratio shows a decrease in ultimate capacity and as expected a relatively more flexible response. Low reinforcement ratios combined with a high shear span-to-depth ratio would result in bending-governed failures, whilst high reinforcement ratios combined with short loaded spans would produce shear-governed failures. Considering the range of parameters accounted for in the parametric assessments along with those from the collated database, the expressions proposed offer a reliable design method and suitable for practical application.