Skip to main content
SpringerLink
Log in

Use of Angularity on Piezoelectric Crystal to Create Frequency Phase Shift for a Wide-Band Energy Harvester

  • Original Paper
  • Published:
Journal of Vibration Engineering & Technologies Aims and scope Submit manuscript

Abstract

Purpose

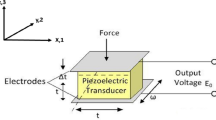
Piezoelectric materials, when positioned angularly with respect to their crystallographic axis, exhibit a fascinating phenomenon: the creation of a frequency phase shift (FPS) during resonance. Traditionally, FPS arises due to changes in shape and material properties, causing the resonance frequency point to shift. However, present research has revealed an unexplored avenue: when a piezoelectric crystal vibrates along an axis that deviates from the normal poling axis, it manifests FPS phenomena. This unique behavior can be harnessed for a wide-bandwidth energy harvester—a novel application that has not been investigated by other researchers.

Methods

Rather than delving into the theoretical nature of FPS, this study focuses on practical implementation of this phenomenon. Clear mathematical assumptions, coupled with experimental validation, confirm the significant impact of angular position on piezoelectric crystals. Overall, 3 methods of mathematical modeling (with explicit formulas in the appendix), experimental setup and finite element method (FEM) have been used in this study.

Results and Conclusions

The results from all the three methods for solving flexural motion demonstrate how power output depends on the angular position of the model and the dimensional variations of the beam and the piezoelectric material. The newly designed energy harvester produces 5 μWatt of power in both analytical and experimental approaches, under an acceleration of \(1 \, m{s}^{-2}\). Operating within a 20 Hz bandwidth for half-power level and utilizing two perpendicular beams, this design ensures that power output remains nonzero regardless of changes in the assembly’s direction of excitation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

References

  1. Kim T, Ko Y, Yoo C, Choi B, Han S, Kim N (2020) Design optimisation of wide-band piezoelectric energy harvesters for self-powered devices. Energy Convers Manag 225(September):113443. https://doi.org/10.1016/j.enconman.2020.113443

    Article  Google Scholar 

  2. Platt SR, Farritor S, Garvin K, Haider H (2005) The use of piezoelectric ceramics for electric power generation within orthopedic implants. IEEE/ASME Trans Mechatron 10(4):455–461. https://doi.org/10.1109/TMECH.2005.852482

    Article  Google Scholar 

  3. Xie X, Wang Q (2015) A mathematical model for piezoelectric ring energy harvesting technology from vehicle tires. Int J Eng Sci 94:113–127. https://doi.org/10.1016/j.ijengsci.2015.05.004

    Article  Google Scholar 

  4. Gatti G, Brennan MJ, Tehrani MG, Thompson DJ (2016) Harvesting energy from the vibration of a passing train using a single-degree-of-freedom oscillator. Mech Syst Signal Process 66–67:785–792. https://doi.org/10.1016/j.ymssp.2015.06.026

    Article  Google Scholar 

  5. Tianchen Y, Jian Y, Ruigang S, Xiaowei L (2014) Vibration energy harvesting system for railroad safety based on running vehicles. Smart Mater Struct. https://doi.org/10.1088/0964-1726/23/12/125046

    Article  Google Scholar 

  6. Bao B, Wang Q, Wu N, Zhou S (2021) Hand-held piezoelectric energy harvesting structure: design, dynamic analysis, and experimental validation. Meas J Int Meas Confed 174(December 2020):109011. https://doi.org/10.1016/j.measurement.2021.109011

    Article  Google Scholar 

  7. Hamid R, Yuce MR (2017) A wearable energy harvester unit using piezoelectric–electromagnetic hybrid technique. Sens Actuat, A Phys 257:198–207. https://doi.org/10.1016/j.sna.2017.02.026

    Article  Google Scholar 

  8. Xie XD, Wang Q, Wu N (2014) Energy harvesting from transverse ocean waves by a piezoelectric plate. Int J Eng Sci 81:41–48. https://doi.org/10.1016/j.ijengsci.2014.04.003

    Article  Google Scholar 

  9. Liu J et al (2020) Wind energy harvesting using piezoelectric macro fiber composites based on flutter mode. Microelectron Eng 231(January):111333. https://doi.org/10.1016/j.mee.2020.111333

    Article  Google Scholar 

  10. Rezaei-Hosseinabadi N, Tabesh A, Dehghani R, Aghili A (2015) An efficient piezoelectric windmill topology for energy harvesting from low-speed air flows. IEEE Trans Ind Electron 62(6):3576–3583. https://doi.org/10.1109/TIE.2014.2370933

    Article  Google Scholar 

  11. Jia J, Shan X, Upadrashta D, Xie T, Yang Y, Song R (2020) An asymmetric bending-torsional piezoelectric energy harvester at low wind speed. Energy 198:117287. https://doi.org/10.1016/j.energy.2020.117287

    Article  Google Scholar 

  12. Mehmood A, Abdelkefi A, Hajj MR, Nayfeh AH, Akhtar I, Nuhait AO (2013) Piezoelectric energy harvesting from vortex-induced vibrations of circular cylinder. J Sound Vib 332(19):4656–4667. https://doi.org/10.1016/j.jsv.2013.03.033

    Article  Google Scholar 

  13. Anton SR, Sodano HA (2007) A review of power harvesting using piezoelectric materials (2003–2006). Smart Mater Struct. https://doi.org/10.1088/0964-1726/16/3/R01

    Article  Google Scholar 

  14. Luo C, Hofmann HF (2011) Wideband energy harvesting for piezoelectric devices with linear resonant behavior. IEEE Trans Ultrason Ferroelectr Freq Control 58(7):1294–1301. https://doi.org/10.1109/TUFFC.2011.1949

    Article  Google Scholar 

  15. Gupta A, Panda S, Reddy RS (2024) Damping capabilities of viscoelastic composites for active/passive constrained layer damping of the plate vibration: a comparative study. J Vib Eng Technol 12(1):891–908. https://doi.org/10.1007/s42417-023-00882-z

    Article  Google Scholar 

  16. Gupta A, Panda S, Reddy RS (2021) Shear actuation-based hybrid damping treatment of sandwich structures using a graphite particle-filled viscoelastic layer. J Intell Mater Syst Struct 32(20):2477–2493. https://doi.org/10.1177/1045389X211002649

    Article  Google Scholar 

  17. Qi S, Shuttleworth R, Oyadiji SO, Wright J (2010) Design of a multiresonant beam for broadband piezoelectric energy harvesting. Smart Mater Struct. https://doi.org/10.1088/0964-1726/19/9/094009

    Article  Google Scholar 

  18. Yang Z, Yang J (2009) Connected vibrating piezoelectric bimorph beams as a wide-band piezoelectric power harvester. J Intell Mater Syst Struct 20(5):569–574. https://doi.org/10.1177/1045389X08100042

    Article  Google Scholar 

  19. Naifar S, Bradai S, Viehweger C, Kanoun O (2017) Survey of electromagnetic and magnetoelectric vibration energy harvesters for low frequency excitation. Meas J Int Meas Confed 106:251–263. https://doi.org/10.1016/j.measurement.2016.07.074

    Article  Google Scholar 

  20. Halim MA, Park JY (2015) Piezoceramic based wideband energy harvester using impact-enhanced dynamic magnifier for low frequency vibration. Ceram Int 41(S1):S702–S707. https://doi.org/10.1016/j.ceramint.2015.03.143

    Article  Google Scholar 

  21. Zhang H, Afzul K (2014) Design and analysis of a connected broadband multi-piezoelectric-bimorph-beam energy harvester. IEEE Trans Ultrason Ferroelectr Freq Control 61(6):1016–1023

    Article  Google Scholar 

  22. Tian L, Shen H, Yang Q, Song R, Bian Y (2023) A novel outer-inner magnetic two degree-of-freedom piezoelectric energy harvester. Energy Convers Manag 283(October 2022):116920. https://doi.org/10.1016/j.enconman.2023.116920

    Article  Google Scholar 

  23. Jackson N, Stam F, Olszewski OZ, Doyle H, Quinn A, Mathewson A (2016) Widening the bandwidth of vibration energy harvesters using a liquid-based non-uniform load distribution. Sens Actuat A Phys 246:170–179. https://doi.org/10.1016/j.sna.2016.04.063

    Article  Google Scholar 

  24. Cottone F, Vocca H, Gammaitoni L (2009) Nonlinear energy harvesting. Phys Rev Lett 102(8):80601. https://doi.org/10.1103/PhysRevLett.102.080601

    Article  Google Scholar 

  25. Zhao L, Yang Y (2018) An impact-based broadband aeroelastic energy harvester for concurrent wind and base vibration energy harvesting. Appl Energy 212(September 2017):233–243. https://doi.org/10.1016/j.apenergy.2017.12.042

    Article  Google Scholar 

  26. Gaertner WW, Schuller M, Heizman C, Levy G (1964) Micropower microelectronic subsystems. Pergamon, pp 57–84

    Google Scholar 

  27. Vullers RJM, van Schaijk R, Doms I, Van Hoof C, Mertens R (2009) Micropower energy harvesting. Solid State Electron 53(7):684–693. https://doi.org/10.1016/j.sse.2008.12.011

    Article  Google Scholar 

  28. Mirzaabedini S, Zhang H, Yenuganti S (2018) Analysis of angular position, dimension and material of a cantilever piezoelectric energy harvester. Ferroelectrics 537(1):1–19. https://doi.org/10.1080/00150193.2018.1528940

    Article  Google Scholar 

  29. Li P, Jin F, Yang J (2013) A piezoelectric energy harvester with increased bandwidth based on beam flexural vibrations in perpendicular directions. IEEE Trans Ultrason Ferroelectr Freq Control 60(10):2214–2218. https://doi.org/10.1109/TUFFC.2013.2813

    Article  Google Scholar 

  30. Xie Z et al (2023) A multimodal E-shaped piezoelectric energy harvester with a built-in bistability and internal resonance. Energy Convers Manag 278(November 2022):116717. https://doi.org/10.1016/j.enconman.2023.116717

    Article  Google Scholar 

  31. Yildirim T, Ghayesh MH, Li W, Alici G (2016) Design and development of a parametrically excited nonlinear energy harvester. Energy Convers Manag 126:247–255. https://doi.org/10.1016/j.enconman.2016.06.080

    Article  Google Scholar 

  32. Challa VR, Prasad MG, Shi Y, Fisher FT (2008) A vibration energy harvesting device with bidirectional resonance frequency tunability. Smart Mater Struct 17(1):15035. https://doi.org/10.1088/0964-1726/17/01/015035

    Article  Google Scholar 

  33. Huang C et al (2022) Origami dynamics based soft piezoelectric energy harvester for machine learning assisted self-powered gait biometric identification. Energy Convers Manag 263:115720. https://doi.org/10.1016/j.enconman.2022.115720

    Article  Google Scholar 

  34. Fan K-Q, Chao F-B, Zhang J-G, Wang W-D, Che X-H (2014) Design and experimental verification of a bi-directional nonlinear piezoelectric energy harvester. Energy Convers Manag 86:561–567. https://doi.org/10.1016/j.enconman.2014.06.021

    Article  Google Scholar 

  35. Tse FS, Morse IE, Hinkle RT (1978) Mechanical vibrations: theory and applications, second. Allyn and Bacon, Boston

    Google Scholar 

  36. American A, Standard N (1988) IEEE standard on piezoelectricity: an American National Standard. ANSI/IEEE STD 176–1987:11–25. https://doi.org/10.1109/IEEESTD.1988.79638

    Article  Google Scholar 

  37. Kholkin AL, Pertsev NA, Goltsev AV (2008) Piezoelectricity and crystal symmetry. https://api.semanticscholar.org/CorpusID:137313331

  38. Yang JS, Fang HY (2002) Analysis of a rotating elastic beam with piezoelectric films as an angular rate sensor. IEEE Trans Ultrason Ferroelectr Freq Control 49(6):798–804

    Article  Google Scholar 

  39. Lan C, Liao Y, Hu G, Tang L (2020) Equivalent impedance and power analysis of monostable piezoelectric energy harvesters. J Intell Mater Syst Struct 31(14):1697–1715. https://doi.org/10.1177/1045389X20930080

    Article  Google Scholar 

  40. Delnavaz A, Voix J (2014) Flexible piezoelectric energy harvesting from jaw movements. Smart Mater Struct 23(10):105020. https://doi.org/10.1088/0964-1726/23/10/105020

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Multidisciplinary Engineering, Texas A&M University, College Station, USA

    Sohrab MirzaAbedini

  2. Department of Mechanical Engineering, University of North Texas, Denton, USA

    Haifeng Zhang

Authors
  1. Sohrab MirzaAbedini
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Haifeng Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sohrab MirzaAbedini.

Ethics declarations

Conflict of Interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix

In these appendices, explicit form of the large equations is presented. These equations were abbreviated from the main context to make it easier to read and reach the achievements of this study. However, it can be of significant use for other researchers endeavoring mathematical calculations as below. These equations are all referenced in the main context.

Appendix A: Explicit forms of momentum equations:

$$\begin{aligned} & M_{211} = \mathop \int \limits_{S}^{{}} x_{2} T_{1} dA \\ & \quad = bE_{b} \mathop \int \limits_{{ - x_{n} - \frac{{t_{b} }}{2}}}^{{\frac{{t_{b} }}{2} - x_{n} }} x_{2} \left( { - u_{2,11} x_{2} - u_{3,11} x_{3} } \right)dx_{2} \\ & \quad \quad + b\mathop \int \limits_{{\frac{{{\text{t}}_{{\text{b}}} }}{2} - x_{{\text{n}}} }}^{{\frac{{t_{b} }}{2} + t_{p} - x_{n} }} x_{2} (s_{11}^{ - 1} \left( { - u_{2,11} x_{2} - u_{3,11} x_{3} - E_{3} d_{31}^{*} \cos^{ - 1} (\theta )} \right)dx_{2} \\ & = D_{211} u_{2,11} + G_{211} (V) \\ & M_{311} = \mathop \int \limits_{S}^{{}} x_{3} T_{1} dA = \left( {t_{b} + t_{p} } \right).E_{b} \mathop \int \limits_{{ - \frac{b}{2}}}^{\frac{b}{2}} - x_{3} \left( {u_{2,11} x_{2} + u_{3,11} x_{3} } \right)dx_{3} \\ & \quad + (t_{b} + t_{p} )\mathop \int \limits_{{ - {\text{b}}/2}}^{b/2} x_{3} (s_{11}^{ - 1} \left( { - u_{2,11} x_{2} - u_{3,11} x_{3} - d_{31}^{*} \cos^{ - 1} (\theta )E_{l} } \right)dx_{3} = D_{311} u_{3,11} + G_{311} \left( V \right) \\ \end{aligned}$$
(21)

Explicit form of momentum equations for where \({l}_{1}<{x}_{1}<{l}_{2}:\)

$$\begin{aligned} & M_{221} = - bE_{b} \mathop \int \limits_{{ - \frac{{t_{b} }}{2}}}^{{\frac{{t_{b} }}{2}}} x_{2} \left( {u_{2,11} x_{2} + u_{3,11} x_{3} } \right)dx_{2} = D_{221} u_{2,11} + G_{221} V \\ & M_{321} = - t_{b} E_{b} \mathop \int \limits_{ - b/2}^{b/2} x_{3} \left( {u_{2,11} x_{2} + u_{3,11} x_{3} } \right)dx_{3} = D_{321} u_{3,11} + G_{321} V \\ \end{aligned}$$
(22)

For the 2nd beam, the following momentum equations exist:

$$\begin{aligned} & M_{312} = \mathop \int \limits_{S}^{{}} x_{3} T_{1} dA \\ & \quad = cE_{b} \mathop \int \limits_{{ - x_{n} - \frac{{t_{b} }}{2}}}^{{\frac{{t_{b} }}{2} - x_{n} }} x_{3} \left( { - u_{2,11} x_{2} - u_{3,11} x_{3} } \right)dx_{3} \\ & \quad \quad + c\mathop \int \limits_{{\frac{{{\text{t}}_{{\text{b}}} }}{2} - x_{{\text{n}}} }}^{{\frac{{t_{b} }}{2} + t_{p} - x_{n} }} x_{3} (s_{11}^{ - 1} (\left( { - u_{2,11} x_{2} - u_{3,11} x_{3} - E_{3} d_{31}^{*} \cos^{ - 1} (\theta )} \right)dx_{3} \\ & \quad = D_{312} u_{3,11} + G_{312} (V) \\ & M_{212} = \mathop \int \limits_{S}^{{}} x_{2} T_{1} dA \\ & \quad = \left( {t_{b} + t_{p} } \right).\mathop \int \limits_{{ - \frac{c}{2}}}^{\frac{c}{2}} x_{2} \left( { - u_{2,11} x_{2} - u_{3,11} x_{3} } \right)dx_{2} \\ & \quad \quad + \mathop \int \limits_{{ - \frac{{\text{c}}}{2}}}^{\frac{c}{2}} x_{2} (s_{11}^{ - 1} (\left( { - u_{2,11} x_{2} - u_{3,11} x_{3} - d_{31}^{*} \cos^{ - 1} (\theta )E_{3} } \right)dx_{2} \\ & \quad = D_{212} u_{3,11} + G_{212} (V) \\ & M_{322} = - cE_{b} \mathop \int \limits_{{ - \frac{{t_{b} }}{2}}}^{{\frac{{t_{b} }}{2}}} x_{3} \left( {u_{2,11} x_{2} + u_{3,11} x_{3} } \right)dx_{3} = D_{322} u_{3,11} + G_{322} V \\ & M_{222} = - cE_{b} \mathop \int \limits_{{ - \frac{c}{2}}}^{\frac{c}{2}} x_{2} \left( {u_{2,11} x_{2} + u_{3,11} x_{3} } \right)dx_{2} = D_{222} u_{3,11} + G_{222} V \\ \end{aligned}$$
(23)

Appendix B: Equations for the boundary conditions are:

Displacement B.C. at x1 = 0

\({u}_{211}(0,t)=Asin\theta {\text{exp}}(i\omega t)\)

(24)

 

\({u}_{311}(0,t)=Acos\theta {\text{exp}}(i\omega t)\)

 

\({u}_{212}\left(0,t\right)=Acos\theta {\text{exp}}(i\omega t)\)

 

\({u}_{312}\left(0,t\right)=Asin\theta {\text{exp}}\left(i\omega t\right)\)

Velocity B.C. at x1 = 0

\({u}_{\mathrm{211,1}}\left(0,t\right)=0\)

 

\({u}_{\mathrm{311,1}}\left(0,t\right)=0\)

 

\({u}_{212}\left(0,t\right)=0\)

 

\({u}_{312}\left(0,t\right)=0\)

Displacement B.C. at \({L}_{1}\)

\({u}_{211}\left({L}_{1},t\right)={u}_{221}({L}_{1},t)\)

 

\({u}_{311}\left({L}_{1},t\right)={u}_{321}({L}_{1},t)\)

 

\({u}_{212}\left({L}_{1},t\right)={u}_{222}({L}_{1},t)\)

 

\({u}_{312}\left({L}_{1},t\right)={u}_{322}({L}_{1},t)\)

Velocity B.C. at \({L}_{1}\)

\({u}_{\mathrm{211,1}}\left({L}_{1},t\right)={u}_{\mathrm{221,1}}({L}_{1},t)\)

 

\({u}_{\mathrm{311,1}}\left({L}_{1},t\right)={u}_{\mathrm{321,1}}({L}_{1},t)\)

 

\({u}_{\mathrm{212,1}}\left({L}_{1},t\right)={u}_{\mathrm{222,1}}({L}_{1},t)\)

 

\({u}_{\mathrm{312,1}}\left({L}_{1},t\right)={u}_{\mathrm{322,1}}({L}_{1},t)\)

Momentum B.C at \({L}_{1}\)

\({M}_{211}\left({L}_{1},t\right)={M}_{221}({L}_{1},t)\)

Which equals:\(-{D}_{211}.{u}_{\mathrm{211,11}}=-{D}_{221}.{u}_{\mathrm{221,11}}\)

 

\({M}_{311}\left({L}_{1},t\right)={M}_{321}({L}_{1},t)\)

 

\({M}_{212}\left({L}_{1},t\right)={M}_{222}({L}_{1},t)\)

 

\({M}_{312}\left({L}_{1},t\right)={M}_{322}({L}_{1},t)\)

Shear B.C. at \({L}_{1}\)

\({N}_{211}\left({L}_{1},t\right)={N}_{221}({L}_{1},t)\)

Which equals:\(-{D}_{211}.{u}_{\mathrm{211,111}}=-{D}_{221}.{u}_{\mathrm{221,111}}\)

 

\({N}_{311}\left({L}_{1},t\right)={N}_{321}({L}_{1},t)\)

 

\({N}_{212}\left({L}_{1},t\right)={N}_{222}({L}_{1},t)\)

 

\({N}_{312}\left({L}_{1},t\right)={N}_{322}({L}_{1},t)\)

Momentum B.C. at \({L}_{1}+{L}_{2}\)

\({M}_{221}\left({L}_{1}+{L}_{2},t\right)=0\)

 

\({M}_{321}({L}_{1}+{L}_{2},t)=0\)

 

\({M}_{222}\left({L}_{1}+{L}_{2},t\right)=0\)

 

\({M}_{322}({L}_{1}+{L}_{2},t)=0\)

Shear at \({L}_{2}\)

\(-{N}_{221}\left({L}_{2},t\right)={m}_{0}{\ddot{u}}_{221}\left({L}_{2},t\right)\)

 

\(-{N}_{321}\left({L}_{2},t\right)={m}_{0}{\ddot{u}}_{321}\left({L}_{2},t\right)\)

 

\(-{N}_{222}\left({L}_{2},t\right)={m}_{0}{\ddot{u}}_{222}\left({L}_{2},t\right)\)

 

\(-{N}_{322}\left({L}_{2},t\right)={m}_{0}{\ddot{u}}_{322}\left({L}_{2},t\right)\)

Complex notation for time-harmonic motion:

$$\left\{ {u_{211} ,u_{311} ,u_{221} ,u_{321} ,u_{212} ,u_{312} ,u_{222} ,u_{322} ,V,Q,I} \right\} = Re\{ \left\{ {f_{1} ,f_{2} ,f_{3} ,f_{4} ,f_{5} ,f_{5} ,f_{6} ,f_{7} ,f_{8} ,\overline{V},\overline{Q},\overline{I}} \right\}\exp \left( {i\omega t} \right)\}$$
(25)

The general solutions to (15) for the 1st and 2nd beams:

$$\begin{gathered} f1 = B_{1} \sin \left( {\alpha x_{1} } \right) + B_{2} \cos \left( {\alpha x_{1} } \right) + B_{3} \sinh \left( {\alpha x_{1} } \right) + B_{4} \cosh (\alpha x_{1} ) \hfill \\ f2 : = B_{5} \sin (\beta x_{1} ) + B_{6} \cos (\beta x_{1} ) + B_{7} \sinh (\beta x_{1} ) + B_{8} \cosh (\beta x_{1} ) \hfill \\ f3 : = B_{9} \sin (\gamma x_{1} ) + B_{10} \cos (\gamma x_{1} ) + B_{11} \sinh (\gamma x_{1} ) + B_{12} \cosh (\gamma x_{1} ) \hfill \\ f4 : = B_{13} \sin (\delta x_{1} ) + B_{14} \cos (\delta x_{1} ) + B_{15} \sinh (\delta x_{1} ) + B_{16} \cosh (\delta x_{1} ) \hfill \\ f5 : = B_{17} \sin (\smallint x_{1} ) + B_{18} \cos (\smallint x_{1} ) + B_{19} \sinh (\smallint x_{1} ) + B_{20} \cosh (\smallint x_{1} ) \hfill \\ f6 : = B_{21} \sin (\zeta x_{1} ) + B_{22} \cos (\zeta x\_1) + B_{23} \sinh (\zeta x_{1} ) + B_{24} \cosh (\zeta x_{1} ) \hfill \\ f7 : = B_{25} \sin (\eta x_{1} ) + B_{26} \cos (\eta x_{1} ) + B_{27} \sinh (\eta x_{1} ) + B_{28} \cosh (\eta x_{1} ) \hfill \\ f8 : = B_{29} \sin (\kappa x_{1} ) + B_{30} \cos (\kappa x_{1} ) + B_{31} \sinh (\kappa x_{1} ) + B_{32} \cosh (\kappa x_{1} ) \hfill \\ \end{gathered}$$
(26)

In (26):

$$\begin{gathered} \alpha = \left( {\frac{{m_{1} \omega^{2} }}{{D_{1} }}} \right)^{\frac{1}{4}} ,\beta = \left( {\frac{{m_{2} \omega^{2} }}{{D_{2} }}} \right)^{\frac{1}{4}} ,\gamma = \left( {\frac{{m_{3} \omega^{2} }}{{D_{3} }}} \right)^{\frac{1}{4}} , \delta = \left( {\frac{{m_{4} \omega^{2} }}{{D_{4} }}} \right)^{\frac{1}{4}} , \in = \left( {\frac{{m_{5} \omega^{2} }}{{D_{5} }}} \right)^{\frac{1}{4}} , \hfill \\ \zeta = \left( {\frac{{m_{6} \omega^{2} }}{{D_{6} }}} \right)^{\frac{1}{4}} ,\eta = \left( {\frac{{m_{7} \omega^{2} }}{{D_{7} }}} \right)^{\frac{1}{4}} ,\kappa = \left( {\frac{{m_{8} \omega^{2} }}{{D_{8} }}} \right)^{\frac{1}{4}} \hfill \\ \end{gathered}$$
(27)

Solutions for \({B}_{1}-{B}_{32}\):

$$\begin{aligned} & B_{2} + B_{4} = Asin(\theta ) \\ & B_{6} + B_{8} = Acos(\theta ) \\ & B_{1} \alpha + B_{3} \alpha = 0 \\ & B_{5} \beta + B_{7} \beta = 0 \\ \end{aligned}$$
(28)
$$\begin{aligned} B_{1} sin(\alpha L_{1} ) + B_{2} cos(\alpha L_{1} ) + B_{3} sinh(\alpha L_{1} ) + B_{4} cosh(\alpha L_{1} ) = B_{9} sin(\gamma L_{1} ) + B_{10} cos(\gamma L_{1} ) + B_{11} sinh(\gamma L_{1} ) + B_{12} cosh(\gamma L_{1} )\end{aligned}$$
$$\begin{aligned} B_{5} sin\left( {\beta L1} \right) + B6*cos\left( {\beta L_{1} } \right) + B_{7} sinh\left( {\beta L_{1} } \right) + B_{8} cosh\left( {\beta L_{1} } \right) = B_{13} sin\left( {\delta L_{1} } \right) + B_{14} cos\left( {\delta L_{1} } \right) + B_{15} sinh\left( {\delta L_{1} } \right) + B_{16} cosh(\delta L_{1} ) \end{aligned}$$
$$\begin{aligned} B_{1} cos\left( {\alpha L_{1} } \right)\alpha - B_{2} sin\left( {\alpha L_{1} } \right)\alpha + B_{3} cosh\left( {\alpha L_{1} } \right)\alpha + B_{4} sinh\left( {\alpha L_{1} } \right)\alpha = B_{9} cos\left( {\gamma L_{1} } \right)\gamma - B_{10} sin\left( {\gamma L_{1} } \right)\gamma + B_{11} cosh\left( {\gamma L_{1} } \right)\gamma + B_{12} sinh\left( {\gamma L_{1} } \right)\gamma \end{aligned}$$
$$\begin{aligned} B_{5} cos\left( {\beta L_{1} } \right)\beta - B_{6} sin\left( {\beta L_{1} } \right)\beta + B_{7} cosh\left( {\beta L_{1} } \right)\beta + B_{8} sinh\left( {\beta L_{1} } \right)\beta = B_{13} cos\left( {\delta L_{1} } \right)\delta - B_{14} sin\left( {\delta L_{1} } \right)\delta + B_{15} cosh\left( {\delta L_{1} } \right)\delta + B_{16} sinh\left( {\delta L_{1} } \right)\delta \end{aligned}$$
$$\begin{aligned} & - D_{{211}} \left( { - B_{1} ~\sin \left( {\alpha L_{1} } \right)\alpha ^{2} - B_{2} \cos \left( {\alpha L_{1} } \right)\alpha ^{2} ~ + B_{3} \sinh \left( {\alpha L_{1} } \right)\alpha ^{2} + B_{4} \cosh \left( {\alpha L_{1} } \right)\alpha ^{2} } \right) + D_{{31}} E_{l} G_{{211}} /s_{{11}} ~ \\ & = - D_{{1221}} \left( { - B_{9} \sin \left( {\gamma L_{1} } \right)\gamma ^{2} - B_{{10}} \cos \left( {\gamma L_{1} } \right)\gamma ^{2} + B_{{11}} \sinh \left( {\gamma L_{1} } \right)\gamma ^{2} + B_{{12}} \cosh \left( {\gamma L_{1} } \right)\gamma ^{2} } \right) \\ \end{aligned}$$
$$\begin{aligned} & - D_{{311}} ( - B_{5} \sin \left( {\beta L_{1} } \right)\beta ^{2} - B_{6} \cos \left( {\beta L_{1} } \right)\beta ^{2} + B_{7} \sinh \left( {\beta L_{1} } \right)\beta ^{2} + B_{8} \cosh \left( {\beta L_{1} } \right)\beta ^{2} ) + D_{{31}} E_{l} G_{{311}} /s_{{11}} ~ \\ & = ~ - D_{{321}} ( - B_{{13}} \sin \left( {\delta L_{1} } \right)\delta ^{2} - B_{{14}} \cos \left( {\delta L_{1} } \right)\delta ^{2} + B_{{15}} \sinh \left( {\delta L_{1} } \right)\delta ^{2} + B_{{16}} \cosh \left( {\delta L_{1} } \right)\delta ^{2} ) \\ \end{aligned}$$
$$\begin{aligned} & - D_{{211}} ( - B_{1} \cos \left( {\alpha L_{1} } \right)\alpha ^{3} ~ + B_{2} \sin \left( {\alpha L_{1} } \right)\alpha ^{3} + B_{3} \cosh \left( {\alpha L_{1} } \right)\alpha ^{3} + B_{4} \sinh \left( {\alpha L_{1} } \right)\alpha ^{3} ) \\ & = ~ - D_{{221}} ( - B_{9} \cos \left( {\gamma L_{1} } \right)\gamma ^{3} + B_{{10}} \sin \left( {\gamma L_{1} } \right)\gamma ^{3} + ~B_{{11}} \cosh \left( {\gamma L_{1} } \right)\gamma ^{3} + B_{{12}} \sinh \left( {\gamma L_{1} } \right)\gamma ^{3} ) \\ \end{aligned}$$
$$\begin{aligned} & - D_{{311}} ( - B_{5} \cos \left( {\beta L_{1} } \right)\beta ^{3} + B_{6} \sin \left( {\beta L_{1} } \right)\beta ^{3} + B_{7} \cosh \left( {\beta L_{1} } \right)\beta ^{3} + B_{8} \sinh \left( {\beta L_{1} } \right)\beta ^{3} ) \\ & = ~ - D_{{321}} ( - B_{{13}} \cos \left( {\delta L_{1} } \right)\delta ^{3} + B_{{14}} \sin \left( {\delta L_{1} } \right)\delta ^{3} + B_{{15}} \cosh \left( {\delta L_{1} } \right)\delta ^{3} + B_{{16}} \sinh \left( {\delta L_{1} } \right)\delta ^{3} ) \\ \end{aligned}$$
$$\begin{aligned} - D_{221} ( - B_{9} sin\left( {\gamma \left( {L_{1} + L_{2} } \right)} \right)\gamma^{2} - B_{10} cos\left( {\gamma \left( {L_{1} + L_{2} } \right)} \right)\gamma^{2} + B_{11} sinh\left( {\gamma \left( {L_{1} + L_{2} } \right)} \right)\gamma^{2} + B_{12} cosh\left( {\gamma \left( {L_{1} + L_{2} } \right)} \right)\gamma^{2} ) = 0 \end{aligned}$$
$$\begin{aligned} - D_{321} ( - B_{13} sin\left( {\delta \left( {L_{1} + L_{2} } \right)} \right)\delta^{2} - B_{14} cos\left( {\delta \left( {L_{1} + L_{2} } \right)} \right)\delta^{2} + B_{15} sinh\left( {\delta \left( {L_{1} + L_{2} } \right)} \right)\delta^{2} + B_{16} cosh\left( {\delta \left( {L_{1} + L_{2} } \right)} \right)\delta^{2} ) = 0 \end{aligned}$$
$$\begin{aligned} - D_{221} \left( { - B_{9} cos\left( {\gamma L_{2} } \right)\gamma^{3} + B_{10} sin\left( {\gamma L_{2} } \right)\gamma^{3} + B_{11} cosh\left( {\gamma L_{2} } \right)\gamma^{3} + B_{12} sinh\left( {\gamma L_{2} } \right)\gamma^{3} } \right) = m_{0} \omega^{2} (B_{9} sin(\gamma L_{2} ) + B_{10} cos(\gamma L_{2} ) + B_{11} sinh(\gamma L_{2} ) + B_{12} cosh(\gamma L_{2} )) \end{aligned}$$
$$\begin{aligned} - D_{321} ( - B_{13} cos\left( {\delta L_{2} } \right)\delta^{3} + B_{14} sin\left( {\delta L_{2} } \right)\delta^{3} + B_{15} cosh\left( {\delta L_{2} } \right)\delta^{3} + B_{16} sinh\left( {\delta L_{2} } \right)\delta^{3} ) = m_{0} \omega^{2} (B_{13} sin(\delta L_{2} ) + B_{14} cos(\delta L_{2} ) + B_{15} sinh(\delta L_{2} ) + B_{16} cosh(\delta L_{2} )) \end{aligned}$$
$$B_{18} + B_{20} = A.cos(\theta )$$
$$B_{22} + B_{24} = A.sin(\theta )$$
$$B_{17} \in + B_{19} \in = 0$$
$$B_{21} \zeta + B_{23} \zeta = 0$$
$$\begin{aligned} & B_{{17}} \sin ( \in L_{1} ) + B_{{18}} \cos ( \in L_{1} ) + B_{{19}} \sinh ( \in L_{1} ) + B_{{20}} \cosh ( \in L_{1} )~ \\ & = B_{{25}} \sin (\eta L_{1} ) + B_{{26}} \cos \left( {\eta L_{1} } \right) + B_{{27}} \sinh (\eta L_{1} ) + B_{{28}} \cosh \left( {\eta L_{1} } \right) \\ \end{aligned}$$
$$\begin{aligned} & B_{{21}} \sin (\zeta L_{1} ) + B_{{22}} \cos (\zeta L_{1} ) + B_{{23}} \sinh (\zeta L_{1} ) + B_{{24}} \cosh (\zeta L_{1} )~ \\ & = B_{{29}} \sin (\kappa L_{1} ) + B_{{30}} \cos (\kappa L_{1} ) + B_{{31}} \sinh (\kappa L_{1} ) + B_{{32}} \cosh (\kappa L_{1} ) \\ \end{aligned}$$
$$\begin{aligned} & B_{{17}} \cos \left( { \in L_{1} } \right) \in - B_{{18}} \sin \left( { \in L_{1} } \right) \in + B_{{19}} \cosh \left( { \in L_{1} } \right) \in + B_{{20}} \sinh \left( { \in L_{1} } \right) \in ~ \\ & = B_{{25}} \cos \left( {\eta L_{1} } \right)\eta - B_{{26}} \sin \left( {\eta L_{1} } \right)\eta + B_{{27}} \cosh \left( {\eta L_{1} } \right)\eta + B_{{28}} \sinh \left( {\eta L_{1} } \right)\eta \\ \end{aligned}$$
$$\begin{aligned} & B_{{21}} \cos \left( {\zeta L_{1} } \right)\zeta - B_{{22}} \sin \left( {\zeta L_{1} } \right)\zeta + B_{{23}} \cosh \left( {\zeta L_{1} } \right)\zeta + B_{{24}} \sinh \left( {\zeta L_{1} } \right)\zeta ~ \\ & = B_{{29}} \cos \left( {\kappa L_{1} } \right)\kappa - B_{{30}} \sin \left( {\kappa L_{1} } \right)\kappa + B_{{31}} \cosh \left( {\kappa L_{1} } \right)\kappa + B_{{32}} \sinh \left( {\kappa L_{1} } \right)\kappa \\ \end{aligned}$$
$$\begin{gathered} - D_{{312}} \left( { - B_{{17}} \sin \left( { \in L_{1} } \right) \in ^{2} - B_{{18}} \cos \left( { \in L_{1} } \right) \in ^{2} + B_{{19}} \sinh \left( { \in L_{1} } \right) \in ^{2} + B_{{20}} \cosh \left( { \in L_{1} } \right) \in ^{2} } \right) + d_{{31}} E_{l} G_{{312}} /s_{{11}} ~ \hfill \\ = - D_{{322}} \left( { - B_{{25}} \sin \left( {\eta L_{1} } \right)\eta ^{2} - B_{{26}} \cos \left( {\eta L_{1} } \right)\eta ^{2} + B_{{27}} \sinh \left( {\eta L_{1} } \right)\eta ^{2} + B_{{28}} \cosh \left( {\eta L_{1} } \right)\eta ^{2} } \right) \hfill \\ \end{gathered}$$
$$\begin{gathered} - D_{{212}} \left( { - B_{{21}} \sin \left( {\zeta L_{1} } \right)\zeta ^{2} - B_{{22}} \cos \left( {\zeta L_{1} } \right)\zeta ^{2} + B_{{23}} \sinh \left( {\zeta L_{1} } \right)\zeta ^{2} + B_{{24}} \cosh \left( {\zeta L_{1} } \right)\zeta ^{2} } \right) + d_{{31}} E_{l} G_{{212}} /s_{{11}} ~ \hfill \\ = - D_{{222}} \left( { - B_{{29}} \sin \left( {\kappa L_{1} } \right)\kappa ^{2} - B_{{30}} \cos \left( {\kappa L_{1} } \right)\kappa ^{2} + B_{{31}} \sinh \left( {\kappa L_{1} } \right)\kappa ^{2} + B_{{32}} \cosh \left( {\kappa L_{1} } \right)\kappa ^{2} } \right) \hfill \\ \end{gathered}$$
$$\begin{aligned} & - D312\left( { - B_{{17}} \cos \left( { \in L_{1} } \right) \in ^{3} + B_{{18}} \sin \left( {\smallint L_{1} } \right) \in ^{3} + B_{{19}} \cosh \left( { \in L_{1} } \right) \in ^{3} + B_{{20}} \sinh \left( { \in L_{1} } \right) \in ^{3} } \right)~ \\ & = - D_{{322}} \left( { - B_{{25}} \cos \left( {\eta L_{1} } \right)\eta ^{3} + B_{{26}} \sin \left( {\eta L_{1} } \right)\eta ^{3} + B_{{27}} \cosh \left( {\eta L_{1} } \right)\eta ^{3} + B_{{28}} \sinh \left( {\eta L_{1} } \right)\eta ^{3} } \right) \\ \end{aligned}$$
$$\begin{aligned} & - D_{{212}} \left( { - B_{{21}} \cos \left( {\zeta L_{1} } \right)\zeta ^{3} + B_{{22}} \sin \left( {\zeta L_{1} } \right)\zeta ^{3} + B_{{23}} \cosh \left( {\zeta L_{1} } \right)\zeta ^{3} + B_{{24}} \sinh \left( {\zeta L_{1} } \right)\zeta ^{3} } \right)~ \\ & = - D_{{222}} \left( { - B_{{29}} \cos \left( {\kappa L_{1} } \right)\kappa ^{3} + B_{{30}} \sin \left( {\kappa L_{1} } \right)\kappa ^{3} + B_{{31}} \cosh \left( {\kappa L_{1} } \right)\kappa ^{3} + B_{{32}} \sinh \left( {\kappa L_{1} } \right)\kappa ^{3} } \right) \\ \end{aligned}$$
$$- D_{322} ( - B_{25} \sin \left( {\eta \left( {L_{1} + L_{2} } \right)} \right)\eta^{2} - B_{26} \cos \left( {\eta \left( {L_{1} + L_{2} } \right)} \right)\eta^{2} + B_{27} \sinh \left( {\eta \left( {L_{1} + L_{2} } \right)} \right)\eta^{2} + B_{28} \cosh \left( {\eta \left( {L_{1} + L_{2} } \right)} \right)\eta^{2} ) = 0$$
$$\begin{aligned} - D_{222} ( - B_{29} \sin \left( {\kappa \left( {L_{1} + L_{2} } \right)} \right)\kappa^{2} - B_{30} \cos \left( {\kappa \left( {L_{1} + L_{2} } \right)} \right)\kappa^{2} + B_{31} \sinh \left( {\kappa \left( {L_{1} + L_{2} } \right)} \right)\kappa^{2} + B_{32} \cosh \left( {\kappa \left( {L_{1} + L_{2} } \right)} \right)\kappa^{2} ) = 0 \end{aligned}$$
$$\begin{aligned} & - D_{{322}} \left( { - B_{{25}} \cos \left( {\eta L_{2} } \right)\eta ^{3} + B_{{26}} \sin \left( {\eta L_{2} } \right)\eta ^{3} + B_{{27}} \cosh \left( {\eta L_{2} } \right)\eta ^{3} + B_{{28}} \sinh \left( {\eta L_{2} } \right)\eta ^{3} } \right) \\ & = m_{0} \omega ^{2} \left( {B_{{25}} \sin (\eta L_{2} ) + B_{{26}} \cos (\eta L_{2} ) + B_{{27}} \sinh (\eta L_{2} ) + B_{{28}} \cosh (\eta L_{2} )} \right) \\ \end{aligned}$$
$$\begin{aligned} & - D_{{222}} \left( { - B_{{29}} \cos \left( {\kappa L_{2} } \right)\kappa^{3} + B_{{30}} \sin \left( {\kappa L_{2} } \right)\kappa^{3} + B_{{31}} \cosh \left( {\kappa L_{2} } \right)\kappa^{3} + B_{{32}} \sinh \left( {\kappa L_{2} } \right)\kappa^{3} } \right) \\ & = ~m_{0} \omega^{2} \left( {B_{{29}} \sin (\kappa L_{2} ) + B_{{30}} \cos (\kappa L_{2} ) + B_{{31}} \sinh (\kappa L_{2} ) + B_{{32}} \cosh (\kappa L_{2} )} \right) \\ \end{aligned}$$

Voltage equations of the first and second beam, individually:

$$\begin{gathered} - i\omega \left\{ {\frac{{bd_{31} }}{{s_{11} }}\left( {t_{b} + t_{p} } \right)\left[ {B_{1} \alpha \cos \alpha L_{1} - B_{2} \alpha \sin \alpha L_{1} + B_{3} \alpha \cosh \alpha L_{1} + B_{4} \alpha \sinh \alpha L_{1} - \alpha B_{1} - \alpha B_{3} } \right] + \overline{{\varepsilon^{*} }}_{33} \sin^{ - 1} (\theta )\frac{{\overline{V}}}{{t_{p} }}bL_{1} } \right\} \hfill \\ - i\omega \left\{ {\frac{{bd_{31}^{*} \cos^{ - 1} (\theta )}}{{s_{11} }}\left( {t_{b} + t_{p} } \right)\left[ {B_{5} \beta \cos \beta L_{1} - B_{6} \beta \sin \beta L_{1} + B_{8} \beta \cosh \beta L_{1} + B_{9} \beta \sinh \beta L_{1} - \beta B_{5} - \beta B_{7} } \right] + \overline{{\varepsilon^{*} }}_{33} \sin^{ - 1} (\theta )\frac{{\overline{V}}}{{t_{p} }}\left( {t_{b} + t_{p} } \right)L_{1} } \right\} \hfill \\ = \frac{{\overline{V}}}{{Z_{L} }} \hfill \\ \end{gathered}$$
(29)
$$\begin{gathered} - i\omega \left\{ {\frac{{cd_{31} }}{{s_{11} }}\left( {t_{b} + t_{p} } \right)\left[ {B_{17} \in \cos \in L_{1} - B_{18} \in \sin \in L_{1} + B_{19} \in \cosh \in L_{1} + B_{20} \in \sinh \in L_{1} - \in B_{17} - \in B_{19} } \right] + \overline{{\varepsilon^{*} }}_{33} \sin^{ - 1} (\theta )\frac{{\overline{V}}}{{t_{p} }}cL_{1} } \right\} \hfill \\ - i\omega \left\{ {\frac{{cd_{31}^{*} \cos^{ - 1} (\theta )}}{{s_{11} }}\left( {t_{b} + t_{p} } \right)\left[ {B_{21} \zeta \cos \zeta L_{1} - B_{22} \zeta \sin \zeta L_{1} + B_{23} \zeta \cosh \zeta L_{1} + B_{24} \zeta \sinh \zeta L_{1} - \zeta B_{21} - \zeta B_{23} } \right] + \overline{{\varepsilon^{*} }}_{33} \sin^{ - 1} (\theta )\frac{{\overline{V}}}{{t_{p} }}\left( {t_{b} + t_{p} } \right)L_{1} } \right\} \hfill \\ = \frac{{\overline{V}}}{{Z_{L} }} \hfill \\ \end{gathered}$$
(30)

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

MirzaAbedini, S., Zhang, H. Use of Angularity on Piezoelectric Crystal to Create Frequency Phase Shift for a Wide-Band Energy Harvester. J. Vib. Eng. Technol. (2024). https://doi.org/10.1007/s42417-024-01355-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s42417-024-01355-7

Keywords

Search

Navigation