Skip to main content
SpringerLink
Log in

Effect of Magnetic Field on Vibration of Electrorheological Fluid Nanoplates with FG-CNTRC Layers

  • Original Paper
  • Published:
Journal of Vibration Engineering & Technologies Aims and scope Submit manuscript

Abstract

Background

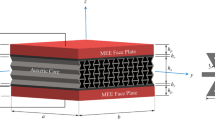
Functionally graded carbon nanotube-reinforced composites (FG-CNTRCs) have attracted significant attention in the field of structural engineering due to their unique mechanical properties.

Objective

In this study, the free vibration of a functionally graded carbon nanotube-reinforced composite sandwich nanoplate with an electrorheological fluid (ERF) layer as its core under a longitudinal magnetic field is examined using the nonlocal elasticity theory.

Results

A comparison with some other item of existing literature is used to evaluate the established solution's accuracy and correctness. The obtained results demonstrate the dependency of the vibration behavior on the aforementioned factors. Specifically, the influences of electric field strength, boundary conditions, magnetic field intensity, volume fraction of carbon nanotubes (CNTs), CNTs distribution, and nonlocal parameter are comprehensively analyzed through a parametric study.

Methods

The governing equations are derived based on Hamilton's principle and solved using the Galerkin technique. While the continuity of physical quantities is required between all layers, the rule of mixing allows us to analyze the distribution of characteristics in this system's thickness direction. Changing the electric field also alters the ERF parameters in the pre-yield region. The developed mathematical model incorporates the nonlocal elasticity theory and the third-order shear deformation theory (TSDT) for different boundary conditions.

Conclusion

The findings contribute to a better understanding of the dynamic response of such composite structures and can aid in their optimal design for various engineering applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data Availability

Data sharing not applicable.

References

  1. Soong TT, Costantinou MC (2014) Passive and active structural vibration control in civil engineering. Springer

  2. Tang J, Wang K-W (2001) Active-passive hybrid piezoelectric networks for vibration control: comparisons and improvement. Smart Mater Struct 10:794

    Google Scholar 

  3. Clark WW (2000) Vibration control with state-switched piezoelectric materials. J Intell Mater Syst Struct 11:263–271

    Google Scholar 

  4. Bonnecaze RT, Brady JF (1992) Dynamic simulation of an electrorheological fluid. J Chem Phys 96:2183–2202

    Google Scholar 

  5. Hao T (2001) Electrorheological fluids. Adv Mater 13:1847–1857

    Google Scholar 

  6. Kolekar S, Venkatesh K, Oh J-S, Choi S-B (2019) Vibration controllability of sandwich structures with smart materials of electrorheological fluids and magnetorheological materials: a review. J Vib Eng Technol 7:359–377

    Google Scholar 

  7. Halsey TC (1992) Electrorheological fluids. Science (80–) 258:761–766

    Google Scholar 

  8. Stangroom JE (1983) Electrorheological fluids. Phys Technol 14:290

    Google Scholar 

  9. Ruzicka M (2007) Electrorheological fluids: modeling and mathematical theory. Springer

  10. Yeh J-Y, Chen L-W (2004) Vibration of a sandwich plate with a constrained layer and electrorheological fluid core. Compos Struct 65:251–258

    Google Scholar 

  11. Wei K, Meng G, Zhang W, Zhou S (2007) Vibration characteristics of rotating sandwich beams filled with electrorheological fluids. J Intell Mater Syst Struct 18:1165–1173

    Google Scholar 

  12. Yeh J-Y (2011) Vibration and damping analysis of orthotropic cylindrical shells with electrorheological core layer. Aerosp Sci Technol 15:293–303

    Google Scholar 

  13. Arani AG, Jamali SA, Zarei HB (2017) Differential quadrature method for vibration analysis of electro-rheological sandwich plate with CNT reinforced nanocomposite facesheets subjected to electric field. Compos Struct 180:211–220

    Google Scholar 

  14. Gholamzadeh Babaki MH, Shakouri M (2021) Free and forced vibration of sandwich plates with electrorheological core and functionally graded face layers. Mech Based Des Struct Mach 49:689–706

    Google Scholar 

  15. Shahali P, Haddadpour H, Shakhesi S (2022) Dynamic analysis of electrorheological fluid sandwich cylindrical shells with functionally graded face sheets using a semi-analytical approach. Compos Struct. https://doi.org/10.1016/j.compstruct.2022.115715

    Article  Google Scholar 

  16. Khorshidi K, Soltannia B, Karimi M, Ghorbani A (2023) Nonlinear vibration of electro-rheological sandwich plates, coupled to quiescent fluid. Ocean Eng 271:113730

    Google Scholar 

  17. Ghavidel N, Alibeigloo A (2023) Free vibration analysis of cylindrical sandwich panel with electro-rheological core and FG-GPLRC facing sheets based on First order shear deformation theory referred by Qatu. J Vib Control. https://doi.org/10.1177/10775463221148535

    Article  Google Scholar 

  18. Dresselhaus MS, Dresselhaus G, Eklund PC, Rao AM (2000) Carbon nanotubes. In: Phys. Fullerene-Based Fullerene-Related Mater. Springer, pp. 331–379

  19. Dai H (2002) Carbon nanotubes: opportunities and challenges. Surf Sci 500:218–241

    Google Scholar 

  20. Iijima S, Ichihashi T (1993) Single-shell carbon nanotubes of 1-nm diameter. Nature 363:603

    Google Scholar 

  21. Thongchom C, Roodgar Saffari P, Roudgar Saffari P, Refahati N, Sirimontree S, Keawsawasvong S, Titotto S (2022) Dynamic response of fluid-conveying hybrid smart carbon nanotubes considering slip boundary conditions under a moving nanoparticle. Mech Adv Mater Struct. https://doi.org/10.1080/15376494.2022.2051101

    Article  Google Scholar 

  22. Chang T, Geng J, Guo X (2006) Prediction of chirality-and size-dependent elastic properties of single-walled carbon nanotubes via a molecular mechanics model. Proc R Soc A Math Phys Eng Sci 462:2523–2540

    Google Scholar 

  23. Ghavanloo E, Fazelzadeh SA (2012) Vibration characteristics of single-walled carbon nanotubes based on an anisotropic elastic shell model including chirality effect. Appl Math Model 36:4988–5000

    Google Scholar 

  24. Strozzi M, Smirnov VV, Pellicano F, Kovaleva M (2022) Nonlocal anisotropic elastic shell model for vibrations of double-walled carbon nanotubes under nonlinear van der Waals interaction forces. Int J Non Linear Mech 146:104172

    Google Scholar 

  25. Mallick PK (2007) Fiber-reinforced composites: materials, manufacturing, and design. CRC press

  26. Joshi SV, Drzal LT, Mohanty AK, Arora S (2004) Are natural fiber composites environmentally superior to glass fiber reinforced composites? Compos Part A Appl Sci Manuf 35:371–376

    Google Scholar 

  27. Li X, Tabil LG, Panigrahi S (2007) Chemical treatments of natural fiber for use in natural fiber-reinforced composites: a review. J Polym Environ 15:25–33

    Google Scholar 

  28. Saffari PR, Sirimontree S, Thongchom C, Jearsiripongkul T, Saffari PR, Keawsawasvong S (2023) Effect of uniform and nonuniform temperature distributions on sound transmission loss of double-walled porous functionally graded magneto-electro-elastic sandwich plates with subsonic external flow. Int J Thermofluids. https://doi.org/10.1016/j.ijft.2023.100311

    Article  Google Scholar 

  29. Sirimontree S, Thongchom C, Saffari PR, Refahati N, Saffari PR, Jearsiripongkul T, Keawsawasvong S (2023) Effects of thermal environment and external mean flow on sound transmission loss of sandwich functionally graded magneto-electro-elastic cylindrical nanoshell. Eur J Mech 97:104774

    MathSciNet  Google Scholar 

  30. Thongchom C, Saffari PR, Refahati N, Saffari PR, Pourbashash H, Sirimontree S, Keawsawasvong S (2022) An analytical study of sound transmission loss of functionally graded sandwich cylindrical nanoshell integrated with piezoelectric layers. Sci Rep 12:1–16

    Google Scholar 

  31. Thongchom C, Jearsiripongkul T, Refahati N, Roudgar Saffari P, Roodgar Saffari P, Sirimontree S, Keawsawasvong S (2022) Sound transmission loss of a honeycomb sandwich cylindrical shell with functionally graded porous layers. Buildings 12:151

    Google Scholar 

  32. Thongchom C, Refahati N, Roodgar Saffari P, Roudgar Saffari P, Niyaraki MN, Sirimontree S, Keawsawasvong S (2021) An experimental study on the effect of nanomaterials and fibers on the mechanical properties of polymer composites. Buildings 12:7

    Google Scholar 

  33. Shen H-S (2009) Nonlinear bending of functionally graded carbon nanotube-reinforced composite plates in thermal environments. Compos Struct 91:9–19

    Google Scholar 

  34. Liew KM, Lei ZX, Zhang LW (2015) Mechanical analysis of functionally graded carbon nanotube reinforced composites: a review. Compos Struct 120:90–97

    Google Scholar 

  35. Thang PT, Nguyen T-T, Lee J (2017) A new approach for nonlinear buckling analysis of imperfect functionally graded carbon nanotube-reinforced composite plates. Compos Part B Eng 127:166–174

    Google Scholar 

  36. Mehar K, Panda SK, Dehengia A, Kar VR (2016) Vibration analysis of functionally graded carbon nanotube reinforced composite plate in thermal environment. J Sandw Struct Mater 18:151–173

    Google Scholar 

  37. Ansari R, Torabi J, Shojaei MF (2017) Buckling and vibration analysis of embedded functionally graded carbon nanotube-reinforced composite annular sector plates under thermal loading. Compos Part B Eng 109:197–213

    Google Scholar 

  38. Nguyen TN, Thai CH, Nguyen-Xuan H, Lee J (2018) NURBS-based analyses of functionally graded carbon nanotube-reinforced composite shells. Compos Struct 203:349–360

    Google Scholar 

  39. Daikh AA, Houari MSA, Belarbi MO, Chakraverty S, Eltaher MA (2021) Analysis of axially temperature-dependent functionally graded carbon nanotube reinforced composite plates. Eng Comput. https://doi.org/10.1007/s00366-021-01413-8

    Article  Google Scholar 

  40. Huan DT, Quoc TH, Van Tham V, Binh CT (2022) Vibration characteristics of functionally graded carbon nanotube-reinforced composite plates submerged in fluid medium. In: Mod. Mech. Appl., Springer, pp 271–286

  41. Chen CQ, Shi Y, Zhang YS, Zhu J, Yan YJ (2006) Size dependence of Young’s modulus in ZnO nanowires. Phys Rev Lett 96:075505. https://doi.org/10.1103/PhysRevLett.96.075505

    Article  Google Scholar 

  42. Stan G, Ciobanu CV, Parthangal PM, Cook RF (2007) Diameter-dependent radial and tangential elastic moduli of ZnO nanowires.https://doi.org/10.1021/NL071986E

  43. Hutchinson J, Fleck N (1997) Strain gradient plasticity. Adv Appl Mech 33:295–361

    Google Scholar 

  44. Gao H, Huang Y, Nix WD, Hutchinson J (1999) Mechanism-based strain gradient plasticity—I. Theory. J Mech Phys Solids 47:1239–1263

    MathSciNet  Google Scholar 

  45. Eringen AC (1972) Linear theory of nonlocal elasticity and dispersion of plane waves. Int J Eng Sci 10:425–435. https://doi.org/10.1016/0020-7225(72)90050-X

    Article  Google Scholar 

  46. Eringen AC (1983) On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J Appl Phys 54:4703–4710. https://doi.org/10.1063/1.332803

    Article  Google Scholar 

  47. Arefi M, Bidgoli EM-R, Dimitri R, Bacciocchi M, Tornabene F (2019) Nonlocal bending analysis of curved nanobeams reinforced by graphene nanoplatelets. Compos Part B Eng 166:1–12

    Google Scholar 

  48. Fleck NA, Hutchinson J (1993) A phenomenological theory for strain gradient effects in plasticity. J Mech Phys Solids 41:1825–1857

    MathSciNet  Google Scholar 

  49. Lam DCC, Yang F, Chong ACM, Wang J, Tong P (2003) Experiments and theory in strain gradient elasticity. J Mech Phys Solids 51:1477–1508. https://doi.org/10.1016/S0022-5096(03)00053-X

    Article  Google Scholar 

  50. Jamalpoor A, Hosseini M (2015) Biaxial buckling analysis of double-orthotropic microplate-systems including in-plane magnetic field based on strain gradient theory. Compos Part B Eng 75:53–64. https://doi.org/10.1016/j.compositesb.2015.01.026

    Article  Google Scholar 

  51. Hosseini M, Bahreman M, Jamalpoor A (2016) Using the modified strain gradient theory to investigate the size-dependent biaxial buckling analysis of an orthotropic multi-microplate system. Acta Mech. https://doi.org/10.1007/s00707-016-1570-0

    Article  MathSciNet  Google Scholar 

  52. Jamalpoor A, Ahmadi-Savadkoohi A, Hosseini-Hashemi S (2016) Free vibration and biaxial buckling analysis of magneto-electro-elastic microplate resting on visco-Pasternak substrate via modified strain gradient theory. Smart Mater Struct 25:105035. https://doi.org/10.1088/0964-1726/25/10/105035

    Article  Google Scholar 

  53. Yang F, Chong ACM, Lam DCC, Tong P (2002) Couple stress based strain gradient theory for elasticity. Int J Solids Struct 39:2731–2743. https://doi.org/10.1016/S0020-7683(02)00152-X

    Article  Google Scholar 

  54. Park SK, Gao X-L (2006) Bernoulli–Euler beam model based on a modified couple stress theory. J Micromech Microeng 16:2355–2359. https://doi.org/10.1088/0960-1317/16/11/015

    Article  Google Scholar 

  55. Lim CW, Zhang G, Reddy JN (2015) A higher-order nonlocal elasticity and strain gradient theory and its applications in wave propagation. J Mech Phys Solids 78:298–313. https://doi.org/10.1016/j.jmps.2015.02.001

    Article  MathSciNet  Google Scholar 

  56. Roodgar Saffari P, Fakhraie M, Roudbari MA (2021) Size-dependent vibration problem of two vertically-aligned single-walled boron nitride nanotubes conveying fluid in thermal environment via nonlocal strain gradient shell model. J Solid Mech

  57. Roodgar Saffari P, Fakhraie M, Roudbari MA (2020) Free vibration problem of fluid-conveying double-walled boron nitride nanotubes via nonlocal strain gradient theory in thermal environment. Mech Based Des Struct Mach:1–18

  58. Saffari PR, Fakhraie M, Roudbari MA (2020) Nonlinear vibration of fluid conveying cantilever nanotube resting on visco-pasternak foundation using non-local strain gradient theory. Micro Nano Lett 15:181–186

    Google Scholar 

  59. Aghababaei R, Reddy JN (2009) Nonlocal third-order shear deformation plate theory with application to bending and vibration of plates. J Sound Vib 326:277–289. https://doi.org/10.1016/J.JSV.2009.04.044

    Article  Google Scholar 

  60. Phung-Van P, Lieu QX, Nguyen-Xuan H, Abdel Wahab M (2017) Size-dependent isogeometric analysis of functionally graded carbon nanotube-reinforced composite nanoplates. Compos Struct 166:120–135. https://doi.org/10.1016/J.COMPSTRUCT.2017.01.049

    Article  Google Scholar 

  61. Phung-Van P, Thanh C-L, Nguyen-Xuan H, Abdel-Wahab M (2018) Nonlinear transient isogeometric analysis of FG-CNTRC nanoplates in thermal environments. Compos Struct 201:882–892. https://doi.org/10.1016/J.COMPSTRUCT.2018.06.087

    Article  Google Scholar 

  62. Thai CH, Tran TD, Phung-Van P (2020) A size-dependent moving Kriging meshfree model for deformation and free vibration analysis of functionally graded carbon nanotube-reinforced composite nanoplates. Eng Anal Bound Elem 115:52–63

    MathSciNet  Google Scholar 

  63. Reddy JN (1984) A simple higher-order theory for laminated composite plates

  64. Hosseini M, Jamalpoor A (2015) Analytical solution for thermomechanical vibration of double-viscoelastic nanoplate-systems made of functionally graded materials. J Therm Stress 38:1428–1456. https://doi.org/10.1080/01495739.2015.1073986

    Article  Google Scholar 

  65. Murmu T, Pradhan SC (2009) Thermo-mechanical vibration of a single-walled carbon nanotube embedded in an elastic medium based on nonlocal elasticity theory. Comput Mater Sci 46:854–859

    Google Scholar 

  66. Murmu T, Pradhan SC (2009) Small-scale effect on the free in-plane vibration of nanoplates by nonlocal continuum model. Phys E Low-Dimen Syst Nanostruct 41:1628–1633

    Google Scholar 

  67. Phung-Van P, Abdel-Wahab M, Liew KM, Bordas SPA, Nguyen-Xuan H (2015) Isogeometric analysis of functionally graded carbon nanotube-reinforced composite plates using higher-order shear deformation theory. Compos Struct 123:137–149

    Google Scholar 

  68. Amir S (2019) Orthotropic patterns of visco-Pasternak foundation in nonlocal vibration of orthotropic graphene sheet under thermo-magnetic fields based on new first-order shear deformation theory. Proc Inst Mech Eng Part L J Mater Des Appl 233:197–208

    Google Scholar 

  69. Refahati N, Jearsiripongkul T, Thongchom C, Saffari PR, Saffari PR, Keawsawasvong S (2022) Sound transmission loss of double-walled sandwich cross-ply layered magneto-electro-elastic plates under thermal environment. Sci Rep 12:1–15

    Google Scholar 

  70. Zarabimanesh Y, Roodgar Saffari P, Roudgar Saffari P, Refahati N (2022) Hygro-thermo-mechanical vibration of two vertically aligned single-walled boron nitride nanotubes conveying fluid. J Vib Control 28:2101–2120

    MathSciNet  Google Scholar 

  71. Hadji L, Avcar M, Civalek Ö (2021) An analytical solution for the free vibration of FG nanoplates. J Braz Soc Mech Sci Eng 43:1–14

    Google Scholar 

  72. Ghahnavieh S, Hosseini-Hashemi S, Rajabi K (2018) A higher-order nonlocal strain gradient mass sensor based on vibrating heterogeneous magneto-electro-elastic nanoplate via third-order shear deformation theory. Eur Phys J Plus 133:518

    Google Scholar 

  73. Hasheminejad SM, Maleki M (2009) Free vibration and forced harmonic response of an electrorheological fluid-filled sandwich plate. Smart Mater Struct 18:55013

    Google Scholar 

Download references

Acknowledgements

This study was supported by Thammasat Postdoctoral Fellowship, Thammasat University research Division, Thammasat University. In addition, this research was supported by Thammasat University research Unit in Structural and Foundation Engineering, Thammasat University.

Funding

This research received no external funding.

Author information

Authors and Affiliations

  1. Department of Civil Engineering, Thammasat School of Engineering, Faculty of Engineering, Thammasat University, Pathumthani, 12121, Thailand

    Peyman Roodgar Saffari, Chanachai Thongchom & Sayan Sirimontree

  2. Department of Engineering, School of Physics, Engineering and Computer Science, University of Hertfordshire, Hatfield, AL10 9AB, England, UK

    Sikiru Oluwarotimi Ismail

  3. Department of Mechanical Engineering, Faculty of Engineering, Thammasat School of Engineering, Thammasat University, Pathumthani, 12121, Thailand

    Thira Jearsiripongkul

Authors
  1. Peyman Roodgar Saffari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sikiru Oluwarotimi Ismail
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Chanachai Thongchom
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Sayan Sirimontree
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Thira Jearsiripongkul
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

PRS: conceptualization, data curation, formal analysis, project administration, software, investigation, validation, supervision, and writing—review and editing. SOI: conceptualization, formal analysis, project administration, software, investigation, validation, supervision, writing—review and editing, and writing—original draft. CT: conceptualization, data curation, formal analysis, project administration, visualization, investigation, validation, supervision, and writing—review and editing. SS: investigation, data curation, validation, and writing—original draft. TJ: investigation, software, validation, and formal analysis. All the authors have read and agreed to the published version of the manuscript.

Corresponding authors

Correspondence to Peyman Roodgar Saffari, Sikiru Oluwarotimi Ismail or Chanachai Thongchom.

Ethics declarations

Conflict of Interest

The authors declare no conflict of interest.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A

The governing equations of the sandwich nanoplate are provided as

$$\delta u_{0t} \to \frac{{\partial N_{xx}^{t} }}{\partial x} + \frac{{\partial N_{xy}^{t} }}{\partial y} - \frac{{3Q_{xz}^{c} }}{{4h_{2} }} + \frac{{9C_{1} R_{xz}^{c} }}{{4h_{2} }} = I_{0}^{t} \ddot{u}_{0t} + \overline{I}_{1}^{t} - I_{3}^{t} C_{1} \frac{{\partial \ddot{W}_{0} }}{\partial x},$$
(A1)
$$\delta u_{0b} \to \frac{{\partial N_{xx}^{b} }}{\partial x} + \frac{{\partial N_{xy}^{b} }}{\partial y} + \frac{{3Q_{xz}^{c} }}{{4h_{2} }} - \frac{{9C_{1} R_{xz}^{c} }}{{4h_{2} }} = I_{0}^{b} \ddot{u}_{0b} + \overline{I}_{1}^{b} \ddot{\theta }_{xb} - I_{3}^{b} C_{1} \frac{{\partial \ddot{W}_{0} }}{\partial x},$$
(A2)
$$\delta v_{0t} \to \frac{{\partial N_{yy}^{t} }}{\partial y} + \frac{{\partial N_{xy}^{t} }}{\partial x} - \frac{{3Q_{yz}^{c} }}{{4h_{2} }} + \frac{{9C_{1} R_{yz}^{c} }}{{4h_{2} }} = I_{0}^{t} \ddot{v}_{0t} + \overline{I}_{1}^{t} \ddot{\theta }_{yt} - I_{3}^{t} C_{1} \frac{{\partial \ddot{W}_{0} }}{\partial y},$$
(A3)
$$\delta v_{0b} \to \frac{{\partial N_{yy}^{b} }}{\partial y} + \frac{{\partial N_{xy}^{b} }}{\partial x} + \frac{{3Q_{yz}^{c} }}{{4h_{2} }} - \frac{{9C_{1} R_{yz}^{c} }}{{4h_{2} }} = I_{0}^{b} \ddot{v}_{0b} + \overline{I}_{1}^{b} \ddot{\theta }_{yb} - I_{3}^{b} C_{1} \frac{{\partial \ddot{W}_{0} }}{\partial y},$$
(A4)
$$\delta {W}_{0}\to \frac{\partial {Q}_{xz}^{t}}{\partial x}+\frac{\partial {Q}_{xz}^{b}}{\partial x}+\frac{\partial {Q}_{yz}^{t}}{\partial y}+\frac{\partial {Q}_{yz}^{b}}{\partial y}-3{C}_{1}\left(\frac{\partial {R}_{xz}^{t}}{\partial x}+\frac{\partial {R}_{yz}^{t}}{\partial y}\right)-3{C}_{1}\left(\frac{\partial {R}_{xz}^{b}}{\partial x}+\frac{\partial {R}_{yz}^{b}}{\partial y}\right)+{C}_{1} \left(\frac{{\partial }^{2}{P}_{xx}^{t}}{\partial {x}^{2}}+2\frac{{\partial }^{2}{P}_{xy}^{t}}{\partial x\partial y}+\frac{{\partial }^{2}{P}_{yy}^{t}}{\partial {y}^{2}}\right)+{C}_{1}\left(\frac{{\partial }^{2}{P}_{xx}^{b}}{\partial {x}^{2}}+2\frac{{\partial }^{2}{P}_{xy}^{b}}{\partial x\partial y}+\frac{{\partial }^{2}{P}_{yy}^{b}}{\partial {y}^{2}}\right) +\left(\frac{3}{4}-\frac{{h}_{1}}{8{h}_{2}}-\frac{{h}_{3}}{8{h}_{2}}\right) \left(\frac{\partial {Q}_{xz}^{c}}{\partial x}+\frac{\partial {Q}_{yz}^{c}}{\partial y}\right)-\left(\frac{9}{4}-\frac{{3h}_{1}}{8{h}_{2}}-\frac{{3h}_{3}}{8{h}_{2}}\right) {C}_{1}\left(\frac{\partial {R}_{xz}^{c}}{\partial x}+\frac{\partial {R}_{yz}^{c}}{\partial y}\right)=q+\left({I}_{0}^{t}+{I}_{0}^{c}+{I}_{0}^{b}\right){\ddot{W}}_{0}+{I}_{3}^{t}{C}_{1}\left(\frac{\partial {\ddot{u}}_{0t}}{\partial x}+\frac{\partial {\ddot{v}}_{0t}}{\partial y}\right)+{I}_{3}^{b}{C}_{1}\left(\frac{\partial {\ddot{u}}_{0b}}{\partial x}+\frac{\partial {\ddot{v}}_{0b}}{\partial y}\right)-\left({I}_{6}^{t}+{I}_{3}^{b}\right) {C}_{1}^{2}\left(\frac{{\partial }^{2}{\ddot{W}}_{0}}{\partial {x}^{2}}+\frac{{\partial }^{2}{\ddot{W}}_{0}}{\partial {y}^{2}}\right)+{\bar{I}}_{4}^{t}{C}_{1}\left(\frac{\partial {\ddot{\theta }}_{xt}}{\partial x}+\frac{\partial {\ddot{\theta }}_{yt}}{\partial y}\right)+{\bar{I}}_{4}^{b}{C}_{1}\left(\frac{\partial {\ddot{\theta }}_{xb}}{\partial x}+\frac{\partial {\ddot{\theta }}_{yb}}{\partial y}\right),$$
(A5)
$$\delta \theta_{xt} \to \frac{{\partial M_{xx}^{t} }}{\partial x} + \frac{{\partial M_{xy}^{t} }}{\partial y} - C_{1} \left( {\frac{{\partial P_{xx}^{t} }}{\partial x} + \frac{{\partial P_{xy}^{t} }}{\partial y}} \right) - Q_{xz}^{t} + 3C_{1} R_{xz}^{t} + \frac{{h_{1} Q_{xz}^{c} }}{{2h_{2} }} - \frac{{3C_{1} h_{1} R_{xz}^{c} }}{{2h_{2} }} = \overline{I}_{1}^{t} \ddot{u}_{0t} + \overline{I}_{2}^{t} \ddot{\theta }_{xt} - C_{1} \overline{I}_{4}^{t} \frac{{\partial \ddot{W}_{0} }}{\partial x},$$
(A6)
$$\delta \theta_{xb} \to \frac{{\partial M_{xx}^{b} }}{\partial x} + \frac{{\partial M_{xy}^{b} }}{\partial y} - C_{1} \left( {\frac{{\partial P_{xx}^{b} }}{\partial x} + \frac{{\partial P_{xy}^{b} }}{\partial y}} \right) - Q_{xz}^{b} + 3C_{1} R_{xz}^{b} + \frac{{h_{3} Q_{xz}^{c} }}{{2h_{2} }} - \frac{{3C_{1} h_{3} R_{xz}^{c} }}{{2h_{2} }} = \overline{I}_{1}^{b} \ddot{u}_{0b} + \overline{I}_{2}^{b} \ddot{\theta }_{xb} - C_{1} \overline{I}_{4}^{b} \frac{{\partial \ddot{W}_{0} }}{\partial x},$$
(A7)
$$\delta \theta_{yt} \to \frac{{\partial M_{yy}^{t} }}{\partial y} + \frac{{\partial M_{xy}^{t} }}{\partial x} - C_{1} \left( {\frac{{\partial P_{yy}^{t} }}{\partial y} + \frac{{\partial P_{xy}^{t} }}{\partial x}} \right) - Q_{yz}^{t} + 3C_{1} R_{yz}^{t} + \frac{{h_{1} Q_{yz}^{c} }}{{2h_{2} }} - \frac{{3C_{1} h_{1} R_{yz}^{c} }}{{2h_{2} }} = \overline{I}_{1}^{t} \ddot{v}_{0t} + \overline{I}_{2}^{t} \ddot{\theta }_{yt} - C_{1} \overline{I}_{4}^{t} \frac{{\partial \ddot{W}_{0} }}{\partial y},$$
(A8)
$$\delta \theta_{yb} \to \frac{{\partial M_{yy}^{b } }}{\partial y} + \frac{{\partial M_{xy}^{b} }}{\partial x} - C_{1} \left( {\frac{{\partial P_{yy}^{b} }}{\partial y} + \frac{{\partial P_{xy}^{b} }}{\partial x}} \right) - Q_{yz}^{t} + 3C_{1} R_{yz}^{b} + \frac{{h_{3} Q_{yz}^{c} }}{{2h_{2} }} - \frac{{3C_{1} h_{3} R_{yz}^{c} }}{{2h_{2} }} = \overline{I}_{1}^{b} \ddot{v}_{0b} + \overline{I}_{2}^{b} \ddot{\theta }_{yb} - C_{1} \overline{I}_{4}^{b} \frac{{\partial \ddot{W}_{0} }}{\partial y},$$
(A9)

where

$$\begin{gathered} \left( {I_{0}^{t} ,I_{1}^{t} ,I_{2}^{t} ,I_{3}^{t} ,I_{4}^{t} ,I_{6}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \rho \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz, \hfill \\ \left( {I_{0}^{b} ,I_{1}^{b} ,I_{2}^{b} ,I_{3}^{b} ,I_{4}^{b} ,I_{6}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \rho \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz, I_{0}^{c} = \mathop \int \limits_{{ - h_{2} /2}}^{{h_{2} /2}} \rho_{f} dz , \hfill \\ \end{gathered}$$
(A10)
$$\overline{I}_{1}^{t} = I_{1}^{t} - I_{3}^{t} C_{1} , \overline{I}_{2}^{t} = I_{2}^{t} - 2C_{1} I_{4}^{t} + C_{1}^{2} I_{6}^{t} , \overline{I}_{4}^{t} = I_{4}^{t} - C_{1} I_{6}^{t} ,$$
$$\overline{I}_{1}^{b} = I_{1}^{b} - I_{3}^{b} C_{1} , \overline{I}_{2}^{b} = I_{2}^{b} - 2C_{1} I_{4}^{b} + C_{1}^{2} I_{6}^{b} , \overline{I}_{4}^{b} = I_{4}^{b} - C_{1} I_{6}^{b} ,$$
$$\left( {N_{xx}^{t} ,M_{xx}^{t} ,P_{xx}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \sigma_{xxt} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {N_{xy}^{t} ,M_{xy}^{t} ,P_{xy}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \tau_{xyt} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {N_{yy}^{t} ,M_{yy}^{t} ,P_{yy}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \sigma_{yyt} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {Q_{xz}^{t} ,R_{xz}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \tau_{xzt} \left( {1,z^{2} } \right)dz,\left( {Q_{xz}^{t} ,R_{xz}^{t} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} \tau_{xzt} \left( {1,z^{2} } \right)dz,$$
$$\left( {N_{xx}^{b} ,M_{xx}^{b} ,P_{xx}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \sigma_{xxb} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {N_{xy}^{b} ,M_{xy}^{b} ,P_{xy}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \tau_{xyb} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {N_{yy}^{b} ,M_{yy}^{b} ,P_{yy}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \sigma_{yyb} \left( {1,z,z^{3} } \right)dz,$$
$$\left( {Q_{xz}^{b} ,R_{xz}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \tau_{xzb} \left( {1,z^{2} } \right)dz,\left( {Q_{xz}^{b} ,R_{xz}^{b} } \right) = \mathop \int \limits_{{ - h_{3} /2}}^{{h_{3} /2}} \tau_{xzb} \left( {1,z^{2} } \right)dz$$
$$\left( {Q_{xz}^{c} ,R_{xz}^{c} } \right) = \mathop \int \limits_{{ - h_{2} /2}}^{{h_{2} /2}} \tau_{xzc} \left( {1,z^{2} } \right)dz, \left( {Q_{yz}^{c} ,R_{yz}^{c} } \right) = \mathop \int \limits_{{ - h_{2} /2}}^{{h_{2} /2}} \tau_{yzc} \left( {1,z^{2} } \right)dz.$$

Appendix B

The size-dependent governing partial differential equations are provided as

$$\delta {u}_{0t}\to {A}_{11}\left(\frac{{\partial }^{2}{u}_{0t}}{\partial {x}^{2}}\right)+{A}_{66}\left(\frac{{\partial }^{2}{u}_{0t}}{\partial {y}^{2}}\right)+\left[{A}_{12}+{A}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0t}}{\partial x\partial y}\right)+\left[{B}_{11}-{C}_{1}{E}_{11}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial {x}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial {y}^{2}}\right)+\left[{B}_{12}+{B}_{66}-{C}_{1}{E}_{12}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial x\partial y}\right)-\left[{C}_{1}{E}_{12}+2{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial x\partial {y}^{2}}\right)-{C}_{1}{E}_{11}\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{3}}\right)-\left(\frac{3}{4{h}_{2}}{f}_{1}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{4}\right)\left({u}_{0t}-{u}_{0b}\right)+\left(\frac{3}{4{h}_{2}}{f}_{2}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{5}\right)\left({h}_{1}{\theta }_{xt}+{h}_{3}{\theta }_{xb}\right)+\left(\frac{3}{4{h}_{2}}{f}_{3}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{6}\right)\frac{\partial {W}_{0}}{\partial x}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({I}_{0}^{t}{\ddot{u}}_{0t}+{\bar{I}}_{1}^{t}{\ddot{\theta }}_{xt}-{I}_{3}^{t}{C}_{1}\frac{\partial {\ddot{W}}_{0}}{\partial x}\right),$$
(B1)
$$\delta {u}_{0b}\to {A}_{11}\left(\frac{{\partial }^{2}{u}_{0b}}{\partial {x}^{2}}\right)+{A}_{66}\left(\frac{{\partial }^{2}{u}_{0b}}{\partial {y}^{2}}\right)+\left[{A}_{12}+{A}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0b}}{\partial x\partial y}\right)+\left[{B}_{11}-{C}_{1}{E}_{11}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial {x}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial {y}^{2}}\right)+\left[{B}_{12}+{B}_{66}-{C}_{1}{E}_{12}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial x\partial y}\right)-\left[{C}_{1}{E}_{12}+2{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial x\partial {y}^{2}}\right)-{C}_{1}{E}_{11}\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{3}}\right)+\left(\frac{3}{4{h}_{2}}{f}_{1}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{4}\right)\left({u}_{0t}-{u}_{0b}\right)-\left(\frac{3}{4{h}_{2}}{f}_{2}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{5}\right)\left({h}_{1}{\theta }_{xt}+{h}_{3}{\theta }_{xb}\right)-\left(\frac{3}{4{h}_{2}}{f}_{3}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{6}\right)\frac{\partial {W}_{0}}{\partial x}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({I}_{0}^{b}{\ddot{u}}_{0b}+{\bar{I}}_{1}^{b}{\ddot{\theta }}_{xb}-{I}_{3}^{b}{C}_{1}\frac{\partial {\ddot{W}}_{0}}{\partial x}\right),$$
(B2)
$$\delta {v}_{0t}\to {A}_{22}\left(\frac{{\partial }^{2}{v}_{0t}}{\partial {y}^{2}}\right)+{A}_{66}\left(\frac{{\partial }^{2}{v}_{0t}}{\partial {x}^{2}}\right)+\left[{A}_{21}+{A}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0t}}{\partial x\partial y}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial {x}^{2}}\right)+\left[{B}_{22}-{C}_{1}{E}_{22}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial {y}^{2}}\right)+\left[{B}_{21}+{B}_{66}-{C}_{1}{E}_{21}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial x\partial y}\right)-\left[{C}_{1}{E}_{21}+2{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{2}\partial y}\right)-{C}_{1}{E}_{22}\left(\frac{{\partial }^{3}{W}_{0}}{\partial {y}^{3}}\right)-\left(\frac{3}{4{h}_{2}}{f}_{1}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{4}\right)\left({v}_{0t}-{v}_{0b}\right)+\left(\frac{3}{4{h}_{2}}{f}_{2}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{5}\right)\left({h}_{1}{\theta }_{yt}+{h}_{3}{\theta }_{yb}\right)+\left(\frac{3}{4{h}_{2}}{f}_{3}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{6}\right)\frac{\partial {W}_{0}}{\partial y}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({I}_{0}^{t}{\ddot{v}}_{0t}+{\bar{I}}_{1}^{t}{\ddot{\theta }}_{yt}-{I}_{3}^{t}{C}_{1}\frac{\partial {\ddot{W}}_{0}}{\partial y}\right),$$
(B3)
$${v}_{0b}\to {A}_{22}\left(\frac{{\partial }^{2}{v}_{0b}}{\partial {y}^{2}}\right)+{A}_{66}\left(\frac{{\partial }^{2}{v}_{0b}}{\partial {x}^{2}}\right)+\left[{A}_{21}+{A}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0b}}{\partial x\partial y}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial {x}^{2}}\right)+\left[{B}_{22}-{C}_{1}{E}_{22}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial {y}^{2}}\right)+\left[{B}_{21}+{B}_{66}-{C}_{1}{E}_{21}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial x\partial y}\right)-\left[{C}_{1}{E}_{21}+2{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{2}\partial y}\right)-{C}_{1}{E}_{22}\left(\frac{{\partial }^{3}{W}_{0}}{\partial {y}^{3}}\right)+\left(\frac{3}{4{h}_{2}}{f}_{1}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{4}\right)\left({v}_{0t}-{v}_{0b}\right)-\left(\frac{3}{4{h}_{2}}{f}_{2}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{5}\right)\left({h}_{1}{\theta }_{yt}+{h}_{3}{\theta }_{yb}\right)-\left(\frac{3}{4{h}_{2}}{f}_{3}-\frac{9}{4{h}_{2}}{{C}_{1}f}_{6}\right)\frac{\partial {W}_{0}}{\partial y}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({I}_{0}^{t}{\ddot{v}}_{0t}+{\bar{I}}_{1}^{t}{\ddot{\theta }}_{yt}-{I}_{3}^{t}{C}_{1}\frac{\partial {\ddot{W}}_{0}}{\partial y}\right),$$
(B4)
$$\delta {W}_{0}\to 2\left[{A}_{44}-6{C}_{1}{H}_{44}+9{C}_{1}^{2}{T}_{44}\right]\left(\frac{{\partial }^{2}{W}_{0}}{\partial {x}^{2}}+\frac{{\partial }^{2}{W}_{0}}{\partial {y}^{2}}\right)+\left[{A}_{44}-6{C}_{1}{H}_{44}+9{C}_{1}^{2}{T}_{44}\right]\left(\frac{\partial {\theta }_{xt}}{\partial x}+\frac{\partial {\theta }_{yt}}{\partial y}+\frac{\partial {\theta }_{xb}}{\partial x}+\frac{\partial {\theta }_{yb}}{\partial y}\right)+{C}_{1}{E}_{11}\left(\frac{{\partial }^{3}{u}_{0t}}{\partial {x}^{3}}+\frac{{\partial }^{3}{u}_{0b}}{\partial {x}^{3}}\right)+{C}_{1}{E}_{22}\left(\frac{{\partial }^{3}{v}_{0t}}{\partial {y}^{3}}+\frac{{\partial }^{3}{v}_{0b}}{\partial {y}^{3}}\right)+\left[{C}_{1}{E}_{12}+2{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{3}{u}_{0t}}{\partial x\partial {y}^{2}}+\frac{{\partial }^{3}{u}_{0b}}{\partial x\partial {y}^{2}}+\frac{{\partial }^{3}{v}_{0t}}{\partial {x}^{2}\partial y}+\frac{{\partial }^{3}{v}_{0b}}{\partial {x}^{2}\partial y}\right)+\left[{C}_{1}{T}_{11}-{C}_{1}^{2}{D}_{11}\right]\left(\frac{{\partial }^{3}{\theta }_{xt}}{\partial {x}^{3}}+\frac{{\partial }^{3}{\theta }_{xt}}{\partial {x}^{3}}\right)+\left[{C}_{1}{T}_{22}-{C}_{1}^{2}{D}_{22}\right]\left(\frac{{\partial }^{3}{\theta }_{yt}}{\partial {y}^{3}}+\frac{{\partial }^{3}{\theta }_{yb}}{\partial {y}^{3}}\right)+\left[{C}_{1}{T}_{12}-{C}_{1}^{2}{D}_{12}+2\left({C}_{1}{T}_{66}-{C}_{1}^{2}{D}_{66}\right)\right]\left(\frac{{\partial }^{3}{\theta }_{xt}}{\partial x\partial {y}^{2}}+\frac{{\partial }^{3}{\theta }_{yt}}{\partial {x}^{2}\partial y}+\frac{{\partial }^{3}{\theta }_{xb}}{\partial x\partial {y}^{2}}+\frac{{\partial }^{3}{\theta }_{yb}}{\partial {x}^{2}\partial y}\right)-2{C}_{1}^{2}{D}_{11}\left(\frac{{\partial }^{4}{W}_{0}}{\partial {x}^{4}}\right)-2{C}_{1}^{2}{D}_{22}\left(\frac{{\partial }^{4}{W}_{0}}{\partial {y}^{4}}\right)-2\left[2{C}_{1}^{2}{D}_{12}+4{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{4}{W}_{0}}{\partial {x}^{2}\partial {y}^{2}}\right)-\left(r{f}_{1}-r{C}_{1}{f}_{4}\right)\left(\frac{\partial {u}_{0t}}{\partial x}-\frac{\partial {u}_{0b}}{\partial x}\right)+\left(r{f}_{2}-r{C}_{1}{f}_{5}\right)\left({h}_{1}\frac{\partial {\theta }_{xt}}{\partial x}+{h}_{2}\frac{\partial {\theta }_{xb}}{\partial x}\right)+\left(r{f}_{3}-r{C}_{1}{f}_{6}\right)\frac{{\partial }^{2}{W}_{0}}{\partial {x}^{2}}-\left(r{f}_{1}-r{C}_{1}{f}_{4}\right)\left(\frac{\partial {v}_{0t}}{\partial y}-\frac{\partial {v}_{0b}}{\partial x}\right)+\left(r{f}_{2}-r{C}_{1}{f}_{5}\right)\left({h}_{1}\frac{\partial {\theta }_{yt}}{\partial y}+{h}_{2}\frac{\partial {\theta }_{yb}}{\partial y}\right)+\left(r{f}_{3}-r{C}_{1}{f}_{6}\right)\frac{{\partial }^{2}{W}_{0}}{\partial {y}^{2}}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left\{q+\left({I}_{0}^{t}+{I}_{0}^{c}+{I}_{0}^{b}\right){\ddot{W}}_{0}+{I}_{3}^{t}{C}_{1}\left(\frac{\partial {\ddot{u}}_{0t}}{\partial x}+\frac{\partial {\ddot{v}}_{0t}}{\partial y}\right)+{I}_{3}^{b}{C}_{1}\left(\frac{\partial {\ddot{u}}_{0b}}{\partial x}+\frac{\partial {\ddot{v}}_{0b}}{\partial y}\right)-\left({I}_{6}^{t}+{I}_{3}^{b}\right){C}_{1}^{2}\left(\frac{{\partial }^{2}{\ddot{W}}_{0}}{\partial {x}^{2}}+\frac{{\partial }^{2}{\ddot{W}}_{0}}{\partial {y}^{2}}\right)+{\bar{I}}_{4}^{t}{C}_{1}\left(\frac{\partial {\ddot{\theta }}_{xt}}{\partial x}+\frac{\partial {\ddot{\theta }}_{yt}}{\partial y}\right)+{\bar{I}}_{4}^{b}{C}_{1}\left(\frac{\partial {\ddot{\theta }}_{xb}}{\partial x}+\frac{\partial {\ddot{\theta }}_{yb}}{\partial y}\right)\right\},$$
(B5)
$$\delta {\theta }_{xt}\to \left[{B}_{11}-{C}_{1}{E}_{11}\right]\left(\frac{{\partial }^{2}{u}_{0t}}{\partial {x}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0t}}{\partial {y}^{2}}\right)+\left[{B}_{12}-{C}_{1}{E}_{12}+{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0t}}{\partial x\partial y}\right)+\left[{H}_{11}-2{C}_{1}{T}_{11}+{C}_{1}^{2}{D}_{11}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial {x}^{2}}\right)+\left[{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial {y}^{2}}\right)+\left[{H}_{12}-2{C}_{1}{T}_{12}\right.\left.+{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{12}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial x\partial y}\right)+\left[6{C}_{1}{H}_{44}-{A}_{44}-9{C}_{1}^{2}{T}_{44}\right]\left({\theta }_{xt}+\frac{\partial {W}_{0}}{\partial x}\right)+\left({C}_{1}^{2}{D}_{11}-{C}_{1}{T}_{11}\right)\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{3}}\right)+\left[{C}_{1}^{2}{D}_{12}-{C}_{1}{T}_{12}+2\left({C}_{1}^{2}{D}_{66}-{C}_{1}{T}_{66}\right)\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial x\partial {y}^{2}}\right)+\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{1}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{4}\right)\left({u}_{0t}-{u}_{0b}\right)-\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{2}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{5}\right)\left({h}_{1}{\theta }_{xt}+{h}_{3}{\theta }_{xb}\right)-\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{3}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{6}\right)\frac{\partial {W}_{0}}{\partial x}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({\bar{I}}_{1}^{t}{\ddot{u}}_{0t}+{\bar{I}}_{2}^{t}{\ddot{\theta }}_{xt}-{C}_{1}{\bar{I}}_{4}^{t}\frac{\partial {\ddot{W}}_{0}}{\partial x}\right),$$
(B6)
$$\delta {\theta }_{xb}\to \left[{B}_{11}-{C}_{1}{E}_{11}\right]\left(\frac{{\partial }^{2}{u}_{0b}}{\partial {x}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0b}}{\partial {y}^{2}}\right)+\left[{B}_{12}-{C}_{1}{E}_{12}+{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0b}}{\partial x\partial y}\right)+\left[{H}_{11}-2{C}_{1}{T}_{11}+{C}_{1}^{2}{D}_{11}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial {x}^{2}}\right)+\left[{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial {y}^{2}}\right)+\left[{H}_{12}-2{C}_{1}{T}_{12}\right.\left.+{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{12}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial x\partial y}\right)+\left[6{C}_{1}{H}_{44}-{A}_{44}-9{C}_{1}^{2}{T}_{44}\right]\left({\theta }_{xb}+\frac{\partial {W}_{0}}{\partial x}\right)+\left({C}_{1}^{2}{D}_{11}-{C}_{1}{T}_{11}\right)\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{3}}\right)+\left[{C}_{1}^{2}{D}_{12}-{C}_{1}{T}_{12}+2\left({C}_{1}^{2}{D}_{66}-{C}_{1}{T}_{66}\right)\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial x\partial {y}^{2}}\right)+\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{1}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{4}\right)\left({u}_{0t}-{u}_{0b}\right)-\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{2}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{5}\right)\left({h}_{1}{\theta }_{xt}+{h}_{3}{\theta }_{xb}\right)-\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{3}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{6}\right)\frac{\partial {W}_{0}}{\partial x}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({\bar{I}}_{1}^{b}{\ddot{u}}_{0b}+{\bar{I}}_{2}^{b}{\ddot{\theta }}_{xb}-{C}_{1}{\bar{I}}_{4}^{b}\frac{\partial {\ddot{W}}_{0}}{\partial x}\right),$$
(B7)
$$\delta {\theta }_{yt}\to \left[{B}_{22}-{C}_{1}{E}_{22}\right]\left(\frac{{\partial }^{2}{v}_{0t}}{\partial {y}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0t}}{\partial {x}^{2}}\right)+\left[{B}_{12}-{C}_{1}{E}_{12}+{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0t}}{\partial x\partial y}\right)+\left[{H}_{22}-2{C}_{1}{T}_{22}+{C}_{1}^{2}{D}_{22}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial {y}^{2}}\right)+\left[{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yt}}{\partial {x}^{2}}\right)+\left[{H}_{12}-2{C}_{1}{T}_{12}\right.\left.+{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{12}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xt}}{\partial x\partial y}\right)+\left[6{C}_{1}{H}_{44}-{A}_{44}-9{C}_{1}^{2}{T}_{44}\right]\left({\theta }_{yt}+\frac{\partial {W}_{0}}{\partial y}\right)+\left({C}_{1}^{2}{D}_{22}-{C}_{1}{T}_{22}\right)\left(\frac{{\partial }^{3}{W}_{0}}{\partial {y}^{3}}\right)+\left[{C}_{1}^{2}{D}_{12}-{C}_{1}{T}_{12}+2\left({C}_{1}^{2}{D}_{66}-{C}_{1}{T}_{66}\right)\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{2}\partial y}\right)+\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{1}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{4}\right)\left({v}_{0t}-{v}_{0b}\right)-\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{2}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{5}\right)\left({h}_{1}{\theta }_{yt}+{h}_{3}{\theta }_{yb}\right)-\left(\frac{{h}_{1}}{2{h}_{2}}{f}_{3}-\frac{{3{C}_{1}h}_{1}}{2{h}_{2}}{f}_{6}\right)\frac{\partial {W}_{0}}{\partial y}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({\bar{I}}_{1}^{t}{\ddot{v}}_{0t}+{\bar{I}}_{2}^{t}{\ddot{\theta }}_{yt}-{C}_{1}{\bar{I}}_{4}^{t}\frac{\partial {\ddot{W}}_{0}}{\partial y}\right),$$
(B8)
$$\delta {\theta }_{yb}\to \left[{B}_{22}-{C}_{1}{E}_{22}\right]\left(\frac{{\partial }^{2}{v}_{0b}}{\partial {y}^{2}}\right)+\left[{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{v}_{0b}}{\partial {x}^{2}}\right)+\left[{B}_{12}-{C}_{1}{E}_{12}+{B}_{66}-{C}_{1}{E}_{66}\right]\left(\frac{{\partial }^{2}{u}_{0b}}{\partial x\partial y}\right)+\left[{H}_{22}-2{C}_{1}{T}_{22}+{C}_{1}^{2}{D}_{22}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial {y}^{2}}\right)+\left[{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{yb}}{\partial {x}^{2}}\right)+\left[{H}_{12}-2{C}_{1}{T}_{12}\right.\left.+{H}_{66}-2{C}_{1}{T}_{66}+{C}_{1}^{2}{D}_{12}+{C}_{1}^{2}{D}_{66}\right]\left(\frac{{\partial }^{2}{\theta }_{xb}}{\partial x\partial y}\right)+\left[6{C}_{1}{H}_{44}-{A}_{44}-9{C}_{1}^{2}{T}_{44}\right]\left({\theta }_{yb}+\frac{\partial {W}_{0}}{\partial y}\right)+\left({C}_{1}^{2}{D}_{22}-{C}_{1}{T}_{22}\right)\left(\frac{{\partial }^{3}{W}_{0}}{\partial {y}^{3}}\right)+\left[{C}_{1}^{2}{D}_{12}-{C}_{1}{T}_{12}+2\left({C}_{1}^{2}{D}_{66}-{C}_{1}{T}_{66}\right)\right]\left(\frac{{\partial }^{3}{W}_{0}}{\partial {x}^{2}\partial y}\right)+\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{1}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{4}\right)\left({v}_{0t}-{v}_{0b}\right)-\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{2}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{5}\right)\left({h}_{1}{\theta }_{yt}+{h}_{3}{\theta }_{yb}\right)-\left(\frac{{h}_{3}}{2{h}_{2}}{f}_{3}-\frac{{3{C}_{1}h}_{3}}{2{h}_{2}}{f}_{6}\right)\frac{\partial {W}_{0}}{\partial y}=\left[1-{\left({e}_{0}a\right)}^{2}{\nabla }^{2}\right]\left({\bar{I}}_{1}^{b}{\ddot{v}}_{0b}+{\bar{I}}_{2}^{b}{\ddot{\theta }}_{yb}-{C}_{1}{\bar{I}}_{4}^{b}\frac{\partial {\ddot{W}}_{0}}{\partial y}\right),$$
(B9)

where

$$\left( {A_{11} ,B_{11} ,H_{11} ,E_{11} ,T_{11} ,D_{11} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} Q_{11} \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz,$$
$$\left( {A_{12} ,B_{12} ,H_{12} ,E_{12} ,T_{12} ,D_{12} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} Q_{12} \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz,$$
$$\left( {A_{22} ,B_{22} ,H_{22} ,E_{22} ,T_{22} ,D_{22} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} Q_{22} \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz,$$
$$\left( {A_{44} ,B_{44} ,H_{44} ,E_{44} ,T_{44} ,D_{44} } \right) = \mathop \int \limits_{{ - h_{1} /2}}^{{h_{1} /2}} Q_{44} \left( {1,z,z^{2} ,z^{3} ,z^{4} ,z^{6} } \right)dz,$$
$$r = \left( {\frac{{h_{1} }}{{8h_{2} }} + \frac{{h_{3} }}{{8h_{2} }} - \frac{3}{4}} \right),f_{1} = \frac{3}{{4h_{2} }}G^{\left( c \right)} \overline{a} - \frac{{9C_{1} }}{{4h_{2} }}G^{\left( c \right)} \overline{b},f_{2} = \frac{1}{{2h_{2} }}G^{\left( c \right)} \overline{a} - \frac{{3C_{1} }}{{2h_{2} }}G^{\left( c \right)} \overline{b}$$
$$f_{3} = G^{\left( c \right)} \overline{a}r - 3C_{1} G^{\left( c \right)} \overline{b}r,f_{4} = \frac{3}{{4h_{2} }}G^{\left( c \right)} \overline{b} - \frac{{9C_{1} }}{{4h_{2} }}G^{\left( c \right)} \overline{d},f_{5} = \frac{1}{{2h_{2} }}G^{\left( c \right)} \overline{b} - \frac{{3C_{1} }}{{2h_{2} }}G^{\left( c \right)} \overline{d}$$
$$f_{6} = G^{\left( c \right)} \overline{b}r - 3C_{1} G^{\left( c \right)} \overline{d}r,\left( {\overline{a},\overline{b},\overline{d}} \right) = \mathop \int \limits_{{ - h_{2} /2}}^{{h_{2} /2}} \left( {1,z^{2} ,z^{4} } \right)dz$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Saffari, P.R., Ismail, S.O., Thongchom, C. et al. Effect of Magnetic Field on Vibration of Electrorheological Fluid Nanoplates with FG-CNTRC Layers. J. Vib. Eng. Technol. 12, 3335–3354 (2024). https://doi.org/10.1007/s42417-023-01048-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s42417-023-01048-7

Keywords

Search

Navigation