Introduction

Functionally graded structures (FGS) have been in the interest of many researchers for the past thirty years because they are tailored to satisfy certain static, dynamic, mechanical,or thermal requirements by spatially varying the constitutive materials contents. Initially, FGS were designed to allow material grading through the thickness of plates, but as new manufacturing technologies are evolving, such as additive manufacturing and 3D printing of metals and polymers, more interests are given to multi-directional functionally graded structures [1,2,3,4]. There are a lot of papers in literature that discuss FG structures, therefore, this section focuses only on the literature review of the vibration analysis of in-plane functionally graded plates.

Starting with the linear vibration analysis, Liu et al. [5] studied the free vibration of in-plane FG rectangular plates using a levy-type solution. The material grading was considered to be uniaxial with a monomial representation. Kermani et al. [6] studied the free vibration of circular and annular plates with material grading through the thickness and along the radial direction using a differential quadrature method. The exponential representation of the constitutive properties was adopted and the effect of the exponent coefficient on the natural frequencies was investigated. Sobhani et al. [7] proposed a six-parameters power law for the material grading of curved panels. The model allows grading in the axial direction and through thickness. The generalized differential quadrature method was adopted to study the effect of the model parameters selection of the natural frequencies and mode shapes. Tahouneh and Naei [8] used the same model and solution method to analyze the vibration characteristics of elastically supported thick rectangular in-plane FG plates. Lal and Saini [9] considered the linear vibration of uni-axially graded rectangular plates subjected to in-plane forces. Exponential material grading law and the differential quadrature method were used for the analysis. Amirpour et al. [10] studied the free vibration of in-plane FG polymeric rectangular plates with monomial variation of the mechanical properties along one direction. Higher order shear deformation plate theory (HSDT) and the finite element method were utilized for the analysis. A comparison between the numerical results and the experiments showed good agreement. Chu et al. [11] used a meshfree method based on the Hermite radial basis collocation to investigate the effect of material grading model (exponential and monomial) on the natural frequencies of rectangular and circular plates. Singh and Kumari [12] proposed a three-dimensional elasticity solution for the free vibration analysis of plates with linear material gradation along one direction. Huang et al. [13] presented a Chebyshev spectral method to analyze the vibration of plates with both in-plane thickness and material grading. Qian and Batra [14] considered the effect of the material grading on the natural frequncies of rectangular plates. The grading was allowed through the thickness and along the axial direction using a monomial representation where the monomial exponent is taken as a design parameter. The Petrov–Galerkin method was used to solve the governing differential equation. Alshabatat et al. [15] investigated the vibration of plates with in-plane bi-directional material grading following a sinusoidal representation. Loja and Barbosa [16] analyzed the linear vibration of plates with different in-plane material grading models using the Rayleigh–Ritz and Bolotin’s method. Recently, the isogeometric approach has been used in literature to the vibration analysis of in-plane FG plates [17,18,19,20,21,22,23].

Moving to the non-linear vibration analysis, Malekzadeh and Beni [24] studied the nonlinear free vibration of bi-directional in-plane FG rectangular plates using the differential quadrature method. Monomial material grading was considered in each direction. The effect of the monomial order on the nonlinear frequency was presented. Kumar et al. [25] investigated the nonlinear forced vibration of axially graded rectangular plates with varying thickness. The Hamilton’s principle was used to derive the system of equations based on assumed displacement functions. Lohar et al. [26] considered the non-linear free vibration of unidirectional in-plane FG rectangular plates resting on elastic foundation. Hussein and Mulani [27] studied the nonlinear aeroelastic vibration of bi-directional FG plates in supersonic flow. Chen et al. [28] analyzed the non-linear forced vibration of bi-directional in-plane FG plates with local geometrical imperfections. The effect of the imperfection nature and location on the nonlinear dynamic behavior was presented.

It can be seen from the literature review that the effect of elastic properties uncertainties on the non-linear free and forced vibrations of pre/post buckled in-plane FG nanocomposite plates has not been investigated in the literature. Also, unlike the work in the literature where the in-plane material grading is assumed and usually one directional or monotonic, this work starts with optimizing the FG plates in the two in-plane directions using polynomial representation of the volume fraction such that the fundamental frequencies of the FG plates match the fundamental frequencies of the homogenous plates using minimum amount of the nano-reinforcement. Magnesium plates reinforced with Silicon Carbide nanoparticles are chosen for this study because of Magnesium’s light weight and high mechanical properties which gives it potentials for aerospace applications [29]. The uncertain nonlinear vibration analysis is, then, performed through a parametric analysis to investigate the effect of the material uncertainty modeling. The effect of the in-plane compression forces in the pre-buckling and post-buckling states is studied which also has not been covered in the literature. A comparison between the non-linear deterministic and non-deterministic behaviors of the FG plates and the behavior of the homogenous plates counterparts is presented.

Mathematical Modeling

Structural Modeling

This paper studies the uncertain vibration of thin plates with in-plane material inhomogeneity. So, the classical plate theory is a suitable choice for the structural modeling which describes the displacement field in terms of the mid-plane displacements (\(u_{o} ,v_{o} {\text{and}} w_{o}\)) as follows [30]:

$$\begin{aligned} u\left( {x,y,z} \right) & = u_{o} \left( {x,y} \right) - z w_{o} ,_{x} \left( {x,y} \right) \\ v\left( {x,y,z} \right) & = v_{o} \left( {x,y} \right) - z w_{o} ,_{y} \left( {x,y} \right) \\ w\left( {x,y,z} \right) & = w_{o} \left( {x,y} \right). \\ \end{aligned}$$
(1)

The corresponding nonlinear von Kármán’s normal strains (\(\varepsilon_{xx} ,{\text{and}} \varepsilon_{yy}\)) and the in-plane shear strain (\(\gamma_{xy}\)) will be as follows:

$$\vec{\varepsilon } = \left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\varepsilon_{yy} } \\ {\gamma_{xy} } \\ \end{array} } \right\} = \left\{ {\begin{array}{*{20}c} {u_{o,x} } \\ {v_{o,y} } \\ {u_{o,y} + v_{o,x} } \\ \end{array} } \right\} - z\left\{ {\begin{array}{*{20}c} {w_{o,xx} } \\ {w_{o,yy} } \\ {2w_{o,xy} } \\ \end{array} } \right\} + \frac{1}{2}\left\{ {\begin{array}{*{20}c} {w_{o} ,_{x}^{2} } \\ {w_{o} ,_{y}^{2} } \\ {2w_{o,x} w_{o,y} } \\ \end{array} } \right\}.$$
(2)

For the in-plane functionally graded plates, the constitutive relations spatially vary in the x and y directions and can be assumed isotropic at the microscale level. So, the mechanical behavior is totally described by the Young’s modulus \(E\left( {x,y} \right)\) and the Poisson’s ratio \(\nu \left( {x,y} \right)\). The constitutive matrix \(\left[ C \right]\) relating the in-plane stress vector \(\vec{\sigma } = \left\{ {\sigma_{xx} ,\sigma_{yy} ,\sigma_{xy} } \right\}\) and the strain vector \(\vec{\varepsilon } = \left\{ {\varepsilon_{xx} ,\varepsilon_{yy} ,\gamma_{xy} } \right\}\) can be written as follows:

$$\left[ C \right] = \frac{{E\left( {x,y} \right)}}{{1 - \nu^{2} \left( {x,y} \right)}}\left[ {\begin{array}{*{20}c} 1 & {\nu \left( {x,y} \right)} & 0 \\ {\nu \left( {x,y} \right)} & 1 & 0 \\ 0 & 0 & {\frac{{1 - \nu \left( {x,y} \right)}}{2}} \\ \end{array} } \right].$$
(3)

The Hashin–Shtrikman upper bound theory gives a very good estimate of the mechanical properties of metals reinforced with nanoparticles. Therefore, the composite bulk (\({\mathcal{K}}\)) and shear (\(G\)) moduli can be estimated in terms of the metal moduli \(\left( { {\mathcal{K}}_{m} , G_{m} { }} \right)\), the reinforcement moduli \(\left( { {\mathcal{K}}_{f} , G_{f} { }} \right)\), and the reinforcement volume fraction \(\left( {{ }v_{f} { }} \right)\) as follows [27]:

$${\mathcal{K}} = {\mathcal{K}}_{f} + \left( {1 - v_{f} } \right)\left[ {\frac{1}{{{\mathcal{K}}_{m} - {\mathcal{K}}_{f} }} + \frac{{3v_{f} }}{{3{\mathcal{K}}_{f} + 4G_{f} }}} \right]^{ - 1}$$
(4)
$$\begin{aligned} G & = G_{f} + \left( {1 - v_{f} } \right)\left[ {\frac{1}{{G_{m} - G_{f} }} + \frac{{6v_{f} \left( {{\mathcal{K}}_{f} + 2G_{f} } \right)}}{{5G_{f} \left( {3{\mathcal{K}}_{f} + 4G_{f} } \right)}}} \right]^{ - 1} \\ E & = \frac{{9{\mathcal{K}}G}}{{3{\mathcal{K}} + G}} , v = \frac{{3{\mathcal{K}} - 2G}}{{6{\mathcal{K}} + 2G}}. \\ \end{aligned}$$
(5)

The reinforcement in-plane volume fraction distribution is represented using polynomial expansions of arbitrary orders (\(p_{x} {\text{and}} p_{y}\)) in the non-dimensional x* and y* directions with coefficient values to be optimized for a given problem as will be discussed later as follows [31,32,33]:

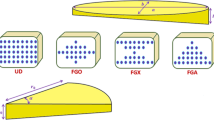
$$\begin{gathered} \nu_{f} \left( {x^{*} ,y^{*} } \right) = v_{fx} \left( {x^{*} } \right)v_{fy} \left( {y^{*} } \right) \hfill \\ v_{fx} \left( {x^{*} } \right) = \mathop \sum \limits_{i = 0}^{{p_{x} }} a_{i} x^{*i} , v_{fy} \left( {y^{*} } \right) = \mathop \sum \limits_{j = 0}^{{p_{y} }} b_{j} y^{*j} . \hfill \\ \end{gathered}$$
(6)

The finite element method (FEM) with four-nodded quadrilateral elements shown in Fig. 1 is used for this study. The mid-plane vertical displacement field over each element is described in terms of the nodes’ out-of- plane degrees of freedom vector \(\left\{ {\vec{q}_{o} } \right\}\) using a third-order shape function vector \(N_{O}\), and the in-plane displacements are described in terms of a bi-linear shape function matrix \(\left[ {N_{I} } \right]\) and the nodes’ in-plane displacement vector \(\left\{ {\vec{q}_{I} } \right\}\) as follows [34]:

$$\left\{ {\begin{array}{*{20}c} {u_{o} \left( {\xi ,\eta } \right)} \\ {v_{o} \left( {\xi ,\eta } \right)} \\ \end{array} } \right\} = \left[ {N_{I} } \right]\left\{ {\vec{q}_{I} } \right\}, w_{o} \left( {\xi ,\eta } \right) = N_{O} \left\{ {\vec{q}_{o} } \right\}.$$
(7)
Fig. 1
figure 1

Quadrilateral four-nodded finite element

Full size image

Using this displacement field description, the following displacement derivatives vectors can be defined:

$$\left\{ {\begin{array}{*{20}c} {u,_{x} } \\ {v,_{y} } \\ {u,_{y} + v,_{x} } \\ \end{array} } \right\} = \left[ {B_{I} } \right]\left\{ {\vec{q}_{I} } \right\},\;\left\{ {\begin{array}{*{20}c} {w,_{xx} } \\ {w,_{yy} } \\ {2w,_{xy} } \\ \end{array} } \right\} = \left[ {B_{o} } \right]\left\{ {\vec{q}_{o} } \right\}$$
(8)
$$\left[ {\mathfrak{S}} \right] = \left[ {\begin{array}{*{20}c} {w,_{x} } & 0 \\ 0 & {w,_{y} } \\ {w,_{y} } & {w,_{x} } \\ \end{array} } \right], \left\{ {\begin{array}{*{20}c} {w,_{x} } \\ {w,_{y} } \\ \end{array} } \right\} = \left[ {B_{{\mathfrak{S}}} } \right]\left\{ {\vec{q}_{o} } \right\}.$$
(9)

The derivation of the elemental matrices is done using the principle of virtual work which can be written in terms of the strain energy (\({\mathcal{U}}\)) and the external work (\({\mathcal{W}}\)) for an element with a volume (V) and a boundary surface (\(S\)) as follows:

$$\delta \left( {{\mathcal{U}} - {\mathcal{W}}} \right) = 0$$
(10)
$$\delta {\mathcal{U}} = \mathop \int \limits_{V}^{{}} \delta \vec{\varepsilon } \left[ C \right]\vec{\varepsilon } dV$$
$$\begin{aligned} \delta {\mathcal{U}} & = \mathop \int \limits_{V}^{{}} \delta \vec{\varepsilon } \left[ C \right]\vec{\varepsilon } {\text{d}}V \\ \delta {\mathcal{W}} & = - \mathop \int \limits_{V}^{{}} \rho \left( {\delta u\ddot{u} + \delta v\ddot{v} + \delta w\ddot{w}} \right) {\text{d}}V + \mathop \int \limits_{S}^{{}} \left( {\delta u f_{x} + \delta v f_{y} + \delta w p} \right) {\text{d}}S, \\ \end{aligned}$$
(11)

where \(f_{x} {\text{and}} f_{y}\) are the in-plane forces per unit length, \(p\) is the external pressure, while \(\ddot{u},\ddot{v}, {\text{and}} \ddot{w}\) are the acceleration components and \(\rho\) is the material density. Therefore, the elemental stiffness matrices can be obtained using Eqs. (7–9) and can be written as follows:

$$\begin{gathered} \left[ {K_{o} } \right]_{e} = \frac{{h^{3} }}{12}\iint {\left[ {B_{o} } \right]^{{\text{T}}} \left[ C \right]\left[ {B_{o} } \right]{\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left[ {K_{I} } \right]_{e} = h\iint {\left[ {B_{I} } \right]^{{\text{T}}} \left[ C \right]\left[ {B_{I} } \right]{\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left[ {K_{{{\text{NL}}}}^{\left( 1 \right)} } \right]_{e} = \frac{h}{2}\iint {\left[ {B_{{\mathfrak{S}}} } \right]^{{\text{T}}} \left[ {\mathfrak{S}} \right]^{{\text{T}}} \left[ C \right]\left[ {B_{I} } \right]{\mathbb{J}}{\text{ d}}\xi {\text{d}}\eta } \hfill \\ \left[ {K_{{{\text{NL}}}}^{\left( 2 \right)} } \right]_{e} = \frac{h}{2}\iint {\left[ {B_{{\mathfrak{S}}} } \right]^{{\text{T}}} \left[ {\mathbb{F}} \right] \left[ {B_{{\mathfrak{S}}} } \right]{\mathbb{J}}{\text{ d}}\xi {\text{d}}\eta } \hfill \\ \left[ {K_{{{\text{NL}}}}^{\left( 3 \right)} } \right]_{e} = \frac{h}{2}\iint {\left[ {B_{{\mathfrak{S}}} } \right]^{{\text{T}}} \left[ {\mathfrak{S}} \right]^{{\text{T}}} \left[ C \right] \left[ {\mathfrak{S}} \right]\left[ {B_{{\mathfrak{S}}} } \right]{\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left[ {M_{o} } \right]_{e} = \rho h\iint {N_{O}^{T} N_{O} {\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left[ {M_{I} } \right]_{e} = \rho h\iint {\left[ {N_{I} } \right]^{T} \left[ {N_{I} } \right]{\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left\{ {F_{o} } \right\}_{e} = \iint {\left[ {N_{I} } \right]^{T} p\left( {\xi ,\eta } \right){\mathbb{J}}{\text{d}}\xi {\text{d}}\eta } \hfill \\ \left\{ {F_{I} } \right\}_{e} = \iint {\left[ {N_{I} } \right]^{{\text{T}}} \left\{ {\begin{array}{*{20}c} {f_{x} } \\ {f_{y} } \\ \end{array} } \right\}{\mathbb{J}}{\text{d}}\xi {\text{d}}\eta }. \hfill \\ \end{gathered}$$
(12)

where \(h\) is the plate’s thickness, \(\left[ {K_{o} } \right]\) is the out-of-plane linear stiffness matrix, \(\left[ {K_{I} } \right]\) is the in-plane linear stiffness matrix, \(\left[ {K_{{{\text{NL}}}}^{\left( 1 \right)} } \right]\) and \(\left[ {K_{{{\text{NL}}}}^{\left( 2 \right)} } \right]\) are second-order nonlinear stiffness matrices, \(\left[ {K_{NL}^{\left( 3 \right)} } \right]\) is the third-order nonlinear stiffness matrix, \(\left[ {M_{o} } \right]\) is the out-of-plane mass matrix, \(\left[ {K_{I} } \right]\) is the in-plane mass matrix, \(\left\{ {\vec{F}_{o} } \right\}\) is the out-of-plane force vector, \(\left\{ {\vec{F}_{I} } \right\}\) is the in-plane force vector, \({\mathbb{J}}\) is the integration Jacobian. The 5-points Gauss quadrature rule is used to estimate these elemental matrices. The global system of equations will be as follows:

$$\left[ {M_{o} } \right]\left\{ {\ddot{\vec{q}}_{o} } \right\} + \left( {\left[ {K_{o} } \right] + \left[ {K_{NL}^{\left( 2 \right)} } \right] + \left[ {K_{NL}^{\left( 3 \right)} } \right]} \right)\left\{ {\vec{q}_{o} } \right\} + \left[ {K_{NL}^{\left( 1 \right)} } \right]\left\{ {\vec{q}_{I} } \right\} = \left\{ {\vec{F}_{o} } \right\}$$
(13)
$$\left[ {M_{I} } \right]\left\{ {\ddot{\vec{q}}_{I} } \right\} + \left[ {K_{I} } \right]\left\{ {\vec{q}_{I} } \right\} + \left[ {K_{NL}^{\left( 1 \right)} } \right]^{T} \left\{ {\vec{q}_{o} } \right\} = \left\{ {\vec{F}_{I} } \right\}.$$
(14)

The in-plane frequencies are much higher than the out-of-plane frequencies, so the in-plane inertia can be neglected, and the desired decoupled out-of-plane equations will be as follows:

$$\left[ {M_{o} } \right]\left\{ {\ddot{\vec{q}}_{o} } \right\} + \left( {\left[ {K_{o} } \right] + \left[ {K_{NL}^{\left( 2 \right)} } \right] + \left[ {K_{NL}^{\left( 3 \right)} } \right] - \left[ {K_{NL}^{\left( 1 \right)} } \right]\left[ {K_{I} } \right]^{ - 1} \left[ {K_{NL}^{\left( 1 \right)} } \right]^{T} } \right)\left\{ {\vec{q}_{o} } \right\} = \left\{ {\vec{F}_{o} } \right\} - \left[ {K_{NL}^{\left( 1 \right)} } \right]\left[ {K_{I} } \right]^{ - 1} \left\{ {\vec{F}_{I} } \right\}$$
(15)

The boundary conditions imposed on the edges of the plates are set to restrain all translational displacement, and for the clamped plates, an additional constraint is imposed on the rotational degrees of freedom perpendicular to the edges. The frequency response analysis is done using modal transformation with the orthogonal mode shapes matrix \(\left[\Phi \right]\) as follows:

$$\left\{{\overrightarrow{q}}_{o}\right\}=\left[\Phi \right]\left\{\overrightarrow{\mathbb{q}}\right\}$$
(16)

For a harmonically excited response that is dominated by a single mode, the nonlinear dynamic equation can be written in terms of the linear natural frequency (\({\omega }_{o}\)), linear stiffness (\({k}_{1}\)), nonlinear cubic stiffness (\({k}_{3}\)), structural damping coefficient (\(\zeta\)), the generalized force (\({\mathbb{f}}_{i}\)), and the excitation frequency (\(\omega\)) as follows:

$${\ddot{\mathbb{q}}}_{i}+2\zeta {\omega }_{o}{\dot{\mathbb{q}}}_{i}+{k}_{1}{\mathbb{q}}_{i}+{k}_{3}{\mathbb{q}}_{i}^{3}={\mathbb{f}}_{i} \mathrm{cos}(\omega t)$$
(17)

This single mode approximation is suitable for the stochastic analysis which requires high number of runs, so a computationally low-cost model is necessary. The harmonic balance method can be used to get an approximate relation between the response amplitude, the applied force amplitude and the excitation frequency as follows [35]:

$${\left(\frac{3}{4}{k}_{3}{\mathbb{q}}_{i}^{3}+({k}_{1}-{\omega }^{2}){\mathbb{q}}_{i}\right)}^{2}+{\left(2\zeta {\omega }_{o}\omega \right)}^{2}{\mathbb{q}}_{i}^{2}={\mathbb{f}}_{i}^{2}$$
(18)

It should be noted that this first order harmonic balance method is valid only in the pre-buckled state, while in the post-buckled state, the fourth-order time marching Runge–Kutta method is used.

Polynomial Chaos Expansion (PCE) for Uncertainty Analysis

Surrogate modeling of a physical systems is a practical approach to estimate its outputs when the system is computationally expensive and large number of runs is required to satisfy certain criteria like in the case of a system optimization or an uncertainty quantification and propagation analysis. The non-intrusive polynomial chaos expansion (PCE) method is used in this paper to estimate the uncertainty in the vibrational parameters due to random inputs. For a single-input single-output system, the PCE approximates an uncertain output (\(Y\)) as a series of orthogonal polynomials of the random input (\(X\)). The orthogonality is with respect to the probability density function of the random input. For a Gaussian random input, Hermite polynomials \({H}_{n}\) are used which can be written as follows [36]:

$$\begin{gathered} H_{o} \left( {\mathbb{Z}} \right) = 1, \hfill \\ H_{1} \left( {\mathbb{Z}} \right) = {\mathbb{Z}} \hfill \\ H_{n} \left( {\mathbb{Z}} \right) = {\mathbb{Z}} H_{n - 1} \left( {\mathbb{Z}} \right) - \left( {n - 1} \right)H_{n - 2} \left( {\mathbb{Z}} \right) \hfill \\ \end{gathered}$$
(19)

\({\mathbb{Z}}\) is a zero-mean unit-variance normal random variable which relates to the original random input in terms of the random input mean (\({\mu }_{X}\)) and standard deviation (\({\mathcal{S}}_{X}\)) as follows:

$${\mathbb{Z}} = \frac{{X - \mu_{X} }}{{{\mathcal{S}}_{X} }}$$
(20)

Therefore, the output and its probabilistic parameters can be written as follows:

$$Y = \mathop \sum \limits_{n = 0}^{p} c_{n} H_{n} \left( {\mathbb{Z}} \right), \mu_{Y} = c_{o} , {\mathcal{S}}_{Y}^{2} = \mathop \sum \limits_{n = 0}^{p} \frac{{c_{n}^{2} }}{n!}.$$
(21)

For a physical system with (d) uncorrelated random inputs (multi-dimensional), an output is represented by the tensor product of the expansions in each dimension which yields an expansion with \(\left( {p + d} \right)!/p!d!\) terms. The coefficients of the expansion are determined by generating samples from the random space and evaluate the exact output for these samples, then solve the system of algebraic equations to estimate the coefficients. In this paper Latin-Hyper Cube sampling (LHS) technique is used to generate the samples.

Karhunen–Loève Expansion (KLE) for a Random Field Representation

The material uncertainty of the in-plane functionally graded plates is modeled as a random field using the Karhunen–Loève expansion. Therefore, the elastic modulus will be modeled as a non-stationary Gaussian random field as follows [37]:

$$E\left(x,y\right)={\mu }_{E}(x,y)+{\sigma }_{E}(x,y)\sum_{i=1}^{N}{\mathbb{Z}}_{i}\sqrt{{\lambda }_{i}} {\psi }_{i}(x,y)$$
(22)

\({\mathbb{Z}}_{i}\)’s are uncorrelated Gaussian random variables with zero mean and unit variance. The functions \({\psi }_{i}(x,y)\) and the coefficients \({\lambda }_{i}\) are the eigen-functions and eigen-values of the covariance function \({\mathbb{K}}({x}_{i}-{x}_{j},{y}_{i}-{y}_{j})\) which are obtained by solving the Fredholm’s integral eigen-value:

$$\int {\mathbb{K}}(x-\fancyscript{x},y-\fancyscript{y}) \psi (\fancyscript{x},\fancyscript{y}) \mathrm{d}\fancyscript{x} \mathrm{d}\fancyscript{y}=\lambda \psi (x,y)$$
(23)

This work assumes an exponential correlation function with a correlation length (\({l}_{\mathrm{c}}\)) which can be written as follows:

$${\mathbb{K}}\left({x}_{i}-{x}_{j},{y}_{i}-{y}_{j}\right)={e}^{- \frac{\left|{x}_{i}-{x}_{j}\right|+\left|{y}_{i}-{y}_{j}\right|}{{l}_{c}}}$$
(24)

for this correlation function, the eigen-functions and vectors are simply the product of the eigen-function and vectors of a one-dimensional random field:

$$\psi \left(x,y\right)={\psi }_{x}\left(x\right){\psi }_{y}\left(y\right), \mathrm{and} \lambda ={\lambda }_{x}{\lambda }_{y}$$
(25)

The number of terms used in the Karhunen–Loève expansion depends on the correlation length. Less correlation length requires more terms for accurate representation of the random field. The convergence of the expansion is checked by observing the eigen values. In this paper, the convergence criterion was set to \({\lambda }_{N+1}<0.001{\lambda }_{1}\).

Numerical Results

Code Verification

The nonlinear vibration code is verified by comparing the results with the published results for homogenous plates. Two cases are considered; The first case is a simply supported Aluminum square plate with a side length of 30 cm and a thickness of 1 mm. The Aluminum elastic modulus is 70 GPa, Poisson’s ratio is 0.3, and its density is 2778 kg/m3. The structural damping coefficient is 0.065 and the plate is subjected to a central concentrated harmonic force with an amplitude of 1.74 N. The second plate is a steel square plate with a side length of 50 cm and thickness of 2.08 mm. The steel elastic modulus is 210 GPa, Poisson’s ratio of 0.3, and its density is 7800 kg/m3. The plate is subjected to a uniform harmonic pressure with an amplitude of 874 N/m2 with zero structural damping. The FE code was run using 30 × 30 finite elements. The results are shown in Table 1 and are in a good agreement with the results in the literature.

Table 1 Verification of the nonlinear forced vibration frequency responses of homogenous plates
Full size table

FG Plates Optimization

The work in this paper considers a square plate with a side length (\(a\)) of 40 cm and thickness (\(h\)) of 2 mm. The composite plate is made of Magnesium with elastic modulus (\({E}_{\mathrm{Mg}}\)) of 44 GPa, Poisson’s ratio (\({v}_{\mathrm{Mg}}\)) of 0.33, and density (\({\rho }_{\mathrm{Mg}}\)) of 1700 kg/m3 which is reinforced by Silicon Carbide nanoparticle with elastic modulus (\({E}_{\mathrm{SiC}}\)) of 570 GPa, Poisson’s ratio (\({v}_{\mathrm{SiC}}\)) of 0.19, and density (\({\rho }_{\mathrm{SiC}}\)) of 3200 kg/m3. The effective elastic properties of the nanocomposite are estimated using the upper-bound Hashin–Shtrikman model which can be curve-fitted to polynomials in terms of the reinforcement volume fraction as follows:

$$\begin{gathered} E = 44.14 + 289.54v_{f} + 152.67v_{f}^{2} \left( {{\text{GPa}}} \right) \hfill \\ v = 0.33 - 0.5160 v_{f} + 1.1816 v_{f}^{2} - 1.248 v_{f}^{3} \hfill \\ \rho = 3200 v_{f} + 1700\left( {1 - v_{f} } \right) \left( {{\text{Kg}}/{\text{m}}^{3} } \right). \hfill \\ \end{gathered}$$
(26)

The in-plane FG plates are optimized such that their fundamental frequencies equal the fundamental frequencies of the homogeneous plates using the minimum amount of the nano-reinforcement. As the problem is bi-symmetric, the material grading can be defined over the first quadrant of the plate with \({x}^{*}=2x/a, {y}^{*}=2y/a\) given that \({v}_{f}\left({y}^{*}\right)={v}_{f}\left({x}^{*}\right)\). The optimization statement can be written as follows:

$$\begin{gathered} {\text{minimize }}\;V_{f} = \mathop \int \limits_{0}^{1} \mathop \int \limits_{0}^{1} v_{f} \left( {x^{*} ,y^{*} } \right){\text{d}}x^{*} {\text{d}}y^{*} \hfill \\ {\text{Subjected}}\;{\text{to}}:\;\omega_{o}^{{{\text{FG}}}} = \omega_{o}^{{{\text{Homo}}.}} \hfill \\ 0 \le a_{o} \le 1, - 3 \le a_{i} \le 3, \mathop \sum \limits_{i = 0}^{{p_{x} }} ia_{i} = 0. \hfill \\ \end{gathered}$$
(27)

The considered homogenous plates have a 9% volume fraction of the silicon carbide nanoparticles. The optimum distribution of the volume fraction for the FG simply supported plate and the FG clamped plate are shown in Figs. 2 and 3 respectively. The optimization was done using the MATLAB gradient-based optimization toolbox. The total volume fraction of the in-plane FG simply supported plate is 7.9% and for the clamped FG plate is 5.36%. This is equivalent to a 14% saving in the nano-reinforcement for the simply supported plate and 70% savings for the clamped plate. The polynomial representation of the nano-reinforcement volume fraction can be written over the first quadrant of the plates as follows:

$$\begin{gathered} v_{f}^{{{\text{ss}}}} \left( {x^{*} } \right) = 0.0915x^{*} + 1.4581x^{*2} - 1.0025x^{*3} \hfill \\ v_{f}^{{{\text{cc}}}} \left( {x^{*} } \right) = 0.5777 - 2.8670x^{*} + 2.9978x^{*2} + 2.4451x^{*3} - 2.6160x^{*4} . \hfill \\ \end{gathered}$$
(28)
Fig. 2
figure 2

Distribution of the nanoparticles volume fraction for the FG clamped plate

Full size image
Fig. 3
figure 3

Distribution of the nanoparticles volume fraction for the FG simply supported plate

Full size image

The vibration mode shapes, and the non-dimensional natural frequencies of the in-plane FG clamped plate and the FG simply supported plate are shown in Figs. 4 and 5 respectively.

Fig. 4
figure 4

The first six natural mode shapes and nondimensional natural frequencies of the FG clamped plate.\(\left(\overline{\omega }=\omega /\sqrt{\left(\frac{\overline{D}}{\rho h{a }^{4}}\right)},\overline{D }= \frac{\overline{E}{h }^{3}}{12\left(1-{\overline{v} }^{2}\right)}\right)\)

Full size image
Fig. 5
figure 5

The first six natural mode shapes and nondimensional natural frequencies of the FG simply supported plate. \(\left(\overline{\omega }=\omega /\sqrt{\left(\frac{\overline{D}}{\rho h{a }^{4}}\right)},\overline{D }= \frac{\overline{E}{h }^{3}}{12\left(1-{\overline{v} }^{2}\right)}\right)\)

Full size image

Deterministic Nonlinear Vibration

This section presents the deterministic nonlinear forced and free vibration of the in-plane FG plates and their homogenous counterparts. The plates are loaded with a harmonic uniform pressure \((q)\) with a non-dimensional amplitude value (\(\overline{P }=\overline{q}{a }^{4}/{\overline{E}h }^{4}\)), where \(\overline{E }\) is the elastic modulus of the homogenous plates. A structural damping ratio of 0.05 is assumed for all the forced vibration study cases considered in this paper. The frequency response curves of the simply supported plates and the clamped plates are shown in Figs. 6 and 7 respectively. The simply supported FG plate has a slight increase in the nonlinear stiffening effect compared to the homogenous plate and vice versa for the clamped plates. and FG plates have less peak amplitude. The frequency response of FG plates for different pressure load values are shown in Figs. 8 and 9. The effect of uniaxial in-plane forces on the nonlinear free vibration in the pre-buckling and post-buckling states are shown in Figs. 10 and 11 for different values of the nondimensional in-plane force (\({N}^{*}=N/{\overline{N} }_{\mathrm{cr}}\)). The critical buckling load is \({\overline{N} }_{\mathrm{cr}}={K}_{\mathrm{cr}} {\pi }^{2}\overline{E}{h }^{3}/12a(1-{\overline{v} }^{2})\),where \({K}_{\mathrm{cr}}\) is 2 for simply supported boundary condition and 5.262 for clamped boundary conditions. For the simply supported plates, the FG plate and the homogenous plate have the same critical buckling load, so the difference is only in the non-linear stiffening effect which cause the FG plate to have slightly less amplitude. On the other hand, the FG clamped plate has 3.5% lower critical buckling load than the homogenous plate which causes lower linear frequency and higher vibration amplitude, besides that the FG clamped plate has less non-linear stiffness.

Fig. 6
figure 6

Free and forced vibration amplitude of the clamped plates \(\overline{P }=15\)

Full size image
Fig. 7
figure 7

Free and forced vibration amplitude of the simply supported plates \(\overline{P }=5\)

Full size image
Fig. 8
figure 8

Free and forced vibration amplitude of the FG clamped plate

Full size image
Fig. 9
figure 9

Free and forced vibration amplitude of the FG simply supported plate

Full size image
Fig. 10
figure 10

The effect of in-plane compression force on the nonlinear free vibration of the clamped plates

Full size image
Fig. 11
figure 11

The effect of in-plane compression force on the nonlinear free vibration of the simply supported plates

Full size image

Non-deterministic Nonlinear Vibration

The effect of the elastic modulus uncertainty on the nonlinear vibration of in-plane FG plates is investigated by considering an uncertain random field of the elastic modulus. The Karhunen–Loève expansion is used to generate the random field for different values of the non-dimensional correlation length (\({l}_{c}^{*}={l}_{c}/a\)). Figures 12, 13 and 14 show realizations for the normalized random field \([ E\left(x,y\right)-{\mu }_{E}\left(x,y\right) ] /{\sigma }_{E}(x,y)\) for non-dimensional correlation lengths of 1, 0.6 and 0.3 respectively. The uncertainty quantification process starts with assessing the uncertainty in the linear and nonlinear stiffness coefficients in Eq. (18). These coefficients are linearly dependent on the elastic modulus, so the first-order Hermite expansion is sufficient to represent their uncertainty. In this study, the coefficient of variation [C.O.V. = \({\sigma }_{E}(x,y\))/ \({\mu }_{E}\left(x,y\right)\)] of the elastic modulus is considered using two models: the first model assumes a uniform C.O.V of 5% at any point in the random field, the second model assumes a linear dependency of the uncertainty on the nanoparticle volume fraction as 5 [\({v}_{f}(x,y)/0.3\)] %. The linear C.O.V model might be more realistic as pure magnesium should have low uncertainty levels, besides that the uncertainty is caused by multiple sources like, the manufacturing method and the homogenization mathematical model which intuitively might lead to increases uncertainty as the volume fraction increases. The coefficients of the Hermite expansion are estimated by using LHS sampling with number of realizations equals the number of terms in the expansion. The uncertainty parameters of the linear and non-linear stiffness coefficients for the uniform C.O.V model are shown in Tables 2 and 3 respectively. For the random variable representation (\({l}_{\mathrm{c}}=\infty\)) of the elastic modulus, both homogenous plates and the FG plates have the same level of uncertainty and as the correlation length decreases the level of uncertainty decreases which is intuitive and this reduction is slow with respect to the correlation length. The FG plates have slightly higher uncertainty level in the linear stiffness compared to the homogenous plates and vice versa for the nonlinear stiffness, but these differences are within 0–4% so they can be considered negligible.

Fig. 12
figure 12

One realization of the normalized elastic modulus random field for \({l}_{\mathrm{c}}=a\)

Full size image
Fig. 13
figure 13

One realization of the normalized elastic modulus random field for \({l}_{\mathrm{c}}=0.6 a\)

Full size image
Fig. 14
figure 14

One realization of the normalized elastic modulus random field for \({l}_{\mathrm{c}}=0.3 a\)

Full size image
Table 2 Uncertainty parameters of the non-dimensional linear frequency \({k}_{1}^{*}={k}_{1}/\left(\frac{\overline{D}}{\rho h{a }^{4}}\right),\overline{D }= \frac{\overline{E}{h }^{3}}{12(1-{\overline{v} }^{2})}\) for the uniform C.O.V model
Full size table
Table 3 Uncertainty parameters of the non-dimensional linear stiffness \({k}_{3}^{*}={k}_{3}/\left(\frac{\overline{D}}{\rho h{a }^{4}}\right),\overline{D }= \frac{\overline{E}{h }^{3}}{12(1-{\overline{v} }^{2})}\) for the uniform C.O.V model
Full size table

For the case of the linear C.O.V model, the results are shown in Tables 4 and 5. The uncertainty level of the FG simply supported plate is higher than the homogenous plate and it reaches double the value of the homogenous plate for small correlation lengths (\({l}_{c}^{*}=0.3\)). On the other hand, the uncertainty level of the FG clamped plate nonlinear stiffness is approximately 20% higher than the homogenous plate for small correlation lengths, while the uncertainty level of the linear stiffness is higher with a 50% increase. FG clamped plate has less uncertainty level than the FG simply supported plate. In general, linear C.O.V. model yields 1/3 the uncertainty level in the output variables compared to the uniform C.O.V. model. The reason that FG plates have more uncertainty levels in the stiffness coefficients is due to two reasons: the first reason is that the overall stiffness of the FG plates is controlled by the stiffness of localized regions with high volume fraction which makes it more sensitive to any uncertainty in the stiffness of these regions and the second reason is that regions with high volume fraction have more uncertainty level than regions with low volume fractions. It should be noted that the uncertainty in the linear vibration frequencies will be half the values given in Tables 2, 3, 4 and 5. The values are given in nondimensional form where \(\overline{E }\), and \(\overline{v }\) are the Young’s modulus and Poisson’s ration of the homogenous plates.

Table 4 Uncertainty parameters of the non-dimensional linear stiffness \({k}_{1}={k}_{1}/\left(\frac{\overline{D}}{\rho h{a }^{4}}\right),\overline{D }= \frac{\overline{E}{h }^{3}}{12(1-{\overline{v} }^{2})}\) for linear the C.O.V model
Full size table
Table 5 Uncertainty parameters of the non-dimensional non-linear stiffness \({k}_{3}^{*}={k}_{3}/\left(\frac{\overline{D}}{\rho h{a }^{4}}\right),\overline{D }= \frac{\overline{E}{h }^{3}}{12(1-{\overline{v} }^{2})}\) for the linear C.O.V model
Full size table

The 6-sigma uncertainty interval of the nonlinear response of the in-plane FG plates for the random variable representation of the elastic modulus is shown in Figs. 15, 16, 17 and 18. The uncertain nonlinear response for other correlation lengths will be bounded between these two curves. It can be seen that the level of uncertainty in the vibration amplitude depends on the excitation frequency for a given force amplitude. The non-dimensional maximum deflection (\({W}^{*}={w}_{\mathrm{max}}/h\)) can be expanded using Hermite polynomials in terms of one auxiliary random variable (\({\mathbb{Z}}\)) because the linear and the non-linear stiffness coefficients are perfectly correlated as follows:

$$\begin{gathered} {\mathbb{K}}_{1} = \mu_{{k_{1} }} + {\mathcal{S}}_{{k_{1} }} {\mathbb{Z}} \hfill \\ {\mathbb{K}}_{3} = \mu_{{k_{3} }} + {\mathcal{S}}_{{k_{3} }} {\mathbb{Z}} \hfill \\ \end{gathered}$$
(29)
Fig. 15
figure 15

Uncertainty bounds of the nonlinear vibration of the FG clamped plate \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=10\) for the uniform C.O.V. model

Full size image
Fig. 16
figure 16

Uncertainty bounds of the nonlinear vibration of the FG simply supported plate \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=3\) for the uniform C.O.V. model

Full size image
Fig. 17
figure 17

Uncertainty bounds of the nonlinear vibration of the FG clamped plate \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=10\) for the linear C.O.V. model

Full size image
Fig. 18
figure 18

Uncertainty bounds of the nonlinear vibration of the FG simply supported plate \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=3\) for the linear C.O.V. model

Full size image

The uncertainty parameters of the vibration amplitude at \(\omega ={\mu }_{{\omega }_{o}}\) for the uniform C.O.V model and the linear C.O.V model are given in Tables 6 and 7 respectively. In the case of the uniform C.O.V model, the uncertainty level in the deflection is double the uncertainty level in the elastic modulus when \({l}_{c}^{*}=\infty\) and the clamped plate has higher uncertainty level compared to the simply supported plate. In the case of linear C.O.V model, the uncertainty level in the deflection is lower than the maximum uncertainty level in the elastic field besides, both simply supported and clamped plates show similar uncertainty levels.

Table 6 Uncertainty parameters of the non-dimensional maximum deflection \({W}^{*}={w}_{max}/h\) in the case of the uniform COV model at \(\omega ={\mu }_{{\omega }_{o}}\) and \({\overline{P} }_{ss}=3\), \({\overline{P} }_{cc}=10\)
Full size table
Table 7 Uncertainty parameters of the non-dimensional maximum deflection \({W}^{*}={w}_{max}/h\) in the case of the linear COV model at \(\omega ={\mu }_{{\omega }_{o}}\) and \({\overline{P} }_{ss}=3\), \({\overline{P} }_{cc}=10\)
Full size table

For a given load value, there is a frequency at which the uncertainty level in the vibration amplitudes is the highest which is represented by the blue dashed lines in Figs. 15, 16, 17 and 18. The Histograms of the maximum deflection for the FG clamped plate and the FG simply supported plate are given in Figs. 19, 20, 21 and 22 respectively which show that the deflection histograms slightly deviates for the normal distribution. The second order Hermite expansion is sufficient for representing the deflection which can be written for the random variable representation of the elastic field in case of the uniform C.O.V. model as follows:

$$\begin{gathered} {\mathbb{W}}_{ss}^{*} = 0.4806 - 0.0399 H_{1} \left( {\mathbb{Z}} \right) + 0.0014 H_{2} \left( {\mathbb{Z}} \right) \hfill \\ {\mathbb{W}}_{cc}^{*} = 0.6998 - 0.0736 H_{1} \left( {\mathbb{Z}} \right) + 0.0027 H_{2} \left( {\mathbb{Z}} \right). \hfill \\ \end{gathered}$$
(30)
Fig. 19
figure 19

Histogram of the nonlinear forced vibration amplitude at \(\omega ={\mu }_{{\omega }_{o}}\) for the FG clamped plate for \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=10\) and uniform C.O.V model

Full size image
Fig. 20
figure 20

Histogram of the forced nonlinear vibration amplitude at \(\omega ={\mu }_{{\omega }_{o}}\) for the FG simply supported plate for \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=3\) and uniform C.O.V model

Full size image
Fig. 21
figure 21

Histogram of the nonlinear forced vibration amplitude at \(\omega ={\mu }_{{\omega }_{o}}\) for the FG clamped plate for \({l}_{\mathrm{c}}=\infty\),\(\overline{ P }=10\) and linear C.O.V model

Full size image
Fig. 22
figure 22

Histogram of the nonlinear forced vibration amplitude at \(\omega ={\mu }_{{\omega }_{o}}\) for the FG simply supported plate for \({l}_{\mathrm{c}}=\infty\),\(\overline{P }=3\) and linear C.O.V model

Full size image

And for the linear C.O.V. model as:

$$\begin{gathered} {\mathbb{W}}_{ss}^{*} = 0.4794 - 0.0170 H_{1} \left( {\mathbb{Z}} \right) + 0.0003 H_{2} \left( {\mathbb{Z}} \right) \hfill \\ {\mathbb{W}}_{cc}^{*} = 0.6974 - 0.0268H_{1} \left( {\mathbb{Z}} \right) + 0.0003 H_{2} \left( {\mathbb{Z}} \right). \hfill \\ \end{gathered}$$
(31)

The effect of the in-plane compression forces on the first-mode vibration frequencies in the pre-buckling and post-buckling states are shown in Figs. 23 and 24 which are obtained by solving Eq. (15) using the Newton–Raphson method and then linearized about the post-buckled state to estimate the frequencies. As the in-plane forces increase the uncertainty level in the frequency increases then it vanishes near the mean buckling load, it again increases to a maximum value, then it keeps slowly decreasing as the in-plane load increases. The uncertainty nature of the frequncies is the same as it was for the vibration amplitude with a near normal distribution, while the uncertainty levels can reach 6 times the maximum uncertainty level in the elastic field (the frequency maximum c.o.v. = 33%) for both the uniform C.O.V model or the linear C.O.V. model.

Fig. 23
figure 23

6-sigma confidence interval of the first mode frequency of the FG clamped plate vs. the in-plane compression force. Uniform C.O.V model (dashed lines) linear C.O.V model (solid lines)

Full size image
Fig. 24
figure 24

6-sigma confidence interval of the first mode frequency of the FG simply supported plate vs. the in-plane compression force. Uniform C.O.V model (dashed lines) linear C.O.V model (solid lines)

Full size image

For the post-buckling forced vibration amplitude uncertainty analysis, the FG simply supported plate with \({N}^{*}=1.5\) is considered as a study case. The fourth-order Runge–Kutta method is used to simulate the vibration amplitude and 5000 LHS realizations are used for the uncertainty quantification analysis. It was found that if the time response of the plate is periodic and its nature is the same within the uncertainty bounds of the stiffness coefficients, then the vibration amplitude will follow a normal distribution exactly as for the pre-buckling state as shown in Figs. 25, 26, 27, 28, 29 and 30. If the periodic response nature changes within the uncertainty bounds of the stiffness coefficients, then the vibration amplitude will follow a distribution with two peaks at the lower and higher bounds as shown in Figs. 31, 32, 33 and 34. Therefore, both the uniform C.O.V. and the linear C.O.V. elastic field models yield the same uncertainty level in the vibration amplitude. Finally, if the response is chaotic, the vibration amplitude distribution will be non-smooth with single peak as shown in Figs. 35 and 36.

Fig. 25
figure 25

One realization of time response of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.5{\mu }_{{\omega }_{o}}\),\(\overline{ P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 26
figure 26

One realization of phase plot of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.5{\mu }_{{\omega }_{o}}\),\(\overline{P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 27
figure 27

Histogram the forced vibration amplitude of the FG simply supported plate at \(\omega =0.5{\mu }_{{\omega }_{o}}\),\(\overline{P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 28
figure 28

One realization of time response of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.1{\mu }_{{\omega }_{o}}\),\(\overline{P }=5\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 29
figure 29

One realization of phase plot of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.1{\mu }_{{\omega }_{o}}\),\(\overline{P }=5\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 30
figure 30

Histogram the forced vibration amplitude of the FG simply supported plate at \(\omega =0.1{\mu }_{{\omega }_{o}}\),\(\overline{ P }=5\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 31
figure 31

One realization of time response of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.05{\mu }_{{\omega }_{o}}\),\(\overline{P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 32
figure 32

One realization of time response of the forced vibration amplitude of the FG simply supported plate at \(\omega =0.05{\mu }_{{\omega }_{o}}\),\(\overline{ P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 33
figure 33

Histogram the forced vibration amplitude of the FG simply supported plate at \(\omega =0.05{\mu }_{{\omega }_{o}}\),\(\overline{ P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 34
figure 34

Histogram the forced vibration amplitude of the FG simply supported plate at \(\omega =0.1{\mu }_{{\omega }_{o}}\),\(\overline{P }=5\) and \({N}^{*}=1.5\) for the linear C.O.V model

Full size image
Fig. 35
figure 35

One realization of phase plot of the forced vibration amplitude of the FG simply supported plate at \(\omega ={\mu }_{{\omega }_{o}}\),\(\overline{P }=5\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image
Fig. 36
figure 36

Histogram the forced vibration amplitude of the FG simply supported plate at \(\omega ={\mu }_{{\omega }_{o}}\),\(\overline{P }=3\) and \({N}^{*}=1.5\) for the uniform C.O.V model

Full size image

Conclusions

The results of the optimization and the vibration uncertainty analysis of the in-plane functionally graded plates can be concluded in the following points:

  • The in-plane optimization of the nano-reinforcement volume fraction saves 14% in the case of the simply supported plate and 70% in the case of the clamped plate compared to the homogenous plates with the same fundamental frequncies.

  • The elastic modulus random field modeling has a great impact on the uncertainty levels of the vibration amplitude and frequencies; for the uniform coefficient of variation elastic field, both the FG plates and the homogenous plates showed similar uncertainty levels in the vibration amplitude and frequency, but for the linear coefficient of variation elastic field, the FG plates may have double the uncertainty levels of the homogenous plates.

  • The linear coefficient of variation elastic field yields uncertainty levels in the output variables that are 1/3 the uncertainty levels in the outputs of the uniform coefficient of variation elastic field.

  • In the pre-buckling state, the uncertainty in the vibration amplitude follows a slightly skewed normal distribution and its coefficient of variation might be multiple the maximum coefficient of variation of the elastic field depending on the excitation frequency.

  • For the post-buckling state, the vibration amplitude probability distribution might be normal, non-normal, smooth, or non-smooth depending on the nature of the response (periodic or chaotic).