1 Introduction

A continuum is a compact connected non-degenerate metric space. A subcontinuum of a continuum X is a nonempty connected closed subset of X, so singletons are subcontinua. An arc is a continuum homeomorphic to the interval [0, 1]. Let \(X^{2}\) denote the Cartesian square of X and \(\Delta \) the diagonal of \(X^{2}\).

The authors studied in [6] conditions under which \(\Delta \) satisfies some of the properties described in [2, Table 1] and [8, Definition 1.1], for a number of examples and families of continua.

Recently, H. Katsuura [7] proved that the arc is the only continuum for which \(\Delta \) does not satisfy that \(X^{2}{\setminus } \Delta \) is connected, and he included the following question [7, p. 4] (Katsuura mentioned that this question was suggested by Wayne Lewis of Texas Tech University in a private conversation):

Question 1.1

If X is a continuum other than the arc, is \(X^{2}\setminus \Delta \) continuumwise connected if and only if X is decomposable?

As an application of dynamical systems theory to continuum theory, we use the dynamical properties of a particular homeomorphism from the Cantor set onto itself to prove that the necessity in Question 1.1 is not satisfied. In fact, we show that no implication in Question 1.1 is satisfied.

2 No Sufficiency

A map is a continuous function. Given continua X and Y, and a number \(\varepsilon >0\), an \(\varepsilon \)-map is an onto map \(f:X\rightarrow Y\) such that for each \(y\in Y\), diameter(\(f^{-1}(y))<\varepsilon \). A continuum X is arc-like provided that for each \(\varepsilon >0\), there exists an \(\varepsilon \)-map \(f:X\rightarrow [0,1]\).

Lemma 2.1

Let X be an arc-like continuum and \(p,q\in X\) such that \(p\ne q\). Then, for every continuum \(K\subset X^2\) containing the points (p, q) and (q, p), \(K\cap \Delta \ne \emptyset \).

Proof

Let d be a metric for X. Let K be a continuum in \(X^2\) such that \((p,q),(q,p)\in K\), for some points \(p\ne q\in X\).

Suppose that \(K\cap \Delta = \emptyset \). Hence, there exists \(\varepsilon >0\) such that for every point \((x,y)\in K\), \(d(x,y)>\varepsilon \). Since X is an arc-like continuum, there exists an \(\varepsilon \)-map \(\lambda :X \rightarrow [0,1]\). Then, for each \((x,y)\in K\), \(\lambda (x)\ne \lambda (y)\).

If there were points \((x_1,y_1),(x_2,y_2)\in K\) such that \(\lambda (x_1)<\lambda (y_1)\) and \(\lambda (y_2)<\lambda (x_2)\). Define \(g:K\rightarrow [-1,1]\) by \(g(x,y)=\lambda (x)-\lambda (y)\). We have that g is continuous, \(g(x_1,y_1)<0\) and \(g(x_2,y_2)>0\). Since K is connected, by the Intermediate Value Theorem, there is \((x_0,y_0)\in K\) such that \(g(x_0,y_0)=0\). So, \(\lambda (x_0)=\lambda (y_0)\), a contradiction.

Thus, we obtain that either \(\lambda (x)<\lambda (y)\) for each \((x,y)\in K\) or \(\lambda (y)<\lambda (x)\) for each \((x,y)\in K\). This contradicts the fact that \((p,q),(q,p)\in K\). \(\square \)

Corollary 2.2

Let X be an arc-like continuum. Then, \(X^{2}\setminus \Delta \) is not continuumwise connected.

Since there are decomposable arc-like continua (for example, the sin\((\frac{1}{x})\)-curve), Corollary 2.2 shows that the sufficiency in Question 1.1 is not satisfied. We also proved in [6, Sect. 7] that, in fact the sin\((\frac{1}{x})\)-curve belongs to a family of curves for which \(\Delta \) satisfies a stronger property in \(X^{2}\). S. B. Nadler, Jr. [10, p. 329] named the compactifications of the ray \([0,\infty )\) whose remainder is an arc as Elsa continua and he proved that they are arc-like [9, Lemma 6] (in fact, it is easy to show that a compactification of \([0,\infty )\) with non-degenerate remainder, is arc-like if and only if the remainder is an arc-like continuum). Then, for each Elsa continuum, X is decomposable and \(X^{2}\setminus \Delta \) is not continuumwise connected.

3 No Necessity

In this section, we present an indecomposable continuum X such that \(X^{2}\setminus \Delta \) is continuumwise connected.

Definition 3.1

Let X be a continuum and A a subcontinuum of X with int\(_{X} (A) =\emptyset \). We say that A is a continuum of colocal connectedness in X if for each open subset U of X with \(A\subset U\), there exists an open subset V of X such that \(A\subset V \subset U\) and \(X\setminus V\) is connected.

By [2, Table 1] and [8, Sect. 1], it is known that if \(X^{2}\) is a continuum and \(\Delta \) is a subcontinuum of \(X^{2}\) which has properties from Definition 3.1, then \(X^{2}{\setminus } \Delta \) is continuumwise connected.

The construction and proof of the properties of X strongly depends on the dynamical properties of a particular homeomorphism of the Cantor set onto itself.

Let C denote the Cantor ternary set. In this section, we will use a homeomorphism \(f:C\rightarrow C\) such that f is minimal (C does not contain any proper nonempty closed subset A such that \(f(A)=A\), or equivalently, every orbit of f is dense) and f is weakly mixing (for any two nonempty subsets U, V of \(X^{2}\), there exists \(n\in {\mathbb {N}}\) such that \((f\times f)^{n}(U)\cap V\ne \emptyset \)). A recent reference for the existence of such homeomorphisms is [3, Theorem 4.1]. It is known that the inverse of \(f^{-1}\) is also minimal [1, Theorem 6.2 (e)]. By [4, Proposition 2], \(f\times f\) has a dense orbit.

We consider the space X obtained by identifying in \(C\times [0,1]\), for each \(p\in C\), the points (p, 1) and (f(p), 0). Let \(\varphi :C\times [0,1]\rightarrow X\) be the quotient map and let \(\sigma =\varphi \times \varphi :(C\times [0,1])^{2}\rightarrow X^{2}\). Let \(\rho \) be the metric on \(C\times [0,1]\) given by \(\rho ((p,s),(q,t))=\vert p-q\vert +\vert s-t\vert \). Choose and fix any metric D for the space X.

In the hypothesis of the following theorem, we write the specific properties that we use of the homeomorphism f.

Theorem 3.2

Suppose that \(f:C\rightarrow C\) is a homeomorphism such that the orbits of f and \(f^{-1}\) are dense and \(f\times f:C^{2}\rightarrow C^{2}\) has a dense orbit. Then, X is an indecomposable continuum such that the diagonal \(\Delta \) in \(X^{2}\) is colocally connected.

Proof

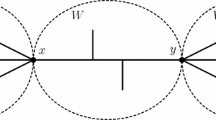
The indecomposability of X is proved in [5, Corollary, p. 552]. So we only need to show that \(\Delta \) is colocally connected in \(X^{2}\). In order to do this, take an open subset U in \(X^{2}\) such that \(\Delta \subset U\). Then, there exists \(\varepsilon >0\) such that for any two points \(x,y\in X\), if \(D(x,y)<\varepsilon \), then \((x,y)\in U\). Choose and fix \(\delta >0\) such that \(\delta <\frac{1}{10}\) and if \((p,s),(q,t)\in C\times [0,1]\) and \(\rho ((p,s),(q,t))<2\delta \), from the continuity of \(\varphi \times \varphi \) it follows that \(D(\varphi (p,s),\varphi (q,t))<\frac{\varepsilon }{3}\) (hence \(\sigma ((p,s),(q,t))\in U\)).

Define

$$\begin{aligned}{} & {} V_{1}=\{((p,s),(q,t))\in (C\times [0,1])^{2}:\vert p-q\vert<\delta \text { and }\vert t-s \vert<\delta \}, \\{} & {} \quad V_{2}=\{((p,s),(q,t))\in (C\times [0,1])^{2}:\vert p-f(q)\vert<\delta , s<\delta \text { and }1-\delta<t\},\\{} & {} \quad V_{3}=\{((p,s),(q,t))\in (C\times [0,1])^{2}:\vert f(p)-q\vert<\delta , t<\delta \text { and }1-\delta <s\},\\{} & {} \qquad V_{0}=V_{1}\cup V_{2}\cup V_{3} \end{aligned}$$

and

$$\begin{aligned} K=(C\times [0,1])^{2}\setminus V_{0}. \end{aligned}$$

We check that K has the following properties.

(a) \(\sigma (K)\) is a continuum, and

(b) \(\Delta \subset X^{2}\setminus \sigma (K)\subset U\).

In order to prove (a), observe that \(V_{0}\) is open in \((C\times [0,1])^{2}\), so K and \(\sigma (K)\) are compact, so we only need to show that \(\sigma (K)\) is connected. Let

$$\begin{aligned} M=(C\times \{0\})\times (C\times \{\frac{1}{2}\}). \end{aligned}$$

Notice that \(M\subset K\).

Claim 1. Let \(z=((p,s),(q,t))\in K\) be such that \(s\le t\). Then, there exists a subcontinuum A of K such that \(z,((p,0),(q,\frac{1}{2}))\in A\).

We prove Claim 1. We consider two cases.

Case 1. \(2\delta \le s\) or \(t\le 1-\delta \).

For each \(r\in [0,1]\), let \(u(r)=rs\) and \(v(r)=rt+(1-r)(t-s)\). Define \(\lambda (r)=((p,u(r)),(q,v(r)))\) and \(A_{1}=\{\lambda (r):r\in [0,1]\}\). Then, \(z,((p,0),(q,t-s))\in A_{1}\). Take \(r\in [0,1]\), observe that \(u(r)\le v(r)\le t\) and \(v(r)-u(r)=t-s\). Since \(u(r)\le v(r)\) and \(\delta <1-\delta \), we have that \(\lambda (r)\notin V_{3}\). Since \(v(r)-u(r)=t-s\) and \(z\notin V_{1}\), we have that \(\lambda (r)\notin V_{1}\).

In the case that \(t\le 1-\delta \), we have that \(v(r)\le 1-\delta \), Hence, \(\lambda (r)\notin V_{2}\).

Now, we consider the case that \(2\delta \le s\). If \(r\le \frac{1}{2}\), then \(\delta \le (1-r)2\delta \le (1-r)s\), so \(t\le 1\le 1-\delta +(1-r)s\). This implies that \(v(r)=rt+(1-r)(t-s)\le 1-\delta \). Thus, \(\lambda (r)\notin V_{2}\). If \(\frac{1}{2}\le r\), then \(\delta \le \frac{s}{2}\le rs=u(r)\). Hence, \(\lambda (r)\notin V_{2}\).

This completes the proof that for each \(r\in [0,1]\), \(\lambda (r)\in K\). Therefore, \(A_{1}\subset K\).

Given \(r\in [0,1]\), let \(w(r)=r(t-s)+(1-r)\frac{1}{2}\) and \(\eta (r)=((p,0),(q,w(r)))\). Let \(A_{2}=\{\eta (r):r\in [0,1]\}\). Observe that \(((p,0),(q,t-s)), ((p,0),(q,\frac{1}{2}))\in A_{2}\) and \(\eta (r)\notin V_{3}\).

Take \(r\in [0,1]\). Since \(\lambda (0)\in K\), we have that \(((p,0),(q,t-s))\notin V_{1}\cup V_{2}\). Since \(((p,0),(q,t-s))\notin V_{1}\), we have that either \(\delta \le \vert p-q\vert \) or \(\delta \le t-s\). If \(\delta \le \vert p-q\vert \), it is clear that \(\eta (r)\notin V_{1}\). If \(\delta \le t-s\), since \(\delta \le \frac{1}{2}\), we have that \(\delta \le w(r)\). Thus, \(\eta (r)\notin V_{1}\). Since \(((p,0),(q,t-s))\notin V_{2}\), we have that either \(\delta \le \vert p-f(q)\vert \) or \(t\le 1-\delta \). If \(\delta \le \vert p-f(q)\vert \), it is clear that \(\eta (r)\notin V_{2}\). If \(t\le 1-\delta \), since \(\frac{1}{2}\le 1-\delta \), we have that \(w(r)\le 1-\delta \). Thus, \(\eta (r)\notin V_{2}\). We have shown that for each \(r\in [0,1]\), \(\eta (r)\notin V_{1}\cup V_{2}\cup V_{3}\). Hence, \(A_{2}\subset K\).

Define \(A=A_{1}\cup A_{2}\). We obtained that \(A_1=\{\lambda (r):r\in [0,1]\}\subset K\) and \(z,((p,0),(q,t-s))\in A_{1}\). Also, \(A_{2}=\{\eta (r):r\in [0,1]\}\subset K\) and \(((p,0),(q,t-s)), ((p,0),(q,\frac{1}{2}))\in A_{2}\). Hence, \(A=A_1\cup A_2\) is a closed and connected subset of K such that \(z,((p,0),(q,\frac{1}{2}))\in A\).

Case 2. \(s<2\delta \) and \(1-\delta <t\).

Given \(r\in [0,1]\), let \(y(r)=r(1-\delta )+(1-r)t\) and \(\gamma (r)=((p,s),(q,y(r)))\). Let \(A_{3}=\{\gamma (r):r\in [0,1]\}\). Notice that \(z,((p,s),(q,1-\delta ))\in A_{3}\) and for each \(r\in [0,1]\), \(1-\delta \le y(r)\), so \(\gamma (r)\notin V_{3}\) and \(\delta <\frac{7}{10}\le y(r)-s\). Thus, \(\gamma (r)\notin V_{1}\). Since \(z\notin V_{2}\) and \(1-\delta < t\), we have that either \(\delta \le \vert p-f(q)\vert \) or \(\delta \le s\), in both cases it is clear that \(\gamma (r)\notin V_{2}\). This completes the proof that for each \(r\in [0,1]\), \(\gamma (r)\in K\). Therefore, \(A_{3}\subset K\).

We apply Case 1 to the point \(z_{0}=((p,s),(q,1-\delta ))\), so there exists a subcontinuum \(A_{4}\) of K such that \(z_{0},((p,0),(q,\frac{1}{2}))\in A_{4}\). Define \(A=A_{3}\cup A_{4}\). Then, A has the required properties.

This finishes the proof of Claim 1.

By the symmetry of the roles of both coordinates in the definition of \(V_{0}\), we obtain that the following claim also holds.

Claim 2. Let \(z=((p,s),(q,t))\in K\) be such that \(t\le s\). Then, there exists a subcontinuum A of K such that \(z,((p,\frac{1}{2}),(q,0))\in A\).

Claim 3. Let \(z=((p,s),(q,t))\in K\). Then, there exists a subcontinuum B of \(\sigma (K)\) such that \(\sigma (z)\in B\) and \(B\cap \sigma (M)\ne \emptyset \).

The proof for the case \(s\le t\) follows from Claim 1. So we may suppose that \(t\le s\). By Claim 2, there exists a subcontinuum \(A_{5}\) of K such that \(z,((p,\frac{1}{2}),(q,0))\in A_{5}\). Let \(A_{6}=\{((p,\frac{1}{2}+r),(q,r)):r\in [0,\frac{1}{2}]\}\). Clearly, \(((p,\frac{1}{2}),(q,0)),((p,1),(q,\frac{1}{2}))\in A_{6}\) and \(A_{6}\subset K\). Let \(A=A_{5}\cup A_{6}\). Then, A is a subcontinuum of K, \(z\in A\) and \(\sigma ((p,1),(q,\frac{1}{2}))=\sigma ((f(p),0),(q,\frac{1}{2}))\in \sigma (A)\cap \sigma (M)\). Hence, \(B=\sigma (A)\) satisfies the required properties.

Claim 4. Let \(z=((p,0),(q,\frac{1}{2}))\in M\). Then, there exists a subcontinuum E of \(\sigma (K)\) such that \(\sigma (z),\sigma ((f(p),0),(f(q),\frac{1}{2}))\in E\).

In order to prove the existence of E, let \(A=\{((p,r),(q,\frac{1}{2}+r)):r\in [0,\frac{1}{2}]\}\cup \{((p,\frac{1}{2}+r),(f(q),r)):r\in [0,\frac{1}{2}]\}\). Since \(\varphi (q,1)=\varphi (f(q),0)\) and \(\varphi (p,1)=\varphi (f(p),0)\), we obtain that \(E=\sigma (A)\) satisfies the required properties. Hence, Claim 4 is proved.

Now, choose and fix a point \((p_{0},q_{0})\in C^{2}\) such that \((p_{0},q_{0})\) has a dense orbit under \(f\times f\). By Claim 4, there exists a sequence \(E_{1},E_{2},E_{3}\ldots \) of subcontinua of \(\sigma (K)\) such that for each \(n\in {\mathbb {N}}\), \(\sigma ((f^{n-1}(p_{0}),0),(f^{n-1}(q_{0}),\frac{1}{2})),\sigma ((f^{n}(p_{0}),0),(f^{n}(q_{0}),\frac{1}{2}))\in E_{n}\). Then, the set \(E_0=E_{1}\cup E_{2}\cup E_{3}\cup \cdots \) is a connected subset of \(\sigma (K)\) and \(\sigma ((p_{0},0),(q_{0},\frac{1}{2}))\in E_{0}\).

Choose any point \(((p,0),(q,\frac{1}{2}))\in M\). Since \((p_{0},q_{0})\) has a dense orbit under \(f\times f\) and \(\sigma \) is continuous, we have that \(\sigma ((p,0),(q,\frac{1}{2}))\in \sigma ({{\,\textrm{cl}\,}}_{K^{2}}(\{((f^{n}(p_{0}),0),(f^{n}(q_{0}),\frac{1}{2})):n\in {\mathbb {N}}\}))\subset {{\,\textrm{cl}\,}}_{X^{2}}(E_0)\). Since \(E_0\) is connected and \(E_0\subset E_0\cup \sigma (M)\subset {{\,\textrm{cl}\,}}_{X^{2}}(E_0)\), we have that \(W=E_0\cup \sigma (M)\) is a connected subset of \(\sigma (K)\).

Given any point \(z\in K\), by Claim 3, there is a subcontinuum \(B_{z}\) of \(\sigma (K)\) such that \(\sigma (z)\in B_{z}\) and \(B_{z}\cup \sigma (M)\ne \emptyset \). So, \(\sigma (K)=W\cup (\cup \{B_{z}:z\in K\})\) is connected. This ends the proof of (a).

In order to prove (b), take \(\sigma (z)\in \Delta \), where \(z=((p,s),(q,t))\). Then, \(\varphi (p,s)=\varphi (q,t)\). Thus, either \((p,s)=(q,t)\) or, \(t=1\) and \((p,s)=(f(q),0)\), or, \(s=1\) and \((q,t)=(f(p),0)\). In the first case, \(z\in V_{1}\), in the second \(z\in V_{2}\) and in the third one, \(z\in V_{3}\). In any case, \(z\notin K\). We have shown that \(\Delta \subset X^{2}{\setminus } \sigma (K)\).

Now, we prove that \(X^{2}\setminus \sigma (K)\subset U\). Take an element \(w=\sigma (z)\), where \(z=((p,s),(q,t))\notin K\). Then, \(z\in V_{1}\cup V_{2}\cup V_{3}\). We consider three cases.

Case 1. \(z\in V_{1}\).

In this case, \(\vert p-q\vert <\delta \) and \(\vert t-s\vert <\delta \), and by the definition of \(\delta \), \(\sigma (z)\in U\).

Case 2. \(z\in V_{2}\).

In this case, \(\vert p-f(q)\vert<\delta , s<\delta \) and \(1-\delta <t\). Then, \(\rho ((p,s),(p,0))<\delta \), \(\rho ((p,0),(f(q),0))<\delta \) and \(\rho ((q,1),(q,t))<\delta \). So, \(D(\varphi (p,s),\varphi (p,0))<\frac{\varepsilon }{3}\), \(D(\varphi (p,0),\varphi (f(q),0))<\frac{\varepsilon }{3}\) and \(D(\varphi (q,1),\varphi (q,t))<\frac{\varepsilon }{3}\). Since \(\varphi (f(q),0)=\varphi (q,1)\), we have that \(D(\varphi (p,s),\varphi (q,t))<\varepsilon \). By the choice of \(\varepsilon \), we conclude that \(\sigma (z)=(\varphi (p,s),\varphi (q,t))\in U\).

Case 3. \(z\in V_{3}\).

This case is similar to Case 2. This completes the proof that \(X^{2}\setminus \sigma (K)\subset U\). Therefore, (b) holds.

Finally, define \(V=X^{2}\setminus \sigma (K)\). Then, V is an open subset of \(X^{2}\) such that \(\Delta \subset V\subset U\), and \(X^{2}\setminus V\) is connected. This finishes the proof that \(\Delta \) is colocally connected in \(X^{2}\). \(\square \)