Modeling of Elastic Waves in a Fluid Loaded and Immersed Piezoelectric Hollow Fiber

Abstract

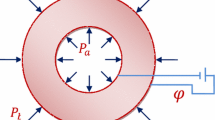
Modeling of elastic waves in an infinite fluid-loaded and immersed homogeneous, transversely isotropic piezoelectric circular fiber is studied using linear elasticity theory. The equations of motion of the fiber are formulated using the constitutive equations of a transversely isotropic piezoelectric material. The equations of motion of the internal and external fluids are formulated using the constitutive equations of an inviscid fluid. Displacement potentials are used to solve the equations of motion of the fiber and the fluids. The frequency equation of the coupled system consisting of the fiber and the internal and external fluid is developed under the assumption of no-slip boundary condition at the fluid–solid interface. The computed non-dimensional frequencies, non-dimensional wave number, phase velocity, attenuation and electro mechanical coupling are plotted in the form of dispersion curves for the lead zirconate titanate (PZT-4) ceramics.

Appendix

$$\begin{aligned} m_{1i}= & {} 2\overline{c} _{66} \left\{ {n\left( n-1 \right) -\overline{c} _{11} \left( \alpha _i a \right) ^{2}-\varsigma \left( \overline{c} _{13} a_i +\overline{e} _{31} b_i \right) } \right\} J_n \left( \alpha _i a \right) \nonumber \\&+\,2\overline{c} _{66} \left( \alpha _i a \right) J_{n+1} \left( \alpha _i a \right) ,\quad i=1,2,3 \end{aligned}$$

(30)

$$\begin{aligned} m_{14}= & {} 2\overline{c} _{66} n\left\{ {\left( n-1 \right) J_n \left( \alpha _4 a \right) -\left( \alpha _4 a \right) J_{n+1} \left( \alpha _4 a \right) } \right\} \end{aligned}$$

(31)

$$\begin{aligned} m_{15}= & {} 2\overline{c} _{66} n\left\{ {\left( n-1 \right) Y_n \left( \alpha _4 a \right) -\left( \alpha _4 a \right) Y_{n+1} \left( \alpha _4 a \right) } \right\} \end{aligned}$$

(32)

$$\begin{aligned} m_{1i}= & {} 2\overline{c} _{66} \left\{ {n\left( n-1 \right) -\overline{c} _{11} \left( \alpha _i a \right) ^{2}-\varsigma \left( \overline{c} _{13} a_i +\overline{e} _{31} b_i \right) } \right\} Y_n \left( \alpha _i a \right) \nonumber \\&+\,2\overline{c} _{66} \left( \alpha _i a \right) Y_{n+1} \left( \alpha _i a \right) ,\quad i=6,7,8 \end{aligned}$$

(33)

$$\begin{aligned} m_{19}= & {} \overline{\rho }_1 \Omega ^{2}J_n \left( \alpha _5 a \right) \end{aligned}$$

(34)

$$\begin{aligned} m_{10}= & {} \overline{\rho }_2 \Omega ^{2}H_n^{(2)} \left( \alpha _6 a \right) \end{aligned}$$

(35)

$$\begin{aligned} m_{2i}= & {} 2n\left\{ {\left( n-1 \right) J_n \left( \alpha _i a \right) +\left( \alpha _i a \right) J_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=1,2,3 \end{aligned}$$

(36)

$$\begin{aligned} m_{24}= & {} \left\{ {\left[ \left( \alpha _4 a \right) ^{2}-2n\left( n-1 \right) \right] J_n \left( \alpha _4 a \right) -2\left( \alpha _4 a \right) J_{n+1} \left( \alpha _4 a \right) } \right\} \end{aligned}$$

(37)

$$\begin{aligned} m_{25}= & {} \left\{ {\left[ \left( \alpha _4 a \right) ^{2}-2n\left( n-1 \right) \right] Y_n \left( \alpha _4 a \right) -2\left( \alpha _4 a \right) Y_{n+1} \left( \alpha _4 a \right) } \right\} \end{aligned}$$

(38)

$$\begin{aligned} m_{2i}= & {} 2n\left\{ {\left( n-1 \right) Y_n \left( \alpha _i a \right) +\left( \alpha _i a \right) Y_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=6,7,8 \end{aligned}$$

(39)

$$\begin{aligned} m_{29}= & {} 0, m_{210} =0 \end{aligned}$$

(40)

$$\begin{aligned} m_{3i}= & {} \left( \left( \varsigma +a_i \right) +\overline{e} _{15} b_i \right) \left\{ {nJ_n \left( \alpha _i a \right) -\left( \alpha _i a \right) J_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=1,2,3 \end{aligned}$$

(41)

$$\begin{aligned} m_{34}= & {} n\varsigma J_n \left( \alpha _4 a \right) \end{aligned}$$

(42)

$$\begin{aligned} m_{35}= & {} n\varsigma Y_n \left( \alpha _4 a \right) \end{aligned}$$

(43)

$$\begin{aligned} m_{3i}= & {} \left( \left( \varsigma +a_i \right) +\overline{e} _{15} b_i \right) \left\{ {nJ_n \left( \alpha _i a \right) -\left( \alpha _i a \right) J_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=6,7,8 \end{aligned}$$

(44)

$$\begin{aligned} m_{39}= & {} 0, m_{310} =0, \end{aligned}$$

(45)

$$\begin{aligned} m_{4i}= & {} b_i J_n \left( \alpha _i a \right) ,\quad i=1,2,3 \end{aligned}$$

(46)

$$\begin{aligned} m_{44}= & {} 0,m_{45} =0 \end{aligned}$$

(47)

$$\begin{aligned} m_{4i}= & {} b_i Y_n \left( \alpha _i a \right) ,\quad i=6,7,8 \end{aligned}$$

(48)

$$\begin{aligned} m_{49}= & {} 0,m_{410} =0 \end{aligned}$$

(49)

$$\begin{aligned} m_{5i}= & {} \left\{ {nJ_n \left( \alpha _i a \right) -\left( \alpha _i a \right) J_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=1,2,3 \end{aligned}$$

(50)

$$\begin{aligned} m_{54}= & {} nJ_n \left( \alpha _4 a \right) \end{aligned}$$

(51)

$$\begin{aligned} m_{55}= & {} nY_n \left( \alpha _4 a \right) \end{aligned}$$

(52)

$$\begin{aligned} m_{5i}= & {} \left\{ {nY_n \left( \alpha _i a \right) -\left( \alpha _i a \right) Y_{n+1} \left( \alpha _i a \right) } \right\} ,\quad i=6,7,8 \end{aligned}$$

(53)

$$\begin{aligned} m_{59}= & {} \Omega ^{2}\overline{\rho }_1 \left\{ {nJ_n \left( \alpha _5 a \right) -\left( \alpha _5 a \right) J_{n+1} \left( \alpha _5 a \right) } \right\} \end{aligned}$$

(54)

$$\begin{aligned} m_{510}= & {} \Omega ^{2}\overline{\rho }_2 \left\{ {nH_n^{(2)} \left( \alpha _6 a \right) -\left( \alpha _6 a \right) H_{n+1}^{(2)} \left( \alpha _6 a \right) } \right\} \end{aligned}$$

(55)