Non-isolated high step-up DC–DC converter adopting auxiliary capacitor and coupled inductor

Abstract

For grid-connected power system based on photovoltaic (PV) source and fuel cells, high step-up and high-efficiency DC–DC converters are needed, due to the bus voltage of the grid-connected inverter is much higher than the output voltage of PV and fuel cells. In this paper, a novel high step-up converter is proposed. An auxiliary capacitor is introduced into the boost converter, which serves as a voltage source. It is in series with the input voltage source with the same voltage polarities. Thus, the input voltage is increased equivalently and the voltage gain is increased accordingly. To reduce the voltage stresses of the switch and the diode, multiple output capacitors are introduced. The voltage of each output capacitor is degraded leading to the reduced voltage stress. To replenish energy for the multiple output capacitors, a coupled inductor is adopted. Based on this, high step-up converter adopting auxiliary capacitor and coupled inductor is derived. The operating principles and voltage gain of the proposed converters are analyzed in this paper. In the end, experiment results are given to verify the theoretical analysis.

1 Introduction

Since it takes centuries for the traditional fossil energy to be replenished, it will be exhausted with the growing demand for energy of human society. Thus the energy crisis is increasingly serious. Meanwhile, the excessive usage of the traditional fossil energy has polluted the environment and resulted in greenhouse effect on a global scale [1]. Therefore, it is becoming more and more important to optimize the energy consumption structure and to utilize clean and renewable energy. Solar energy and hydrogen energy are two promising renewable energy, and have extensive application prospect. As the utilization methods of the two new energy, photovoltaic (PV) and fuel cells power generations have been applied on a large scale [2,3,4,5,6,7], such as photovoltaic power station and electric vehicle.

In recent years, the grid-connected power generation based on PV source for residential application has become globally popular. Usually, an interface unit is necessary, as the output voltage of PV source is relatively too low for the line voltage. If the line voltage is 220 V, the input voltage needed by the grid-connected inverter would approach 380 V. But the output voltage of PV source generally varies from 25 to 45 V. To boost the output voltage of PV source, one possible solution to is to make series-connected PV arrays. But the total output power of PV arrays will be degraded due to module mismatch or partial shading [8]. Another promising solution is to utilize a high step-up DC–DC converter to match the low output voltage of PV source and high input voltage of the inverter. Then every PV source can realize the function of maximum power point tracking. For the isolated DC–DC converters, the voltage gain can be increased by adjusting the turns ratio of the transformer. But the energy stored in the leakage inductor is difficult to be transferred to the output. Thus, for the application without galvanic isolation requirement, non-isolated high step-up DC–DC converters is preferred.

The boost converter is wildly used for voltage step-up. However, its duty cycle would be too large when the input voltage is much smaller than the output. And for the actual power switch and diode, a certain delay between turn-on and turn-off state will probably result that the power switch is turned on before being cut off completely and the diode is cut off before conducting. Thus, the reliability is degraded. When the duty cycle approaches unity, a large pulse current will conduct through the diode in a short time, which leads to large the current stress of the diode and severe reverse recovery problem. This will greatly affect the efficiency and brings about a serious electromagnetic interference problem [9,10,11,12]. By cascading another boost converter, a high voltage gain can be easily obtained. But the additional switch makes the control scheme more complex. And it may cause instability issue for cascaded systems.

The impedance source networks are widely used in the inverters [13,14,15,16] to increase the voltage boost inversion ability. Likewise, the impedance source networks can also be used in high step-up DC–DC converters to achieve high voltage gain [17,18,19,20]. The converters can operate with a much smaller duty cycle, which improves the reliability. By combining quasi-Z-source network and transformer, the voltage stress of the switch is reduced. But most of the converters adopt multiple power switches, and have a relatively complex control scheme. In [21], a single power switch was adopted to simplify the control design. However, the voltage stress of the switch is as high as the output voltage, which brings large switching loss.

Another method of increasing the voltage gain is to introduce a coupled inductor [22,23,24,25,26,27,28]. However, the current through the coupled inductor is discontinuous. It goes against the lifetime of PV and fuel cell when the coupled inductor is placed on the input side. Especially for the low input voltage application, the input current is very large. Thus, the continuous input current is preferred.

To overcome the respective disadvantages of quasi-Z-source network and coupled inductor, some isolated high step-up DC–DC converters combining quasi-Z-source network and coupled inductor are proposed in [29, 30]. However, due to the isolation, the energy stored in the leakage inductor of the primary winding is difficult to be transferred to the output. In this paper, a non-isolated high step-up DC–DC converter with single switch based on quasi-Z-source network and coupled inductor is proposed. The input current is continuous and the voltage stress of the switch is low. Besides, the energy stored in the leakage inductor can be absorbed by the output capacitor, which is beneficial for the efficiency. And single switch is used to simplify the control scheme. The operating principle and parameter calculation are given in this paper, and an experiment is conducted to verify the theoretical analysis. The experiment results indicate that the proposed converters can achieve a higher efficiency.

2 Derivation of high step-up DC–DC converters adopting auxiliary capacitor and coupled inductor

2.1 High step-up converter adopting auxiliary capacitor

Figure 1 gives the basic boost converter, where V _g is the input voltage, L ₁ is the boost inductor, Q is the switch. To increase the voltage gain of boost converter, an auxiliary voltage source V _a can be introduced to the input terminal and the voltage polarity is the same as V _g, as shown in Fig. 2. When Q is turned on, V _g is in series with V _a to charge L ₁. When Q is turned off, V _g is in series with V _a and L ₁ to supply the load. And the output voltage is the sum of V _g, V _a and the voltage of L ₁. Obviously, the auxiliary voltage source increases the input voltage equivalently, and a high voltage gain is obtained.

Fig. 1

Basic boost converter

Fig. 2

Boost converter adopting auxiliary voltage source

The auxiliary voltage source V _a in Fig. 2 can be implemented with a capacitor C _a1, which is defined as the auxiliary capacitor. To replenish energy for the auxiliary capacitor C _a1, the inductor L ₂, and the diode D₁ is introduced as shown in Fig. 3a. Obviously, the larger the voltage of the auxiliary capacitor is, the higher the derived voltage gain will be. To obtain a higher voltage for C _a1, an additional auxiliary voltage source V _a can be added in the charging path of L ₂, as shown in Fig. 3b. When Q_a is turned on, V _a is in series with the input voltage source to charge L ₂. When Q_a is turned off, L ₂ replenishes energy for C _a1. If V _a is equal to the electric potential difference between nodes a and b, the electric potentials of the drain electrodes are identical and can be connected directly. In doing so, Q_a can be removed to simplify the structure. Likewise, the auxiliary voltage source V _a can be implemented by a capacitor C _a2, as shown in Fig. 4. As seen, L ₁ can be used to replenish energy for C _a2. This topology has been proposed and analyzed in [19], which is called Z-source DC–DC converter.

Fig. 3

Derivation process

Fig. 4

Transition structure (Z-source DC–DC converter)

As the voltages of C _a1 and V _g in Fig. 4 are constant, the voltage between nodes c and d is also constant and equals the voltage sum of C _a1 and V _g. Thus, a capacitor can be added between nodes C and D to serve as a new input source of the boost inductor L ₁. As the new capacitor charges L ₁ instead of the original one, the original auxiliary capacitor can be removed as shown in Fig. 5. This structure consisting of the capacitor and inductor is called quasi-Z-source network [13]. Its operating modes when the network is applied for DC–DC converter with single switch has not been analyzed in detail in [13].

Fig. 5

High step-up converter adopting auxiliary capacitor

Compared with the converter shown in Fig. 4, the voltage stress of C _a1 in Fig. 5 is larger. But the input current of the converter in Fig. 5 is continuous, which is beneficial for improving the lifetime of PV and fuel cell.

As shown in Fig. 5, the voltage stresses of the switch and the diode are both as high as the output voltage which leads to a large conduction resistor of the switch and severe reverse recovery problem of the diode. Thus, the conduction loss and the switching loss are both large.

2.2 High step-up converter adopting auxiliary capacitor and coupled inductor

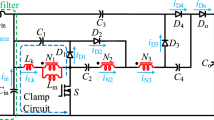
To reduce the voltage stress of the switch and the diode, referring to [31], multiple output capacitors can be used to supply the load, as shown in Fig. 6a. In doing so, the voltage of C _o1 is reduced, and the voltage stress of Q, D₁ and D_o1 are reduced as well. To replenish energy for C _o2, the inductor L ₁ in Fig. 5 is replaced by the coupled inductor L _cp, and the secondary winding of the coupled inductor is used to charge C _o2. In [31], the inductor of the boost converter is replaced by the coupled inductor, which leads to a discontinuous input current. On the contrary, the input current of the converter in Fig. 6b remains continuous, which is beneficial for the lifetime of PV and fuel cell.

Fig. 6

High step-up converter adopting auxiliary capacitor and coupled inductor

As shown in Fig. 6b, the voltage doubling rectifier circuit is adopted to further increase the voltage of C _o2 in order to reduce the voltage stresses of Q, D₁ and D_o1. As a result, a switch with a lower conduction resistor can be selected, and the conduction loss and switching loss can be reduced.

3 Analysis of high step-up DC–DC converters adopting auxiliary capacitor and coupled inductor

3.1 Operating principle of CCM

Considering the parasitic parameters of the coupled inductor, the equivalent circuit is given in Fig. 7. When the currents of L ₁ and the magnetizing inductor L _m are both continuous, there exist four operating modes and the key waveforms are shown in Fig. 8, where i _L1 is the current of L ₁, i _{Tr_s} is the current of the secondary winding of the coupled inductor, i _Lm and i _Llk are the currents of the magnetizing inductor and the leakage inductor of the coupled inductor respectively, i _D1 and i _Do1 are the currents of D₁ and D_o1. The operating modes are shown in Fig. 9.

Fig. 7

Equivalent circuit

Fig. 8

Key waveforms in CCM

Fig. 9

Operating modes in CCM

Mode 1 [t ₁ ~ t ₂]: When Q is turned on, V _g is in series with C _a2 to charge L ₁, and C _a1 charges the magnetizing inductor L _m. In this mode, the secondary winding of the coupled inductor charges C _o3 through D_o3.

Mode 2 [t ₂ ~ t ₃]: When Q is turned off, L ₁ and L _m charge C _a1 and C _a2 through D₁ respectively, and the input voltage source is in series with L ₁ and L _m to charge C _o1. In this mode, i _Lm is smaller than i _Llk, where i _Lm and i _Llk are the currents of the leakage inductor and the magnetizing inductor, respectively. Thus, the current direction of the secondary winding remains and the secondary winding still charges C _o3 through D_o3.

Mode 3 [t ₃ ~ T _s]: In this mode, i _Lm is larger than i _Llk, leading to the current direction of the secondary winding changed. And the secondary winding charges C _o2 in series with C _o3 through D_o2.

Mode 4 [T _s ~ t ₄]: In this mode, since i _Lm is larger than i _Llk, the secondary winding still charges C _o2 in series with C _o3 through D_o2.

3.2 Operating principle of DCM

When the current of L ₁ is discontinuous, the key waveforms and operating modes in DCM are shown as Figs. 10 and 11, respectively. There are five operating modes for DCM, in which Mode 1, Mode 2, and Mode 3 are the same with those in CCM. Here, only Mode 4 and Mode 5 are given in detail.

Fig. 10

Key waveforms in DCM

Fig. 11

Operating modes in DCM

Mode 4 [t ₄ ~ t ₅]: When i _L1 = i _Do1 − i _Llk, the current through D₁ decreases to zero and is cut off.

Mode 5 [t ₅ ~ T _s]: The currents through L ₁ and L _cp are zero, and C _o1 and C _o2 are in series to supply the load.

3.3 Voltage gain of CCM

As the leakage inductor is much smaller compared with the magnetizing inductor, the duration of Modes 2 and 4 in Fig. 8 are relatively short. Thus, the leakage inductor is neglected here, to simplify the analysis.

For steady state, according to the volt-second relationship of L ₁, we have:

$$\left( {V_{\text{g}} + V_{\text{Ca2}} } \right)DT_{\text{s}} = \left( {V_{\text{Ca1}} - V_{\text{g}} } \right)\left( {1 - D} \right)T_{\text{s}}$$

(1)

where V _g is the input voltage; D is the duty cycle of the switch; T _s is the switching period; V _a1 and V _a2 are the average voltages of C _a1 and C _a2, respectively.

Similarly, the volt-second relationship can be applied to L _m, then we have:

$$V_{\text{Ca1}} DT_{\text{s}} = V_{\text{Ca2}} \left( {1 - D} \right)T_{\text{s}}$$

(2)

According to (1) and (2), V _Ca1, V _Ca2 can be derived as:

$$V_{\text{Ca1}} = \frac{{\left( {1 - D} \right)V_{\text{g}} }}{1 - 2D}$$

(3)

$$V_{\text{Ca2}} = \frac{{DV_{\text{g}} }}{1 - 2D}$$

(4)

Referring to Fig. 9c, the voltage of C _o1 equals the voltage sum of C _a1 and C _a2.

$$V_{\text{Co1}} = V_{\text{Ca1}} + V_{\text{Ca2}} = \frac{{V_{\text{g}} }}{1 - 2D}$$

(5)

The voltage of C _o3 can be expressed as:

$$V_{\text{Co3}} = \frac{{N_{\text{sp}} \left( {1 - D} \right)V_{\text{g}} }}{1 - 2D}$$

(6)

where N _sp = N _s/N _p; N _s is the secondary winding turns; N _p is the primary winding turns.

The voltage of C _o2 is:

$$V_{\text{Co2}} = \frac{{N_{\text{sp}} DV_{\text{g}} }}{1 - 2D} + V_{\text{Co3}} = \frac{{N_{\text{sp}} V_{\text{g}} }}{1 - 2D}$$

(7)

Combing (5) and (7), the output voltage is:

$$V_{\text{o}} = V_{\text{Co1}} + V_{\text{Co2}} = \frac{{\left( {N_{\text{sp}} + 1} \right)V_{\text{g}} }}{1 - 2D}$$

(8)

Referring to Fig. 9a and c, the voltage stresses of Q, D₁ and D_o1 equal V _Co1, which are much smaller than the output voltage. And it is beneficial for improving the efficiency of the converter.

3.4 Voltage gain of DCM

To simplify the analysis, an assumption is made that the leakage inductor is neglected. Thus, Mode 2 and Mode 4 are neglected as well. Then only Mode 1, Mode 3 and Mode 5 need to be considered.

For steady state, applying the volt-second balance principle to L ₁ and L _m, we have:

$$(V_{\text{g}} + V_{{{\text{Ca}}2}} )DT_{\text{s}} = (V_{{{\text{Ca}}1}} - V_{g} )D_{\text{r}} T_{\text{s}}$$

(9)

$$V_{{{\text{Ca}}1}} DT_{\text{s}} = V_{{{\text{Ca}}2}} D_{\text{r}} T_{\text{s}}$$

(10)

where D _r T _s = t ₄ − t ₁.

Referring to Fig. 11, we have:

$$V_{\text{Co1}} = V_{\text{Ca1}} + V_{\text{Ca2}}$$

(11)

$$V_{\text{Co3}} = N_{\text{sp}} V_{\text{Ca1}}$$

(12)

$$V_{\text{Co2}} = V_{\text{Co3}} + N_{\text{sp}} V_{\text{Ca2}} = N_{\text{sp}} \left( {V_{\text{Ca1}} + V_{\text{Ca2}} } \right)$$

(13)

$$V_{\text{o}} = V_{\text{Co1}} + V_{\text{Co2}} = \left( {N_{\text{sp}} + 1} \right)V_{\text{Co1}}$$

(14)

Combining (9), (10) and (11), we can derive:

$$V_{\text{Ca1}} = \frac{{V_{\text{Co1}} + V_{\text{g}} }}{2}$$

(15)

$$V_{\text{Ca2}} = \frac{{V_{\text{Co1}} - V_{\text{g}} }}{2}$$

(16)

$$D_{\text{r}} = \frac{{V_{\text{Co1}} + V_{\text{g}} }}{{V_{\text{Co1}} - V_{\text{g}} }}D$$

(17)

Thus, the average current of L ₁ can be derived:

$$\begin{aligned} I_{{{\text{L1\_avg}}}} &= \frac{{V_{\text{g}} + V_{\text{Ca2}} }}{{L_{1} }}DT_{\text{s}} \frac{{D + D_{\text{r}} }}{2} \hfill \\ &= \frac{{V_{\text{g}} + V_{\text{Co1}} }}{{2L_{1} }}DT_{\text{s}} \frac{{V_{\text{Co1}} D}}{{V_{\text{Co1}} - V_{\text{g}} }} \hfill \\ \end{aligned}$$

(18)

If the power loss is neglected, the input average current is:

$$ I_{{{\text{L1\_avg}}}} = \frac{{V_{\text{o}} I_{\text{o}} }}{{V_{\text{g}} }} $$

(19)

Combining (14), (18) and (19), we can derive:

$$V_{\text{o}} = \left( {N_{\text{sp}} + 1} \right)V_{\text{g}} \frac{{ 2\left( {N_{\text{sp}} + 1} \right)I_{\text{o}} L_{1} + V_{\text{g}} D^{2} T_{\text{s}} }}{{ 2\left( {N_{\text{sp}} + 1} \right)I_{\text{o}} L_{1} - V_{\text{g}} D^{2} T_{\text{s}} }}$$

(20)

3.5 Comparison of high step-up converters

Table 1 gives the comparison of the proposed converter with state-of-art high step-up converters adopting coupled inductor [31,32,33,34,35]. Fig. 12 gives the curves of the voltage gains in Table 1, where N _sp is selected as 4. As seen, the proposed configuration in Fig. 6b has larger voltage gain than the other high step-up converters with the same duty cycle and reaches a considerable value although the duty cycle has not been close to 0.5. As shown in Fig. 6b, since the coupled inductor replaces L ₁ in Fig. 5 rather than L ₂ on the input side, the input current is continuous, which is beneficial for the lifetime of PV and fuel cell. As a consequence, the voltage stress of Q in the proposed converter is a little larger.

Table 1 Comparison of high step-up converters

Fig. 12

Comparison of voltage gain

4 Experiment verification

To verify the effectiveness of the proposed configurations in Figs. 5 and 6b, two prototypes are fabricated in the lab for contrast with the following specifications:

• 1) Input voltage V _g: 25–45 V _dc;

• 2) Output voltage V _o: 380 V _dc;

• 3) Switching frequency f _s: 100 kHz;

• 4) Maximal output power P _o: 300 W.

4.1 Parameter design

As the design progress of the converters in Figs. 5 and 6b are similar, the topology as shown in Fig. 6b is taken as an example to design the parameter.

Prior to the design procedure of capacitors and inductors, an assumption is made to simplify the analysis. Since the leakage inductor is relatively small compared with the magnetizing inductor, its influence can be ignored.

• 1) Design of coupled inductor L _cp

As shown in Table 1, the voltage stress of the switch decreases with the increase of N _sp. However, higher N _sp would increase the current stress of the switch. Thus, a trade-off design should be considered between the voltage stress and current stress of the switch. Here, we prefer the voltage stress lower than 100 V, and the resultant N _sp is 4.

Referring to Fig. 7, according to Kirchhoff’s circuit laws, we have:

$$\begin{aligned} i_{\text{L1}} = i_{\text{D1}} - i_{\text{Ca2}} = i_{\text{Ca1}} + i_{\text{Lm}} + i_{{{\text{Tr\_p}}}} - i_{\text{Ca2}} \hfill \\ = i_{\text{Ca1}} + i_{\text{Lm}} + N_{\text{sp}} i_{{{\text{Tr\_s}}}} - i_{\text{Ca2}} \hfill \\ = i_{\text{Ca1}} + i_{\text{Lm}} + N_{\text{sp}} i_{\text{Co3}} - i_{\text{Ca2}} \hfill \\ \end{aligned}$$

(21)

Based on the charge balance principle, the average currents of capacitors, I _{Ca1_avg}, I _{Ca2_avg}, and I _{Co3_avg} are zero. Thus, the average current of L ₁ equals the average current of L _m, and we have:

$$ I_{\text{L1}\_avg} = \, I_{\text{Lm}\_avg} = P_{\text{o}} /V_{\text{g}} $$

(22)

Setting the maximum current ripple of L _m is 30% of the maximum average current, then we have:

$$\Delta i_{\text{Lm}} = \frac{{V_{\text{Ca1}} DT_{\text{s}} }}{{L_{\text{m}} }} = \frac{{V_{\text{g}} \left( {1 - D} \right)DT_{\text{s}} }}{{\left( {1 - 2D} \right)L_{\text{m}} }} \le 30\% I_{{{\text{Lm\_avg}}}}$$

(23)

Substituting (22) into (23), yields:

$$L_{\text{m}} \ge \frac{{V_{\text{g}}^{2} \left( {1 - D} \right)DT_{\text{s}} }}{{30\% \left( {1 - 2D} \right)P_{\text{o}} }}$$

(24)

When V _g = 25 V, the right part of (24) reaches the maximum value. Then we have L _m = 50 μH.

• 2) Design of inductor L ₁

Likewise, the maximum current ripple of L ₁ can be expressed as:

$$\Delta i_{{{\text{L}}1}} = \frac{{\left( {V_{\text{g}} + V_{\text{Ca2}} } \right)DT_{\text{s}} }}{{L_{1} }} = \frac{{V_{\text{g}} \left( {1 - D} \right)DT_{\text{s}} }}{{\left( {1 - 2D} \right)L_{1} }} \le 30\% I_{{{\text{L1\_avg}}}}$$

(25)

Substituting (22) into (25), yields:

$$L_{ 1} \ge \frac{{V_{\text{g}}^{2} \left( {1 - D} \right)DT_{\text{s}} }}{{30\% \left( {1 - 2D} \right)P_{\text{o}} }}$$

(26)

When V _g = 25 V, the right part of (26) reaches the maximum value. Then we have L _m = 50 μH.

• 3) Design of auxiliary capacitor C _a1 and C _a2

The capacitor C _a1 and C _a2 can be derived according to the voltage ripple ΔV _Ca1 and ΔV _Ca2:

$$C_{\text{a1}} = \frac{{\int_{0}^{{DT_{\text{s}} }} {i_{\text{Ca1}} {\text{d}}t} }}{{\Delta V_{\text{Ca1}} }}$$

(27)

$$C_{\text{a2}} = \frac{{\int_{0}^{{DT_{\text{s}} }} {i_{\text{Ca2}} {\text{d}}t} }}{{\Delta V_{\text{Ca2}} }}$$

(28)

Referring to Fig. 9a, during the turn on interval, we have:

$$ i_{\text{Ca1}} = i_{\text{Lm}} + N_{\text{sp}} i_{\text{Tr\_s}} = i_{\text{Lm}} + N_{\text{sp}} i_{\text{Do3}} $$

(29)

$$i_{\text{Ca2}} = i_{\text{L1}}$$

(30)

Since the average currents of C _o2 and C _o3 are zero, the average current through D_o3 equals to I _o. Then we have:

$$\int_{0}^{{DT_{\text{s}} }} {i_{\text{Do3}} {\text{d}}t} = I_{\text{o}} T_{\text{s}}$$

(31)

Substituting (29), (30) and (31) into (27) and (28), yields:

$$ C_{\text{a1}} = \frac{{I_{{{\text{Lm\_avg}}}} DT_{\text{s}} }}{{\Delta V_{\text{Ca1}} }} + \frac{{N_{\text{sp}} I_{\text{o}} T_{\text{s}} }}{{\Delta V_{\text{Ca1}} }} = \frac{{P_{\text{o}} DT_{\text{s}} }}{{V_{\text{g}} \Delta V_{\text{Ca1}} }} + \frac{{N_{\text{sp}} I_{\text{o}} T_{\text{s}} }}{{\Delta V_{\text{Ca1}} }} $$

(32)

$$ C_{\text{a2}} = \frac{{I_{{{\text{L1\_avg}}}} DT_{\text{s}} }}{{\Delta V_{\text{Ca2}} }} = \frac{{P_{\text{o}} DT_{\text{s}} }}{{V_{\text{g}} \Delta V_{\text{Ca2}} }} $$

(33)

Setting the voltage ripple is lower than 5% of the maximum average voltage, we have C _a1 = 24 μF, C _a2 = 32 μF.

• 4) Design of output capacitor C _o1, C _o2 and C _o3

Likewise, the output capacitors can be derived as:

$$C_{\text{o1}} = \frac{{\int_{0}^{{DT_{\text{s}} }} {i_{\text{Co1}} {\text{d}}t} }}{{\Delta V_{\text{Co1}} }}$$

(34)

$$C_{\text{o2}} = \frac{{\int_{0}^{{DT_{\text{s}} }} {i_{\text{Co2}} {\text{d}}t} }}{{\Delta V_{\text{Co2}} }}$$

(35)

$$C_{\text{o3}} = \frac{{\int_{0}^{{DT_{\text{s}} }} {i_{\text{Co3}} {\text{d}}t} }}{{\Delta V_{\text{Co3}} }}$$

(36)

Referring to Fig. 9a, during the turn on interval, we have:

$$i_{\text{Co1}} = i_{\text{Co2}} = I_{\text{o}}$$

(37)

$$i_{\text{Co3}} = i_{\text{Do3}}$$

(38)

Substituting (31), (37) and (38) into (34), (35) and (36), yields:

$$C_{\text{o1}} = \frac{{I_{\text{o}} DT_{\text{s}} }}{{\Delta V_{\text{Co1}} }}$$

(39)

$$C_{\text{o2}} = \frac{{I_{\text{o}} DT_{\text{s}} }}{{\Delta V_{\text{Co2}} }}$$

(40)

$$C_{\text{o3}} = \frac{{I_{\text{o}} T_{\text{s}} }}{{\Delta V_{\text{Co3}} }}$$

(41)

Since C _o1, C _o2 is placed on the output side, the voltage ripples of C _o1 and C _o2 are limited to a smaller scale. If the voltage ripples of C _o1 and C _o2 are limited to 1% of the respective maximum average voltage, and the voltage ripple of C _o3 is limited to 5% of the maximum average voltage, we have C _o1 = 4 μF, C _o2 = 3 μF, C _o3 = 3 μF.

• 5) Switch Q

The voltage stress of the switch is V _o/(N _sp + 1). When Q is turned on, the current of the switch i _Q can be expressed as:

$$\begin{aligned} i_{\text{Q}} = i_{\text{L1}} + i_{\text{Lm}} + N_{\text{sp}} i_{\text{Do3}} \hfill \\ = \frac{{2V_{\text{g}} \left( {1 - D} \right)t}}{{\left( {1 - 2D} \right)L_{1} }} + \frac{{2P_{\text{o}} }}{{V_{\text{g}} }} - \frac{{V_{\text{g}} \left( {1 - D} \right)DT_{\text{s}} }}{{\left( {1 - 2D} \right)L_{1} }} + \frac{{2N_{\text{sp}} I_{\text{o}} t}}{{DDT_{\text{s}} }} \hfill \\ \end{aligned}$$

(42)

When V _g = 25 V, the RMS current of Q reaches its maximum value 19.8 A.

• 6) Diode D₁, D_o1, D_o2 and D_o3

The voltage stresses of D₁ and D_o1 are V _o/(N _sp + 1), and the voltage stresses of D_o2 and D_o3 are N _sp V _o/(N _sp + 1).

The currents through D_o2 and D_o3 can be expressed as:

$$ i_{\text{Do2}} = \left\{ \begin{aligned} \begin{array}{*{20}c} 0 & {\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;t \in [0,DT_{\text{s}} ]} \\ \end{array} \hfill \\ \begin{array}{*{20}c} {\frac{{2I_{\text{o}} \left( {t - DT_{\text{s}} } \right)}}{{\left( {1 - D} \right)\left( {1 - D} \right)T_{\text{s}} }}} & {\;\;\;\;\;\;t \in [DT_{\text{s}} ,T_{\text{s}} ]} \\ \end{array} \hfill \\ \end{aligned} \right. $$

(43)

$$i_{\text{Do3}} = \left\{ \begin{aligned} \begin{array}{*{20}c} {\frac{{2I_{\text{o}} t}}{{DDT_{\text{s}} }}} & {\;\;\;\;\;t \in [0,DT_{\text{s}} ]} \\ \end{array} \hfill \\ \begin{array}{*{20}c} 0 & {\;\;\;\;\;\;\;\;\;\;\;\;t \in [DT_{\text{s}} ,T_{\text{s}} ]} \\ \end{array} \hfill \\ \end{aligned} \right.$$

(44)

According to Fig. 9c, we have:

$$v_{\text{Ca1}} + v_{\text{Ca2}} = v_{\text{Co1}}$$

(45)

$$\frac{{{\text{d}}v_{\text{Ca1}} }}{{{\text{d}}t}} + \frac{{{\text{d}}v_{\text{Ca2}} }}{{{\text{d}}t}} = \frac{{{\text{d}}v_{\text{Co1}} }}{{{\text{d}}t}}$$

(46)

$$\left\{ \begin{aligned} C_{\text{a1}} \frac{{{\text{d}}v_{\text{Ca1}} }}{{{\text{d}}t}} + C_{\text{o1}} \frac{{{\text{d}}v_{\text{Co1}} }}{{{\text{d}}t}} + I_{\text{o}} = i_{\text{L1}} \hfill \\ C_{\text{a2}} \frac{{{\text{d}}v_{\text{Ca2}} }}{{{\text{d}}t}} + C_{\text{o1}} \frac{{{\text{d}}v_{\text{Co1}} }}{{{\text{d}}t}} + N_{\text{sp}} i_{\text{Do2}} + I_{\text{o}} = i_{\text{Lm}} \hfill \\ \end{aligned} \right.$$

(47)

where v _Ca1, v _Ca2 and v _Co1 are the instantaneous voltages of C _a1, C _a2 and C _o1, respectively.

Combining (46) and (47), the currents through D₁ and D_o1 can be expressed as:

$$\begin{aligned} i_{\text{D1}} = i_{\text{L1}} + C_{\text{a2}} \frac{{{\text{d}}v_{\text{Ca2}} }}{{{\text{d}}t}} = \frac{{\left( {C_{\text{a1}} C_{\text{a2}} + C_{\text{a2}} C_{\text{o1}} } \right)\left( {i_{\text{Lm}} - N_{\text{sp}} i_{\text{Do2}} } \right)}}{{C_{\text{a1}} C_{\text{a2}} + C_{\text{a1}} C_{\text{o1}} + C_{\text{a2}} C_{\text{o1}} }} \hfill \\ \frac{{ + \left( {C_{\text{a1}} C_{\text{a2}} + C_{\text{a1}} C_{\text{o1}} } \right)i_{\text{L1}} - C_{\text{a1}} C_{\text{a2}} I_{\text{o}} }}{{C_{\text{a1}} C_{\text{a2}} + C_{\text{a1}} C_{\text{o1}} + C_{\text{a2}} C_{\text{o1}} }} \hfill \\ \end{aligned}$$

(48)

$$\begin{aligned} i_{\text{Do1}} = I_{\text{o}} + C_{\text{o1}} \frac{{{\text{d}}v_{\text{Co1}} }}{{{\text{d}}t}} = \hfill \\ \frac{{C_{\text{a1}} C_{\text{a2}} I_{\text{o}} + C_{\text{a1}} C_{\text{o1}} \left( {i_{\text{Lm}} - N_{\text{sp}} i_{\text{Do2}} } \right) + C_{\text{a2}} C_{\text{o1}} i_{\text{Lb}} }}{{C_{\text{a1}} C_{\text{a2}} + C_{\text{a1}} C_{\text{o1}} + C_{\text{a2}} C_{\text{o1}} }} \hfill \\ \end{aligned}$$

(49)

According to (43), (44), (48) and (49), the current stresses of D₁, D_o1, D_o2 and D_o3 can be calculated.

4.2 Experiment results

The main components used in the prototypes are listed in the following.

High step-up converter adopting auxiliary capacitor:

• 1) Q: IPW65R045C7;

• 2) D₁: IDW20G65C5, D_o: C3D10060A;

• 3) L ₁: 263 μH, L ₂: 263 μH;

• 4) C _a1: 5.6 μF, C _a2: 6.8 μF, C _f: 220 μF.

High step-up converter adopting auxiliary capacitor and coupled inductor:

• 1) Q: IPP110N20N3G;

• 2) D₁: STPS20120C, D_o1: STPS10120C;

• 3) D_o2: C3D10060A, D_o3: C3D10060A;

• 4) L ₁: 50 μH, L _m: 50 μH, N _sp: 4;

• 5) C _a1: 24 μF, C _a2: 32 μF, C _f: 220 μF;

• 6) C _o1: 4 μF, C _o2: 3 μF, C _o3: 3 μF.

where C _f is used to realize the power decoupling if high step-up converter is cascaded with an inverter. And it is parallel with C _o1 and C _o2 in the high step-up converter adopting auxiliary capacitor and coupled inductor.

The experimental waveforms of high step-up converter based on quasi-Z-source network under different input voltages at full load are shown in Figs. 13 and 14, where v _ds is the drain-source voltage of the switch. As seen in Fig. 14, D₁ and D_o conducts simultaneously. It can be explained as follows. Due to the forward recovery phenomenon of D₁, the voltage sum of C _a1, C _a2 and the voltage drop of D₁ is larger than the output filter capacitor when the switch is turned off. The voltage difference will drop on ESR of the output filter capacitor and cause a large current through D_o. As shown in Fig. 14, at the instant of turning off the switch, v _ds is slightly large, and there is a large current through D_o. Thus, the current supplied by L ₁ to replenish energy for C _a2 is small, and i _D1 is small. With the voltage drop of D₁ reducing gradually, the difference between v _ds and the voltage of C _f decreases, resulting that i _Do decreases and i _D1 increases. As C _a1 and C _a2 are much smaller than C _f, the voltage ripples of C _a1 and C _a2 are larger. Thus, with the voltages of the auxiliary capacitors increasing, the difference between v _ds and the voltage of C _f increases, leading to i _Do increasing and i _D1 decreasing.

Fig. 13

Experiment waveforms of high step-up converter adopting auxiliary capacitor

Fig. 14

Experiment waveforms of high step-up converter adopting auxiliary capacitor (V _g = 45 V, P _o = 300 W)

The experimental waveforms of high step-up converter adopting auxiliary capacitor and coupled inductor under different input voltages at full load are shown in Figs. 15 and 16 where i _{cp_p} is the current of the primary winding, i _Do3 is the current of D_o3, v _D1 and v _Do1 are the voltages of D₁ and D_o1. According to the theoretical analysis, i _Do3 should rise up from zero when the switch is turned on. However, in the real case, there exist reverse recovery problem for D_o2 at the instant of turning on the switch. During the time interval of the reverse recovery, the voltage of the secondary winding is clamped by C _o2 and C _o3. The voltage is reflected to the primary side by electro-magnetic induction and in series with C _a1 to charge the leakage inductor which leads to the current of the leakage inductor rising up rapidly. After the reverse recovery of D_o2 is over, the current of the leakage inductor is larger than that of the magnetizing inductor. Thus, i _Do3 rises up from a positive value. The voltage stresses of the switch, D₁ and D_o1 shown in Fig. 16 are much smaller than the output voltage which will reduce the power loss and improve the efficiency.

Fig. 15

Experiment waveforms of high step-up converter adopting auxiliary capacitor and coupled inductor

Fig. 16

Voltage stresses of the switch D₁ and D_o1

The dynamic response of high step-up converter adopting auxiliary capacitor and coupled inductor is shown in Fig. 17. The output voltage is regulated in closed loop with single voltage compensator. The experimental result shows that the dynamic performance of the proposed converter is good. The startup waveform (V _g = 36 V, P _o = 300 W) is shown in Fig. 18, where v _o is the output voltage, and i _L1 is the current of L ₁. As seen, the overshoot of the output voltage is less than 30 V, which is 7.9% of the steady-state value.

Fig. 17

Dynamic response of high step-up converter adopting auxiliary capacitor and coupled inductor when the load step varies between the full load and the half load

Fig. 18

Startup waveform

The comparison experiments of the basic boost converter and the cascaded boost converter are also performed. To avoid the instability issue in the cascaded boost converter, the first stage is under open loop control with constant voltage gain of 4. The second stage of the cascaded boost converter regulate the output voltage. The efficiency curves of high step-up converter adopting auxiliary capacitor, high step-up converter adopting auxiliary capacitor and coupled inductor, the basic boost converter, and the cascaded boost converter are shown in Fig. 19. As the conducting period of D_o in the basic boost converter is extremely short, the current stress is large and the reverse recovery problem is severe which leads to a large switching loss of the switch. Thus, the efficiency of the basic boost converter is lower than that of high step-up converter adopting auxiliary capacitor at heavy load as shown in Fig. 19. When the coupled inductor is adopted, the efficiency is further improved. Due to the voltage stresses of the switch, D₁ and D_o1 decreasing, the switching loss can be reduced effectively and the efficiency is superior to that of the cascaded boost converter over a wide load range.

Fig. 19

Efficiency comparison when V _g = 45 V

According to the calculation of the power loss in Appendix A, the estimated efficiency is shown as Fig. 20, which matches the measured efficiency. And the power loss ratios are given in Fig. 21, where r _{Q_sw} = (P _{Q_on} + P _{Q_off})/P _in, r _{Q_con} = P _{Q_con}/P _in, r _fe = (P _{L1_fe} + P _{Lcp_fe})/P _in, r _cu = (P _{L1_cu} + P _{Lcp_cu})/P _in, r _D = (P _D1 + P _Do1 + P _Do2 + P _Do3)/P _in. As seen, r _{Q_sw} and r _fe decrease with the increase of the output power, while r _{Q_con} and r _cu increase with the increase of the output power. Thus, the efficiency increases at light load and decreases at heavy load. And the present of the maximum efficiency depends on the parasitic parameter of the components.

Fig. 20

Estimated efficiency and measured efficiency (V _g = 45 V)

Fig. 21

Power loss ratio (V _g = 45 V)

The experiment results indicate that the proposed converters can achieve a higher efficiency and good dynamic performance as well.

5 Conclusion

A novel high step-up DC–DC converter adopting auxiliary capacitor and coupled inductor is proposed in this paper. The input current is continuous, and the voltage stress of the power switch is low, which reduces the switching loss. Thus, the efficiency of the converter is improved. The operating mode of the proposed topology is analyzed and an experiment is conducted. The results indicate that the converters proposed in this paper can operate steadily and the performance is good.

Appendix A

The main power losses include the power loss of the switch, the diodes, the inductor and the coupled inductor. According to the parameter design in Section 4, the current stresses of the switch, the diodes, the inductor and the coupled inductor are given, and then the power loss of each component can be calculated as follows.

• 1) Power loss of the switch Q

The power loss of the switch mainly includes the switching loss and the conduction loss. The switching loss can be derived as:

$$P_{{{\text{Q\_on}}}} = \frac{{f_{\text{s}} R_{\text{g}} V_{\text{ds}} i_{{{\text{Q\_on}}}} }}{2}\left[ {\frac{{C_{\text{iss}} \left( {V_{\text{miller}} - V_{\text{th}} } \right)}}{{V_{\text{gate}} - 0.5\left( {V_{\text{miller}} + V_{\text{th}} } \right)}} + \frac{{C_{\text{rss}} V_{\text{ds}} }}{{V_{\text{gate}} - V_{\text{miller}} }}} \right]$$

(A1)

$$ P_{{{\text{Q\_off}}}} = \frac{{f_{\text{s}} R_{\text{g}} V_{\text{ds}} i_{{{\text{Q\_off}}}} }}{2}\left[ {\frac{{2C_{\text{iss}} \left( {V_{\text{miller}} - V_{\text{th}} } \right)}}{{V_{\text{miller}} + V_{\text{th}} }} + C_{\text{rss}} \frac{{V_{\text{ds}} }}{{V_{\text{miller}} }}} \right] $$

(A2)

where f _s is the switching frequency; R _g is the driving resistor; V _ds is the voltage stress of the switch; i _{Q_on} is the current through the switch when Q is turned on; V _gate is the driving voltage; C _iss, C _rss, V _miller, V _th are the parasitic parameters of the switch, referring to the datasheet of the switch.

The conduction loss can be derived as:

$$ P_{{{\text{Q\_con}}}} = I_{{{\text{Q\_rms}}}}^{2} R_{\text{dson}} $$

(A3)

where I _{Q_rms} is RMS current through the switch; R _dson is the on-state resistor of the switch.

• 2) Power loss of the diodes D₁, D_o1, D_o2, D_o3

The voltage drop of the diode can be viewed as a constant value. Then the power loss of D₁, D_o1, D_o2, D_o3 can be derived as:

$$ \left\{ \begin{aligned} P_{\text{D1}} = V_{{{\text{f\_D1}}}} I_{{{\text{D1\_avg}}}} \hfill \\ P_{\text{Do1}} = V_{{{\text{f\_Do1}}}} I_{{{\text{Do1\_avg}}}} \hfill \\ P_{\text{Do2}} = V_{{{\text{f\_Do2}}}} I_{{{\text{Do2\_avg}}}} \hfill \\ P_{\text{Do3}} = V_{{{\text{f\_Do3}}}} I_{{{\text{Do3\_avg}}}} \hfill \\ \end{aligned} \right. $$

(A4)

where V _{f_D1}, V _{f_Do1}, V _{f_Do2}, V _{f_Do3} are the voltage drops of D₁, D_o1, D_o2, D_o3, which are listed in the datasheet; I _{D1_avg}, I _{Do1_avg}, I _{Do2_avg}, I _{Do3_avg} are the average currents through D₁, D_o1, D_o2, D_o3, respectively.

• 3) Power loss of the inductor L ₁, and coupled inductor L _cp

The power losses of L ₁ and L _cp include the copper loss and the core loss. The copper loss of L ₁, L _cp can be derived as:

$$ \left\{ \begin{aligned} P_{{{\text{L1\_cu}}}} = I_{{{\text{L1\_dc}}}}^{ 2} R_{{{\text{L1\_dc}}}} + I_{{{\text{L1\_ac}}}}^{ 2} R_{{{\text{L1\_ac}}}} \hfill \\ P_{{{\text{Lcp\_cu}}}} = I_{{{\text{Lcp\_p\_dc}}}}^{ 2} R_{{{\text{Lcp\_p\_dc}}}} + I_{{{\text{Lcp\_s\_dc}}}}^{ 2} R_{{{\text{Lcp\_s\_dc}}}} \hfill \\ + I_{{{\text{Lcp\_p\_ac}}}}^{ 2} R_{{{\text{Lcp\_p\_ac}}}} + I_{{{\text{Lcp\_s\_ac}}}}^{ 2} R_{{{\text{Lcp\_s\_ac}}}} \hfill \\ \end{aligned} \right. $$

(A5)

where I _{L1_dc} and I _{L1_ac} are the dc current and ac RMS current of L ₁, respectively; I _{Lcp_p_dc} and I _{Lcp_p_ac} are the dc current and ac RMS current of the primary winding, respectively; I _{Lcp_s_dc} and I _{Lcp_s_ac} are the dc current and ac RMS current of the secondary winding, respectively; R _{L1_dc}, R _{L1_ac}, R _{Lcp_p_dc}, R _{Lcp_s_dc}, R _{Lcp_p_ac} and R _{Lcp_s_ac} can be measured by the impedance analyzer.

The core loss of L ₁, L _cp can be derived as:

$$ \left\{ \begin{aligned} P_{{{\text{L1\_fe}}}} = kf_{\text{s}}^{m} \Delta B_{\text{L1}}^{n} V_{\text{e}} \hfill \\ P_{{{\text{Lcp\_fe}}}} = kf_{\text{s}}^{m} \Delta B_{\text{Lcp}}^{n} V_{\text{e}} \hfill \\ \end{aligned} \right. $$

(A6)

$$ \left\{ \begin{aligned} \Delta B_{\text{L1}} = \frac{{\left( {V_{\text{g}} + V_{\text{Ca2}} } \right)DT_{\text{s}} }}{{N_{\text{L1}} A_{\text{e}} }} \hfill \\ \Delta B_{\text{Lcp}} = \frac{{V_{\text{Ca1}} DT_{\text{s}} }}{{N_{{{\text{Lcp\_p}}}} A_{\text{e}} }} \hfill \\ \end{aligned} \right. $$

(A7)

where V _e is the core volume; A _e is the effective area of the core; k, m, n can refer to the datasheet of the core; N _L1 is the turns of L ₁; N _{Lcp_p} is the turns of the primary winding.