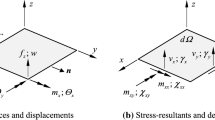
Abstract
The paper presents an explicit matrix algorithm to solve the problem of an elastic wedge with three loaded surfaces. The algorithm makes use of a recently published concept of transformation matrix, by which the original surface loads are converted to equivalent loads in half-space. The three loaded edges are considered simultaneously. The developed algorithm is used to study the effects of two free edges of a steel block and tapered rollers with different contact angles. The two load-free edges can substantially increase deformation if the two edges are close in distance. The results of the tapered roller simulation show that deformation is considerably sensitive to the contact angle of the tapered roller. The largest deformation appears at the big end of the roller. Furthermore, empirical formulae for correction factors for the calculation of block or quarter-space deformation based on half-space solutions are summarized.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Zhang C, Gu L, Mao Y Z, Wang L Q. Modeling the factional torque of a dry-lubricated tapered roller bearing considering the roller skewing. Friction (2018) doi: https://doi.org/10.1007/s40544-018-0232-8 (in Press)
Koshy M, Gohar R. Pressure distribution on radially loaded rollers. In Proceedings of the 18th International Machine Tool Design and Research, 1977: 595–604.
Johns P M, Gohar R. Roller bearings under radial and eccentric loads. Tribol Int14(3): 131–136 (1981)
Rahnejat H, Gohar R. Design of profiled taper roller bearings. Tribol Int12(6): 269–75 (1979)
Hartnett M J. The analysis of contact stresses in rolling element bearings. J Lubr Technol101(1): 105–109 (1979)
Kannel J W. Comparison between predicted and measured axial pressure distribution between cylinders. J Lubr Technol96(3): 508–514 (1974)
Zantopulos H. The effect of misalignment on the fatigue life of tapered roller bearings. J Lubr Technol94(2): 181–186 (1972).
Stejskal E O, Cameron A. Optical interferometry study of film formation in lubrication of sliding and/or rolling contacts. NASA Lewis Research Center, 1972.
Wymer D G. Elastohydrodynamic lubrication of a rolling line contact. Ph.D Thesis. London (England): University of London, 1972.
Wymer D G, Cameron P A. Elastohydrodynamic lubrication of a line contact. Proc Inst Mech Eng188(1): 221–238 (1974)
Wang Z J, Shen X J, Chen X Y, Tao D H, Shi L, Liu S B. Experimental investigation of EHD grease lubrication in finite line contacts. Friction (2018) doi: https://doi.org/10.1007/s40544-018-0208-8 (in Press)
Bahadoran H, Gohar R. Research note: End closure in elastohydrodynamic line contact. J Mech Eng Sci16(4): 276–278 (1974)
Mostofi A, Gohar R. Elastohydrodynamic lubrication of finite line contacts. J Lubr Technol105(4): 598–604 (1983)
Park T J, Kim K W. Elastohydrodynamic lubrication of a finite line contact. Wear223(1–2): 102–109 (1998)
Kushwaha M, Rahnejat H, Gohar R. Aligned and misaligned contacts of rollers to races in elastohydrodynamic finite line conjunctions. Proc Inst Mech Eng Part C: J Mech Eng Sci216(11): 1051–1070 (2002)
Kushwaha M, Rahnejat H. Transient concentrated finite line roller-to-race contact under combined entraining, tilting and squeeze film motions. J Phys D: Appl Phys37(14): 2018–2034 (2004)
Liu X L, Yang P R. Analysis of the thermal elastohydrodynamic lubrication of a finite line contact. Tribol Int35(3): 137–144 (2002)
Liu X L, Yang P R. Numerical analysis of the oil-supply condition in isothermal elastohydrodynamic lubrication of finite line contacts. Tribol Lett38: 115–124 (2010)
Sun H Y, Chen X Y. Thermal EHL analysis of cylindrical roller under heavy load. In IUTAM Symposium on Elastohydrodynamics and Micro-Elastohydrodynamics. Snidle R W, Evans H P, Eds. Dordrecht: Springer, 2006: 107–120.
Zhu D, Wang J X, Ren N, Wang Q J. Mixed elastohy-drodynamic lubrication in finite roller contacts involving realistic geometry and surface roughness. J Tribol134(1): 011504 (2012)
He T, Wang J X, Wang Z J, Zhu D. Simulation of plasto-elastohydrodynamic lubrication in line contacts of infinite and finite length. J Tribol137(4): 041505 (2015)
Boussinesq J V. Application Des Potentiels a L’etude de L’equilibre et du Mouvement Des Solides Elastiques. Gautier-Villars (Paris): Impr. L. Danel, 1885.
Love A E H. The stress produced in a semi-infinite solid by pressure on part of the boundary. Philos Trans Roy Soc A, Math Phys Eng Sci228(659–669): 377–420 (1929)
Najjari M, Guilbault R. Edge contact effect on thermal elastohydrodynamic lubrication of finite contact lines. Tribol Int71: 50–61 (2014)
Guilbault R. A fast correction for elastic quarter-space applied to 3D modeling of edge contact problems. J Tribol133(3): 031402 (2011)
Wang W, Guo L, Wong P L, Zhang Z M. Surface normal deformation in elastic quarter-space. Tribol Int114: 358–364 (2017)
Guo L, Wang W, Zhang Z M, Wong P L. Study on the free edge effect on finite line contact elastohydrodynamic lubrication. Tribol Int116: 482–490 (2017)
Zhang Z M, Wang W, Wong P L. An explicit solution for the elastic quarter-space problem in matrix formulation. Int J Solids Struct50(6): 976–980 (2013)
Zhang H B, Wang W Z, Zhang S G, Zhao Z Q. Elastohy-drodynamic lubrication analysis of finite line contact problem with consideration of two free end surfaces. J Tribol139(3): 031501 (2017)
Hetényi M. A general solution for the elastic quarter space. J Appl Mech37(1): 70–76 (1970)
Zhang H B, Wang W Z, Zhang S G, Zhao Z Q. Modeling of finite-length line contact problem with consideration of two free-end surfaces. J Tribol138(2): 021402 (2016)
Guo L, Zhang Z M, Wang W, Wong P L. An explicit matrix algorithm for solving three-dimensional elastic wedge under surface loads Int J Solids Struct128: 231–242 (2017)
Ahmadi N, Keer L M, Mura T, Vithoontien V. The interior stress field caused by tangential loading of a rectangular patch on an elastic half space. J Tribol109(4): 627–629 (1987)
Hanson M T, Keer L M. A simplified analysis for an elastic quarter-space. Quart J Mech Appl Math43(4): 561–587 (1990)
Acknowledgements
This research is completely supported by the Research Grants Council of Hong Kong (Project No. CityU11213914).
Author information
Authors and Affiliations
Corresponding author
Additional information
Liang GUO. He received his Ph.D. degree from the Department of Mechanical and Biomedical Engineering, City University of Hong Kong in 2016. He is currently a postdoctoral fellow in the same department. His research interests include interfacial effect in hydrodynamic lubrication and contact mechanics.
Patrick WONG. He obtained his Ph.D. degree from the University of Hong Kong in 1990, and his BSc degree from City University, London in 1983. Dr. Wong started his academic career in the City University of Hong Kong since 1990. He is currently an associate professor in the Department of Mechanical Engineering in the City University of Hong Kong. His research interests include rolling element bearings, interfacial phenomena, and lubrication.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made.
The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.
To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Guo, L., Zhang, Z.M., Wang, W. et al. An explicit solution to a three-dimensional wedge problem considering two edges effect. Friction 8, 370–383 (2020). https://doi.org/10.1007/s40544-019-0265-7
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40544-019-0265-7