PROCYON Mission Reanalysis: Low-Thrust Asteroid Flyby Trajectory Design leveraging Convex Programming

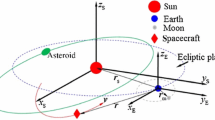
Abstract

PROCYON is the world’s first deep-space micro spacecraft launched on December 3, 2014, as a secondary payload on the launch of JAXA’s Hayabusa2 spacecraft. The mission objectives include the high-speed flyby observation of a near-Earth asteroid, which is enabled by utilizing miniature electric propulsion and an Earth swing-by. Through the PROCYON mission design, we encountered a limitation with the zero-radius sphere-of-influence patched-conics approach used in typical mission design. The patched-conics approach could not find a trajectory toward 2000 DP107, the nominal target asteroid, which is attainable only by a distant Earth swing-by under multi-body dynamics. This limitation mainly comes from the low orbital control capabilities of small spacecraft systems. To overcome this difficulty, we propose a preliminary mission design method that quickly calculates low-thrust and gravity assist trajectories using a convex programming approach. The method enables us to search for reachable asteroids extensively under multi-body dynamical systems. We reanalyze the PROCYON mission by the proposed mission design procedure and broadly perform trade studies regarding candidate asteroids in terms of transfer costs and operational requirements. The numerical result demonstrates that the proposed method efficiently finds the nominal target that we could not find by the patched-conics approach.

Appendices

Appendix A: Calculation of Partial Derivatives

The partial derivative $\frac {\partial \mathrm {F}_{k}}{\partial \mathrm {x}_{k}}$, or the STM, is numerically calculated by propagating the following ordinary differential equation between [t_k,t_k+ 1]. Taking the partial derivatives of Eq. 1 with respect to x_k yields

$$ \frac{\partial}{\partial \mathrm{x}_{k}}\frac{d\mathrm{x}}{dt} = \frac{\partial \mathrm{f}}{\partial \mathrm{x}_{k}} = \frac{\partial \mathrm{f}}{\partial \mathrm{x}}\frac{\partial \mathrm{x}}{\partial \mathrm{x}_{k}} + \frac{\partial \mathrm{f}}{\partial \mathrm{u}}\frac{\partial \mathrm{u}}{\partial \mathrm{x}_{k}} $$

(A.1)

where

$$ \frac{\partial \mathrm{x}}{\partial \mathrm{x}_{k}} = \mathrm{{\varPhi}}(t;t_{k}) \textrm{ \ and \ } \frac{\partial \mathrm{u}}{\partial \mathrm{x}_{k}} = \mathrm{O}_{3\times 6}. $$

Note that u = u_k (fixed) between [t_k,t_k+ 1). Changing the order of the differentiation yields

$$ \begin{array}{@{}rcl@{}} \frac{d}{dt}\mathrm{{\varPhi}}(t;t_{k}) &= \frac{\partial \mathrm{f}}{\partial \mathrm{x}}\cdot \mathrm{{\varPhi}}(t;t_{k}) \end{array} $$

(A.2)

$$ \begin{array}{@{}rcl@{}} &= \begin{bmatrix} \mathrm{O}_{3\times 3} & \mathrm{I}_{3\times 3}\\ \frac{\partial\mathrm{a}}{\partial \mathrm{r}} & \frac{\partial\mathrm{a}}{\partial \mathrm{v}} \end{bmatrix}\cdot \mathrm{{\varPhi}}(t;t_{k}) \end{array} $$

(A.3)

where both partial derivatives $\frac {\partial \mathrm {a}}{\partial \mathrm {r}}$ and $\frac {\partial \mathrm {a}}{\partial \mathrm {v}}$ are evaluated at (t,x,u). The initial value of this ordinary differential equation is

$$ \mathrm{{\varPhi}}(t_{k};t_{k})=\mathrm{I}_{6\times 6} $$

(A.4)

by the definition of the STM. The partial derivative $\frac {\partial \mathrm {F}_{k}}{\partial \mathrm {u}_{k}}$ is also computed by replacing x_k by u_k. This paper approximates $\frac {\partial \mathrm {F}_{k}}{\partial \mathrm {u}_{k}}$ by $\frac {\partial \mathrm {F}_{k}}{\partial \mathrm {v}_{k}}{\varDelta } t$ and simplifies the computation of the partial derivatives.

Appendix B: Maximum and Minimum Reachable Solar Distance

Suppose that the Earth is orbiting in a circular motion. Then the velocity of the Earth is

$$ v_{\oplus} = \sqrt{\frac{GM_{\odot}}{r_{\oplus}}}. $$

(B.1)

Let us calculate the reachable maximum solar distance, that is, the aphelion radius r_a. From the assumption, the spacecraft has the Earth swing-by at the perihelion. The spacecraft velocity at the Earth swing-by is

$$ v_{\text{sc}} = v_{\oplus} + v_{\infty}. $$

(B.2)

From the vis-viva equation and the conservation of the angular momentum

$$ \begin{array}{@{}rcl@{}} (v_{\oplus}+v_{\infty})^{2} - \frac{2GM_{\odot}}{r_{\oplus}} &=& v_{\mathrm{a}}^{2} - \frac{2GM_{\odot}}{r_{\mathrm{a}}} \end{array} $$

(B.3)

$$ \begin{array}{@{}rcl@{}} r_{\oplus}(v_{\oplus}+v_{\infty}) &=& r_{\mathrm{a}} v_{\mathrm{a}}, \end{array} $$

(B.4)

we obtain

$$ v_{\mathrm{a}} = \sqrt{\frac{GM_{\odot}}{r_{\mathrm{a}}}}\sqrt{\frac{2r_{\oplus}}{r_{\oplus}+r_{\mathrm{a}}}}. $$

(B.5)

Eliminating v_a in the equation of the angular momentum conservation and solving the equations for r_a yields

$$ r_{\mathrm{a}} = \frac{\left( 1 + v_{\infty}/v_{\oplus}\right)^{2}}{2 - \left( 1 + v_{\infty}/v_{\oplus}\right)^{2}} r_{\oplus}. $$

(B.6)

As for the reachable minimum solar distance, that is, the perihelion radius r_p, the spacecraft has the Earth swing-by at the aphelion, and the spacecraft velocity at the swing-by is

$$ v_{\text{sc}} = v_{\oplus} - v_{\infty}. $$

(B.7)

Solving the vis-vita equation and the equation of angular momentum conservation yields the perihelion radius

$$ r_{\mathrm{p}} = \frac{\left( 1 - v_{\infty}/v_{\oplus}\right)^{2}}{2 - \left( 1 - v_{\infty}/v_{\oplus}\right)^{2}} r_{\oplus}. $$

(B.8)

Appendix C: Computation of Pericenter Position and Velocity from V-infinity Vectors

Given $\mathrm {v}_{\infty }^{\text {in}}$ and $\mathrm {v}_{\infty }^{\text {out}}$ such that $\|\mathrm {v}_{\infty }^{\text {in}}\|=\|\mathrm {v}_{\infty }^{\text {out}}\|$, let us find the pericenter position r_p and velocity v_p. The deflection angle is calculated as

$$ \delta = \sin^{-1}\left( \frac{\mathrm{v}_{\infty}^{\text{in}}}{v_{\infty}}\times\frac{\mathrm{v}_{\infty}^{\text{out}}}{v_{\infty}}\right). $$

(C.1)

Using the basic equations of the Kepler orbits, we can calculate the norm of the perigee radius and velocity as

$$ \begin{array}{@{}rcl@{}} r_{\mathrm{p}} &= \frac{\mu}{v_{\infty}^{2}}\left( \frac{1}{\sin\left( \frac{\delta}{2}\right)}-1\right) \end{array} $$

(C.2)

$$ \begin{array}{@{}rcl@{}} v_{\mathrm{p}} &= \sqrt{v_{\infty}^{2} + \frac{2\mu}{r_{\mathrm{p}}}}. \end{array} $$

(C.3)

Finally, the pericenter position and velocity vectors are calculated as

$$ \begin{array}{@{}rcl@{}} \mathrm{r}_{\mathrm{p}}& = \frac{\mathrm{v}_{\mathrm{p}} \times \mathrm{z}}{\|\mathrm{v}_{\mathrm{p}} \times \mathrm{z}\|} r_{\mathrm{p}} \end{array} $$

(C.4)

$$ \begin{array}{@{}rcl@{}} \mathrm{v}_{\mathrm{p}} &= \frac{\mathrm{v}_{\infty}^{\text{in}}+\mathrm{v}_{\infty}^{\text{out}}}{\|\mathrm{v}_{\infty}^{\text{in}}+\mathrm{v}_{\infty}^{\text{out}}\|} v_{\mathrm{p}} \end{array} $$

(C.5)

where $\mathrm {z} = (\mathrm {v}_{\infty }^{\text {in}}\times \mathrm {v}_{\infty }^{\text {out}})/(\|\mathrm {v}_{\infty }^{\text {in}}\times \mathrm {v}_{\infty }^{\text {out}}\|)$.

Appendix D: Asteroid Observable Duration

In asteroid flyby missions, the spacecraft usually observes the approaching asteroid for the identification and optical navigation. Therefore, the asteroid observable duration should be long enough for these operations. Let us calculate the asteroid observable duration in this section.

The maximum observable distance of the asteroid from the spacecraft can be computed as follows [35]

$$ {\varDelta} = \frac{1}{r_{\mathrm{S}}} 10^{\left\{ \frac{V-H+2.5\log_{10} \left\{ (1-G)\phi_{1} + G\phi_{2}\right\} }{5} \right\}} $$

(D.1)

where Δ(au) is the limiting distance between the asteroid and spacecraft, r_S(au) is the distance of the asteroid from the Sun, V is the limiting magnitude of the telescope, H and G are respectively the absolute magnitude and slope parameter of the asteroid, and ϕ₁ and ϕ₂ are defined as

$$ \phi_{i} = \exp \left\{ -A_{i}\left( \tan\frac{\alpha}{2}\right)^{B_{i}} \right\} $$

(D.2)

where A₁ = 3.33,A₂ = 1.87,B₁ = 0.63,B₂ = 1.22, and α is the Sun-asteroid-spacecraft angle during the approach. For example, α = 0 if the asteroid is fully illuminated. The H and G values are taken from The MPC Orbit (MPCORB) Database of the IAU Minor Planet Center.

The observable duration of the asteroid is evaluated as

$$ T_{\text{obs}} = \frac{{\varDelta}}{V_{\text{rel}}} $$

(D.3)

where Δ is defined in Eq. D.1 and V_rel is the relative velocity of the spacecraft with respect to the asteroid.

Appendix E: Comparison between CP and NLP Solutions

Figures 12 and 13 illustrate the control profiles and trajectories of Phase 1 towards 2000 DP107, the primary candidate in Table 3, under a N-body dynamical system. This result shows that the control profiles of the first thrusting arc resemble each other, but the second thrusting arc appears only for the CP solution.

Fig. 12

Control profiles of CP and NLP

Fig. 13

Trajectories of CP and NLP (ECLIPJ2000 inertial frame, Sun-center)