Perturbed HEO Satellite Hovering Investigation in the Earth-Moon System

Abstract

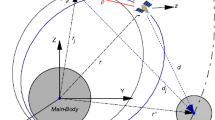
The relative hovering of satellites in highly elliptic orbits (HEO), as one of the most crucial space operations, is modeled and analyzed in this paper. The proposed modeling is based on the new perturbed relative dynamics equations, uses the time-varied parameters depending on the motion of the target satellite. This proposed model considered the dynamic air drag, oblateness of Earth (including all zonal harmonics coefficients) and the Lunar perturbation as an inclined third-body disturbing effect on both follower and target orbits. The non-simplified relative motion model has been obtained by employing the Lagrangian mechanics principals and completed along with the target satellite’s motion characteristic. Then the required thrust for relative hovering mission has been obtained without any simplifications to ensure the accuracy of long-duration flight analyses. To validate the presented model, another model has been built as an ECI based Relative Motion (ERM) model. Then, according to effective parameters on hovering mission design around HEOs such as the eccentricity and inclination of the target obit, the fuel consumption, optimal positioning of the follower, maximum required thrust, and the appropriate time to perform the operation, several examples are provided. Furthermore, the hybrid IWO/PSO algorithm has been used to find the location and the minimum/maximum amounts of thrust force.

Appendices

Appendix 1

For completing of absolute and relative equations of motion of target and follower satellites, the displacement [x_m, y_m, z_m] and velocity $\left[{\dot{x}}_m,{\dot{y}}_m,{\dot{z}}_m\right]$ components of Moon in the LVLH frame must be known. These vectors are achieved by introducing procedure:

• 1- The Lunar classical orbital elements (a_m, i_m, e_m, ω_m, Ω_m, f_m.) are obtained in the mean ecliptic and mean equinox of date coordinate system from [45]. The Moon’s orbital elements are provided in the form of time series, and based on some specific coefficients [36].

• 2- From classical orbital elements, the position and velocity vectors of the Moon in the Earth-Moon perifocal frame are obtained by:

$$ {\overline{r}}_m=\frac{h_m}{\mu_m}\frac{1}{1+{e}_m\ {c}_{f_m}}\left({c}_{f_m}\boldsymbol{p}+{s}_{f_m}\boldsymbol{q}\right) $$

(64)

$$ {\dot{\overline{r}}}_m={\overline{v}}_m=\frac{h_m}{\mu_m}\left[-{c}_{f_m}\boldsymbol{p}+\left({e}_m+{c}_{f_m}\right)\boldsymbol{q}\right] $$

(65)

• 3- the position and velocity vectors of the Moon in the ECI frame is obtained from the perifocal frame by:

$$ {Q}_p^E=\left[\begin{array}{ccc}{c}_{\omega_m}{c}_{\Omega_m}-{s}_{\omega_m}{c}_{{\mathrm{i}}_m}{s}_{\Omega_m}& -{s}_{\omega_m}{c}_{\Omega_m}-{c}_{\omega_m}{c}_{{\mathrm{i}}_m}{s}_{\Omega_m}& {s}_{\omega_m}{s}_{\Omega_m}\\ {}{c}_{\omega_m}{s}_{\Omega_m}+{s}_{\omega_m}{c}_{{\mathrm{i}}_m}{c}_{\Omega_m}& -{s}_{\omega_m}{s}_{\Omega_m}-{c}_{\omega_m}{c}_{{\mathrm{i}}_m}{c}_{\Omega_m}& -{s}_{i_m}{c}_{\Omega_m}\\ {}{s}_{\omega_m}{s}_{{\mathrm{i}}_m}& {c}_{\omega_m}{s}_{{\mathrm{i}}_m}& {c}_{{\mathrm{i}}_m}\end{array}\right] $$

(66)

$$ {r}_m^E={Q}_p^E{\overline{r}}_m $$

(67)

$$ {v}_m^E={Q}_p^E{\overline{v}}_m $$

(68)

• 4- Finally, the position and velocity of the Moon in the LVLH coordinate system (located on the target satellite) can be obtained by:

$$ {\Phi}_E^L=\left[-\begin{array}{ccc}{c}_{\theta }{c}_{\Omega}-{s}_{\theta }{c}_i{s}_{\Omega}& {c}_{\theta }{c}_{\Omega}+{s}_{\theta }{c}_i{c}_{\Omega}& {s}_{\theta }{s}_i\\ {}{s}_{\theta }{c}_{\Omega}-{c}_{\theta }{s}_i{s}_{\Omega}& -{s}_{\theta }{s}_{\Omega}-{c}_{\theta }{c}_i{c}_{\Omega}& {c}_{\theta }{s}_i\\ {}{s}_i{s}_{\Omega}& -{s}_i{c}_{\Omega}& {c}_i\end{array}\right] $$

(69)

$$ {r}_m={\Phi}_E^L\ {r}_m^E={\left[{x}_m\kern0.5em {y}_m\kern0.5em {z}_m\right]}^T $$

(70)

$$ {v}_m={\Phi}_E^L\ {v}_m^E={\left[{\dot{x}}_m\kern0.5em {\dot{y}}_m\kern0.5em {\dot{z}}_m\right]}^T $$

(71)

Appendix 2: Proof of relative motion equations

In this appendix, the procedure to obtain the proposed satellite relative motion by employing of the Lagrangian principle of is briefly summarized.

The Lagrange’s equation used for developing the relative motion model for satellite j is as follows [46]:

$$ \frac{d}{dt}\left(\frac{\partial {K}_j}{\partial {\dot{q}}_n}\right)-\frac{\partial {K}_j}{\partial {q}_n}+\frac{\partial {U}_j}{\partial {q}_n}={Q}_n $$

(72)

In this equation, ${q}_j={\left[{x}_j\kern0.5em {y}_j\kern0.5em {z}_j\right]}^T$is the generalized displacement and ${\dot{q}}_j={\left[{\dot{x}}_j\kern0.5em {\dot{y}}_j\kern0.5em {\dot{z}}_j\right]}^T$ is the generalized velocity. K_j and U_j are kinetic and potential energy (per unit mass) for satellite j. Also, Q_n represents the sum of the non-conservative forces (Here, includes the air drag and the control force) applied on controllable satellite. Now, by exactly computing the potential and kinetic functions for the jth satellite and the non-conservative forces applied on the jth satellite, the relative dynamic motion equations of satellite in the LVLH frame can be derived.

The kinetic energy (per unit mass) for satellite j is obtained by means of:

$$ {K}_j=\frac{1}{2}{\dot{\boldsymbol{r}}}_{\boldsymbol{j}}{\dot{\boldsymbol{r}}}_{\boldsymbol{j}}=\frac{1}{2}\left[{\left({v}_x-{\dot{x}}_j-{y}_i{\omega}_z\right)}^2+{\left(\frac{h}{r}+{\dot{y}}_j+{x}_j{\omega}_x\right)}^2+{\left({\dot{z}}_j+{y}_j{\omega}_x\right)}^2\right] $$

(73)

By substituting Eq. (73) into the first two terms of the Lagrange’s equation (72) will have:

$$ {\displaystyle \begin{array}{l}\frac{d}{dt}\left(\frac{\partial {K}_j}{\partial {\dot{q}}_n}\right)-\frac{\partial {K}_j}{\partial {q}_n}=\\ {}\left[\begin{array}{c}{\ddot{x}}_j-2{\dot{y}}_j{\omega}_z-2{\dot{y}}_j{\omega}_z-{y}_j{\alpha}_z+{z}_j{\omega}_x{\omega}_z-r{\left({\omega}_z\right)}^2+\left({\dot{v}}_x\right)\\ {}{\ddot{y}}_j+2{\dot{x}}_j{\omega}_z-2{\dot{z}}_j{\omega}_x+{x}_j{\alpha}_z-{y}_j{\omega}_z^2-{y}_j{\omega}_x^2+{z}_j{\alpha}_x+2{V}_x\left({\omega}_z\right)+\mathrm{r}\left({\alpha}_z\right)\\ {}\ddot{z}+2{\dot{x}}_j{\omega}_z+{x}_j{\omega}_x{\omega}_z+{y}_j{\alpha}_x-{z}_j{\omega}_x^2-r\left({\omega}_z{\omega}_x\right)\end{array}\right]\end{array}} $$

(74)

By using Equations (2), (37), (38) and (40) and substituting into the expression within parenthesis in Eq. (74), the following expression is obtained:

$$ {\displaystyle \begin{array}{l}\frac{d}{dt}\left(\frac{\partial {K}_j}{\partial {\dot{q}}_n}\right)-\frac{\partial {K}_j}{\partial {q}_n}=\\ {}\left[\begin{array}{c}{\ddot{x}}_j-2{\dot{y}}_j{\omega}_z-{x}_j{\omega}_z^2-{y}_j{\alpha}_z+{z}_j{\omega}_x{\omega}_z-r{\eta}^2-\zeta {s}_i{s}_{\theta }-C\left\Vert {V}_a\right\Vert {v}_x-\frac{\mu_m}{d^3}-{\kappa}_m\ {x}_m\\ {}{\ddot{y}}_j+2{\dot{x}}_j{\omega}_z-2{\dot{z}}_j{\omega}_x+{x}_j{\alpha}_z-{y}_j{\omega}_z^2-{y}_j{\omega}_x^2-{z}_j{\alpha}_x-\zeta {s}_i{c}_{\theta }-C\left\Vert {V}_a\right\Vert \left(\frac{h}{r}-{\omega}_er{c}_i\right)-{\kappa}_m{y}_m\\ {}\ddot{z}+2{\dot{y}}_j{\omega}_x+{x}_j{\omega}_x{\omega}_z+{y}_j{\alpha}_x-{z}_j{\omega}_x^2-\zeta {c}_i-C\left\Vert {V}_a\right\Vert {\omega}_er{c}_{\theta }{s}_i-{\kappa}_m\ {z}_m\end{array}\right]\end{array}} $$

(75)

The sum of gravitational potential functions of satellite j due to the gravitational fields of Earth and Moon as a third-body is expressed as equation (76):

$$ {U}_j\left({r}_j,{c}_{\varphi_j}\right)=-\frac{\mu }{r_j}+\frac{\mu }{r_j}\left[\sum \limits_{n=2}^{\infty }{J}_n{\left(\frac{R_e}{r}\right)}^n\ {P}_n\left({c}_{\varphi_j}\right)\ \right]-{\mu}_m\left(\frac{1}{d_j}-\frac{1}{r_m^3}{\boldsymbol{r}}_{\boldsymbol{j}}\ {\boldsymbol{r}}_{\boldsymbol{m}}\right) $$

(76)

where r_j = (r + x_j)x + y_j y + z_j z.

Also, ${c}_{\phi_j}={r}_{jZ}/{r}_j$; and r_jz is the projection of satellite j’s position vector on the Z axis in the ECI frame.

$$ {\boldsymbol{r}}_{\boldsymbol{j}\boldsymbol{Z}}={\boldsymbol{r}}_{\boldsymbol{j}}\ \boldsymbol{Z}=\left(r+{x}_j\right){s}_i{s}_{\theta }+{y}_j{c}_{\theta }{s}_i+{z}_j{c}_i $$

(77)

Therefore, by using Equations (48), (51) and (77) will have:

$$ \frac{\partial {U}_j}{\partial {q}_j}=\left[\begin{array}{c}{\eta}_j^2\left(r+{x}_i\right)+\zeta {s}_i{s}_{\theta }+{\kappa}_j{x}_m+{\mu}_m\left(r+{x}_m\right)/{d}_j^3\\ {}{\eta}_j^2{y}_j+\zeta {s}_i{c}_{\theta }+{\kappa}_j{y}_m+{\mu}_m{x}_m/{d}_j^3\\ {}{\eta}_j^2{z}_j+{\zeta}_j{c}_i+{\kappa}_j{z}_m+{\mu}_m{z}_m/{d}_j^3\end{array}\right] $$

(78)

At this point, the effects of the non-conservative air drag and control forces should be considered in the relative nonlinear equations of motion. So in this paper, in addition to the gravitational effect, the effects of the control force and the air drag have been incorporated into the Lagrange’s equations as Q_n terms. To extract the drag force, the relative velocity of satellite j should be determined first. This term is expressed as follows:

$$ {\boldsymbol{V}}_{\boldsymbol{j}}=\boldsymbol{V}+{\boldsymbol{\rho}}_{\boldsymbol{j}}+\boldsymbol{\omega} \times {\boldsymbol{\rho}}_{\boldsymbol{j}} $$

(79)

$$ {\boldsymbol{V}}_{\boldsymbol{aj}}={\boldsymbol{V}}_{\boldsymbol{j}}-{\boldsymbol{\omega}}_{\boldsymbol{e}}\times {\boldsymbol{r}}_{\boldsymbol{j}} $$

(80)

Now by using Equations (18) and (78) and substituting into Eq. (79), the velocity vector of the jth satellite will be obtained:

$$ {\boldsymbol{V}}_{{\boldsymbol{a}}_{\boldsymbol{j}}}=\left[\begin{array}{c}{v}_x+{\dot{x}}_j-{y}_j{\omega}_z-{\omega}_e{z}_j{c}_{\theta }{s}_i+{\omega}_e{y}_j{c}_i\\ {}\frac{h}{r}+{\dot{y}}_j+{x}_j{\omega}_x-{z}_j{\omega}_x+{z}_j{\omega}_e{s}_i{s}_{\theta }-{\omega}_e\left(r+{x}_j\right){c}_i\\ {}{\dot{z}}_j+{\omega}_e{y}_j{s}_i{s}_{\theta }+{\omega}_e\left(r+{x}_j\right){c}_{\theta }{s}_i\end{array}\right] $$

(81)

Now, the radial, along-track and cross-track components of non-conservative force can be obtained with consideration of drag force ${F}_{drag}=-{C}_j\ \left\Vert {\mathbf{V}}_{a_j}\right\Vert {\mathbf{V}}_{a_j}$ as follows:

$$ {Q}_{xj}={C}_j\left\Vert {\boldsymbol{V}}_{\boldsymbol{aj}}\right\Vert \left({v}_x+{\dot{x}}_j-{y}_j{\omega}_z-{\omega}_e{z}_j{c}_{\theta }{s}_i+{\omega}_e{y}_j{c}_i\right)+{T}_{xj} $$

(82)

$$ {Q}_{yj}={C}_j\left\Vert {\boldsymbol{V}}_{\boldsymbol{aj}}\right\Vert \left(\frac{h}{r}+{\dot{y}}_j+{x}_j{\omega}_x-{z}_j{\omega}_x+{z}_j{\omega}_e{s}_i{s}_{\theta }-{\omega}_e\left(r+{x}_j\right){c}_i\right)+{T}_{yj} $$

(83)

$$ {Q}_{zj}={C}_j\left\Vert {\boldsymbol{V}}_{\boldsymbol{aj}}\right\Vert \left({\dot{z}}_j+{\omega}_e{y}_j{s}_i{s}_{\theta }+{\omega}_e\left(r+{x}_j\right){c}_{\theta }{s}_i\right)+{T}_{zj} $$

(84)

Finally, by substituting Equations (75), (78) and (82–84) into Eq. (72) and considering the conditions of the hovering operation and with consideration of Appendix1, Equations (45–47) will be proved.