Abstract
The interactions of nitrogen dioxide molecule with TiO2-supported Au nanoparticles were investigated using density functional theory. Surface Au atoms on the TiO2-supported Au overlayer were found to be the most favorable binding sites, thus making the adsorption process very strong. Both oxygen and nitrogen atoms of the NO2 molecule can bind to the Au surface by forming strong chemical bonds. The adsorption of NO2 molecule on the considered structures gives rise to significant changes in the bond lengths, bond angles, and adsorption energies of the complex systems. The results indicate that NO2 adsorption on the TiO2-supported Au nanoparticle by its oxygen atoms is energetically more favorable than the NO2 adsorption by its nitrogen atom, indicating the strong binding of NO2 to the TiO2-supported Au through its oxygen atoms. Thus, the bridge configuration of TiO2/Au + NO2 is found to be the most stable configuration. Both oxygen and nitrogen atoms of NO2 move favorably towards the Au surface, as confirmed by significant overlaps in the PDOSs of the atoms that forming chemical bonds. This study not only suggests a theoretical basis for gas-sensing properties of the TiO2-supported Au nanoparticles, but also offers a rational approach to develop nanostructure-based chemical sensors with improved performance.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
TiO2 is one of the most broadly studied transition metal semiconductors with outstanding properties, such as non-toxicity, high catalytic efficiency, and extensive bandgap [1]. Until now, various kinds of well-known applications have been proposed for TiO2, such as photo-catalysis, gas sensor devices, organic dye-sensitized solar cells, water splitting, and air pollution control [2,3,4,5]. Anatase, rutile, and brookite are the most important polymorphs of TiO2 [6]. Of the three polymorphs of TiO2, the rutile form is found to be the most stable phase. There is not any detailed theoretical investigation on the physical and chemical properties of brookite because of its metastable property. This meta-stability results in some troubles during the synthesis of brookite [7]. The improved reactivity of anatase is comparable with that of rutile and brookite phases [8,9,10,11,12,13,14]. Anatase has been extensively studied due to its enhanced activity in some photo-catalysis reactions, such as TiO2-supported metal particle reactions, compared to the rutile and brookite phases [15,16,17]. Unfortunately, as a most promising material, the widely application of TiO2-based gas sensors is influenced by its wide bandgap (3–3.2 eV). This results in the absorption of a small percentage of the incoming solar light (3–5%). An enormous amount of effort has been invested in enhancing the optical response of TiO2 by nitrogen doping [8].
Recently, gold was considered as an inactive metal, which possesses less activity than the other metals in many reactions. Haruta and co-workers showed that gold particles can increase the combustion of CO molecule and promote different catalytic reactions [18]. The gold particles supported by metal oxides (oxide-supported gold particles) have gained more attention due to their higher activities in the surface processes [19,20,21,22]. This leads to the structures with enhanced catalytic activity and higher stability [23, 24]. There are a large number of important reactions, in which the oxide-supported Au overlayers play a key role, including the epoxidation of C3H6 [25], reduction of NO x molecules [26], and dissociation of SO2 molecule [27]. TiO2 has been considered as one of the most appropriate support materials for gold particles [28, 29]. The interactions of gold nanoparticles with TiO2 (rutile and anatase) have been widely studied in the last few years. Vittadini et al. considered the adsorption behaviors of gold clusters on the TiO2 anatase (101) surfaces [30]. Metiu and co-workers investigated the adsorption site and electronic structures of the TiO2 rutile-supported Au nanoparticles [31].
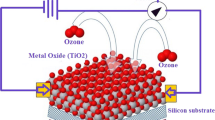
The adsorption of the O2 and CO2 on gold nanoparticles supported by TiO2 has been investigated by DFT calculations [32]. The main source of nitrogen dioxide emission is internal combustion engines, burning fossil fuels. It also results from cigarette smoke, kerosene heaters, and vehicle engines and stoves. Thus, finding an efficient sensor for the removal of this toxic molecule is an important issue to public health and environmental protection [33]. An ideal semiconductor oxide-based gas sensor should have properties, such as high sensitivity to the expected toxic material, low price fabrication, and compatibility with modern electronic devises. Among different gas sensors, the oxide-supported gold nanoparticles have been characterized as efficient sensor materials because of their higher activities. Therefore, establishing multi-component structures in sensor materials has long been regarded as the best strategy for improving the sensing performance of TiO2 particles. The mechanism of gas sensing for the removal of toxic NO2 molecules by metal oxide-based sensors is represented in Fig. 1. We have decided to perform a DFT study of the interaction of NO2 molecule with the TiO2-supported Au overlayers to fully exploit the gas-sensing capabilities of these nanocomposites.
The consecutive adsorption of NO2 molecules on the TiO2-supported Au overlayers probably produces N2 molecule formed from the central nitrogen atoms of the two adsorbed NO2 molecules. This is a consequence of the formation of weak chemical bonds between oxygen atoms of NO2 molecule and Ti sites of the adsorbent. This leads to weakening of the bond between central nitrogen and the side oxygen atoms of the adsorbed NO2 molecules. Based on this fact, we can conclude desorption of NO2 molecule from the surface of the TiO2-supported Au overlayer. The next NO2 molecule then can be adsorbed on the considered nanocomposite, and this consecutive process was repeated over and over again to obtain the enhanced sensing performance of the adsorbent material. Figure 1 shows a schematic structure of a metal oxide-based gas sensor. We have also commented on the charge analysis of the complex system according to the Mulliken population analysis. In this study, the main objective is to perform a systematic investigation on the adsorption behaviors of the TiO2-supported Au nanoparticles as potentially efficient gas sensors for NO2 detection.
Computational methods
Details of computation
All of DFT calculations [34, 35] were carried out using the Open source Package for Material eXplorer (OPENMX3.8) [36]. OPENMX is an efficient software package for nano-scale materials simulations based on norm-conserving pseudo-potentials and pseudo-atomic localized basis functions [37, 38]. To optimize the structures, the pseudoatomic orbitals (PAOs) centered on atomic sites were used as basis sets. The calculations were done with a considered energy cutoff of 150 Ry. Pseudo-atomic orbitals were constructed by minimal basis sets (three s-state, three p-state, and one d-state radial functions) for the Ti, (three s-state, three p-state, two d-state, and one f-state radial functions) for the Au, and (two s-state, two p-state radial functions) for O and N atoms, within cut-off radii of basis functions set to the values of seven for Ti, nine for Au, and five for O and N (all in Bohrs). The total energy of the system was computed within the Perdew–Burke–Ernzerhof (PBE) form of the generalized gradient approximation (GGA) exchange–correlation potential [39]. Mulliken population analysis was also conducted to fully analyze the charge transfer between NO2 and nanocomposite. To optimize the adsorption configurations of the TiO2-supported Au overlayers with adsorbed NO2 molecules, all atoms of the system are entirely relaxed until the force on each atom is less than 0.01 eV/Å. The size of the simulation box containing pristine TiO2-supported Au nanoparticles is 20 Å × 20 Å × 30 Å, being larger than the realistic size of the composite system.
Three possible orientations of NO2 towards the TiO2-supported Au nanoparticles are studied in this work. XCrysDen program was used for visualization of the figures presented in this study [40]. The total number of atoms of the nanocomposite in the considered box is 88 atoms (16 Au, 48 O, and 24 Ti atoms) of undoped TiO2-supported Au overlayer. The effects of vdW interactions were also taken into account in this study. Both LDA and GGA methods cannot describe the vdW interactions in the systems, such as NO2 adsorption, on the TiO2-supported Au nanoparticles. Thus, an inclusion of additional functional into standard DFT methods would be required, which correctly describes the effects of vdW interactions. Grimme’s DFT-D2 [41] and DFT-D3 methods [42, 43] were used in this study to correct the adsorption energies for dispersion energy. The adsorption energy, E ad, is estimated as the following equation:
where E (composite + adsorbate) is the total energy of the TiO2-supported Au overlayers with adsorbed NO2, E composite is the energy of bare TiO2-supported Au overlayer, and E adsorbate represents the energy of a free gas-phase NO2 molecule. As distinct from this equation, the adsorption energies of energy favorable configurations are negative.
Modeling TiO2-supported Au nanoparticles
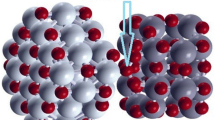
We have constructed TiO2 anatase nanoparticle using a 3 × 2 × 1 supercell of TiO2 anatase. The considered unit cell is available at “American Mineralogists Database” webpage [44] and reported by Wyckoff [45]. The size of the studied nanoparticles was chosen following Lei et al. [46] and Liu et al. [47]. The results published by Lei et al. [46] show that the smaller the particle is, the higher the average energy is. They have explained that the nanoparticles containing 72 atoms have the lowest energy (the highest stability among the different types of nanoparticles. The optimized structure of the pristine TiO2 nanoparticle is shown in Fig. 2. The constructed structure of pristine TiO2 nanoparticle was geometrically optimized and then coupled with Au nanoparticle to model a metal oxide-supported Au overlayer. The calculated structural parameters for the chosen Au nanoparticle are listed in Table S1. The atomic number ratio between titanium and oxygen atoms should be set as 1:2, which was obtained by setting two dangling oxygen atoms in TiO2. Spin polarization is not used for the optimization of pristine TiO2 particles due to the even electron number of pure particles. During the optimization process, “Cluster” method was used as efficient eigenvalue solver. For electronic structure calculations, the convergence criterion of 1.0 × 10−6 Hartree was used, whereas the criterion for geometry optimization was set at 1.0 × 10−4 Hartree/bohr. In addition, “Opt” was used as geometry optimizer, which presents a robust scheme for optimization of solid-state structures based on cluster method.
The surface energies for TiO2 anatase were computed and summarized in Table 1. The calculated data from GGA are in reasonable agreement with the experimentally reported data or other computational works [48]. It indicates that GGA pseudo-potential possesses a reasonable accuracy for calculating the properties of anatase particles. The energy calculations were carried out at the Γ point. The considered simulation box has the dimension of 20 Å × 15 Å × 30 Å. To reduce the additional interactions between neighbor particles, a 11.5 Å distance in three directions was considered.
TiO2 includes two types of titanium atoms, namely, five-fold coordinated (5f-Ti) and six-fold coordinated (6f-Ti), as well as two types of oxygen atoms, indicated by three-fold coordinated (3f-O) and two-fold coordinated (2f-O) atoms [49, 50]. For the bent geometry of the NO2 molecule, the calculated N–O bond length and O–N–O bond angle are about 1.20 Å and 134.3°, respectively, which agree reasonably with the previous gas-phase data [51]. Au nanoparticle was supported by TiO2 anatase to model a TiO2-supported Au overlayer. Figure 3 displays the equilibrium structure of the undoped TiO2-supported Au nanoparticle.
Results and discussion
Structural parameters and adsorption energies
Three possible orientations of the NO2 molecule towards the TiO2-supported Au overlayers were considered, in which the NO2 molecule can bind to the surface of Au atoms either by its nitrogen or by oxygen atoms. The relevant configurations of NO2 adsorption on the TiO2-supported Au nanoparticles are shown in Fig. 4, as indicated by adsorption types A–C. We found that the NO2 interaction with Au atoms is stronger than the interaction with TiO2 nanoparticle. Thus, the surfaces of Au atoms are strongly favored during the adsorption process. Over the TiO2-supported Au nanoparticle, the NO2 molecule preferentially interacts with the Au nanoparticle. The interaction by oxygen atoms leads to a bridge configuration of NO2 on the nanocomposite. As a closer comparison, it is of eminent importance to describe the adsorption configurations and relative orientations in detail. Configuration A shows the adsorption of NO2 on the top-site Au atoms of the TiO2-supported Au, while configuration C represents the interaction of NO2 with the lateral-site Au atoms. In configuration B, we can see that NO2 molecule interacts with the top-site Au atoms by its nitrogen atom, providing a single contacting point between NO2 and nanocomposite. Configurations A and C indicate a double contacting point between NO2 and TiO2-supported Au. Figure S1 also displays the top views of NO2 molecule adsorbed on the TiO2-supported Au overlayers.
Optimized geometry configurations of the NO2 molecule adsorbed on the undoped TiO2-supported Au overlayers in different orientations, a NO2 adsorption on the top-Au sites by its oxygen atoms (configuration A), b NO2 adsorption on the top-Au site by its nitrogen atom (configuration B), and c NO2 adsorption on the side-Au sites by its oxygen atoms (configuration C)
Table 2 summarizes the lengths and distances for the newly formed Au–O bonds, N–O bonds of the adsorbed NO2 molecule, and O–N–O bond angles of NO2 after the adsorption process. Based on the obtained results, we found that the N–O bonds of the adsorbed NO2 molecule were elongated due to the considerable electronic density shifts from the Au–Au bonds of Au nanoparticle and N–O bonds of the NO2 molecule to the newly formed Au–O and Au–N bonds between the nanocomposite and NO2. Thus, the adsorption process results in weakening the N–O bonds of the NO2 molecule. The O–N–O bond angles of NO2 were increased compared to those of non-adsorbed NO2 molecule. This increase in the bond angles could be mostly attributed to the elongation of the N–O bonds of the adsorbed NO2 molecule. In configuration B, the bond angle increase and geometry changing could be ascribed to the formation of new Au–N bond. The formation of new bond is a key reason, which is responsible for changing the sp hybridization of the nitrogen atom in the NO2 molecule to hybridization with higher p contribution (near-sp 2). Consequently, the p characteristics of bonding molecular orbitals of the nitrogen atom in the adsorbed NO2 molecule become higher.
For clear comparison, the adsorption configurations of NO2 molecule on pristine Au and TiO2 nanoparticles are also represented in Fig. 5, indicating less stable adsorption of NO2 on the considered bare nanoparticles. Table 3 summarizes the adsorption energies of NO2 molecules adsorbed on the pristine TiO2-supported Au nanoparticles. Of the three configurations, configuration A has the highest value of adsorption energy, thus making it the most favorable adsorption configuration and, consequently, the most likely binding site to be located on the TiO2-supported Au.
Therefore, the adsorption of NO2 on the TiO2-supported Au nanoparticle (configuration A) is more favorable in energy than the adsorptions in configurations B and C. For both adsorption types A and C, the adsorption energy is higher (more negative) than the adsorption energy of adsorption type B. The reason is that the configurations A and C provide a double contacting point between the NO2 and TiO2-supported Au, whereas configuration B shows a single contacting point. NO2 molecule was strongly coordinated to the TiO2-supported Au by its two oxygen atoms. In other words, two oxygen atoms of the NO2 molecule can interact with the TiO2-supported Au overlayer more efficiently. The adsorption energies calculated from DFT-D2 and DFT-D3 methods are significantly larger than those obtained from the standard DFT calculations. Tamijani et al. [52] reported the results of the adsorption of noble-gas atoms on the TiO2 (110) surface-based van der Waals corrected DFT approach and clearly demonstrated the increase in the adsorption energies caused by vdW interactions.
The adsorption energies are considerably increased when the adsorption energies are corrected for dispersion energy, as shown in Table 3. We have calculated the adsorption energies for NO2 molecule on the bare Au and TiO2 nanoparticles. As can be seen from Table 3, the adsorption energy of NO2 molecule on the Au nanoparticle is about −1.23 eV and that of pristine TiO2 is −0.72 eV, and NO2 adsorption on the TiO2-supported Au nanocomposite is found to be −1.48 eV. The higher adsorption energy gives rise to a strong interaction between the adsorbent and adsorbed molecules, and its more negative sign also represents an energy favorable process. Thus, NO2 adsorption on the TiO2-supported Au nanocomposite is more energetically favorable than the adsorption on the bare Au and TiO2 nanoparticles. In the TiO2-supported Au overlayers, the interactions of NO2 and TiO2 are stronger than those between NO2 and bare TiO2 nanoparticles, indicating that Au nanoparticle is conducive to the interaction of NO2 molecule with TiO2 nanoparticles. In other words, Au nanoparticle enhances NO2 detection by means of the TiO2-supported Au nanocomposite-based sensors. Therefore, the results of adsorption energies suggest that the TiO2-supported Au nanoparticle is a good candidate to be utilized in sensing of toxic NO2 molecules in the environment.
Electronic structures
Figure 6 displays the projected density of states (PDOSs) for NO2 adsorbed on the pristine TiO2-supported Au overlayers. Panels (a, b) in this figure show the PDOSs of the Au atom of gold nanoparticle and oxygen atoms of the NO2 molecule (configuration A). The great overlaps between the PDOSs of these two atoms indicate that the Au atoms form chemical bonds with the oxygen atoms of NO2. In panels (c, d), we can see also the PDOSs of the Au atom of gold nanoparticle and the nitrogen atoms (configuration B), indicating substantial overlaps and thus formation of chemical Au–N bond. For configuration C, the pertinent PDOSs of the oxygen atoms of NO2 molecule and the Au atoms are displayed in panels (e, f). As distinct from these PDOSs, the large overlaps show that both oxygen atoms of NO2 molecule form chemical bonds with the Au atoms of the gold nanoparticle. This delivers a double interaction point between the NO2 and TiO2-supported Au. The interaction of NO2 molecule with bare Au and TiO2 nanoparticles was also examined, and the relevant PDOSs are shown in Fig. 7, representing noticeable overlaps between the PDOSs of the interacting atoms. This implies that the gold and titanium atoms form chemical bonds with the oxygen atoms of NO2.
We also presented the PDOSs of five d orbitals of the Au atom and the oxygen atoms of the NO2 molecule (configuration A). Figures 8, 9 show the PDOSs of the oxygen atom of NO2 and different d orbitals of the Au atom, representing higher overlaps between the PDOSs of the oxygen with d 3 orbital of the Au atom. This indicates that the oxygen atom has a substantial mutual interaction with the d 3 orbital of the Au atom. Similarly, Fig. 10 displays the PDOSs of the nitrogen atom of the NO2 and d orbitals of the Au atom, demonstrating noticeable overlaps between the nitrogen atom and d 2 orbital. For configuration C, the calculated PDOSs of the atom and different orbitals of Au atom are represented in Fig. S2.
We geometrically optimized the structure of TiO2 anatase, and then calculated its band structure. The energy band structure of pristine anatase system is shown in Fig. 11. This figure represents that the calculated band structure of the valence band maximum (VBM) and the conduction band minimum (CBM) was both positioned at the G point. It also represents that pristine TiO2 was a direct-gap semiconductor material. The calculated band-gap (BG) energy was 2.16 eV for TiO2 anatase, which was slightly lower than the experimental result of 3.2 eV. Important to note is that, the electronic structure calculation using GGA pseudo-potential usually underestimates energy bandgaps [53, 54]. This band-gap underestimation is mostly ascribed to the well-known limitation of the exchange–correlation functional in describing excited states. Here, valance band corresponds to the O 2p orbitals and the conduction band arises from Ti 3d orbitals.
Mulliken charge analysis
The Mulliken population analysis was also conducted in this work to fully describe the charge exchange between the NO2 molecule and TiO2-supported Au overlayer. This method of charge analysis provides a means of estimating partial atomic charges from calculations implemented by computational chemistry packages. The calculated Mulliken charge values are listed in Table 3. This method assigns an electronic charge to a given atom in the considered system, that is, the gross atom population (GAP). The charge difference, ∆Q A, is a measure of the difference between the number of electrons on the isolated free atom (Z A) and the gross atom population:
For instance, configuration A represents a sizeable charge transfer of about 0.15 |e| (e, the electron charge) from the TiO2-supported Au nanoparticle to the NO2 molecule, implying that NO2 plays an important role as a charge acceptor. It is worth mentioning that the charge exchange between adsorbent and adsorbed molecule affects the conductivity of the system, being a great strategy to design more efficient and more appropriate sensor devices for the detection of NO2 in the environment.
One of the most important problems in Mulliken charge analysis is the intense sensitivity of Mulliken charges to the basis set choice. Fundamentally, a comprehensive basis set for a molecule can be covered by placing a large set of functions on a single atom. In the Mulliken scheme, all the electrons would then be assigned to the single atom. Therefore, it is well known that the Mulliken charge approach has no complete basis set limit, as the precise value which strongly depends on the way the limit is approached. Consequently, an efficient convergence for charges does not exist, and different basis set families may produce extremely different results. To overcome this problem, many modern approaches can be tried for estimating net atomic charges, such as electrostatic potential and natural population methods [55]. We have also calculated the Mulliken charges with the large basis sets of higher accuracy and then found that increasing basis set can simply modify Mulliken charges by approximately 0.22 e. The obtained results are summarized in Table 3. This table shows that the strong basis sets give rise to an increase in the Mulliken charge values.
Conclusions
DFT calculations were carried out to investigate the sensing performance of undoped TiO2-supported Au nanoparticles for the detection of NO2 molecules. The adsorption behaviors of NO2 on the TiO2-supported Au nanoparticles were investigated in detail. The results show that the N–O bonds of the adsorbed NO2 molecule were elongated after the adsorption process, which indicates the weakening N–O bonds of the NO2 molecule. The results also suggest that the interaction of the NO2 molecule with the TiO2-supported Au overlayer through its oxygen atoms is energetically more favorable than the interaction of NO2 through its nitrogen atom. This interaction provides a double interacting point between the NO2 and TiO2-supported Au, suggesting the strong adsorption of NO2 over the substrate. The current results suggest that Au nanoparticle in the TiO2-supported Au nanocomposites affects the final configuration of TiO2 nanoparticles with adsorbed NO2 molecules and, therefore, strengthens the interaction between NO2 and TiO2. The substantial overlaps between the PDOSs of the Au and oxygen atoms, as well as, Au and nitrogen atoms indicate the formation of chemical bonds between them. Mulliken population analysis reveals a noticeable charge transfer from the TiO2-supported Au to the NO2 molecule, indicating the acceptor characteristic of the NO2 molecule. Based on the inclusion of vdW interactions, we found that the adsorption energies become larger. However, our findings thus suggest that the TiO2-supported Au nanoparticles can be utilized as potentially efficient gas sensors for NO2 recognition.
References
Fujishima, A., Honda, K.: Electrochemical photolysis of water at a semiconductor electrode. Nature 238, 37–38 (1972)
Fujishima, A., Hashimoto, K., Watanabe, T.: TiO2 photocatalysis: fundamentals and applications. Bkc, Tokyo (1999)
Abbasi, A., Sardroodi, J.J.: N-doped TiO2 anatase nanoparticles as a highly sensitive gas sensor for NO2 detection: insights from DFT computations. J. Environ. Sci. Nano. 3, 1153–1164 (2016)
Abbasi, A., Sardroodi, J.J.: Modified N-doped TiO2 anatase nanoparticle as an ideal O3 gas sensor: insights from density functional theory calculations. J. Comp. Theor. Chem. 600, 2457–2469 (2016)
Fujishima, A., Zhang, X., Tryk, D.A.: TiO2 photocatalysis and related surface phenomena. J. Surf. Sci. Rep. 63, 515–582 (1992)
Batzilla, M., Morales, E.H., Diebold, U.: Surface studies of nitrogen implanted TiO2. J. Chem. Phys. 339, 36–43 (2007)
Isley, S.L., Penn, R.L.: Relative brookite and anatase content in sol–gel synthesized titanium dioxide nanoparticles. J. Phys. Chem. B 110, 15134 (2006)
Rumaiz, A.K., Woicik, J.C., Cockayne, E., Lin, H.Y., Jaffari, G.H., Shah, S.I.: Oxygen vacancies in N doped anatase TiO2: experiment and first-principles calculations. J. Appl. Phys. Lett. 95, 262111 (2009)
Buonsanti, R., Grillo, V., Carlino, E., Giannini, C., Kipp, T., Cingolani, R., Cozzoli, P.D.: Nonhydrolytic synthesis of high-quality anisotropically shaped brookite TiO2 nanocrystals. J. Am. Chem. Soc. 130, 11223–11233 (2008)
Cassaignon, S., Koelsch, M., Jolivet, J.P.: Selective synthesis of brookite, anatase and rutile nanoparticles: thermolysis of TiCl4 in aqueous nitric acid. J. Mater. Sci. 42, 6689–6695 (2007)
Di Paola, A., Addamo, M., Bellardita, M., Cazzanelli, E., Palmisano, L.: Preparation of photocatalytic brookite thin films. Thin Solid Films 515(7), 3527–3529 (2007)
Djaoued, Y., Bruning, R., Bersani, D., Lottici, P.P., Badilescu, S.: Sol–gel nanocrystalline brookite-rich titania films. Mater. Lett. 58(21), 2618–2622 (2004)
Iskandar, F., Nandiyanto, A.B.D., Yun, K.M., Hogan, C.J., Okuyama, K., Biswas, P.: Enhanced photocatalytic performance of brookite TiO2 macroporous particles prepared by spray drying with colloidal templatings. Adv. Mater. 19, 1408–1412 (2007)
Kobayashi, M., Petrykin, V.V., Kakihana, M.: One-step synthesis of TiO2 (B) nanoparticles from a water-soluble titanium complex. Chem. Mater. 19, 5373–5376 (2007)
Li, J.G., Ishigaki, T., Sun, X.D.: Anatase, brookite, and rutile nanocrystals via redox reactions under mild hydrothermal conditions: phase-selective synthesis and physicochemical properties. J. Phys. Chem. C 111, 4969–4976 (2007)
Reddy, M.A., Kishore, M.S., Pralong, V., Caignaert, V., Varadaraju, U.V.: Room temperature synthesis and Li insertion into nanocrystalline rutile TiO2. Electrochem. Commun. 8(8), 1299–1303 (2006)
Shibata, Y., Irie, H., Ohmori, M., Nakajima, A., Watanabe, T., Hashimoto, K.: Comparison of photochemical properties of brookite and anatase TiO2 films. Phys. Chem. Chem. Phys. 6, 1359–1362 (2007)
Haruta, M., Kobayashi, T., Sano, H., Yamada, N.: Novel gold catalysts for the oxidation of carbon monoxide at a temperature far below 0 & #xB0;C. J. Chem. Lett. 16(2), 405–408 (1987)
Landon, P., Collier, P.J., Papworth, A.J., Kiely, C.J., Hutchings, G.J.: Direct formation of hydrogen peroxide from H2/O2 using gold catalysts. Chem. Commun. 18, 2058 (2002)
Molina, L.M., Hammer, B.: Some recent theoretical advances in the understanding of the catalytic activity of Au. Appl. Catal. A Gen. 291, 21–31 (2005)
Okumura, M., Tsubota, S., Haruta, M.: Preparation of supported gold catalysts by gas-phase grafting of gold acethylacetonate for low-temperature oxidation of CO and of H2. J. Mol. Catal. A: Chem. 199, 73–84 (2003)
Lopez, N., Norskov, J.K.: Catalytic CO oxidation by a gold nanoparticle: a density functional study. J. Am. Chem. Soc. 124, 11262–11263 (2002)
Chen, M.S., Goodman, D.W.: Structure–activity relationships in supported Au catalysts. Catal. Today. 111, 22–33 (2006)
Kung, H.H., Kung, M.C., Costello, C.K.: Supported Au catalysts for low temperature CO oxidation. J. Catal. 216, 425–432 (2003)
Hayashi, T.M., Tanaka, K., Haruta, M.: Selective vapor-phase epoxidation of propylene over Au/TiO2 catalysts in the presence of oxygen and hydrogen. J. Catal. 178, 566–575 (1998)
Salama, T., Ohnishi, R., Shido, T., Ichikawa, M.: Highly selective catalytic reduction of NO by H2 over Au 0 and Au(I) impregnated in NaY zeolite catalysts. J. Catal. 162(2), 169–178 (1996)
Rodriguez, J.A., Liu, G., Jirsak, T., Hrbek, J., Chang, Z.P., Dvorak, J., Maiti, A.: Activation of gold on titania: adsorption and reaction of SO2 on Au/TiO2 (110). J. Am. Chem. Soc. 124, 5242–5250 (2002)
Chen, M.S., Goodman, D.W.: The structure of catalytically active gold on titania. Science 306(5694), 252–255 (2004)
Cosandey, F., Madey, T.E.: Growth, morphology, interfacial effects and catalytic properties of au on TiO2. Surf. Rev. Lett. 8, 73 (2001)
Vittadini, A., Selloni, A.: Small gold clusters on stoichiometric and defected TiO2 anatase (101) and their interaction with CO: a density functional study. J. Chem. Phys. 117, 353–361 (2002)
Chrétien, S., Metiu, H.: O2 evolution on a clean partially reduced rutile TiO2 (110) surface and on the same surface precovered with Au1 and Au2: the importance of spin conservation. J. Chem. Phys. 127, 084704 (2007)
Molina, L.M., Rasmussen, M.D., Hammer, B.: Adsorption of O2 and oxidation of CO at Au nanoparticles supported by TiO2 (110). J. Chem. Phys. 120(16), 7673 (2004)
Lin, C., Wen, G., Liang, A., Jiang, Z.: A new resonance Rayleigh scattering method for the determination of trace O3 in air using rhodamine 6G as probe. J. RSC. Adv. 3, 6627–6630 (2013)
Hohenberg, P.M., Kohn, W.: Inhomogeneous electron gas. J. Phys. Rev. 16, B864–B868 (1964)
Kohn, W., Sham, L.: Self-consistent equations including exchange and correlation effects. J. Phys. Rev. 140, A1133–A1138 (1965)
Ozaki, T., Kino, H., Yu, J., Han, M.J., Kobayashi, N., Ohfuti, M., Ishii, F., et al.: The code OpenMX, pseudoatomic basis functions, and pseudopotentials are available on a web site ‘http://www.openmxsquare.org’ (2017). Accessed 2 Mar 2017
Ozaki, T.: Variationally optimized atomic orbitals for large-scale electronic structures. J. Phys. Rev. B 67, 155108 (2003)
Ozaki, T., Kino, H.: Numerical atomic basis orbitals from H to Kr. J. Phys. Rev. B 69, 195113 (2004)
Perdew, J.P., Burke, K., Ernzerhof, M.: Generalized gradient approximation made simple. J. Phys. Rev. Lett. 78, 1396 (1981)
Koklj, A.: Computer graphics and graphical user interfaces as tools in simulations of matter at the atomic scale. J. Comput. Mater. Sci. 28, 155–168 (2003)
Grimme, S.: Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comput. Chem. 27, 1787–1799 (2006)
Grimme, S., Antony, J., Ehrlich, S., Krieg, H.: A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H–Pu. J. Chem. Phys. 132, 154104 (2010)
Grimme, S., Ehrlich, S., Goerigk, L.: Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 32, 1456–1465 (2011)
Downs, R.T.: Web page at: http://rruff.geo.arizona.edu/AMS/amcsd.php (2014). Accessed 9 May 2014
Wyckoff, R.W.G.: Crystal structures, 2nd edn. Interscience Publishers, New York (1963)
Lei, Y., Liu, H., Xiao, W.: First principles study of the size effect of TiO2 anatase nanoparticles in dye-sensitized solar cell. Modelling Simul. Mater. Sci. Eng. 18, 025004 (2010)
Liu, J., Dong, L., Guo, W., Liang, T., Lai, W.: CO adsorption and oxidation on N-doped TiO2 nanoparticles. J. Phys. Chem. C 117, 13037–13044 (2013)
Lazzeri, M., Vittadini, A., Selloni, A.: Structure and energetics of stoichiometric TiO2 anatase surfaces. Phys. Rev. B. 63, 155409 (2001)
Wu, C., Chen, M., Skelton, A.A., Cummings, P.T., Zheng, T.: Adsorption of arginine–glycine–aspartate tripeptide onto negatively charged rutile (110) mediated by cations: the effect of surface hydroxylation. ACS Appl. Mat. Interfaces 5, 2567–2579 (2013)
Liu, J., Liu, Q., Fang, P., Pan, C., Xiao, W.: First principles study of the adsorption of a NO molecule on N-doped anatase nanoparticles. J. Appl. Surf. Sci. 258, 8312–8318 (2012)
Breedon, M., Spence, R.M., Yarovsky, I.: Adsorption of NO2 on oxygen deficient ZnO (2110) for gas Sensing applications: a DFT study. J. Phys. Chem. C 14(39), 16603–16610 (2010)
Tamijani, A.A., Salam, A., de-Lara-Castells, P.: Adsorption of noble-gas atoms on the TiO2 (110) surface: an ab initio assisted study with van der Waals corrected DFT. J. Phys. Chem. C 120(32), 18126–18139 (2016)
Longa, M., Cai, W., Wang, Z., Liu, G.: Correlation of electronic structures and crystal structures with photocatalytic properties of undoped, N-doped and I-doped, TiO2. Chem. Phys. Lett. 420, 71–76 (2006)
Gao, H., Zhou, J., Dai, D., Qu, Y.: Photocatalytic activity and electronic structure analysis of N-doped anatase TiO2: a combined experimental and theoretical study. Chem. Eng. Technol. 32(9), 867–872 (2009)
Reed, A.E., Weinstock, R.B., Weinhold, F.: Natural population analysis. J. Chem. Phys. 83(2), 735–746 (1985)
Acknowledgements
This work was supported by Azarbaijan Shahid Madani University.
Author information
Authors and Affiliations
Corresponding author
Electronic supplementary material
Below is the link to the electronic supplementary material.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Abbasi, A., Sardroodi, J.J. A novel nitrogen dioxide gas sensor based on TiO2-supported Au nanoparticles: a van der Waals corrected DFT study. J Nanostruct Chem 7, 121–132 (2017). https://doi.org/10.1007/s40097-017-0226-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40097-017-0226-5