Introduction

In recent years, the piezoelectric materials and structures have drawn much attention because of their potential advantage in sensing and actuating. They are considered as the most appropriate and perfect solution of many technical issues such as shape and vibration control, buckling control and noise reduction. They are also effectively used for crack detention, damage identification and structural health monitoring purposes. Smart piezolaminated composite beams with self-monitoring and control capabilities are of great technological interest due to the increasing requirements in structural engineering. The self-monitoring and controlling capability of smart beams have numerous applications in shape and vibration control of structures. One main objective of piezoelectric shape control is to optimize control parameters which include the number, location and size of the piezoelectric patches and the amount of electric potential to be applied so that the desired shapes are achieved or best matched. Optimization of such parameters and configurations of piezoelectric actuators for acquiring efficient and precise shape control has been an interesting subject of research in recent years which is investigated in the present work. For this, an accurate mathematical model for shape control is established by considering a critical factor for the performance of the smart beams. The determination of the optimal location of the piezoelectric actuators together with the optimal actuation voltages is worked out with optimization technique.

Various theories for analysis of thick beams were developed in the earlier time. Kirchhoff (1850a, b) developed Classical plate theory which underestimates the deflection and overestimates natural frequencies and buckling loads. Mindlin (1951) developed displacement-based first-order shear deformation theory. In these theories, the transverse shear strain distribution is assumed to be constant through the thickness, and therefore, shear correction factor is required to account for the strain energy due to shear deformation.

In general, these shear correction factors are problem dependent. Crawley and Anderson (1990) studied detailed models of the piezoceramic actuation for beams. Tzou and Tseng (1990) investigated performance of distributed piezoelectric sensor/actuator design for dynamic measurement/control of distributed parameter systems based upon finite element approach. Pan et al. (1992) studied the response of a simply supported beam which is excited by a piezoelectric actuator. Onoda and Hanawa (1993) first time applied and presented improved simulated annealing approach for shape accuracy of space structures using genetic algorithm. Friedman and Kosmatka (1993) used an improved two-node Timoshenko beam finite element.

Thomson and Loughlan (1995) investigated active buckling control of some composite column using piezoceramic actuators. Fuller et al. (1996) studied active control of vibration. Dhonthireddy and Chandrashekhara (1996) worked on modeling and shape control of composite beams with embedded piezoelectric actuators. Chandrashekhara and Varadarajan (1997) stressed upon adaptive shape control of composite beams with piezoelectric actuators. Han et al. (1997) extended work to an experimental study of active vibration control of composite structures with a pizo-ceramic actuator and a piezo-film sensor. Reddy (1997) considered locking-free shear deformable beam finite elements. Aldraihem et al. (1997) studied distributed control of laminated beams with Timoshenko versus Euler–Bernoulli theory. Soares et al. (1999) proposed optimal design of piezolaminated structures. Faria and Almeida (1999) investigated enhancement of pre-buckling behavior of composite beams with geometric imperfections using piezoelectric actuators. Agrawal and Treanor (1999) provided the analytical and experimental results on optimal placement of piezoceramic actuators for shape control of beam structures. The objective achieved was to determine the optimum piezoelectric actuator locations and voltage to minimize the error between the desired shape and the achieved shape.

Aldraihem and Khdeir (2000) studied smart beams with extension and thickness-shear piezoelectric actuators. Bruch et al. (2000) investigated optimal piezo-actuator locations/lengths and applied voltage for shape control of beams. Wang (2002) observed buckling of column structures with a pair of piezoelectric layers. Kang et al. (2002) further put forth interaction of active and passive vibration control of laminated composite beams with piezoceramic sensors/actuators. Irschik Hans (2002) carried out review on static and dynamic shape control of structures by piezoelectric actuation. Kekana et al. (2003) considered shape control model for piezo-elastic structures based on divergence-free electric displacement International. Haupt et al. (2004) investigated practical genetic algorithms. da Mota et al. (2004) proposed the application of genetic algorithms for shape control with piezoelectric patches with an experimental comparison. Hadjigeorgiou et al. (2006) investigated shape control and damage identification of beams using piezoelectric actuation and genetic optimization. Kayacik et al. (2008) developed integral equation approach for piezopatch vibration control of beams with various types of damping. Krommer et al. (2008) proposed design of actuator networks for dynamic displacement tracking of beams. Azrar et al. (2008) performed nonlinear vibration analysis of actively loaded sandwich piezoelectric beams with geometric imperfections. Yu et al. (2009) focused on optimal shape control of a beam using piezoelectric actuators with low control voltage. Dhuri and Seshu (2009) used multi-objective optimization technique for piezo-actuator placement and sizing using genetic algorithm. Kucuk et al. (2011) used optimal vibration control to piezolaminated smart beams by the maximum principle. Bajoria and Wankhade (2012) effectively employed finite element method for free vibration analysis of simply supported plates. Simply supported plates were provided with piezoelectric patches for controlling the response in vibration. Takács et al. (2012) put model predictive vibration control with efficient constrained MPC vibration control for lightly damped mechanical structures. Wankhade and Bajoria (2012) studied stability of simply supported smart piezolaminated composite plates using finite element method. Beheshti-Aval et al. (2013) developed finite element model based on coupled refined high-order global–local theory for static analysis of electromechanical-embedded shear-mode piezoelectric sandwich composite beams with various widths. Wankhade and Bajoria (2013a, b) focused on buckling analysis of piezolaminated plates using higher-order shear deformation theory. Elshafei and Alraiess (2013) studied modeling and analysis of smart piezoelectric beams using simple higher-order shear deformation theory. Wankhade and Bajoria (2013a, b) carried out free vibration and stability analysis of piezolaminated plates using finite element method. Bajoria and Wankhade (2015) further demonstrated vibration of cantilever piezolaminated beam with extension and shear-mode piezo-actuators. Bendine and Wankhade (2016) proposed vibration control of FGM piezoelectric plate based on LQR genetic search. Wankhade and Bajoria (2016) investigated shape control and vibration analysis of piezolaminated plates subjected to electromechanical loading. Wankhade and Bajoria (2017) further proposed numerical optimization of piezolaminated beams subjected to static and dynamic excitations. For these different models of beams with varying geometry and boundary conditions are developed.

In this paper, we present an efficient finite element approach based on shear deformable beam theory for the case of laminated beam with bonded piezoelectric actuators. The developed model is used to investigate the shape control of composite beam with three sets of boundary condition clamped-free (C-F), clamped–clamped (C-C) and simply supported (S-S). The effect of the actuator locations on the beam shape is presented. For final study, the required voltage to maintain a desired shape of beam is optimized using genetic algorithm.

Constitutive equations and mathematical modeling

Displacement function

The displacement field equations at any point through the thickness of the beam based on Timoshenko’s beam theory (Reddy 1997; Hadjigeorgiou et al. 2006; Elshafei and Alraiess 2013) are presented by:

$$u\,\left( {x,z,t} \right)\, = \,z\varphi_{x} \,\left( {x,t} \right)$$
$$w\,\left( {x,z,t} \right)\, = \,\,w_{0} \,\left( {x,t} \right)$$
(1)

where w 0 is the transverse deflection of the mid-plane point, and φ x denotes the rotation angle along the x-axis. Using Eq. (1), the strain components can be expressed as:

$$\varepsilon_{xx} \,\, = \,\,\,z\,\frac{{\partial \varphi_{x} }}{\partial x}$$
$$\gamma_{xz} \,\, = \,\,\varphi_{x} \,\, + \,\,\frac{{\partial w_{0} }}{\partial x}$$
(2)

The strain vector can be rewritten under the following matrix form:

$$\left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\gamma_{xz} } \\ \end{array} } \right\}\, = \,\,\,\left[ {\begin{array}{*{20}c} 0 & {z\frac{\partial }{\partial x}} \\ {\frac{\partial }{\partial x}} & 1 \\ \end{array} } \right]\,\,\left( {\begin{array}{*{20}c} {w_{0} } \\ {\varphi_{x} } \\ \end{array} } \right)$$
(3)

The coupling relationship between the electrical and mechanical behavior (Tzou and Tseng 1990) can be described by

$$\begin{aligned} \left\{ {\begin{array}{*{20}c} {\sigma_{xx} } \\ {\sigma_{yy} } \\ {\sigma_{zz} } \\ {\tau_{yz} } \\ {\tau_{xz} } \\ {\tau_{xy} } \\ {D_{x} } \\ {D_{y} } \\ {D_{z} } \\ \end{array} } \right\}\,\, & = \,\,\left[ {\begin{array}{*{20}c} {Q_{11} } & {Q_{21} } & 0 & 0 & 0 & 0 \\ {Q_{21} } & {Q_{22} } & 0 & 0 & 0 & 0 \\ 0 & 0 & {Q_{33} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {Q_{44} } & 0 & 0 \\ 0 & 0 & 0 & 0 & {Q_{55} } & 0 \\ 0 & 0 & 0 & 0 & 0 & {Q_{66} } \\ 0 & 0 & 0 & 0 & {e_{15} } & 0 \\ 0 & 0 & 0 & {e_{24} } & 0 & 0 \\ {e_{31} } & {e_{32} } & {e_{33} } & 0 & 0 & 0 \\ \end{array} } \right]\,\,\left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\varepsilon_{yy} } \\ {\varepsilon_{zz} } \\ {\gamma_{yz} } \\ {\gamma_{xz} } \\ {\gamma_{xy} } \\ \end{array} } \right\}\,\, \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, & \quad+ \,\,\left[ {\begin{array}{*{20}c} 0 & 0 & { - e_{31} } \\ 0 & 0 & { - e_{32} } \\ 0 & 0 & { - e_{33} } \\ 0 & { - e_{24} } & 0 \\ { - e_{15} } & 0 & 0 \\ 0 & 0 & 0 \\ { \in_{11} } & 0 & 0 \\ 0 & { \in_{22} } & 0 \\ 0 & 0 & { \in_{33} } \\ \end{array} } \right]\,\,\left\{ {\begin{array}{*{20}c} {E_{x} } \\ {E_{y} } \\ {E_{z} } \\ \end{array} } \right\} \hfill \\ \end{aligned}$$
(4)

where σ is the stress vector, D represents the electric displacement components, E denotes the electric field component, Q, e and ∊ are the elastic, the piezoelectric coupling and the dielectric permittivity constants, respectively. In the case of a beam, the following assumptions are made:

The width in the y direction is stress-free and the plane stress assumption is used. Therefore, it is possible to set

$$\sigma_{yy} = \sigma_{zz} = \tau_{yz} = \tau_{xy} = 0$$

The electric field is neglected along the x and y directions (E x  = E y  = 0). Thus, only D z in Eq. (4) is considered.

Therefore the constitutive relation Eq. (4) is reduced to:

$$\left\{ {\begin{array}{*{20}c} {\sigma_{xx} } \\ {\tau_{xz} } \\ {D_{z} } \\ \end{array} } \right\}\, = \,\left[ {\begin{array}{*{20}c} {Q_{11} } & 0 & { - e_{31} } \\ 0 & {Q_{55} } & 0 \\ {e_{31} } & 0 & { \in_{33} } \\ \end{array} } \right]\,\,\left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\gamma_{xz} } \\ {E_{z} } \\ \end{array} } \right\}$$
(5)

Assuming that the voltage is applied only in thickness direction, the electric field vector {E} can be expressed as follows:

$$E_{z} \,\, = \,\,\frac{{V^{e} }}{{h_{a} }}$$
(6)

The Lagrangian ‘Π’ of the laminate beam can be obtained by the summation of the kinetic energy ψ potential energy ϕ (including strain and electrical energies of the piezoelectric):

$$\varPi \,\, = \,\,\int\limits_{v} {\left( {\psi \, - \,\varphi } \right)\,\,{\text{d}}v}$$
(7)

Using Eqs. (1) and (2) and integrating over the cross-section of the beam, the kinetic energy term may be written as

$$\psi = \frac{1}{2}\int\limits_{y} {\left\{ {\begin{array}{*{20}c} {\dot{u}} \\ {\dot{w}} \\ \end{array} } \right\}^{T} \left\{ {\begin{array}{*{20}c} {\dot{u}} \\ {\dot{w}} \\ \end{array} } \right\}{\text{d}}v = \frac{1}{2}\left[ {\int\limits_{0}^{L} {I_{3} \left( {\dot{\varphi }_{x}^{2} } \right) + I_{1} \left( {\dot{w}} \right)^{2} } } \right]{\text{d}}x}$$
(8)

where

$$\left( {I_{ 1} , I_{ 3} } \right) = \int_{s} \rho \left( { 1, z^{ 2} } \right){\text{d}}s$$
(9)

The potential energy ϕ (including strain and electrical energies of the piezoelectric) is expressed as

$$\phi \,\, = \,\,\frac{1}{2}\,\,\int\limits_{v} {\left\{ \varepsilon \right\}^{T} } \left\{ \sigma \right\}{\text{d}}v\,\,\, + \,\,\,\frac{1}{2}\,\,\int\limits_{v} {\left( {\left\{ \varepsilon \right\}^{T} \,\left\{ \sigma \right\}\,\, - \,\,\left\{ E \right\}^{T} \,\left\{ D \right\}} \right)} \,{\text{d}}v$$
(10)

Substituting Eqs. (1), (3), (5) and (6) in Eq. (10), the potential term may be rewritten as

$$\phi \, = \,\,\int\limits_{0}^{L} {\left[ \begin{aligned} & \frac{1}{2}\,\left( {\text{EI}} \right)\,\left( {\frac{{\partial \phi_{x} }}{\partial x}} \right)^{2} \,\,\, + \,\,\,\frac{1}{2}\,\,\left( {\text{GA}} \right)\,\left( {\phi_{x} \, + \,\frac{\partial w}{\partial x}} \right)^{2} \,\, \hfill \\ \,\, - & \,\,M^{\text{el}} \,\frac{{\partial \phi_{x} }}{\partial x}\, - \,\frac{1}{2}\,\left( { \in_{33z} \,E_{z}^{2} } \right) \hfill \\ \end{aligned} \right]\,{\text{d}}x}$$
(11)

with

$$\left( {\text{EI}} \right) = \int\limits_{S} {z^{ 2} Q_{ 1 1} {\text{d}}s}$$
(12.a)
$$\left( {\text{GA}} \right)\,\, = \,\,k\,\int\limits_{S} {Q_{11} \,{\text{d}}s}$$
(12.b)
$$M^{\text{el}} \, = \,\,\,\int\limits_{S} {{\text{ze}}_{31} \,E_{z} \,{\text{d}}s}$$
(12.c)

The virtual work done by the external mechanical forces is given by

$$\delta W_{e} \, = \,\,\,\int\limits_{0}^{{L_{e} }} {\left( {{\text{qw}}\, + \,m\varphi_{x} } \right)}$$
(13)

Based on the equations above, the dynamic equations of the laminated beam structure can be derived using Hamilton’s principle:

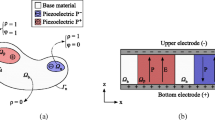
$$\delta \int\limits_{{t_{1} }}^{{t_{2} }} {\left( {\varPi \,\, - \,\,W_{e} } \right)} \,\, = \,\,\,0$$
(14)

In this work, a two-node beam element (Fig. 1) with two mechanical degrees of freedoms at each node is used (Friedman and Kosmatka 1993; Reddy 1997).

Fig. 1
figure 1

Two degrees of freedom element beam

Full size image

The displacement components u and w in Eq. (1) will be approximated using six mechanical nodal variables:

$$w\left( {x,t} \right)\,\,\, = \,\,\,\sum\limits_{i = 1}^{n} {N_{wi} \,\left( x \right)\,\forall_{i} \,\left( t \right)}$$
(15.a)
$$\varphi_{x} \,\left( {x,t} \right)\,\,\, = \,\,\,\sum\limits_{i = 1}^{n} {N_{\phi i} \,\left( x \right)\,\forall_{i} \,\left( t \right)}$$
(15.b)

where (N wi , \(N_{\phi i}\)) are respectively the shape functions (see “Appendix”) and ∀ = [W 1 ϕ x1, W 2 ϕ x2] is element nodal displacements for ith node.

Substituting Eqs. (15.a), (15.b) into Eq. (3), the generalized strain vectors {ɛ} at any point within the element are obtained by

$$\left\{ {\begin{array}{*{20}c} {\varepsilon_{xx} } \\ {\gamma_{xz} } \\ \end{array} } \right\}\,\,\, = \,\,\left[ {\begin{array}{*{20}c} {\left[ {B_{w} } \right]} \\ {\left[ {B_{\phi } } \right]} \\ \end{array} } \right]\,\,\left\{ \forall \right\}$$
(16)

where [B w ] and [B ϕ ] are given in the “Appendix”.

In the proposed model, the electric potential is a function of the length, it can be represented by:

$$V^{e} \,\left( x \right)\,\, = \,\,\sum\nolimits_{i = 1}^{2} {V_{i}^{e} \,\theta_{j} }.$$
(17)

Numerical analysis

Numerical analysis using the developed methodology is performed. The fitness function is developed to investigate the optimal shape of the beams. A flow chart for genetic algorithm is shown in Fig. 2.

Fig. 2
figure 2

Flow chart for genetic algorithm

Full size image

Hence, the electric field intensity can be written as (Yu et al. 2009):

$$E_{z} \,\, = \,\,\frac{1}{{h_{a} }}\,\,\left[ {\begin{array}{*{20}c} {1 - \frac{{x_{e} }}{{L_{e} }}} & {\frac{{x_{e} }}{{L_{e} }}} \\ \end{array} } \right]\,\,\left[ {\begin{array}{*{20}c} {V_{1}^{e} } \\ {V_{2}^{e} } \\ \end{array} } \right]$$
(18)

The shape functions Eqs. (15a, 15b)–(18) are substituted into the kinetic and potential equations. After performing the integration of Hamilton’s principle [Eq. (14)], the dynamic equation of the laminate beam can be expressed as:

$$\left[ {M^{e} } \right]\,\left\{ {\ddot{\forall }} \right\}\, + \,\,\,\left[ {K^{e} } \right]\,\left\{ \forall \right\}\,\, = \,\,\,\left\{ {f_{\text{mec}}^{e} } \right\}\,\, + \,\,\left\{ {f_{\text{elec}}^{e} } \right\}$$
(19)

where {\(\ddot{\forall }\)} and {∀} represent vector of double derivative of displacement and vector of displacement, respectively.

[M e] and represent the element mass and stiffness matrices corresponding to the vector of mechanical displacement.

{f emec } and {f eelec } represent the external mechanical and electric forces (i.e., all the matrices are expressed in “Appendix”).

A fitness function is developed as a sum of the errors at the node points. Chandrashekhara and Varadarajan (1997):

$$J\,\, = \,\,\sum\limits_{i = 1}^{n} {\forall_{i} \,\, - \,\,w_{di} }.$$
(20)

Results and discussions

In the present section, numerical examples which are carried out for the shape control of beam with different boundary conditions, the shape control is provided using five piezoelectric actuators made of PZT G-1195 mounted at the top and bottom surfaces of the beam, see Fig. 3. All actuators are assumed to be perfectly bonded to the beam. The material properties and the geometrical dimensions of the beam and piezoelectric actuators are listed in Table 1.

Fig. 3
figure 3

A beam with surface-bonded piezoelectric actuators

Full size image
Table 1 Material properties
Full size table

In the first analysis case, a composite beam (300 mm × 40 mm × 10 mm) with five distributed piezoelectric patches on the top and the bottom of the beam with a thickness of 0.2 mm serving as actuators. Three cases of boundary conditions are considered (clamped-free (C-F), clamped–clamped (C-C), and simply supported (S-S). At first, we considered a clamped-free beam subjected to a fixed point loading equal to 4 N at the free end at y = b/2. Each actuator is subject to constant actuation voltage of 200 V.

The effect of the actuators position on the beam shape is studied for each single actuator. Each time one actuator has been activated, from Fig. 4 we noticed that the effect of actuation on beam shape is significant when the actuator is closer to the free end of the beam. The results were in good agreement with those presented by (Yuet al. 2009).

Fig. 4
figure 4

The midline deflection of the C-F beam for each actuator position with F = − 4, V = 200 v

Full size image

In the second example, we studied a clamped–clamped beam subjected to a fixed point loading equal to 4 N acting downward at the mid span of the beam (x = a/2, y = b/2). Each actuator is subject to constant actuation voltage of 200 V, as it can be seen in Fig. 5, the actuators are succeeded to reduce the shape of the beam, and the shape control is more significant when the actuator position is far away from the centre on the beam.

Fig. 5
figure 5

The midline deflection of the C-C beam for each actuator position with F = − 4, V = 200 v

Full size image

It is also seen that deflection is symmetrical means, the position of the actuators in the left and in the right of the applied force gives the same shape of the beam.

The last example in this case, a simply supported beam subjected to a fixed point loading equal to 4 N acting downward at the mid span of the beam (x = a/2, y = b/2) is considered. Each actuator is subject to constant actuation voltage of 200 V as it can be seen in Fig. 6, the same notes as the case of S-S beam can be noticed.

Fig. 6
figure 6

The midline deflection of the S-S beam for each actuator position with F = − 4, V = 200 v

Full size image

The problem in shape control is to find the number and the required piezoelectric actuator voltage to maintain the desired shape. Several fitness functions are developed in the literature and in present paper based on the errors between the desired shape and the achieved shape. A fitness function is developed as a sum of the errors at the node points. Chandrashekhara and Varadarajan (1997):

$$J\,\, = \,\,\sum\limits_{i = 1}^{n} {\forall_{i} \,\, - \,\,w_{di} }$$
(20)

where w d is the desired displacement of the ith node and ∀ i is the actual displacement.

The genetic algorithms (Haupt et al. 2004) are the most known and efficient method for the optimization problems, it is based on four key functions; selection, crossover and mutation and elitism. In the present study, the genetic algorithm is used to minimize the fitness function of Eq. (20) for the goal of finding the required voltage for a desired shape of the beam. The optimization procedure is performed in Matlab with following configuration: population size: 150, crossover rate: 0.4, generation number: 50. The actuator voltage limit is taken as −200 < V i  < + 200.

Beam dimensions

In the second analysis case, the numerical study is carried out for the case of composite beam with dimensions of 500 mm × 40 mm × 10 mm. The beam is discretized into 30 elements. Each six element is covered by one actuator. The material proprieties are the same as the first case and it is given in Table 1. Actuator voltage and fitness function with respect to different boundary conditions are shown in Table 2. Responses obtained are shown in Figs. 7, 8, 9, respectively, for different support conditions of beam.

Table 2 Actuator voltage and fitness function for different boundary conditions
Full size table
Fig. 7
figure 7

The desired and achieved shape for the C-F beam

Full size image
Fig. 8
figure 8

The desired and achieved shape for the C-C beam

Full size image
Fig. 9
figure 9

The desired and achieved shape for the S-S beam

Full size image

In the third analysis case, the numerical study is carried out for the case of composite beam with dimensions of 600 mm × 40 mm × 10 mm. The beam is discretized into 30 elements. Each six element is covered by one actuator. The material proprieties are the same as the first case and it is given in Table 1. For this beam Actuator voltage and fitness function with respect to different boundary conditions are shown in Table 3.

Table 3 Actuator voltage and fitness function for different boundary conditions
Full size table

Figure 10 presents the desired and the achieved shape of the C-F beam. In this case, the desired deflection is taken as \(W_{d} = 1e^{ - 5} \times ( 1 - { \cos } \left( {\pi x/L} \right)\) where L is the length of the beam. The required voltage and the fitness function value obtained from the GA optimization are presented in Table 3. It can be noticed that the required actuator voltage decreases as the actuator position is far away from the clamped edge.

Fig. 10
figure 10

The desired and achieved shape for the C-F beam

Full size image

As a second example, a C-C beam is considered. The desired shape is assumed to be \(W_{d} = 1e^{ - 5} \times ( { \cos } \left( { - \pi / 2 + \pi x/L} \right)\).

The required voltage and the corresponding fitness obtained by GA optimization technique are obtained. Hence, the achieved shape corresponding to those voltages is shown in Fig. 11. As it can be seen for the Figs. 11 and 12. A good response is observed between the achieved and desired shape.

Fig. 11
figure 11

The desired and achieved shape for the C-C beam

Full size image
Fig. 12
figure 12

The desired and achieved shape for the S-S beam

Full size image

In the last example, a simply supported (S-S) beam is considered. The desired shape is taken to be \(W_{d} = 1e^{ - 5} \times { \sin } \left( { 3\pi \times \pi x/L} \right)\).

The required voltage obtained by GA optimization technique is presented in Table 3 for this also. The achieved shape corresponding to those voltages is shown in Fig. 11. As it can be seen from Fig. 11, a good response is obtained between the achieved and desired shape.

Conclusions

In the present paper, a methodology using finite element and genetic algorithms is proposed to investigate the shape control of composite beam with surface-mounted piezoelectric actuators. Three different geometry of beams are examined for the optimal shape control with different boundary condition and loading using presented genetic algorithm technique. The numerical investigation reveals the following points:

  1. 1.

    For the case of C-F beam, the effect of actuation on beam shape is significant when the actuator is closer to the free end of the beam. For the case of C-C and S-S beam, the shape control is more significant when the actuator position is far away from the centre of the beam it is also seen that deflection is symmetrical.

  2. 2.

    An optimization of the required voltage actuation to achieve a desired shape is developed based on genetic algorithm; different cases of a desired function are presented for the case of composite beam with three boundary conditions.