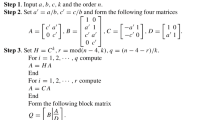Abstract
We show that the characteristic polynomial of a symmetric pentadiagonal Toeplitz matrix is the product of two polynomials given explicitly in terms of the Chebyshev polynomials.

Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Barrera, M.; Grudsky, S.M.: Asymptotics of eigenvalues for pentadiagonal symmetric Toeplitz matrices. Oper. Theory Adv. Appl. 259, 179–212 (2017)
Chu, M.T.; Diele, F.; Ragnion, S.: On the inverse problem of constructing symmetric pentadiagonal Toeplitz matrices from three largest eigenvalues. Inverse Probl. 21, 1879–1894 (2005)
Doman, B.G.S.: The Classical Orthogonal Polynomials. World Scientific Publishing Company, Singapore (2015)
Elouafi, M.: A widom like formula for some Toeplitz plus Hankel determinants. J. Math. Anal. Appl. 422(1), 240–249 (2015)
Elouafi, M.: An eigenvalue localization theorem for pentadiagonal symmetric Toeplitz matrices. Linear Algebra Appl. 435, 2986–2998 (2011)
Elouafi, M.: A note for an explicit formula for the determinant of pentadiagonal and heptadiagonal symmetric Toeplitz matrices. Appl. Math. Comput. 219(9), 4789–4791 (2013)
Elouafi, M.: On a relationship between Chebyshev polynomials and Toeplitz determinants. Appl. Math. Comput. 229(25), 27–33 (2014)
Fasino, D.: Spectral and structural properties of some pentadiagonal symmetric matrices. Calcolo 25, 301–310 (1988)
Grenander, U.; Szeg ö, G.: Toeplitz Forms and their Applications. Chelsea, New York (1984)
Jia, J.T.; Yang, B.T.; Li, S.M.: On a homogeneous recurrence relation for the determinants of general pentadiagonal Toeplitz matrices. Comput. Math. Appl. 71, 1036–1044 (2016)
Mason, J.C.; Handscomb, D.: Chebyshev Polynomials. Chapman & Hall, New York (2003)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Elouafi, M. On formulae for the determinant of symmetric pentadiagonal Toeplitz matrices. Arab. J. Math. 7, 91–99 (2018). https://doi.org/10.1007/s40065-017-0194-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40065-017-0194-0