Skip to main content
SpringerLink
Log in

Exact Similarity Solution for the Propagation of Spherical Shock Wave in a van der Waals Gas with Azimuthal Magnetic Field, Radiation Heat Flux, Radiation Pressure and Radiation Energy Under Gravitational Field

  • Research Article
  • Published:
Proceedings of the National Academy of Sciences, India Section A: Physical Sciences Aims and scope Submit manuscript

Abstract

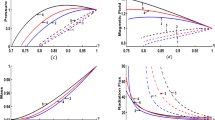
We investigated the exact similarity solutions for shock wave propagation in non-ideal gas with radiation heat flux under gravitational field by taking radiation pressure and radiation energy into account in the presence of azimuthal magnetic field for spherical geometry. The solutions are in terms of analytical expressions. Similarity method is used to transform the basic equation from a system of partial differential equation into a system of ordinary differential equations. The system of ODE yields exact solutions for initial magnetic field distributions as power law. Consideration of isothermal approximations, radiation pressure, radiation energy and adiabatic compressibility in gravitating medium into accounts leads to new insights into explosion problem in contrast to earlier model. Finally, the product solution of progressive wave given by Mc. Vittie is used to obtain the exact similarity solution under the consideration that the radiation pressure is not equal to zero and the energy loss due to radiation escape is significant. The Alfven–Mach number effect, the parameter of gravitational effect, the parameter of non-idealness of the gas, the radiation pressure number and the adiabatic exponent gamma of the gas are discussed in detail.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Sedov LI (1946) Propagation of strong shock waves. J Appl Math Mech 10:241–250

    Google Scholar 

  2. Taylor G (1950) The formation of a blast wave by a very intense explosion. I. Theoretical discussion. Proc R Soc Lond Ser A 201:159–174

    ADS  MATH  Google Scholar 

  3. Noh WF (1987) Errors for calculations of strong shocks using an artificial viscosity and an artificial heat flux. J Comput Phys 72:78–120

    ADS  MATH  Google Scholar 

  4. Meyer HWJ (1988) Derivation of a Godunov one dimensional fluid dynamics code, technical report, Army Ballistic Research Lab Aberdeen Proving Ground MD

  5. Gehmeyr M, Cheng B, Mihalas D (1997) Noh’s constant-velocity shock problem revisited. Shock Waves 7:255–274

    ADS  MATH  Google Scholar 

  6. Allen GE, Chow K, DeLaney T, Filipovic MD, Houck JC, Pannuti TG, Stage MD (2015) On the expansion rate, age, and distance of the supernova remnant G266.2-1.2 (Vela Jr.). Astrophys J 798:82

    ADS  Google Scholar 

  7. Leahy DA, Ranasinghe S (2016) Distance and evolutionary state of the supernova remnant 3C 397 (G41.1-0.3). Astrophys J 817:74

    ADS  Google Scholar 

  8. Lerche I, Vasyliunas VM (1976) Mathematical theory of isothermal blast waves and the question of their applicability to supernova remnants. Astrophys J 210:85–99

    ADS  Google Scholar 

  9. Solinger A, Buff J, Rappaport S (1975) Isothermal blast wave model of supernova remnants. Astrophys J 201:381–386

    ADS  Google Scholar 

  10. Vink J (2012) Supernova remnants: The X-ray perspective. Astron Astrophys Rev 20:49. https://doi.org/10.1007/s00159-011-0049-1

    Article  ADS  Google Scholar 

  11. Woltjer L (1972) Supernova remnants. Ann Rev Astron Astrophys 10:129–158

    ADS  Google Scholar 

  12. Sedov LI (1959) Similarity and dimensional methods in mechanics. Academic Press, New York

    MATH  Google Scholar 

  13. Korobeinikov VP (1956) The problem of a strong point explosion in a gas with zero temperaturegradient. Dokl Akad Nauk 109:271–273

    Google Scholar 

  14. Korobeinikov VP (1976) Problems in the Theory of Point Explosion in Gases. American Mathematical Society, Providence

    Google Scholar 

  15. Zel’dovich Ya B, Raizer YuP (1967) Physics of shock waves and high temperature hydrodynamic phenomena, vol II. Academic Press, New York

    Google Scholar 

  16. Lerche I (1977) Isothermal self-similar blast wave theory of supernova remnants driven by relativistic gas pressure. Astrophys Space Sci 50:323–342

    ADS  MATH  Google Scholar 

  17. Stephan C, Deschner Y, Tobias F, Illenseer Y, Wolfgang J, Duschl Z (2018) Self-similarity solutions to isothermal shock problems, SIAM. J Appl Maths 78:80–103

    MATH  Google Scholar 

  18. Sponholz H, Molteni D (1994) Steady-state shocks in an accretion disc around a Kerr black hole. MNRAS 271:233–242

    ADS  Google Scholar 

  19. Kato S, Inagaki S, Mineshige S, Fukue J (1996) Physics of accretion disks. Gordon and Breach, Amsterdam

    Google Scholar 

  20. Kato S, Fukue J, Mineshige S (1998) Black-hole accretion disks. Kyoto Univ. Press, Kyoto

    Google Scholar 

  21. Abramowicz MA, Chakrabarti SK (1990) Standing shocks in adiabatic black hole accretion of rotating matter. ApJ 350:281–287

    ADS  Google Scholar 

  22. Yang R, Kafatos M (1995) Shock study in fully relativistic isothermal flows, 2. A&A 295:238–244

    ADS  Google Scholar 

  23. Lu JF, Yuan F (1997) Isothermal Shocks in adiabatic black-hole accretion flows. PASJ: Publ Astron Soc Jpn 49:525–533

    ADS  Google Scholar 

  24. Lu JF, Yuan F (1998) Global solution of adiabatic accretion flows with isothermal shocks in Kerr black hole geometry. Mon Not R Astron Soc 295:66–74

    ADS  Google Scholar 

  25. Fukumura K, Tsuruta S (2004) Isothermal shock formation in nonequatorial accretion flows around Kerr black holes. APJ 611:964–976

    ADS  Google Scholar 

  26. Carrus P, Fox P, Hass F, Kopal Z (1951) The propagation of shock waves in a stellar model with continuous density distribution. Astrophys J 113:496–518

    ADS  MathSciNet  Google Scholar 

  27. Rogers MH (1957) Analytic solutions for blast wave problem with an atmosphere of varying density. Astrophys J 125:478–493

    ADS  MathSciNet  Google Scholar 

  28. Summers D (1975) An idealized model of a magnetohydrodynamic spherical blast wave applied to a flare produced shock in the solar wind. Astron. Astophys. 45:151–158

    ADS  Google Scholar 

  29. Lee TS, Chen T (1968) Hydrodynamic interplanetary shock waves. Planet Space Sci 16:1483–1502

    ADS  Google Scholar 

  30. Wang KC (1964) The piston problem with thermal radiation. J Fluid Mech 20:447–455

    ADS  MathSciNet  MATH  Google Scholar 

  31. Vishwakarma JP, Shrivastava RC, Kumar A (1987) An exact similarity solution in radiation magneto gas dynamics for the flows behind a spherical shock. Astrophys Space Sci 129:45–52

    ADS  MATH  Google Scholar 

  32. Marshak RE (1958) Effects of radiation on shock wave behavior. Phys Fluids 1:24–29

    ADS  MathSciNet  MATH  Google Scholar 

  33. Elliot LA (1960) Similarity methods in radiation hydrodynamics. Proc R Soc 258A:287–301

    ADS  MathSciNet  Google Scholar 

  34. Sachdev PL, Ashraf S (1970) Exact similarity solution in radiation-gas-dynamics. Proc Indian Acad Sci A71:275–281

    Google Scholar 

  35. Verma BG, Vishwakarma JP (1978) An exact similarity solution for spherical shock wave in magnetoradiative gas. Astrophys Space Sci 58:139–147

    ADS  Google Scholar 

  36. Rosenau P (1977) Equatorial propagation of axisymmetric magnetohydrodynamic shocks II. Phys Fluids 20:1097–1103

    ADS  Google Scholar 

  37. Shaviv NJ (2000) The porous atmosphere of η Carinae. Astrophys J 532:L137–L140

    ADS  Google Scholar 

  38. Pegoraro F, Bulanov SV (2007) Photon Bubbles and ion acceleration in a plasma dominated by the radiation pressure of an electromagnetic pulse. Phys Rev Lett 99:065002

    ADS  Google Scholar 

  39. Arons J (1992) Photon bubbles: overstability in a magnetized atmosphere. Astrophys J 388:561

    ADS  Google Scholar 

  40. Esirkepov T, Borghesi M, Bulanov SV, Mourou G, Tajima T (2004) Highly efficient relativistic-ion generation in the laser-piston regime. Phys Rev Lett 92:175003

    ADS  Google Scholar 

  41. Macchi A, Cattani F, Liseykina TV, Cornolti F (2005) Laser acceleration of ion bunches at the front surface of over-dense plasmas. Phys Rev Lett 94:165003

    ADS  Google Scholar 

  42. Goldreich P (1978) On the radiative acceleration of quasar absorption line clouds. Phys Scr 17:225

    ADS  Google Scholar 

  43. Landau LD, Lifshitz EM (1975) The classical theory of fields. Pergamon Press, New York

    MATH  Google Scholar 

  44. Borghesi M, Fuchs J, Bulanov SV, MacKinnon AJ, Patel PK, Roth M (2006) Fast ion generation by high-intensity laser irradiation of solid targets and applications. Fus Sci Technol 49:412–439

    Google Scholar 

  45. Moon SJ, Wilks SC, Klein RI, RemingtonB A, Ryutov DD, Mackinnon AJ, Patel PK, Spitkovsky A (2005) A neutron star atmosphere in the laboratory with petawatt lasers. Astrophys Space Sci 298:293–298

    ADS  MATH  Google Scholar 

  46. Mourou GA, Tajima T, Bulanov SV (2006) Optics in the relativistic regime. Rev Mod Phys 78:309–371

    ADS  Google Scholar 

  47. Kifonidis K, Plewa T, Janka HTh, Müller E (2003) Non-spherical core collapse supernovae I. Neutrino-driven convection, Rayleigh-Taylor instabilities, and the formation and propagation of metal clumps. Astron Astrophys 408:621–649

    ADS  Google Scholar 

  48. Robinson APL, Zepf M, Kar S, Evans RG, Bellei C (2008) Radiation pressure acceleration of thin foils with circularly polarized laser pulses. New J Phys 10:013021

    Google Scholar 

  49. Tamburini M, Pegoraro F, Piazza A, Di Keitel CH, Macchi A (2010) Radiation reaction effects on radiation pressure acceleration. New J Phys 12:123005

    Google Scholar 

  50. Badziak J, Glowacz S, Jablonski S, Parys P, Wolowski J, Hora H, Krása J, Láska L, Rohlena K (2004) Production of ultrahigh ion current densities at skin-layer subrelativistic laser-plasma interaction. Plasma Phys Control Fus 46(B):541–556

    Google Scholar 

  51. Kar S et al (2008) Plasma jets driven by ultraintense-laser interaction with thin foils Phys. Rev Lett 100:225004

    ADS  Google Scholar 

  52. Akli KU et al (2008) Laser heating of solid matter by light-pressure-driven shocks at ultrarelativistic intensities Phys. Rev Lett 100:165002

    ADS  Google Scholar 

  53. Macchi A, Veghini S, Tatyana V (2010) Liseykina and francesco pegoraro, radiation pressure acceleration of ultrathin foils. New J Phys 12:045013

    Google Scholar 

  54. Krumholz MR, Christopher DM (2009) The dynamics of radiation pressure dominated H-II regions. Astrophys J 703:1352–1362

    ADS  Google Scholar 

  55. Ashkin A (1970) Acceleration and trapping of particles by radiation pressure. Phys Rev Lett 24:156–159

    ADS  Google Scholar 

  56. Letokhov VS (1977) Laser control of atomic motion: velocity selection, cooling and trapping. Comments At Mol Phys 6:119–131

    Google Scholar 

  57. Wineland DJ, Drullinger HE, Walls FL (1978) Radiation pressure cooling of bound resonant absorbers Phys. Rev Lett 40:1639–1642

    ADS  Google Scholar 

  58. Ashkin A (1980) Applications of laser radiation pressure. Science 210:1081–1088

    ADS  Google Scholar 

  59. Vishwakarma JP, Nath G (2010) Propagation of a cylindrical shock wave in a rotating dusty gas with heat conduction and radiation heat flux. Phys Scr 81:045401

    ADS  MATH  Google Scholar 

  60. Vishwakarma JP, Nath G (2012) Spherical shock wave generated by a moving piston in mixture of a non-ideal gas and small solid particles under a gravitational field. Commun Nonlinear Sci Numer Simul 17:2382–2393

    ADS  MathSciNet  MATH  Google Scholar 

  61. Anisimov SI, Spiner OM (1972) Motion of an almost ideal gas in the presence of a strong point explosion. J Appl Maths Mech 36:883–887

    Google Scholar 

  62. Ranga Rao MP, Purohit NK (1976) Self-similar problem in non-ideal gas. Int J Eng Sci 14:91–97

    MATH  Google Scholar 

  63. Wu CC, Roberts PH (1993) Shock wave propagation in a sonoluminescing gas bubble. Phys Rev Lett 70:3424–3427

    ADS  Google Scholar 

  64. Roberts PH, Wu CC (1996) Structure and stability of a spherical implosion. Phys Lett A 213:59–64

    ADS  Google Scholar 

  65. Nath G (2015) Similarity solutions for unsteady flow behind an exponential shock in an axisymmetric rotating non-ideal gas. Meccanica 50:1701–1715

    MathSciNet  MATH  Google Scholar 

  66. Vishwakarma JP, Nath G (2007) Similarity solutions for the flow behind an exponential shock in a non-ideal gas. Meccanica 42:331–339

    MathSciNet  MATH  Google Scholar 

  67. Vishwakarma JP, Nath G (2009) A self-similar solution of a shock propagation in a mixture of a non-ideal gas and small solid particles. Meccanica 44:239–254

    MATH  Google Scholar 

  68. Nath G, Dutta M, Pathak RP (2017) An exact solution for the propagation of shock waves in selfgravitating medium in the presence of magnetic field and radiative heat flux. Model Measur Control B 86(4):907–927. https://doi.org/10.18280/mmc_b.860406

    Article  Google Scholar 

  69. Rosenau P, Frankenthal S (1976) Shock disturbances in a thermally conducting solar wind. Astrophys J 208:633–637

    ADS  Google Scholar 

  70. Rosenau P, Frankenthal S (1978) Propagation of magnetohydrodynamic shocks in a thermally conducting medium. Phys Fluids 21:559–566

    ADS  Google Scholar 

  71. Helliwell JB (1969) Self-similar piston problems with radiative heat transfer. J Fluid Mech 37:497–512

    ADS  MATH  Google Scholar 

  72. Vishwakarma JP, Pandey VK (2013) Magnetogasdynamic spherical shock waves in a non- ideal gas with radiation. Int J Appl Math Mech 9:91–102

    Google Scholar 

  73. Bhowmick JB (1981) An exact analytical solution in radiation gasdynamics. Astrophys Space Sci 74:481–485

    ADS  MathSciNet  MATH  Google Scholar 

  74. Mc Vittie G C (1953) Spherically symmetric solutions of the equations of gas dynamics. Proc Roy Soc 220:339–455

    ADS  MathSciNet  Google Scholar 

  75. Nath G, Vishwakarma JP, Shrivastava VK, Sinha AK (2013) Propagation of magnetogasdynamic shock waves in a self-gravitating gas with exponentially varying density. J Theor Appl Phys 7:1–8

    Google Scholar 

  76. Whitham GB (1958) On the propagation of shock waves through regions of non-uniform area or flow. J Fluid Mech 4:337–360

    ADS  MathSciNet  MATH  Google Scholar 

  77. Landau LD, Lifshitz EM (1958) Course of theoretical physics, statistical physics, vol 5. Pergamon Press, Oxford

    Google Scholar 

  78. Chandrasekhar S (1939) An introduction to the study of stellar structure. University Chicago Press, Chicago

    MATH  Google Scholar 

  79. Pai SI (1969) Inviscid flow of radiation gasdynamics (High temperature inviscid flow of ideal radiating gas, analyzing effects of radiation pressure and energy on flow field). J Math Phys Sci 3:361–370

    MATH  Google Scholar 

  80. Vishwakarma JP, Patel N (2015) Magnetogasdynamic cylindrical shock waves in a rotating non-ideal gas with radiation heat flux. J Eng Phys Thermophys 88:521–530

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Motilal Nehru National Institute of Technology Allahabad, Allahabad, Uttar Pradesh, 211004, India

    G. Nath

  2. Department of Mathematics, National Institute of Technology, Raipur, G. E. Road, Raipur, Chhattisgarh, 492001, India

    Mrityunjoy Dutta & R. P. Pathak

Authors
  1. G. Nath
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mrityunjoy Dutta
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. R. P. Pathak
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. Nath.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Significance statement

Exact similarity solution for magnetogasdynamic shock waves in non-ideal gas with radiation flux under gravitational field with radiation pressure and radiation energy is studied. The product solution of progressive wave given by Mc. Vittie is used to obtain the exact solution. The radiation pressure is taken into consideration. The radiation pressure is used for cooling such as radiation-pressure cooling of bound resonant absorbers which is elastically bound to laboratory fixed equipment in optical levitation of liquid drops. Other major application of radiation pressure is in the study of the light scattering.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nath, G., Dutta, M. & Pathak, R.P. Exact Similarity Solution for the Propagation of Spherical Shock Wave in a van der Waals Gas with Azimuthal Magnetic Field, Radiation Heat Flux, Radiation Pressure and Radiation Energy Under Gravitational Field. Proc. Natl. Acad. Sci., India, Sect. A Phys. Sci. 90, 789–801 (2020). https://doi.org/10.1007/s40010-019-00625-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40010-019-00625-4

Keywords

Search

Navigation