1 Introduction

Recently, the study of the approximation properties of the sampling Kantorovich (SK) operators (that we will denote by \(K^\chi _w\), where \(\chi \) is the kernel function and \(w>0\)) turned out to be very interesting (see, e.g., [2, 15, 18, 40]), in particular in view of their positive implications on the real world applications based on image analysis and enhancement (see, e.g., [11, 41]). Concerning the theoretical and applications properties of such operators, several papers are available in the literature; in the references of this paper, only a very little selection of them have been quoted.

In the recent paper [23], it has been proved that (see Theorem 4.2) the SK operators based upon certain kernel function \(\chi \) and for \(f \in L^p(\mathbb {R})\), \(1 \le p \le +\infty \), belong to the classical Sobolev spaces. In order to establish such a regularity theorem, a crucial role was played by a so-called Strang-Fix type condition, a very common assumption in Approximation Theory and Fourier Analysis, when certain classes of problems are faced (see, e.g., [19,20,21]).

Motivated by the above theorem, it has been quite natural to ask if, considering functions f in the (usual) Sobolev spaces \(W^{n,p}(\mathbb {R})\), \(n \in \mathbb {N}^+\), \(1 \le p \le +\infty \), and \(\chi \in W^{n,1}(\mathbb {R})\) satisfying suitable conditions, the family \(K^\chi _w f \rightarrow f\), as \(w \rightarrow +\infty \), with respect to the usual norm of \(W^{n,p}(\mathbb {R})\). The answer of the above question is positive, and the corresponding proof has been given in Section 3 herein. One of the main consequence of the above approximation result, is that it allows to solve certain open problems related to the question of the "convergence in variation" of the SK operators considered in [4]. In fact, in [4] the convergence with respect to the total variation of the SK operators was obtained only in two special cases: for band-limited kernels and for kernels that are not necessarily band-limited but that are represented in the so-called averaged form (see eqn. (3.3)). Here, we establish the convergence in variation in case of kernels with compact support, that are not (necessarily) of the previous considered forms.

Furthermore, based on the recent development of several generalizations of the notion of Sobolev spaces in the fractional-sense, and on the importance of the latter settings in several fields of mathematical analysis, we considered the problem of the convergence of SK operators in such abstract context.

The possibility to have a constructive approximation process in fractional type spaces can also open the way to consider new applications to numerical methods, for the approximate solutions of pdes and integral-type equations, or to the implementation of algorithms for digital image processing (see, e.g., [7, 10, 16]).

It is well-known that, in the literature, several notions of fractional Sobolev spaces are available, such as, the Gagliardo Sobolev spaces (GSs) defined by means of the Gagliardo semi-norm (see, e.g., [3]), the Bessel potential space, (see, e.g., [3, 28]) the weak Riemann-Liouville Sobolev spaces (wRLSs) defined by the weak (left and right) Riemann-Liouville fractional derivatives ( [31, 32]), the (bilateral) Riemann–Liouville Fractional Sobolev spaces ( [6, 38, 39]) and so on.

Here, in order to face the above convergence problem, we introduced a new (at least from what we know) definition of fractional Sobolev spaces, that we called the tight fractional Sobolev spaces (tfSs), \({\mathcal {W}}^{s,p}(\mathbb {R})\), \(s \in (0,1)\), \(1 \le p \le +\infty \), generated as the intersection of the GSs and the symmetric fractional Sobolev spaces (i.e., that given by the intersection of the left and the right wRLSs).

We made this choice since the GSs spaces have been deeply studied from both mathematical and applicative perspectives (see, e.g., [17, 26, 29, 30]) and then they are important; on the other hands, in the fractional Sobolev spaces wRLSs we have at disposal a theory with several computational and theoretical tools that revealed to be very suitable in order to face the above approximation problem.

In the tight Sobolev spaces we introduced a suitable norm, that we denote by \(\Vert \cdot \Vert _{{\mathcal {W}}^{s,p}}\), substantially defined by the sum of norms of GSs and symmetric fractional Sobolev spaces. The latter choices have been motivated by technical reasons and seems to be not restrictive, since recently has been conjectured (see Remark 4.57 of [32]) that the GSs and the symmetric fractional Sobolev spaces coincide in \(\mathbb {R}\).

For instance, in order to better understand the main peculiarity of the tight Sobolev spaces, we proved that the space \(C^\infty _0(\mathbb {R})\) of the smooth functions f with compact support is included in \({\mathcal {W}}^{s,p}(\mathbb {R})\), and it is also dense with respect to the introduced norm \(\Vert \cdot \Vert _{{\mathcal {W}}^{s,p}}\) (see Section 4 herein).

In Section 5, we proved the convergence of the SK operators in the new setting of tight fractional Sobolev spaces. In order to reach the above aim, we proceed using density arguments. First we establish a continuity inequality for the \(\Vert \cdot \Vert _{{\mathcal {W}}^{s,p}}\) norm of the SK operators, from which we can deduce that \(K_w^\chi \) maps \({\mathcal {W}}^{s,p}(\mathbb {R})\) into itself. Then, the convergence of the family \(K^\chi _w f\) to f with respect to the above norm, in case of functions f belonging to the space \(C^\infty _0(\mathbb {R})\), is established. Finally, exploiting the density of the space \(C^\infty _0(\mathbb {R})\) in \({\mathcal {W}}^{s,p}(\mathbb {R})\), we get the desired result.

2 Preliminary results

In what follows, we denote by

$$\begin{aligned} W^{n, p}(\mathbb {R})\ :=\ \left\{ f \in L^p(\mathbb {R}):\ f^{(i)} \in L^p(\mathbb {R}),\ 1\le i\le n,\ i \in \mathbb {N}\right\} ,\, 1\ \le \ p\ \le \ +\infty , \end{aligned}$$

\(n \in \mathbb {N}^+\), the (usual) Sobolev spaces endowed with the norm \(\Vert f\Vert _{n,p}:=\Vert f\Vert _p+\Vert f^{(n)}\Vert _p\), where all the derivatives \(f^{(i)}\) must be intended in the weak sense.

Before to start, we need to recall a fundamental inequality present in [35], Theorem 202.

Theorem 1

(General Minkowski inequality) Let f be a measurable function. Assume that the symbol \(\sum \) can be interpreted as a series or a finite sum, and the symbol \(\int \) means that the domain of integration is arbitrary. Then, for every \(k \ge 1\), we have

$$\begin{aligned} \left[ \int \left( \int f\left( x,y\right) dy\right) ^{k}dx\right] ^{1/k}\le \int \left( \int f^{k}\left( x,y\right) dx\right) ^{1/k}dy \end{aligned}$$

unless \(f\equiv \phi \left( x\right) \psi \left( y\right) \), where the inequality must to be interpreted in the sense that if the right-hand side of any inequality is finite, then so it is the left-hand side, and the two are related as started.

In order to introduce the definitions of the operators studied in this paper, we first recall the following notations.

We will say that a function \(\chi :\mathbb {R\rightarrow \mathbb {R}}\) is a \(n-\)kernel if there exists a \(n\in \mathbb {N}^{+}\) such that the following assumptions are satisfied:

\(\chi \)1)\(\chi \in W^{n,1}(\mathbb {R})\) is bounded, \(\text {supp}\, \chi \subseteq [-T, T]\), \(T>0\);

\(\chi \)2) the discrete algebraic moment of order 0, i.e.:

$$\begin{aligned} m_{0}(\chi ,u):=\sum _{k\in \mathbb {Z}}\chi (u-k)=1,\,\forall u\in \mathbb {R}; \end{aligned}$$

\(\chi 3)\) all the discrete algebraic moments of order \(\nu \in [1,n]\cap \mathbb {N}\) are constants, that is:

$$\begin{aligned} m_{\nu }(\chi ,u):=\sum _{k\in \mathbb {Z}}(k-u)^{\nu } \chi (u-k)=:A_{\nu }^{\chi }\in \mathbb {R},u\in \mathbb {R}, \end{aligned}$$

and if \(n>1\) the following additional conditions hold

$$\begin{aligned} \sum _{\ell =0}^{j}\genfrac(){0.0pt}0{j}{\ell }\frac{A_{j-\ell }^{\chi }}{\ell +1} =0,\,j=1,\dots ,n-1,\qquad \sum _{\ell =0}^{n}\genfrac(){0.0pt}0{n}{\ell }\frac{A_{n-\ell }^{\chi }}{\ell +1}\ne 0; \end{aligned}$$
(2.1)

\(\chi 4)\) all the following discrete absolute moments of order zero are finite, namely

$$\begin{aligned} M_0\left( \chi ^{(i)}\right) \ :=\ \sup _{u \in \mathbb {R}} \sum _{k \in \mathbb {Z}}|\chi ^{(i)}(u-k)|\ <\ +\infty ,\ \quad i=1, ..., n, \end{aligned}$$

where \(\chi ^{(i)}\) denote the i-th weak derivative of \(\chi \).

Remark 1

Note that, condition (2.1) is a kind of Strang-Fix type assumption (see [34]), very common in Approximation Theory and Fourier Analysis. More precisely, such condition has been introduced in the present form in [23]. Moreover, even in [23] several examples of kernels satisfying all the above conditions have been presented.

Remark 2

In view of assumption \(\chi 1)\), it is quite easy to see that all the discrete absolute moments of \(\chi \) are finite, i.e.,

$$\begin{aligned} M_\nu (\chi )\ :=\ \sup _{u \in \mathbb {R}} \sum _{k \in \mathbb {Z}} |\chi (u-k)|\, |u-k|^\nu \ <\ +\infty , \end{aligned}$$

for every \(\nu \ge 0\) (see Lemma 2.1 of [22]). In addition, since \(\chi \) has compact support, if its weak derivatives are bounded, we immediately get \(M_0(\chi ^{(i)})<+\infty \), \(i=1,...,n\), as observed above.

Now, we are able to recall the definition of the well-known sampling Kantorovich (SK) operators ( [5]). Let \(\chi \) be a given n-kernel, and let \(f:\mathbb {R}\rightarrow \mathbb {R}\) be a locally integrable function, we define

$$\begin{aligned} (K^\chi _w f)(x)\ :=\ \sum _{k \in \mathbb {Z}}\left[ w \int _{k/w}^{(k+1)/w}f(u)\, du \right] \, \chi (wx-k), \quad w>0. \end{aligned}$$
(2.2)

Remark 3

The definition of the SK operators can be given under weaker assumptions on \(\chi \); here, we directly considered the conditions necessary to establish the approximation results of the next sections. For more details, see, e.g., [5].

We now recall the following well-known regularity theorem for the SK operators.

Theorem 2

(Theorem 4.2 of [23]) Let \(\chi \) be a fixed n-kernel. Then, for any \(f \in L^p(\mathbb {R})\), \(1 \le p \le +\infty \), it turns out that \(K^\chi _wf \in W^{n,p}(\mathbb {R})\), \(w>0\), and the weak derivatives of \(K^\chi _w f\) can be expressed as follows

$$\begin{aligned} (K^\chi _w f)^{(i)}(x)\ :=\ w^i\sum _{k \in \mathbb {Z}}\left[ w \int _{k/w}^{(k+1)/w}f(u)\, du \right] \, \chi ^{(i)}(wx-k), \quad x \in \mathbb {R}. \end{aligned}$$
(2.3)

Remark 4

Note that, if in Theorem 2 the kernel \(\chi \in C^n(\mathbb {R})\), \(n \ge 1\), then also \(K^\chi _w f \in C^n(\mathbb {R})\) and, obviously, the derivatives of the SK operators given in (2.3) must be intended in the usual sense (see Proposition 4 of [13]) and Remark 8 of [14]).

Finally, we recall the following lemma that will be useful in the rest of the paper.

Lemma 1

(Lemma 5.3 of [23]) Let \(\chi \) be a n-kernel. Then:

$$\begin{aligned} m_\nu (\chi ^{(i)},x)\, =\ \sum _{k\in \mathbb {Z}}\left( k-x\right) ^{\nu } \chi ^{\left( i\right) }\left( x-k\right) ={\left\{ \begin{array}{ll} i!, &{} \nu =i\\ 0, &{} \nu \ne i, \end{array}\right. } \end{aligned}$$
(2.4)

where \(i=1,...,n\), and \(\nu =0, ..., i\).

Note that, Lemma 1 represents a generalization of Lemma 7 of [1] in case of non-smooth kernels.

3 Convergence in Sobolev spaces

Now, we are able to prove the following theorem.

Theorem 3

Let \(\chi \) be a fixed \(n-\)kernel. For every \(f\in W^{n,p}\left( \mathbb {R}\right) \), \(1 \le p \le +\infty \), we have

$$\begin{aligned} \left\| K_{w}^{\chi }f - f\right\| _{n,p}\rightarrow 0 \end{aligned}$$

as \(w\rightarrow +\infty .\)

Proof

We first observe that, by Theorem 2, we have \(K^\chi _w f \in W^{n,p}(\mathbb {R})\), \(w>0\). Since it is well known that, for any \(f\in L^{p}\left( \mathbb {R}\right) \) and any \(n-\)kernel \(\chi \), \(n\ge 1\), it turns out that \(\left\| \left( K_{w}^{\chi }f\right) -f\right\| _{p}\rightarrow 0\) as \(w\rightarrow +\infty \) (see Corollary 5.2 of [5]), then, in order to get the thesis, it is enough to show that \(\left\| \left( K_{w}^{\chi }f\right) ^{\left( n\right) }-f^{\left( n\right) }\right\| _{p}\rightarrow 0\) as \(w\rightarrow +\infty \). Recalling that, for \(f\in W^{n,p}\left( \mathbb {R}\right) \), the following formula holds:

$$\begin{aligned} f(u)=\sum _{j=0}^{n-1}\frac{(u-x)^{j}f^{(j)}(x)}{j!}+\int _{x}^{u}\frac{f^{\left( n\right) }\left( t\right) }{\left( n-1\right) !}\left( u-t\right) ^{n-1}dt \end{aligned}$$
(3.1)

(see [24], p. 37), using Theorem 2, (2.4) of Lemma 1, the expression (3.1), and the Binomial Newton formula, we can get what follows

$$\begin{aligned}{} & {} \left( K_{w}^{\chi }f\right) ^{\left( n\right) }=w^{n}\sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) }\left( wx-k\right) w\int _{k/w}^{(k+1)/w}f\left( u\right) du\\{} & {} \quad =w^{n+1}\sum _{k\in \mathbb {Z}}\chi ^{(n)}(wx-k)\sum _{j=0}^{n-1}\frac{f^{(j)}(x)}{j!} \sum _{\ell =0}^{j}\left( {\begin{array}{c}j\\ \ell \end{array}}\right) \!\left( \frac{k}{w}-x\right) ^{j-\ell }\!\!\!\int _{k/w}^{(k+1)/w}\!\!\left( u-\frac{k}{w}\right) ^{\ell }\!\!\!du \\{} & {} \qquad +\, w^{n}\sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) }\left( wx-k\right) w\int _{k/w}^{(k+1)/w} \int _{x}^{u}\frac{f^{\left( n\right) }\left( t\right) }{\left( n-1\right) !}\left( u-t\right) ^{n-1}dtdu \\{} & {} \quad =\ f(x)w^nm_0(\chi ^{(n)},wx) + \sum _{j=1}^{n-1}{f^{(j)}(x) \over j! w^{j-n}} \left[ \sum _{\ell =0}^j \left( {\begin{array}{c}j\\ \ell \end{array}}\right) { m_{j-\ell }(\chi ^{(n)},wx) \over \ell +1} \right] \\{} & {} \qquad +\, w^{n}\sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) } \left( wx-k\right) w\int _{k/w}^{(k+1)/w}\int _{x}^{u} \frac{f^{\left( n\right) }\left( t\right) }{\left( n-1\right) !}\left( u-t\right) ^{n-1}dtdu \end{aligned}$$
$$\begin{aligned} =w^{n}\sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) }\left( wx-k\right) w\int _{k/w}^{(k+1)/w} \int _{x}^{u}\frac{f^{\left( n\right) }\left( t\right) }{\left( n-1\right) !}\left( u-t\right) ^{n-1}dtdu. \end{aligned}$$

Furthermore, again by (2.4), we also have that

$$\begin{aligned} f^{\left( n\right) }\left( x\right) =w^{n}\sum _{k\in \mathbb {Z}} \chi ^{\left( n\right) }\left( wx-k\right) w\int _{k/w}^{(k+1)/w} \int _{x}^{u}\frac{f^{\left( n\right) }\left( x\right) }{\left( n-1\right) !}\left( u-t\right) ^{n-1}dtdu, \end{aligned}$$

where the latter equality is true since

$$\begin{aligned}{} & {} \frac{w^{n}}{n!}\sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) } \left( wx-k\right) w\int _{k/w}^{(k+1)/w}\left( u-x\right) ^{n}du \\{} & {} \quad =\sum _{m=0}^{n+1}\genfrac(){0.0pt}0{n+1}{m}\sum _{k\in \mathbb {Z}} \frac{\chi ^{\left( n\right) }\left( wx-k\right) \left( k-wx\right) ^{m}}{(n+1)!}-\sum _{k\in \mathbb {Z}}\frac{\chi ^{\left( n\right) }\left( wx-k\right) \left( k-wx\right) ^{n+1}}{(n+1)!} \\{} & {} \quad = {1 \over (n+1)!} \left( {\begin{array}{c}n+1\\ n\end{array}}\right) \sum _{k\in \mathbb {Z}} \chi ^{\left( n\right) }\left( wx-k\right) \left( k-wx\right) ^{n}\ =\ 1. \end{aligned}$$

So, we finally have:

$$\begin{aligned} \left\| \left( K_{w}^{\chi }f\right) ^{\left( n\right) } -f^{\left( n\right) }\right\| _{p}=\left( \int _{\mathbb {R}}\left| w^{n} \sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) }\left( wx-k\right) w\right. \right. \end{aligned}$$
$$\begin{aligned} \left. \left. \cdot \int _{k/w}^{(k+1)/w}\int _{x}^{u}\frac{f^{\left( n\right) } \left( t\right) -f^{\left( n\right) }\left( x\right) }{\left( n-1\right) !} \left( u-t\right) ^{n-1}dtdu\right| ^{p}dx\right) ^{1/p}. \end{aligned}$$
(3.2)

Now, since by \(\chi 1)\) the kernel \(\chi \) is a function with compact support, with \(\text {supp}\, \chi \subset \left[ -T,T\right] \), \(T>0\), the series can be considered only for the \(k\in \mathbb {Z}\) such that \(\left| wx-k\right| \le T.\) Hence we can also note that

$$\begin{aligned}{} & {} w^{n+1}\int _{k/w}^{(k+1)/w}\left| \int _{x}^{u}\left| f^{\left( n\right) } \left( t\right) -f^{\left( n\right) }\left( x\right) \right| \left| u-t\right| ^{n-1}dt\right| du \\{} & {} \quad \le w^{n+1}\int _{k/w}^{(k+1)/w}\left| u-x\right| ^{n-1}\left| \int _{x}^{u} \left| f^{\left( n\right) }\left( t\right) -f^{\left( n\right) }\left( x\right) \right| dt\right| du \\{} & {} \quad =w^{n+1}\int _{k/w}^{(k+1)/w}\left| u-x\right| ^{n-1}\left| \int _{0}^{u-x} \left| f^{\left( n\right) }\left( z+x\right) -f^{\left( n\right) }\left( x\right) \right| dz\right| du \\{} & {} \quad \le w^{n+1}\int _{k/w}^{(k+1)/w}\left| u-x\right| ^{n-1}\int _{\left| z\right| \le \frac{1}{w}+\left| \frac{k}{w}-x\right| }\left| f^{\left( n\right) }\left( z+x\right) -f^{\left( n\right) }\left( x\right) \right| dz\, du \\{} & {} \quad \le w^{n+1}\int _{k/w}^{(k+1)/w}\left| u-x\right| ^{n-1}\int _{\left| z\right| \le \frac{T+1}{w}}\left| f^{\left( n\right) }\left( z+x\right) -f^{\left( n\right) }\left( x\right) \right| dz\, du. \end{aligned}$$

Then, we can write

$$\begin{aligned}{} & {} \frac{w^{n+1}}{\left( n-1\right) !}\left| \sum _{k\in \mathbb {Z}}\chi ^{\left( n\right) } \left( wx-k\right) w\int _{k/w}^{(k+1)/w}\int _{x}^{u}f^{\left( n\right) } \left( t\right) -f^{\left( n\right) }\left( x\right) \left( u-t\right) ^{n-1}dtdu\right| \\{} & {} \quad \le \frac{w^{n+1}}{\left( n-1\right) !}\int _{\left| z\right| \le \frac{T+1}{w}} \left| f^{\left( n\right) }\left( z+x\right) -f^{\left( n\right) }\left( x\right) \right| dz \\{} & {} \qquad \cdot \sum _{\left| wx-k\right| \le T}\left| \chi ^{\left( n\right) } \left( wx-k\right) \right| \int _{k/w}^{(k+1)/w}\left| u-x\right| ^{n-1}du \\{} & {} \quad \le \frac{2^{n-2}}{\left( n-1\right) !}\left[ \frac{M_{0} \left( \chi ^{\left( n\right) }\right) }{n}+M_{n-1}\left( \chi ^{\left( n\right) }\right) \right] w\int _{\left| z\right| \le \frac{T+1}{w}}\left| f^{\left( n\right) } \left( z+x\right) -f^{\left( n\right) }\left( x\right) \right| dz. \end{aligned}$$

By the generalized Minkowsky inequality (Theorem 1), we get

$$\begin{aligned} \left\| \left( K_{w}^{\chi }f\right) ^{\left( n\right) }-f^{\left( n\right) } \right\| _{p}&\le \frac{2^{n-2}}{\left( n-1\right) !}\left[ \frac{M_{0} \left( \chi ^{\left( n\right) }\right) }{n}+M_{n-1}\left( \chi ^{\left( n\right) }\right) \right] \\&\cdot w\int _{\left| z\right| \le \frac{T+1}{w}}\left\| f^{\left( n\right) } \left( \cdot +z\right) -f^{\left( n\right) }\left( \cdot \right) \right\| _{p}dz\\&\le \frac{2^{n-1}}{\left( n-1\right) !}\left[ \frac{M_{0} \left( \chi ^{\left( n\right) }\right) }{n}+M_{n-1}\left( \chi ^{\left( n\right) }\right) \right] \\&\cdot \left( T+1\right) \sup _{\left| z\right| \le \frac{T+1}{w}} \left\| f^{\left( n\right) }\left( \cdot +z\right) -f^{\left( n\right) }\left( \cdot \right) \right\| _{p}. \end{aligned}$$

Since \(f^{\left( n\right) }\in L^{p}\left( \mathbb {R}\right) \) we have that for every \(\varepsilon >0\) there exists a \(\gamma >0\) such that for every \(\left| z\right| <\gamma \) we obtain \(\left\| f^{\left( n\right) }\left( \cdot +z\right) -f^{\left( n\right) }\left( \cdot \right) \right\| _{p}<\varepsilon \). Hence, for a sufficiently large \(w>0\), we get \(\left\| \left( K_{w}^{\chi }f\right) ^{\left( n\right) }-f^{\left( n\right) }\right\| _{p}<\varepsilon \). This completes the proof.

Remark 5

From formula (3.2) we can easily deduce that it is possible to bound the \(L^{p}\) norm of the n-th weak derivative of the operators \(\left( K_{w}^{\chi }f\right) ^{\left( n\right) }\) in terms of the \(L^{p}\) norm of the n-th weak derivative of the function itself. Let us consider, just for simplicity, the case \(n=1\). With the same hypotheses of the previous theorem, we easily get

$$\begin{aligned} \left\| \left( K_{w}^{\chi }f\right) ^{\prime }\right\| _{p}&=\left( \int _{\mathbb {R}}\left| w\sum _{k\in \mathbb {Z}}\chi ^{\prime } \left( wx-k\right) w\int _{k/w}^{(k+1)/w}\int _{x}^{u}f^{\prime } \left( t\right) dtdu\right| ^{p}dx\right) ^{1/p}\\&\le 2\, M_{0}\left( \chi ^{\prime }\right) \left( T+1\right) \left\| f^{\prime }\right\| _{p}. \end{aligned}$$

Now, denoting by \(AC(\mathbb {R})\), the usual space of the absolutely continuous functions of \(\mathbb {R}\), endowed with the usual semi-norm of the (Jordan) variation, i.e.,

$$\begin{aligned} V_\mathbb {R}[f]\ :=\ \Vert f'\Vert _1, \quad f \in AC(\mathbb {R}), \end{aligned}$$

it is quite natural to consider the problem of the convergence in variation, i.e., with respect to the semi-norm \(V_\mathbb {R}[\cdot ]\), of the SK operators.

Exploiting the well-know relation between \(W^{1,1}(\mathbb {R})\) and \(AC(\mathbb {R})\) (that is, the two spaces are equivalent), Theorem 2, and repeating the proof of Theorem 3 for the case \(n=p=1\) we immediately get the following.

Theorem 4

Let \(\chi \) be a n-kernel, \(n \ge 1\). For every \(f \in AC(\mathbb {R})\), it turns out that:

$$\begin{aligned} \lim _{w \rightarrow +\infty } V_\mathbb {R}[K^\chi _w f - f]\ =\ 0. \end{aligned}$$

Proof

Identifying \(AC(\mathbb {R})\) with \(W^{1,1}(\mathbb {R})\), and using Theorem 2 we have that \(K^\chi _w f \in AC(\mathbb {R})\). Furthermore, retracing the proof of Theorem 3 we can immediately see that:

$$\begin{aligned} V_\mathbb {R}[K^\chi _w f - f] = \Vert (K^\chi _w f)'-f' \Vert _1 \rightarrow 0, \quad \text{ as } \quad w\rightarrow +\infty . \end{aligned}$$

Remark 6

The problem of the convergence in variation for the SK operators has been firstly faced in [4], where two possible approaches have been followed.

  1. 1.

    In Theorem 2.3 of [4] has been proved the convergence in variation for the SK operators in case of band-limited kernels \(\chi \) and for functions belonging to the Bernstein class \(B^1_{\pi w}(\mathbb {R})\), \(w>0\).

  2. 2.

    In Theorem 2.1 of [4] a characterization of the space \(AC(\mathbb {R})\) has been established in terms of the convergence in variation of the SK operators based on the so-called averaged kernels (not necessarily band-limited), i.e., kernels of the following special form:

    $$\begin{aligned} \bar{\chi }_m(x) := {1 \over m}\int _{-m/2}^{m/2} \chi (u+x) du, \quad x \in \mathbb {R}, \quad m \in \mathbb {N}^+. \end{aligned}$$
    (3.3)

The result provided by Theorem 4 is not included in neither of the previous approaches. In fact, since the n-kernels have compact support, they are not band-limited; moreover, the n-kernel are not necessarily of the averaged kind recalled in (3.3).

4 Fractional Sobolev spaces

In this section, we introduce the fractional Sobolev space that we are going to use. In particular, we are interested to the spaces defined as the intersection of the fractional Sobolev spaces endowed with the Gagliardo semi-norm and the fractional Sobolev spaces equipped with (a recent definition) weak fractional derivatives.

Note that our choice is not particularly unusual; indeed, these two spaces have a lot of connections, for example, as we will explain better later, the space \(C_{0}^{\infty }\left( \mathbb {R}\right) \) is dense in both of them, with the respective norms. Furthermore, as we have already said in the introduction, it has been conjectured that actually these two spaces coincide.

Definition 1

Let \(s\in \left( 0,1\right) \), \(p\in \left[ 1,+\infty \right) \). We define the Gagliardo fractional Sobolev space

$$\begin{aligned} \widehat{W}^{s,p}\left( \mathbb {R}\right) :=\left\{ u\in L^{p}\left( \mathbb {R}\right) :\frac{\left| u\left( x\right) -u\left( y\right) \right| }{\left| x-y\right| ^{\frac{1}{p}+s}}\in L^{p}\left( \mathbb {R}\times \mathbb {R}\right) \right\} \end{aligned}$$

i.e., an intermediary Banach space between \(L^{p}\left( \mathbb {R}\right) \) and \(W^{1,p}\left( \mathbb {R}\right) \), endowed with the natural norm

$$\begin{aligned} \left\| u\right\| _{\widehat{W}^{s,p}\left( \mathbb {R}\right) }:=\left( \int _{\mathbb {R}}\left| u\left( x\right) \right| ^{p}dx+\int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| u\left( x\right) -u\left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy\right) ^{1/p}. \end{aligned}$$

We will indicate the Gagliardo semi-norm \(\int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| u\left( x\right) -u\left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy\) as \(\left[ u\right] _{\widehat{W}^{s,p}\left( \mathbb {R}\right) }^{p}\), (see, e.g., [27], Chapter 3).

Now we present some well-known results about \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \) that will be useful for our aims.

Theorem 5

Let \(p\in \left[ 1,+\infty \right) \) and \(0<s\le s^{\prime }<1.\) Let \(\Omega \) be an open set in \(\mathbb {R}\) and \(f:\,\Omega \rightarrow \mathbb {R}\) be a mesaurable function. Then

$$\begin{aligned} \left\| f\right\| _{\widehat{W}^{s,p}\left( \Omega \right) }\le C\left\| f\right\| _{\widehat{W}^{s^{\prime },p}\left( \Omega \right) } \end{aligned}$$

for some suitable \(C\ge 1.\)

For a proof, see [25], Proposition 2.1.

Theorem 6

Let \(p\in \left[ 1,+\infty \right) \) and \(0<s<1\), with \(sp<1.\) Then, the space \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \) is continuously embedded in \(L^{q}\left( \mathbb {R}\right) \) for any \(q\in \left[ p,p^{\star }\right] \), where \(p^{\star }=\frac{p}{1-sp}\) is the so-called fractional critical exponent. If \(sp=1\), then \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \) is continuously embedded in \(L^{q}\left( \mathbb {R}\right) \) for any \(q\in \left[ p,+\infty \right) \).

For a reference, see [25], Theorem 6.5, Theorem 6.7, Theorem 6.9, Theorem 6.10 or [3], Theorem 7.34, which provide a more general version of the previous result.

Theorem 7

(see [27], equation (3.2.2)) For any \(0<s<1\), the space \(C_{0}^{\infty }\left( \mathbb {R}\right) \) of smooth functions with compact support is dense in \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \).

As mentioned above, there is not a unique definition for the fractional Sobolev spaces. Here, we will show another possible choice which is useful for our aims, but first we have to recall some well-known facts about fractional calculus.

Definition 2

(Left and right Riemann-Liouville fractional operators) Let \(0<\sigma <1\) and \(f:\,\left[ a^{*},b^{*}\right] \rightarrow \mathbb {R}\). The \(\sigma \) order left Riemann-Liouville fractional integral of f is defined by

$$\begin{aligned} _{a^{*}}I_{x}^{\sigma }f(x):=\frac{1}{\Gamma \left( \sigma \right) }\int _{a^{*}}^{x} \frac{f\left( y\right) }{\left( x-y\right) ^{1-\sigma }}dy,\,\forall x\in \left[ a^{*},b^{*}\right] \end{aligned}$$

and the \(\sigma \) order right Riemann-Liouville fractional integral of f is defined by

$$\begin{aligned} _{x}I_{b^{*}}^{\sigma }f(x):=\frac{1}{\Gamma \left( \sigma \right) }\int _{x}^{b^{*}} \frac{f\left( y\right) }{\left( y-x\right) ^{1-\sigma }}dy,\,\forall x\in \left[ a^{*},b^{*}\right] \end{aligned}$$

where \(a^{*}=a\in \mathbb {R}\) or \(a^{*}=-\infty ,\) \(b^{*}=b\in \mathbb {R}\) or \(b=+\infty \), where the indeterminate forms at \(x=a^*\) and \(x=b^*\) must be interpreted as limits (here and in all the definitions below). We will use the notation \(^{-}I^{\alpha }f=_{a^{*}}I_{x}^{\alpha }f\) and \(^{+}I^{\alpha }f={}_{x}I_{b^{*}}^{\alpha }f\), i.e., to indicate both the case with finite and infinite domain (for a reference, see [42], Definition 2.1 and Section 5.1).

Definition 3

(Left and right Riemann-Liouville fractional derivatives) Let \(n-1<\alpha <n\), with \(n \in \mathbb {N}^+\), and \(f:\,\left[ a^{*},b^{*}\right] \rightarrow \mathbb {R}\). The \(\alpha \) order left Riemann-Liouville fractional derivative of f is defined by

$$\begin{aligned} _{a^{*}}D_{x}^{\alpha }f(x):=\frac{1}{\Gamma \left( n-\alpha \right) }\frac{d^{n}}{dx^{n}}\int _{a^{*}}^{x}\frac{f\left( y\right) }{\left( x-y\right) ^{1+\alpha -n}} dy=\frac{d^{n}}{dx^{n}}\,_{a^{*}}I_{x}^{n-\alpha }f(x), \end{aligned}$$

for all \(x\in \left[ a^{*},b^{*}\right] \) and the \(\alpha \) order right Riemann-Liouville fractional derivative of f is defined by

$$\begin{aligned} _{x}D_{b^{*}}^{\alpha }f(x):=\frac{1}{\Gamma \left( n-\alpha \right) } \frac{d^{n}}{dx^{n}}\int _{x}^{b^{*}}\frac{f\left( y\right) }{\left( y-x\right) ^{1+\alpha -n}} dy=\frac{d^{n}}{dx^{n}}{}_{x}I_{b^{*}}^{n-\alpha }f(x), \end{aligned}$$

for all \(x\in \left[ a^{*},b^{*}\right] \). We will use the notation \(^{-}D^{\alpha }f=_{a^{*}}D_{x}^{\alpha }f\) and \(^{+}D^{\alpha }f={}_{x}D_{b^{*}}^{\alpha }f\), where \(a^{*}=a\in \mathbb {R}\) or \(a^{*}=-\infty ,\) \(b^{*}=b\in \mathbb {R}\) or \(b=+\infty \) (for a reference, see [42], Definition 2.2 and Section 5.1).

Remark 7

In this paper, we will use the symbols \(^{\pm }D^{\alpha }\) and \(^{\pm }I^{\alpha }\) for both the infinite and finite cases, but the possible ambiguity will be resolved by the context; essentially, if we work with \(^{\pm }D^{\alpha }\) and \(^{\pm }I^{\alpha }\) under the improper integral \(\int _{\mathbb {R}}\), then we are working with the infinite case; if we are under a proper integral, we are working with the finite case.

Remark 8

Note that, any function \(\varphi \) belonging to \(C^\infty _0\) (with both bounded or unbounded domain) admits finite left and right Riemann-Liouville fractional derivative of any order.

We now present a simple result that will be useful later.

Proposition 1

Let \(1<p\le +\infty \), \(s\in (0,1)\) and \(\left[ a,b\right] \subset \mathbb {R}\). If \(f\in \widehat{W}^{s,p}\left( \mathbb {R}\right) \), we have

$$\begin{aligned} \lim _{x\rightarrow a^{+}}\int _{a}^{x}\frac{f\left( y\right) }{\left( x-y\right) ^{s}} dy=0,\, \lim _{x\rightarrow b^{-}}\int _{x}^{b}\frac{f\left( y\right) }{\left( y-x\right) ^{s}}dy\rightarrow 0. \end{aligned}$$

Proof

Assume that \(sp<1\). Then, since \(f\in \widehat{W}^{s,p}\left( \mathbb {R}\right) \), we know, by Theorem 6, that \(f\in L^{q}\left( \mathbb {R}\right) \) for every \(q\in \left[ p,p^{\star }\right] \), where \(p^{\star }=\frac{p}{1-sp}\). Now, if we take \(q=p^{\star }\), we note that \(\frac{1}{q}=\frac{1}{p}-s<1-s\), and so, if we set \(\frac{1}{q^{\prime }}:=1-\frac{1}{q}>s\), which means that \(q^{\prime }s<1\). So, by \(H\ddot{o}lder\) inequality,

$$\begin{aligned} \left| \int _{a}^{x}\frac{f\left( y\right) }{\left( x-y\right) ^{s}}dy\right| \le \left\| f\right\| _{q}\left( \int _{a}^{x} \frac{1}{\left( x-y\right) ^{sq^{\prime }}}dy\right) ^{1/q'}=\left\| f \right\| _{q}\frac{\left( x-a\right) ^{1/q^{\prime }-s}}{\left( 1-sq^{\prime } \right) ^{1/q^{\prime }}}\rightarrow 0\nonumber \\ \end{aligned}$$
(4.1)

as \(x\rightarrow a^{+}.\) Now assume \(sp=1\). Then, \(f\in L^{q}\left( \mathbb {R}\right) ,\,\forall q\ge p.\) So, we take q sufficiently large, such that \(\frac{1}{q}<1-s\) and so we can use again (4.1). Finally, if \(sp>1\) then there exists a \(s^{\prime }<s\) such that \(s^{\prime }p=1\). Now, since we know, by Theorem 5, that if \(f\in \widehat{W}^{s,p}\left( \mathbb {R}\right) \) then it belongs also to \(\widehat{W}^{s^{\prime },p}\left( \mathbb {R}\right) \) then we can conclude that \(f\in L^{q}\left( \mathbb {R}\right) ,\,\forall q\ge p\) and so the thesis follows using again (4.1) and arguing as in the previous case. The other case can be treated in a similar way.

Theorem 8

(See [42], Lemma 2.2) Let \(\left[ a,b\right] \subset \mathbb {R}\) and \(0<\alpha <1\). Assume that \(f\in AC\left( \left[ a,b\right] \right) \). Then, \(_{a}D_{x}^{\alpha }f\) and \(_{x}D_{b}^{\alpha }f\) exist almost everywhere, \(_{a}D_{x}^{\alpha }f,\,_{x}D_{b}^{\alpha }f\in L^{r} \left( a,b\right) ,\,1\le r<\frac{1}{\alpha }\) and

$$\begin{aligned} _{a}D_{x}^{\alpha }f\left( x\right) =\frac{f\left( a\right) }{\Gamma \left( 1-\alpha \right) \left( x-a\right) ^{\alpha }}+{}_{a}I_{x}^{1-\alpha }f^{\prime }\left( x\right) , \\ _{x}D_{b}^{\alpha }f\left( x\right) =\frac{f\left( b\right) }{\Gamma \left( 1-\alpha \right) \left( b-x\right) ^{\alpha }}+{}_{x}I_{b}^{1-\alpha }f^{\prime }\left( x\right) . \end{aligned}$$

It is quite easy to observe that the previous identities hold also in the infinite case, assuming that f is a “good function”. For example, if \(f\in AC\left( \mathbb {R}\right) \), it is straighfoward to obtain

$$\begin{aligned} _{-\infty }D_{x}^{\alpha }f\left( x\right) =_{-\infty }I_{x}^{1-\alpha }f^{\prime }\left( x\right) , \end{aligned}$$
(4.2)
$$\begin{aligned} _{x}D_{+\infty }^{\alpha }f\left( x\right) ={}_{x}I_{+\infty }^{1-\alpha }f^{\prime }\left( x\right) . \end{aligned}$$
(4.3)

Theorem 9

(Fractional integration by parts, see [42], equation (2.20)) Let \(\left[ a,b\right] \subset \mathbb {R}\) and \(0<s<1\). If \(\varphi \in L^{p}\left( a,b\right) ,\,\psi \in L^{q}\left( a,b\right) ,\) with \(p,q\ge 1,\,\frac{1}{p}+\frac{1}{q}\le 1+s\) and \(p\ne 1,\,q\ne 1\) if \(\frac{1}{p}+\frac{1}{q}=1+s\), then

$$\begin{aligned} \int _{a}^{b}\varphi \left( x\right) {}_{a}I_{x}^{s}\psi \left( x\right) dx= \int _{a}^{b}{}_{x}I_{b}^{s}\varphi \left( x\right) \psi \left( x\right) dx. \end{aligned}$$

Definition 4

(Weak fractional derivatives, see [31], Definition 3.1.) For \(\alpha >0\), let \(\left\lfloor \alpha \right\rfloor \) be the integer part of \(\alpha \). Let \(\Omega \) be a finite interval or the whole real line \(\mathbb {R}\). For \(u\in L^{1}\left( \Omega \right) \) a function \(v\in L_{loc}^{1}\left( \Omega \right) \) is called the left weak fractional derivative of u if

$$\begin{aligned} \int _{\Omega }v\left( x\right) \varphi \left( x\right) dx=\left( -1\right) ^{\left\lfloor \alpha \right\rfloor }\int _{\Omega }u\left( x\right) {}^{+}D^{\alpha }\widetilde{\varphi }\left( x\right) dx,\,\forall \varphi \in C_{0}^{\infty }\left( \Omega \right) \end{aligned}$$

and we write \(^{-}\mathcal {D}^{\alpha }u:=v\). A function \(w\in L_{loc}^{1}\left( \Omega \right) \) is called the right weak fractional derivative of u if

$$\begin{aligned} \int _{\Omega }w\left( x\right) \varphi \left( x\right) dx=\left( -1\right) ^{\left\lfloor \alpha \right\rfloor }\int _{\Omega }u\left( x\right) {}^{-}D^{\alpha }\widetilde{\varphi }\left( x\right) dx,\,\forall \varphi \in C_{0}^{\infty }\left( \Omega \right) \end{aligned}$$

and we write \(^{+}\mathcal {D}^{\alpha }u:=w\). The function \(\widetilde{\varphi }\) is such that \(\widetilde{\varphi }=\varphi \) if \(\Omega \ne \mathbb {R}\) and it is the zero extension of \(\varphi \) if \(\Omega =\mathbb {R}\).

Remark 9

It is known that the weak fractional derivative exists also for function such that the Riemann-Liouville fractional derivative does not. However, if f is Riemann-Liouville differentiable of order s and \(^{\pm }D^{s}f\in L_{loc}^{1}\left( \mathbb {R}\right) \), then \(^{\pm }\mathcal {D}^{s}f={}^{\pm }D^{s}f\) almost everywhere (see [31], Proposition 3.3). Actually, it is simple to deduce from the proof of this claim that it is enough to have f Riemann-Liouville differentiable of order s almost everywhere.

The weak fractional derivatives can be defined also for functions belonging to \(L^{p}\left( \Omega \right) \). Indeed, we have the following theorem.

Theorem 10

Let \(u\in L^{p}\left( \mathbb {R}\right) \) for \(1\le p<+\infty \) and \(\alpha >0\). Then \(v:=^{\pm }\mathcal {D}^{\alpha }u\in L_{loc}^{q}\left( \mathbb {R}\right) \) for \(1\le q<+\infty \) if and only if there exists \(\left( u_{j}\right) _{j=1}^{+\infty }\in C_{0}^{\infty }\left( \mathbb {R}\right) \) such that \(u_{j}\longrightarrow u\) in \(L^{p}\left( \mathbb {R}\right) \) and \(^{\pm }\mathcal {D}^{\alpha }u_{j}\longrightarrow v\) in \(L_{loc}^{q}\left( \mathbb {R}\right) \).

For a proof of Theorem 10, see [31], Corollary 3.10. For our aims, it is important to recall that the weak fractional derivative operator is linear, that is, for every weakly differentiable u, v of order \(\alpha >0\), we have

$$\begin{aligned} ^{\pm }\mathcal {D}^{\alpha }\left( \lambda u+\mu v\right) =\lambda {}^{\pm }\mathcal {D}^{\alpha }u+\mu {}^{\pm }\mathcal {D}^{\alpha }v,\,\lambda ,\,\mu \in \mathbb {R}. \end{aligned}$$

For a reference, see [31], Proposition 3.11.

Theorem 11

(Fundamental theorem of weak fractional derivatives in finite intervals case) Let \(\left( a,b\right) \subset \mathbb {R}\) be a finite interval and \(0<\alpha <1\). Suppose that \(f\in L^{p}\left( a,b\right) \) and \(^{\pm }\mathcal {D}^{\alpha }f\in L^{p}\left( a,b\right) \) for some \(1\le p<+\infty .\) Then there holds

$$\begin{aligned} f=^{\pm }I^{s}{}^{\pm }\mathcal {D}^{s}f+c_{\pm }^{1-s}\kappa _{\pm }^{s}, \quad a.e.\ in\ (a,b), \end{aligned}$$
(4.4)

where

$$\begin{aligned} c_{-}^{1-s}=\frac{_{a}I_{x}^{1-s}f\left( a\right) }{\Gamma \left( 1-s\right) },\, c_{+}^{1-s}=\frac{_{x}I_{b}^{1-s}f\left( b\right) }{\Gamma \left( 1-s\right) }, \\ \kappa _{-}^{s}=\kappa _{-}^{s}\left( x\right) =\left( x-a\right) ^{s-1},\,\kappa _{+}^{s}=\kappa _{+}^{s}\left( x\right) =\left( b-x\right) ^{s-1}. \end{aligned}$$

For a proof of Theorem 11, see [31], Theorem 3.16.

Definition 5

(See [32], Definition 3.1) (Left/right fractional Sobolev spaces) For \(0<s<1\) and \(1\le p\le \infty \) the left/right fractional Sobolev spaces \(^{\pm }W^{s,p}\left( \mathbb {R}\right) \) are defined by

$$\begin{aligned} ^{\pm }W^{s,p}\left( \mathbb {R}\right) :=\left\{ f\in L^{p}\left( \mathbb {R}\right) :\,{}^{\pm }\mathcal {D}^{s}f\in L^{p}\left( \mathbb {R}\right) \right\} \end{aligned}$$

which are endowed respectively with the norms

$$\begin{aligned} \left\| f\right\| _{^{\pm }W^{s,p}\left( \mathbb {R}\right) }:={\left\{ \begin{array}{ll} \left( \left\| f\right\| _{L^{p}\left( \mathbb {R}\right) }^{p}+\left\| ^{\pm }\mathcal {D}^{s}f\right\| _{L^{p}\left( \mathbb {R}\right) }^{p}\right) ^{1/p}, &{} 1\le p<\infty \\ \left\| f\right\| _{L^{\infty }\left( \mathbb {R}\right) }+\left\| ^{\pm }\mathcal {D}^{s}f\right\| _{L^{\infty }\left( \mathbb {R}\right) }, &{} p=\infty . \end{array}\right. } \end{aligned}$$

We recall that there exist, in the literature, similar spaces to the left/right fractional Sobolev spaces (see, e.g., [6, 38, 39]).

Definition 6

(Symmetric fractional Sobolev space, see [32], Definition 3.2) For \(s>0\) and \(1\le p\le \infty \) the symmetric fractional Sobolev space is defined by

$$\begin{aligned} \widetilde{W}^{s,p}\left( \mathbb {R}\right) :={}^{+}W^{s,p}\left( \mathbb {R}\right) \cap {}^{-}W^{s,p}\left( \mathbb {R}\right) , \end{aligned}$$

which is endowed with the norm

$$\begin{aligned} \left\| f\right\| _{\widetilde{W}^{s,p}\left( \mathbb {R}\right) }:={\left\{ \begin{array}{ll} \left( \left\| f\right\| _{^{+}W^{s,p}\left( \mathbb {R}\right) }^{p}+\left\| f\right\| _{^{-}W^{s,p}\left( \mathbb {R}\right) }^{p}\right) ^{1/p}, &{} 1\le p<\infty \\ \left\| f\right\| _{^{+}W^{s,\infty }\left( \mathbb {R}\right) }+\left\| f\right\| _{^{-}W^{s,\infty }\left( \mathbb {R}\right) }, &{} p=\infty . \end{array}\right. } \end{aligned}$$

Remark 10

In Lemma 4.33 of [32] it is proved that if \(f\in \widetilde{W}^{s,p}\left( \mathbb {R}\right) \) the constants \(c_{+}^{1-s},\,c_{-}^{1-s}\) defined in (4.4) are equal to 0, but in such lemma there is indicated only an explicit range for s (that is, \(0<s<1\)), nothing is specified about p. However, following the proof, it is possible to deduce that the correct range is \(p\in \left( 1,+\infty \right) \) and this fact has been confirmed to us by one of the authors (X. Feng, personal communication, 5th February 2023), so we have the same results of Proposition 1 also in the case of \(f\in \widetilde{W}^{s,p}\left( \mathbb {R}\right) \).

Now we present, as far as we know, a new family of fractional Sobolev spaces.

Definition 7

(Tight fractional Sobolev space) For \(s>0\) and \(1\le p\le \infty \) the tight fractional Sobolev space is defined by

$$\begin{aligned} {{\mathcal {W}}}^{s,p}\left( \mathbb {R}\right) :=\widehat{W}^{s,p}\left( \mathbb {R}\right) \cap \widetilde{W}^{s,p}\left( \mathbb {R}\right) \end{aligned}$$

which is endowed with the norm

$$\begin{aligned} \left\| f\right\| _{{{\mathcal {W}}}^{s,p}\left( \mathbb {R}\right) }:={\left\{ \begin{array}{ll} \left\| f\right\| _{\widehat{W}^{s,p}\left( \mathbb {R}\right) }+\left\| f\right\| _{\widetilde{W}^{s,p}\left( \mathbb {R}\right) }, &{} 1\le p<\infty \\ \left\| f\right\| _{\widehat{W}^{s,\infty }\left( \mathbb {R}\right) }+\left\| f\right\| _{\widetilde{W}^{s,\infty }\left( \mathbb {R}\right) }, &{} p=\infty . \end{array}\right. } \end{aligned}$$

As we said before, the set \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \cap \widetilde{W}^{s,p}\left( \mathbb {R}\right) \) is non-empty; actually, \(C_{0}^{\infty }\left( \mathbb {R}\right) \) is dense in both sets (see Theorem 7 for \(\widehat{W}^{s,p}\left( \mathbb {R}\right) \) and Theorem 4.5 of [32] for the space \(\widetilde{W}^{s,p}\left( \mathbb {R}\right) \)). Furthermore, we emphasize again that it is conjectured that \(\widehat{W}^{s,p}\left( \mathbb {R}\right) =\widetilde{W}^{s,p}\left( \mathbb {R}\right) \) for \(1\le p\le \infty \) and \(0<s<1\) (see Remark 4.57 of [32]).

Now we prove that \(C_{0}^{\infty }\left( \mathbb {R}\right) \) is dense also in \({\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \).

Lemma 2

Let \(\eta \in C_{0}^{\infty }\left( \mathbb {R}\right) \) be such that \(\eta \ge 0\) in \(\mathbb {R}\) with \(\text {supp}\, \eta \subset B_{1}\), where \(B_{1}\) is the ball with radius 1. Assume that \(\int _{B_{1}}\eta (x)dx=1\) and define \(\eta _{\frac{1}{j}}(x)=j\eta \left( xj\right) ,j\in \mathbb {N}^{+}.\) Then, for every \(f\in {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \), the sequence

$$\begin{aligned} \left\{ f_{j}\left( x\right) \right\} :=\left\{ \left( \eta _{\frac{1}{j}}*f\right) \left( x\right) \right\} \subset C_{0}^{\infty }\left( \mathbb {R}\right) ,\quad j\in \mathbb {N}^{+} \end{aligned}$$

converges to f in \({\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \) as \(j\rightarrow +\infty \), where \(*\) denotes the usual convolution product. Hence, \(C_{0}^{\infty }\left( \mathbb {R}\right) \) is dense in \({\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \).

Proof

The convergence in \(L^{p}(\mathbb {R})\) can be found in the classical text [9], Theorem IV.22, and the convergence with respect the Gagliardo semi-norm is a special case of [33], Lemma 11. It remains to observe that, since \(f\in \widetilde{W}^{s,p}\left( \mathbb {R}\right) \), then \(^{\pm }\mathcal {D}^{s}f\in L^{p}\left( \mathbb {R}\right) \), and using the fact that

$$\begin{aligned} ^{\pm }\mathcal {D}^{s}f_{j}=\eta _{\frac{1}{j}}*{}^{\pm }\mathcal {D}^{s}f \end{aligned}$$

a.e. in \(\mathbb {R}\) and for every \(j\in \mathbb {N}^{+}\)(see Lemma 3.8 of [31]), we have, from the previous part, that \(^{\pm }\mathcal {D}^{s}f_{j}\) converges to \(^{\pm }\mathcal {D}^{s}f\) in \(L^{p}\left( \mathbb {R}\right) \).\(\square \)

We want to underline that the space \(\widetilde{W}^{s,p}\left( \mathbb {R}\right) \) is not the first fractional Sobolev space defined in the literature using Riemann-Liouville operators. Indeed, the readers can also see, e. g., [6, 8, 12, 36, 37].

5 Convergence of the sampling Kantorovich operators in \({\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \)

We want to prove the convergence of the SK operators in \({\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \) with respect the norm \(\left\| \cdot \right\| _{{\mathcal {W}}^{s,p}\left( \mathbb {R}\right) }\) using a density argument. For simplicity, we will use the notation \(\left\| \cdot \right\| _{{\mathcal {W}}^{s,p}\left( \mathbb {R}\right) }=\left\| \cdot \right\| _{s,p}\), that we must not confuse with that one used in Section 2.

Throughout this section, in order to get the desired approximation results we need to assume the following additional regularity condition on the n-kernel \(\chi \) (\(n \ge 1\)), that is the following:

$$\begin{aligned} \chi 5)\,\,\chi \in C^{1}\left( \mathbb {R}\right) . \end{aligned}$$
(5.1)

We start with the following theorem.

Theorem 12

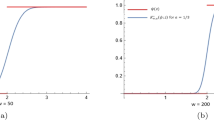
Let \(\chi \) be a given n-kernel (\(n \ge 1\)) satisfying (5.1), and let \(f\in {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \), with \(0<s<1\) and \(1<p<+\infty \). Then,

$$\begin{aligned} \left\| K_{w}^{\chi }f\right\| _{s,p}^{p}\le \widetilde{C}\left( s,p,\chi \right) \left( \left\| f\right\| _{p}+\left\| ^{-}\mathcal {D}^{s}f\right\| _{p}^{p}+\left\| ^{+}\mathcal {D}^{s}f\right\| _{p}^{p}\right) \end{aligned}$$

where \(\widetilde{C}\left( s,p,\chi \right) \) is an effectively calculable absolute constant depending only on \(\chi \), p and s. The above inequality implies that \(K^\chi _w: {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \rightarrow {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \), \(w>0\).

Proof

In order to get the theorem we need to estimate \(\Vert K^\chi _w f\Vert _p^p\), \([K^\chi _w f]^p_{\widehat{W}^{s,p}(\mathbb {R})}\), \(\Vert ^\pm \mathcal {D}^s K^\chi _w f\Vert _{p}^p\).

We know (see [5], Corollary 5.1) that

$$\begin{aligned} \left\| K_{w}^{\chi }f\right\| _{p}^{p}\le M_{0}\left( \chi \right) ^{p-1}\left\| \chi \right\| _{1}\left\| f\right\| _{p} \end{aligned}$$
(5.2)

\(f \in {\mathcal {W}}^{s,p}(\mathbb {R})\subset L^p(\mathbb {R})\). Now, we estimate

$$\begin{aligned}{}[K^\chi _w f]^p_{\widehat{W}^{s,p}(\mathbb {R})}\ =\ \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy. \end{aligned}$$

Since \(\chi \) satisfies \(\chi 1)\) we have that \(\text {supp}\, \chi \subset [-T,T]\), \(T>0\). Let us define

$$\begin{aligned} A_{x,w,T}:=\left\{ k\in \mathbb {Z}:-T+wx<k<T+wx\right\} . \end{aligned}$$

Hence, we have

$$\begin{aligned}{} & {} [K^\chi _w f]^p_{\widehat{W}^{s,p}(\mathbb {R})} = \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dx\, dy \\{} & {} \quad =\int _{\mathbb {R}}\int _{\mathbb {R}}\frac{1}{\left| x-y\right| ^{1+sp}}\left| \sum _{k\in A_{x,w,T}}w\int _{k/w}^{(k+1)/w}f(u)du\chi \left( wx-k\right) \right. \\{} & {} \qquad \left. -\sum _{k\in A_{y,w,T}}w\int _{k/w}^{(k+1)/w}f(u)du\chi \left( wy-k\right) \right| ^{p}dxdy \\{} & {} \quad =\int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \sum _{k\in A_{x,w,T}\cup A_{y,w,T}}w\int _{k/w}^{(k+1)/w}f(u)du\left[ \chi \left( wx-k\right) -\chi \left( wy-k\right) \right] \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \end{aligned}$$

where the last identity follows from the fact that if \(k \in A_{y,w,T}\) but \(k\notin A_{x,w,T}\), then

$$\begin{aligned} w\int _{k/w}^{(k+1)/w}f(u)du\chi \left( wx-k\right) =0 \end{aligned}$$

hence

$$\begin{aligned} w\int _{k/w}^{(k+1)/w}f(u)du[\chi \left( wx-k\right) -\chi (wy-k)]= - w\int _{k/w}^{(k+1)/w}f(u)du\chi \left( wy-k\right) \end{aligned}$$

and the same holds for the converse case. Let for simplicity

$$\begin{aligned} A:= A_{x,w,T}\cup A_{y,w,T}, \end{aligned}$$
(5.3)

and denote by |A| its cardinality. By Jensen inequality we get

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \\{} & {} \quad \le \int _{\mathbb {R}}\int _{\mathbb {R}}\left( \sum _{k\in A}\frac{\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| \left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| }{\left| x-y\right| ^{\frac{1}{p}+s}}\right) ^{p}dxdy \\{} & {} \quad =\int _{\mathbb {R}}\int _{\mathbb {R}}\left( \sum _{k\in A}\frac{\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| \left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| |A| }{\left| x-y\right| ^{\frac{1}{p}+s}|A| }\right) ^{p}dxdy \\{} & {} \quad \le \int _{\mathbb {R}}\int _{\mathbb {R}}\sum _{k\in A}\frac{\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| ^{p}\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}|A|^{p}}{\left| x-y\right| ^{1+sp}|A|}dxdy. \end{aligned}$$

Now note that

$$\begin{aligned} 1\ \le \ |A_{x,w,T}|\ \le \ |A|\ \le |A_{x,w,T}|\, +\, |A_{y,w,T}|\ \le \ 4T + 2, \end{aligned}$$
(5.4)

since the real intervals defining the sets \(A_{x,w,T}\) and \(A_{y,w,T}\) have both a lenght equal to 2T, hence both contain at most \(2T+1\) integer values. So we have

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \\{} & {} \quad \le (4T+2)^{p}\int _{\mathbb {R}}\int _{\mathbb {R}}\sum _{k\in A}\frac{\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| ^{p}\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \end{aligned}$$

Now we have to focus on \(w\int _{k/w}^{(k+1)/w}f(u)du\). Since \(f\in {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \subseteq ^{-}W^{s,p}\left( \mathbb {R}\right) \), we have that \(f,\,{}^{-}\mathcal {D}^{s}f\in L^{p}\left( \mathbb {R}\right) \), which implies that \(f,\,{}^{-}\mathcal {D}^{s}f\in L^{p}\left( \left[ \frac{k}{w},\frac{k+1}{w}\right] \right) \) for every \(w>0\) and \(k\in \mathbb {Z}\) (we remark that there is no particular reason to use \(^{-}W^{s,p}\left( \mathbb {R}\right) \); if one take \(^{+}W^{s,p}\left( \mathbb {R}\right) \) the proof is essentially the same). Then, by Theorem 11, we have that

$$\begin{aligned} f=^{-}I^{s}{}^{-}\mathcal {D}^{s}f+c_{-}^{1-s}\kappa _{-}^{s} \end{aligned}$$
(5.5)

almost everywhere in \(\left[ \frac{k}{w},\frac{k+1}{w}\right] \), where \(c_{-}^{1-s}=\frac{_{\frac{k}{w}}I_{x}^{1-s}f\left( \frac{k}{w}\right) }{\Gamma \left( 1-s\right) },\,\kappa _{-}^{s}=\kappa _{-}^{s}\left( u\right) =\left( u-\frac{k}{w}\right) ^{s-1}\) Now, since \(1<p<+ \infty \), by Proposition 1, we have that \(c_{-}^{1-s}=0\), so, using (5.5) we can write

$$\begin{aligned} w\int _{k/w}^{(k+1)/w}f(u)du=w\int _{k/w}^{(k+1)/w}\left( ^{-}I^{s}{}^{-}\mathcal {D}^{s}f\right) (u)du. \end{aligned}$$

Now, since \(^{-}\mathcal {D}^{s}f\) is, in particular, in \(L^{p}\left( \left[ \frac{k}{w},\frac{k+1}{w}\right] \right) \), we can apply the fractional integration by parts rule (Theorem 9); since \(_{x}I_{\frac{k+1}{w}}^{s}1=\frac{\left( \frac{k+1}{w}-x\right) ^{s}}{\Gamma \left( s+1\right) }\) (see [42], equation (2.44)), we obtain the following chain of identities

$$\begin{aligned} w\int _{k/w}^{(k+1)/w}f(u)du&=w\int _{k/w}^{(k+1)/w}\left( ^{-}I^{s}{}^{-}\mathcal {D}^{s}f\right) (u)du \nonumber \\&=\frac{w}{\Gamma \left( s+1\right) }\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du \end{aligned}$$
(5.6)

hence we have to bound the quantity

$$\begin{aligned}{} & {} \frac{(4T+2)^{p}}{\Gamma (s+1)^{p}}\int _{\mathbb {R}}\int _{\mathbb {R}}\sum _{k\in A}\left| w\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \quad \cdot \frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy. \end{aligned}$$

Now, extending the series to all \(k \in \mathbb {Z},\) from Fubini-Tonelli theorem and Jensen inequality we get

$$\begin{aligned}{} & {} \frac{(4T+2)^{p}}{\Gamma (s+1)^{p}}\int _{\mathbb {R}}\int _{\mathbb {R}}\sum _{k\in A}\left| w\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \\{} & {} \quad \le \frac{(4T+2)^{p}}{\Gamma (s+1)^{p}}\sum _{k\in \mathbb {Z}}\left| w\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dxdy \\{} & {} \quad \le \frac{(4T+2)^{p}\left[ \chi \right] _{\widehat{W}^{s,p}\left( \mathbb {R}\right) }^{p}w^{sp}}{\Gamma \left( s+1\right) ^{p}}\sum _{k\in \mathbb {Z}}\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{sp}\left| ^{-}\mathcal {D}^{s}f(u)\right| ^{p}du \end{aligned}$$
$$\begin{aligned} \le \frac{(4T+2)^{p}\left[ \chi \right] _{\widehat{W}^{s,p}\left( \mathbb {R}\right) }^{p}}{\Gamma \left( s+1\right) ^{p}}\left\| ^{-}\mathcal {D}^{s}f\right\| _{p}^{p}. \end{aligned}$$
(5.7)

Now we have to bound

$$\begin{aligned} \Vert ^\pm \mathcal {D}^s K^\chi _w f\Vert _p^p\ =\ \int _{\mathbb {R}}\left| ^{\pm }\mathcal {D}^{s}K_{w}^{\chi }f\right| ^{p}dx. \end{aligned}$$

Again, we focus only on the case \(^{-}\mathcal {D}^{s}\) since the other case is similar. Recalling that \(\chi \) satisfies (5.1), we have that \(K_{w}^{\chi }f\in C^{1}\left( \mathbb {R}\right) \) (see Remark 4), then its weak fractional derivatives match with its fractional derivatives. Moreover, we also know that Theorem 2 holds, hence \(K_{w}^{\chi }f\in L^{p}\left( \mathbb {R}\right) \). So, using the following equality coming by a special application of the usual integration by parts:

$$\begin{aligned} \int _{-\infty }^{x}\frac{\left( K_{w}^{\chi }f\right) ^{\prime }\left( y\right) }{\left( x-y\right) ^{s}}dy&= \left[ \frac{\left( K_{w}^{\chi }f\right) \left( y\right) -\left( K_{w}^{\chi }f\right) \left( x\right) }{\left( x-y\right) ^{s}}\right] _{-\infty }^x \nonumber \\ {}&- s \int _{-\infty }^{x}\frac{\left( K_{w}^{\chi }f\right) \left( y\right) -\left( K_{w}^{\chi }f\right) \left( x\right) }{\left( x-y\right) ^{s+1}}dy \nonumber \\&=\ - s \int _{-\infty }^{x}\frac{\left( K_{w}^{\chi }f\right) \left( y\right) -\left( K_{w}^{\chi }f\right) \left( x\right) }{\left( x-y\right) ^{s+1}}dy \end{aligned}$$
(5.8)

and by (4.2), we can get what follows:

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\left| ^{-}\mathcal {D}^{s}K_{w}^{\chi }f\right| ^{p}dx=\frac{1}{\Gamma \left( 1-s\right) ^{p}}\int _{\mathbb {R}}\left| \int _{-\infty }^{x}\frac{\left( K_{w}^{\chi }f\right) ^{\prime }\left( y\right) }{\left( x-y\right) ^{s}}dy\right| ^{p}dx \\{} & {} \quad =\frac{s^{p}}{\Gamma \left( 1-s\right) ^{p}}\int _{\mathbb {R}}\left| \int _{-\infty }^{x}\frac{\left( K_{w}^{\chi }f\right) \left( y\right) -\left( K_{w}^{\chi }f\right) \left( x\right) }{\left( x-y\right) ^{s+1}}dy\right| ^{p}dx \\{} & {} \quad \le \frac{2^{p-1}s^{p}}{\Gamma \left( 1-s\right) ^{p}}\left[ \int _{\mathbb {R}}\left( \int _{-\infty }^{x-1/w}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx\right. \\{} & {} \qquad \left. +\int _{\mathbb {R}}\left( \int _{x-1/w}^{x}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx\right] \\{} & {} \quad =:\frac{2^{p-1}s^{p}}{\Gamma \left( 1-s\right) ^{p}}\left[ I_{1}+I_{2}\right] . \end{aligned}$$

Let us consider \(I_{1}\). Noting that:

$$\begin{aligned} \int _{-\infty }^{x-1/w} \frac{1}{(x-y)^{s+1}}\, dy\ =\ {w^s \over s}, \end{aligned}$$

by Jensen inequality we obtain

$$\begin{aligned}{} & {} I_1 = \int _{\mathbb {R}}\left( \int _{-\infty }^{x-1/w}\frac{\left| \left( K_{w}^{\chi } f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx\ \\{} & {} \quad \le \ s^{1-p}\,w^{sp-s}\int _{\mathbb {R}}\int _{-\infty }^{x-1/w}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) \right| ^{p}}{\left( x-y\right) ^{s+1}}dydx \\{} & {} \quad \le s^{1-p}w^{sp-s}\int _{\mathbb {R}}\int _{-\infty }^{x-1/w}\left( \sum _{k\in A}\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| \right. \\{} & {} \qquad \cdot \left. \left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| \right) ^{p}\frac{1}{\left( x-y\right) ^{s+1}}dydx \end{aligned}$$

where A is the set defined in (5.3). So, using again the Jensen inequality (in the same way we used in order to find the inequality in (5.7)), we have to deal with

$$\begin{aligned}{} & {} s^{1-p}\,(4T+2)^{p}\,w^{sp-s}\int _{\mathbb {R}}\int _{-\infty }^{x-1/w} \sum _{k\in A}\left| w\int _{k/w}^{(k+1)/w}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p} \frac{1}{\left( x-y\right) ^{s+1}}dydx \\{} & {} \quad \le \ s^{1-p}\, (4T+2)^p\, w^{sp-s}\sum _{k\in \mathbb {Z}}\left| w \int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \int _{\mathbb {R}}\int _{-\infty }^{x-1/w}\frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| ^{p}}{\left( x-y\right) ^{s+1}}dydx \\{} & {} \quad \le s^{1-p}\, (4T+2)^p\, w^{sp-1}\sum _{k\in \mathbb {Z}}\left| w \int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \chi \left( v\right) -\chi \left( h\right) \right| ^{p}}{\left| v-h\right| ^{s+1}}dv\, dh. \end{aligned}$$

Now, since \(\chi \) is a smooth function with bounded support it is not difficult to see that

$$\begin{aligned} J:=\int _{\mathbb {R}}\int _{\mathbb {R}}\frac{\left| \chi \left( v\right) - \chi \left( h\right) \right| ^{p}}{\left| v-h\right| ^{s+1}}dvdh>0 \end{aligned}$$

is finite. In fact, we have

$$\begin{aligned} J&=\int _{|v|\le T}dv \left[ \int _{\mathbb {R}}{|\chi (v)-\chi (h)|^p \over |v-h|^{1+s}}\, dh \right] \ +\ \int _{|v|> T}dv \left[ \int _{\mathbb {R}}{|\chi (h)|^p \over |v-h|^{1+s}}\, dh \right] \ \\&=:\ J_1 + J_2, \end{aligned}$$

where

$$\begin{aligned} J_1&= \int _{|v|\le T}dv \left[ \int _{|h|\le T}{|\chi (v)-\chi (h) |^p \over |v-h|^{1+s}}\, dh\ +\ |\chi (v)|^p \int _{|h|> T}{1 \over |v-h|^{1+s}}\, dh \right] \ \\&=: J_{1,1} + J_{1,2}, \end{aligned}$$

and

$$\begin{aligned} J_{2}&= \int _{|v|> T}dv \left[ \int _{|h|\le T}{|\chi (h)|^p \over |v-h|^{1+s}}\, dh \right] \\&= \int _{|h|\le T}|\chi (h)|^p \left[ \int _{|v| > T}{1 \over |v-h|^{1+s}}\, dv \right] dh\\&= \frac{1}{s}\int _{|h|\le T}|\chi (h)|^p \left[ {1 \over (T-h)^s} + {1 \over {(T+h)^s}} \right] \, dh < +\infty , \end{aligned}$$

since \(s \in (0,1)\) and \(\chi \) is bounded. Similarly, we can see that \(J_{1,2}<+\infty \) since \(s+1>1\) and \(\chi \) is bounded, while

$$\begin{aligned} J_{1,1}\ =\ \int _{|v|\le T}dv \int _{|h|\le T}{|\chi (v)-\chi (h)| \over |v-h|}\, {|\chi (v)-\chi (h)|^{p-1} \over |v-h|^{s}}dh \\ \le \ \Vert \chi '\Vert _{\infty } (2\Vert \chi \Vert _{\infty })^{p-1}\int _{|v|\le T}dv\int _{|h|\le T} {1 \over |v-h|^s} dh<+\infty \end{aligned}$$

using again that \(s\in (0,1)\). Now, denoting by

$$\begin{aligned} C(s,p,T,\chi ) := s^{1-p}\, (4T+2)^p J \end{aligned}$$

the above absolute positive constant depending only from s, p, T and \(\chi \), and using again the Jensen inequality, we obtain

$$\begin{aligned} I_1\ \le \ C(s,p,T,\chi )\, w^{sp}\sum _{k\in \mathbb {Z}}\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}-u\right) ^{sp}\left| ^{-}\mathcal {D}^{s}f(u)\right| ^{p}du \end{aligned}$$
$$\begin{aligned} \le C(s,p,T,\chi )\left\| ^{-}\mathcal {D}^{s}f\right\| _{p}^{p}. \end{aligned}$$
(5.9)

Now we have to study \(I_{2}\). We can write what follows

$$\begin{aligned} I_{2}\le \int _{\mathbb {R}}\left( \int _{x-1/w}^{x}\frac{\sum _{k\in A} \left| w\int _{k/w}^{(k+1)/w}f(u)du\right| \left| \chi \left( wx-k\right) - \chi \left( wy-k\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx. \end{aligned}$$

Since \(y\in \left( x-1/w,x\right) \), we have

$$\begin{aligned}{} & {} \left| A\right| \le \left| \left\{ k\in \mathbb {Z}:-T+wy<k<T+wx\right\} \right| \\{} & {} \quad \le \left| \left\{ k\in \mathbb {Z}:-T+w\left( x-\frac{1}{w}\right)<k<T+wx\right\} \right| \ =: |A_1| \end{aligned}$$

and consequently

$$\begin{aligned} I_{2}\le \int _{\mathbb {R}}\left( \int _{x-1/w}^{x}\frac{\sum _{k\in A_{1}} \left| w\int _{k/w}^{(k+1)/w}f(u)du\right| \left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx \end{aligned}$$

and since the set \(A_{1}\) does not depend on y we have, using Fubini-Tonelli theorem, that

$$\begin{aligned} I_{2}\le \int _{\mathbb {R}}\left( \sum _{k\in A_{1}}\left| w\int _{k/w}^{(k+1) /w}f(u)du\right| \int _{x-1/w}^{x}\frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx. \end{aligned}$$

Now, arguing as in (5.4), we have

$$\begin{aligned} 1\ \le \ |A_1|\ \le \ 2T+2, \end{aligned}$$

so using Jensen inequality, Fubini-Tonelli theorem, and the identity (5.6)

$$\begin{aligned}{} & {} I_{2}\le (2T+2)^p \int _{\mathbb {R}}\sum _{k\in A_{1}}\left| w\int _{k/w}^{(k+1)/w} \left( \frac{k+1}{w}-u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \left( \int _{x-1/w}^{x}\frac{\left| \chi \left( wx-k\right) -\chi \left( wy-k\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx \\{} & {} \quad \le (2T+2)^p \sum _{k\in \mathbb {Z}}\left| w\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}- u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p}\ \\{} & {} \qquad \cdot \int _{\mathbb {R}}\left( \int _{\mathbb {R}}\frac{\left| \chi \left( wx-k\right) - \chi \left( wy-k\right) \right| }{\left| x-y\right| ^{s+1}}dy\right) ^{p}dx \\{} & {} \quad = (2T+2)^p w^{sp-1}\sum _{k\in \mathbb {Z}}\left| w\int _{k/w}^{(k+1)/w}\left( \frac{k+1}{w}- u\right) ^{s}{}^{-}\mathcal {D}^{s}f(u)du\right| ^{p} \\{} & {} \qquad \cdot \int _{\mathbb {R}}\left( \int _{\mathbb {R}}\frac{\left| \chi \left( v\right) - \chi \left( h\right) \right| }{\left| v-h\right| ^{s+1}}dv\right) ^{p}dh. \end{aligned}$$

Again, proceeding as for the estimate of the integral J, using the regularity of \(\chi \), it is simple to note that \(L:=\int _{\mathbb {R}}\left( \int _{\mathbb {R}}\frac{\left| \chi \left( v\right) -\chi \left( h\right) \right| }{\left| v-h\right| ^{s+1}}dv\right) ^{p}dh>0\) is convergent, so, again by Jensen inequality, we finally get

$$\begin{aligned} I_2\ \le \ L\, (2T+2)^p\, w^{-1}\sum _{k\in \mathbb {Z}} \left( w\int _{k/w}^{(k+1)/w}|^{-}\mathcal {D}^{s}f(u)|du\right) ^{p} \le L\, (2T+2)^p\, \left\| ^{-}\mathcal {D}^{s}f\right\| _{p}^{p},\nonumber \\ \end{aligned}$$
(5.10)

an the thesis follows arguing in the same manner with \(^{+}\mathcal {D}^{s}f\) and taking the maximum of the constants involved.

Now, we can establish the following auxiliary convergence theorem.

Theorem 13

Let \(s\in \left( 0,1\right) \), \(p\in \left[ 1,+\infty \right) \), and \(\chi \) be a given n-kernel satisfying (5.1). Assume that \(f\in C_{0}^{\infty }\left( \mathbb {R}\right) \). Then

$$\begin{aligned} \left\| K_{w}^{\chi }f-f\right\| _{s,p}\rightarrow 0 \end{aligned}$$

as \(w\rightarrow +\infty .\)

Proof

First of all, under the above assumptions we know that, by Theorem 12, \(K^\chi _w f \in {\mathcal {W}}^{s,p}(\mathbb {R})\). Since the convergence in \(L^{p}\) of the SK operators is well-known (see Corollary 5.2 of [5]) it is sufficent to show that the following quantities \([K_w^\chi f - f]^p_{\widehat{W}^{s,p}(\mathbb {R})}\), \(\Vert ^\pm \mathcal {D}^s (K_w^\chi f - f)\Vert _p^p\) go to zero as \(w\rightarrow +\infty \).

We start considering the convergence with respect the Gagliardo semi-norm. Observe that

$$\begin{aligned}{}[K_{w}^{\chi }f-f]_{\widehat{W}^{s,p}(\mathbb {R})}^{p}&=\int _{\mathbb {R}} \left[ \int _{\left| x-y\right| \ge 1}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -f\left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) +f\left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}\,dx\right. \\&\quad +\left. \int _{|x-y|<1}\frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -f\left( x\right) -\left( K_{w}^{\chi }f\right) \left( y\right) +f\left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}\,dx\,\right] dy\\&=:G_{1}+G_{2}. \end{aligned}$$

Using the change of variable \(x=z+y\) in the integrals below, and the Fubini-Tonelli theorem, we have

$$\begin{aligned} G_{1}&\le \left[ \int _{\mathbb {R}}\left( \int _{|x-y|\ge 1} \frac{\left| \left( K_{w}^{\chi }f\right) \left( x\right) -f \left( x\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dx\right) dy\right. \\&\quad \left. \int _{\mathbb {R}}\left( \int _{|x-y|\ge 1}\frac{\left| \left( K_{w}^{\chi }f\right) \left( y\right) -f\left( y\right) \right| ^{p}}{\left| x-y\right| ^{1+sp}}dx\right) dy\right] \\&\le 2^{p}\left\| \left( K_{w}^{\chi }f\right) -f\right\| _{p}^{p} \int _{\left| z\right| \ge 1}\frac{dz}{\left| z\right| ^{1+sp}}\\&=:\ C_{1}\left( s,p\right) \left\| K_{w}^{\chi }f -f\right\| _{p}^{p}. \end{aligned}$$

Now, proceeding similarly to above, let us consider

$$\begin{aligned} G_2= & {} \int _{\mathbb {R}}\int _{|z|<1 }\frac{\left| \left( K_{w}^{\chi }f\right) \left( y+z\right) -f\left( y+z\right) -\left( K_{w}^{\chi }f\right) \left( y\right) +f\left( y\right) \right| ^{p}}{\left| z\right| ^{1+sp}}dzdy \\= & {} \int _{\mathbb {R}}\int _{|z|<1}\left| \int _{0}^{1}\left[ \left( wK_{w}^{\chi ^{\prime }} f\right) \left( y+tz\right) -f^{\prime }\left( y+tz\right) \right] dt\right| ^{p}\frac{1}{\left| z\right| ^{1+(s-1)p}}dzdy \\\le & {} \int _{\mathbb {R}}\int _{|z|<1}\int _{0}^{1}\left| \left( wK_{w}^{\chi ^{\prime }} f\right) \left( y+tz\right) -f^{\prime }\left( y+tz\right) \right| ^{p}\frac{1}{\left| z\right| ^{1+(s-1)p}}dtdzdy \\\le & {} \left\| \left( K_{w}^{\chi }f-f\right) ^{\prime }\right\| _{p}^{p} \int _{|z|<1}\frac{1}{\left| z\right| ^{1+(s-1)p}}dz\ =:\ C_{2}\left( s,p\right) \left\| \left( K_{w}^{\chi }f-f\right) ^{\prime }\right\| _{p}^{p} \end{aligned}$$

and since \(f \in C_{0}^{\infty }\left( \mathbb {R}\right) \) it also belongs to the usual Sobolev space \(W^{1,p}\left( \mathbb {R}\right) \) and since \(\chi \) is a n-kernel, by Theorem 3, we get the wanted convergence of \(G_1+G_2\).

Now we have to estimate the quantity

$$\begin{aligned}{} & {} \Vert ^\pm {{\mathcal {D}}}^s (K_w^\chi f - f)\Vert _p^p= \int _{\mathbb {R}}\left| ^{\pm }\mathcal {D}^{s}\left( K_{w}^{\chi }f-f\right) \right| ^{p}dx=\int _{\mathbb {R}}\left| ^{\pm }D^{s}\left( K_{w}^{\chi }f-f\right) \left( x\right) \right| ^{p}dx \\{} & {} \quad =\int _{\mathbb {R}}\left| ^{\pm }I^{1-s}\left( \left[ K_{w}^{\chi }f-f\right] ^{\prime }\right) \left( x\right) \right| ^{p}dx \end{aligned}$$

where the second identity follows from the fact that if a function is sufficiently smooth, then the weak fractional derivative is the classical fractional derivative almost everywhere; the third identity follows from Theorem 8. We focus only for the case \(^{-}I^{1-s}\) since the other one is similar.

Note that

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\left| ^{-}I^{1-s}\left( \left[ K_{w}^{\chi }f-f\right] ^{\prime }\right) \left( x\right) \right| ^{p}dx=\frac{1}{\Gamma \left( 1-s\right) ^{p}}\int _{\mathbb {R}}\left| \int _{-\infty }^{x}\frac{\left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( y\right) }{\left( x-y\right) ^{s}}dy\right| ^{p}dx\nonumber \\{} & {} \quad =\frac{s^{p}}{\Gamma \left( 1-s\right) ^{p}}\int _{\mathbb {R}}\left| \int _{-\infty }^{x}\frac{\left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) }{\left( x-y\right) ^{s+1}}dy\right| ^{p}dx \nonumber \\{} & {} \quad \le \frac{s^{p}}{\Gamma \left( 1-s\right) ^{p}}\int _{\mathbb {R}}\left( \int _{-\infty }^{x}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx\nonumber \\{} & {} \quad \le \frac{s^{p}2^{p-1}}{\Gamma \left( 1-s\right) ^{p}}\left[ \int _{\mathbb {R}}\left( \int _{-\infty }^{x-1}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}\right. \\{} & {} \qquad +\int _{\mathbb {R}}\left( \int _{x-1}^{x}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}=:\frac{s^{p}2^{p-1}}{\Gamma \left( 1-s\right) ^{p}}\left\{ H_1+H_2 \right\} .\nonumber \end{aligned}$$
(5.11)

Now, we have

$$\begin{aligned}{} & {} \left( \int _{-\infty }^{x-1}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p} \\{} & {} \quad \le 2^{p-1}\left[ \frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) \right| ^{p}}{s} +\left( \int _{1}^{+\infty }\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x-z\right) \right| }{z^{s+1}}dz\right) ^{p}\right] \end{aligned}$$

hence, by Jensen inequality and Fubini-Tonelli theorem, we obtain

$$\begin{aligned} H_1\ {}&=\ \int _{\mathbb {R}}\left( \int _{-\infty }^{x-1}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p} \nonumber \\&\le 2^{p-1}\left[ \int _{\mathbb {R}}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) \right| ^{p}}{s}dx + \int _{\mathbb {R}}\left( \int _{1}^{+\infty }\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x-z\right) \right| }{z^{s+1}}dz\right) ^{p}dx\right] \nonumber \\&\le 2^{p-1}\left[ \int _{\mathbb {R}}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) \right| ^{p}}{s}dx +\frac{1}{s^{p-1}}\int _{\mathbb {R}}\int _{1}^{+\infty }\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x-z\right) \right| ^{p}}{z^{s+1}}dzdx\right] \nonumber \\&=2^{p-1}\left[ \frac{1}{s}+\frac{1}{s^{p}}\right] \left\| K_{w}^{\chi }f-f\right\| _{p}^{p}. \end{aligned}$$
(5.12)

Now we have to estimate \(H_2\). From Fubini-Tonelli theorem and using Theorem 1 (several times), we have

$$\begin{aligned}{} & {} \int _{\mathbb {R}}\left( \int _{x-1}^{x}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( y\right) \right| }{\left( x-y\right) ^{s+1}}dy\right) ^{p}dx \\{} & {} \quad =\int _{\mathbb {R}}\left( \int _{0}^{1}\frac{\left| \left[ K_{w}^{\chi }f-f\right] \left( x\right) -\left[ K_{w}^{\chi }f-f\right] \left( x-z\right) \right| }{z^{s+1}}dz\right) ^{p}dx \\{} & {} \quad =\int _{\mathbb {R}}\left( \int _{0}^{1}\frac{1}{z^{s}}\left| \int _{0}^{1}\left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( x-zt\right) dt\right| dz\right) ^{p} \\{} & {} \quad \le \int _{\mathbb {R}}\left( \int _{0}^{1}\frac{1}{z^{s}}\left[ \int _{0}^{1}\left| \left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( x-zt\right) \right| dt\right] dz\right) ^{p}dx \\{} & {} \quad =\int _{\mathbb {R}}\left( \int _{0}^{1}\left[ \int _{0}^{1}\frac{1}{z^{s}}\left| \left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( x-zt\right) \right| dz\right] dt\right) ^{p}dx \\{} & {} \quad \le \left( \int _{0}^{1}\left( \int _{\mathbb {R}}\left( \int _{0}^{1}\frac{1}{z^{s}}\left| \left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( x-zt\right) \right| dz\right) ^{p}dx\right) ^{1/p}dt\right) ^{p} \\{} & {} \quad \le \left( \int _{0}^{1}\int _{0}^{1}\frac{1}{z^{s}}\left( \int _{\mathbb {R}}\left| \left[ K_{w}^{\chi }f-f\right] ^{\prime }\left( x-zt\right) \right| ^{p}dx\right) ^{1/p}dzdt\right) \\{} & {} \quad \le \ \frac{1}{1-s} \left\| \left( K_{w}^{\chi }f-f\right) ^{\prime }\right\| _{p}^{p}\end{aligned}$$

and so, again, the convergence follows by Theorem 3.\(\square \)

Theorem 14

Let \(\chi \) be a n-kernel, \(n \ge 1\), satisfying (5.1). Moreover, let \(0<s<1\) and \(p\in \left( 1,+\infty \right) \). Then, for every \(f\in {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \) we have

$$\begin{aligned} \left\| K_{w}^{\chi }f-f\right\| _{s,p}\rightarrow 0 \end{aligned}$$

as \(w\rightarrow +\infty .\)

Proof

Let \(f\in {\mathcal {W}}^{s,p}\left( \mathbb {R}\right) \) be fixed. From Lemma 2, we know that for every \(\varepsilon >0\) we can find a \(g\in C_{0}^{\infty }\left( \mathbb {R}\right) \) such that \(\left\| f-g\right\| _{s,p}<\varepsilon \). This means that:

$$\begin{aligned} \Vert f-g\Vert _p< \varepsilon , \quad \Vert ^+{{\mathcal {D}}}^s(f-g)\Vert _p^p<\varepsilon , \quad \Vert ^-{{\mathcal {D}}}^s(f-g)\Vert _p^p<\varepsilon . \end{aligned}$$

Now, using the triangle inequality of the norms, the above property, and the estimate established in Theorem 12 we can write what follows:

$$\begin{aligned} \Vert K^\chi _w f - f \Vert _{s,p} \le \Vert K^\chi _w f - K^\chi _w g \Vert _{s,p} + \Vert K^\chi _w g - g \Vert _{s,p} + \Vert g - f \Vert _{s,p} \\ \le \ \Vert K^\chi _w (f - g) \Vert _{s,p} + \Vert K^\chi _w g - g \Vert _{s,p} + \varepsilon \ \\ \le \widetilde{C}(s,p,\chi ) \left\{ \Vert f-g\Vert _p + \Vert ^+{{\mathcal {D}}}^s(f-g)\Vert _p^p + \Vert ^-{{\mathcal {D}}}^s(f-g)\Vert _p^p \right\} + \Vert K^\chi _w g - g \Vert _{s,p} + \varepsilon \\ \le \ \varepsilon \left\{ 3\, \widetilde{C}(s,p,\chi ) + 1 \right\} + \Vert K^\chi _w g - g \Vert _{s,p}. \end{aligned}$$

Now, the proof immediately follows by Theorem 13 and assuming \(w>0\) sufficiently large.

From Theorem 12 we observe that we are able to bound the Gagliardo semi-norm and the norm of the weak fractional derivative of the operators \(K_{w}^{\chi }f\) in terms of the \(L^p\) norm of the weak fractional derivative of f. From this fact and from Remark 10, it is quite simple to deduce that the previous arguments hold also if \(f\in \widetilde{W}^{s,p}\left( \mathbb {R}\right) \). Indeed, we have the following corollary:

Corollary 1

Let \(\chi \) be a n-kernel, \(n \ge 1\), satisfying (5.1). Moreover, let \(0<s<1\) and \(p\in \left( 1,+\infty \right) \) Then, for every \(f\in \widetilde{W}^{s,p}\left( \mathbb {R}\right) \) we have

$$\begin{aligned} \left\| K_{w}^{\chi }f-f\right\| _{\widetilde{W}^{s,p}\left( \mathbb {R}\right) }\rightarrow 0 \end{aligned}$$

as \(w\rightarrow +\infty .\)

6 Future works

The results here established seem to be (from what we know) the first approach to the study of sampling-type operators in the setting of fractional Sobolev spaces, and hence they can open the way to new theoretical and applied researches.

Indeed, as future studies, we would like to face some interesting and delicate problems of Approximation Theory in the context of tight Sobolev spaces, such as the study of the so-called saturation order. This kind of results can be useful in order to understand what are the best possible reconstruction performances of the considered approximation operators.

Another interesting problem is to consider the above approximation problems in other types of fractional Sobolev spaces (non-equivalent, in general, to the tfSs); this can be useful from both the theoretical and the applicative point of view. Indeed, it is well-known that, there exist definitions of fractional Sobolev spaces deeply studied for applications (e.g., in image processing) that are not equivalent to the spaces considered in this paper.

As an example, we can consider the well-known fractional Sobolev spaces defined as follows:

$$\begin{aligned} \mathbf{W^{s,p}}(\mathbb {R}):=\left\{ u\in L^{p}(\mathbb {R}):\,\int _{\mathbb {R}}\left( 1+\left| \xi \right| ^{sp}\right) \left| \hat{u}(\xi )\right| ^{p}d\xi <+\infty \right\} \end{aligned}$$
(6.1)

where \(1 \le p \le +\infty ,\,s>0\) and \(\hat{u}(\xi )\) is the usual (distributional) Fourier transform of u. It is a known fact that this spaces have interesting applications in different subjects and that they are not equivalent to the GSs if \(p\ne 2\).