Mass Evolution of Schwarzschild Black Holes

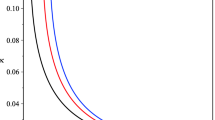
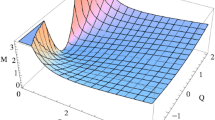
Abstract

In the classical theory of general relativity, black holes can only absorb and not emit particles. When quantum mechanical effects are taken into account, then the black holes emit particles as hot bodies with temperature proportional to κ, its surface gravity. This thermal emission can lead to a slow decrease in the mass of the black hole, and eventually to its disappearance, also called black hole evaporation. This characteristic allows us to analyze what happens with the mass of the black hole when its temperature is increased or decreased and how the energy is exchanged with the external environment. This paper has the aim to make a discussion about the mass evolution, due to Hawking radiation emission, of Schwarzschild black holes with different initial masses and external conditions as the empty space, the cosmic microwave background with constant temperature, and with temperature varying in accordance with the eras of the universe. Our main contributions are to take into account these variations in the temperature of the cosmic background radiation and use them into the black holes dynamics and also to analyze the asymptotic behavior of the solutions. As a result, we have the complete evaporation of the black holes in most cases, although their masses can increase in some cases, and even diverge for specific conditions.

Appendix: Thermal Evolution of the Universe

The thermal evolution of the universe can be viewed using the flat universe model (ϰ = 0 in the Friedmann’s Eqs. (31) and (32)) [2, 4, 41]. In the present appendix, we are going to introduce the Friedmann-Lemaître-Robertson-Walker (FLRW) metric, Friedmann’s equations, energy momentum tensor for a perfect fluid, and the necessary approximations to this model.

We know that the universe is homogeneous and isotropic and evolves in time. To describe the universe, we use the FLRW metric

$$ ds^{2} =\! - dt^{2} \!+ a(t)^{2} \left[ \frac{dr^{2}}{\left( 1 - \varkappa r^{2}\right)} + r^{2} \left( d \theta^{2} + \sin^{2} \theta d \phi^{2} \right)\right] , $$

(30)

where a(t) is the expansion factor of the universe, r is the radial coordinate, 𝜃 and ϕ are the angular spherical coordinates, and ϰ¹⁰ is a parameter related with the curvature and with dimension L^− 2. The perfect fluid consideration¹¹ as curvature generator and the Einstein’s equations lead us to the so-called Friedmann’s equations, and these equations define the time evolution of matter and energy. They can be written as

$$ \begin{array}{@{}rcl@{}} \left( \frac{\dot{a}}{a}\right)^{2} & =& \frac{8 \pi}{3} \rho - \frac{\varkappa}{a^{2}} , \end{array} $$

(31)

$$ \begin{array}{@{}rcl@{}} \frac{\ddot{a}}{a} & =& - \frac{4 \pi}{3} \left( \rho + 3 p \right) , \end{array} $$

(32)

with $\dot {a} = \frac {d a}{d t}$, ρ the matter density and p its pressure. Besides Friedmann’s equations, we can use some properties of the energy-momentum tensor and compute

$$ \nabla_{\mu} T^{\mu}_{0} = \partial_{0} (- \rho) + \left( {\varGamma}^{1}_{0 1} + {\varGamma}^{2}_{0 2} + {\varGamma}^{3}_{0 3}\right)(- \rho) - \left( {\varGamma}^{1}_{0 1} \!+ {\!\varGamma}^{2}_{0 2} + {\varGamma}^{3}_{0 3}\right) p . $$

(33)

Since ∇_μT^μν = 0, we have

$$ \begin{array}{@{}rcl@{}} - \partial_{t} \rho - \frac{3}{2} \frac{\partial_{t} a^{2}}{a^{2}} \rho = \frac{3}{2} \frac{\partial_{t} a^{2}}{a^{2}} p . \end{array} $$

(34)

Note that, writing ∂_ta³ = 3a²∂_ta and ∂_ta² = 2a∂_ta, we get the following state equation

$$ \frac{d (a^{3} \rho)}{d t} = - p \frac{d a^{3}}{d t} . $$

(35)

It is worthy to say that Eq. (35) does not replace Friedmann’s equations because, although it provides a temporal evolution of the matter and energy content of the universe, it is not straightly related to the universe curvature.

The division of the matter and energy content of the universe is radiation, matter, and dark energy. The radiation includes the photons, the cosmic microwave background, the gravitons, and the neutrinos, i.e., particles that satisfies the state equation $p_{R} = \frac {\rho _{R}}{3}$. By matter, we refer to hadronic and dark matter¹², entities whose pressure will be negligible when compared to the density: p_M = 0. And finally, the dark energy¹³ satisfies p_Λ = −ρ_Λ. Given those state equations, we can integrate Eq. (35) and obtain

$$ \begin{array}{@{}rcl@{}} \rho_{R} & =& \rho_{R_{0}} \left( \frac{a_{0}}{a}\right)^{4} \end{array} $$

(36)

$$ \begin{array}{@{}rcl@{}} \rho_{M} & =& \rho_{M_{0}} \left( \frac{a_{0}}{a}\right)^{3} \end{array} $$

(37)

$$ \begin{array}{@{}rcl@{}} \rho_{{\varLambda} } & =& \rho_{{\varLambda}_{0}} , \end{array} $$

(38)

where $\rho _{i_{0}}$ is the radiation density (i = R), matter density (i = M) and dark energy density (i = Λ) today and a₀ is the expansion factor of the universe today.

Using the experimental values, found in [45], to the radiation, matter, and dark energy, we compute their respective present densities $\rho _{R_{0}} \simeq 4.6 \cdot 10^{- 31} \frac {kg}{m^{3}}$, $\rho _{M_{0}} \simeq 2.7 \cdot 10^{- 27} \frac {kg}{m^{3}}$ and $\rho _{{\varLambda }_{0}} \simeq 5.8 \cdot 10^{- 27} \frac {kg}{m^{3}}$ and we plot the evolution of the densities ρ_R, ρ_M, and ρ_Λ versus the expansion factor of the universe normalized $\frac {a}{a_{0}}$, viewed in Fig. 12. Observe that we have highlighted three different phases, that we called of radiation era, matter era, and dark energy era. All phases are dominated by matter or energy content of the universe. They are the so-called universe eras. The divisions are represented by the vertical dashed lines, where the densities intersect each other: $p_{1} = \frac {a}{a_{0}} = \frac {\rho _{R_{0}}}{\rho _{M_{0}}} \simeq 1.7 \cdot 10^{-4}$ and $p_{2} = \frac {a}{a_{0}} = \left (\frac {\rho _{M_{0}}}{\rho _{{\varLambda }_{0}}}\right )^{1/3} = 7.7 \cdot 10^{-1}$.

Fig. 12

Density evolution of radiation, matter and dark energy according to expansion factor $\frac {a}{a_{0}}$ of the universe. Note that we have used the logarithm scale in both axes

Still using the experimental values of Reference [45], we can say that nowadays the universe is flat, in other words, the curvature parameter ϰ = 0. This statement can be viewed rewriting Eq. (31) as

$$ {\varOmega} - 1 = \frac{\varkappa}{H^{2} a^{2}} , $$

(39)

where ${\varOmega } = \frac {8 \pi }{3 H^{2}} \rho = \frac {\rho }{\rho _{crit}}$ is the density parameter, $\rho _{crit} = \frac {3 H^{2}}{8 \pi }$ is the critical density, and $H = \frac {\dot {a}}{a}$ the Hubble’s parameter. As Ω is the sum of the parameters of radiation, matter, and dark energy densities, using their experimental values we can see that

$$ {\varOmega} = {\varOmega}_{R} + {\varOmega}_{M} + {\varOmega}_{{\varLambda} } \simeq 5.46 \cdot 10^{-5} + 0.315 + 0.685 \sim 1 , $$

(40)

which implies, by Eq. (39), that ϰ = 0.

Now we are going to connect the time evolution of the matter and energy content of the universe with Einstein’s equations. To do this, we just need to replace the results (36-38) in Friedmann’s Eqs. (31) and (32). In order to do this, let’s then consider a flat universe (ϰ = 0) not just today but throughout the evolution of the universe (as commented above)¹⁴. Thus, replacing the densities in the 1st Friedmann’s Eqs. (31), we find

$$ \begin{array}{@{}rcl@{}} \left( \frac{a}{a_{0}}\right) & =& \left( \frac{32 \pi}{3} \rho_{R_{0}}\right)^{1/4} t^{1/2} , t \in (0, t_{p_{1}}) \end{array} $$

(41)

$$ \begin{array}{@{}rcl@{}} \left( \frac{a}{a_{0}}\right) & =& (6 \pi \rho_{M_{0}})^{1/3} t^{2/3} , t \in (t_{p_{1}}, t_{p_{2}}) \end{array} $$

(42)

$$ \begin{array}{@{}rcl@{}} \left( \frac{a}{a_{0}}\right) & =& \textnormal{Exp}\left[\left( \frac{8 \pi}{3} \rho_{{\varLambda}_{0}} \right)^{1/2} t\right] , t \in (t_{p_{2}}, \infty), \end{array} $$

(43)

where, recovering the units using SI, $t_{p_{1}} = \left [\frac {3}{32 \pi G \rho _{R_{0}}} \left (\frac {\rho _{R_{0}}}{\rho _{M_{0}}}\right )^{4}\right ]^{1/2} \simeq 9.3 \cdot 10^{11} s$ and $t_{p_{2}} = \frac {1}{(6 \pi G \rho _{{\varLambda }_{0}})^{1/2}} \simeq 3.7 \cdot 10^{17} s$, and these values were obtained replacing p₁ and p₂ respectively in (41) and (42). Note that we integrate indefinitely, in a and in t, the expressions (41) and (42), disregarding any integration constant. We have also integrated indefinitely in (43), defining a₀ as integration constant. This is an approximation to the solution of (31) in terms of dominance intervals of radiation, matter, and dark energy, and the result stays undefined due to a lack of the integration constants. However, since we are not interested in the continuity of the solution to Eq. (31), but in its form, our result is convenient to our analysis.

Finally, we need to relate the temperature with the universe densities. We know that a black body emits radiation with energy density according to Stefan-Boltzmann’s law, i.e., L ∝ T⁴. We saw that the density radiation of the universe can be written as $\rho _{R} \propto \left (\frac {a_{0}}{a}\right )^{4}$. Thus, doing a dimensional analogy, we can say that the radiation temperature evolves as

$$ T_{R} = T_{\text{CMB}} \left( \frac{a_{0}}{a}\right) , $$

(44)

where T_CMB is the temperature of the cosmic microwave background today. Therefore, considering that the radiation has temperature T_i, with i = R,M,Λ, related to the fraction $\frac {a_{0}}{a}$, and that this fraction assumes different expressions according to the universe eras: radiation (R), matter (M), and dark energy (Λ), we have

$$ \begin{array}{@{}rcl@{}} T_{R} & =& \frac{T_{\text{CMB}}}{\left( \frac{32 \pi}{3} \rho_{R_{0}}\right)^{1/4}} \frac{1}{t^{1/2}}, t \in (0, t_{p_{1}}) , \\ T_{M} & =& \frac{T_{\text{CMB}}}{\left( 6 \pi \rho_{M_{0}}\right)^{1/3}} \frac{1}{t^{2/3}} , t \in (t_{p_{1}}, t_{p_{2}}) , \\ T_{{\varLambda} } & =& T_{\text{CMB}} \textnormal{Exp} \left[- \left( \frac{8 \pi}{3} \rho_{{\varLambda}_{0}}\right)^{1/2} t\right] , t \in (t_{p_{2}}, \infty) . \end{array} $$

(45)

Hence, we can use these results in Section 6 assuming that the black hole is exchanging energy with the universe, considering the three different ways of thermal evolution.