Exploring spherical units as perceptual clues in enumerating 3D arrays

Abstract

This study continues an investigation of how spherical units, compared to cubical units, can facilitate students’ units-locating and organizing units in composites. We analyze how Norwegian grade 3 students enumerate 3D arrays with cubical and spherical units. Our results show how spherical units can act as perceptual clues that facilitate cognitive processes, underlying students’ strategies in the enumeration of 3D arrays. In particular, the results show how spherical units facilitate the units-locating process, which, in turn, supports processes of organizing-by-composites and spatial structuring of the array, in the action of developing a proper iterative strategy such as layer-based thinking.

Introduction

Battista (2004, 2010) claimed that five cognitive processes are essential for enumerating units in 3D arrays: Units-locating, spatial structuring, organizing-by-composites, forming and using mental models and abstraction. An enumeration strategy is based on and considered to be the result of these cognitive processes. In Alstad et al. (2021) we showed how students’ difficulties in enumerating a 2D representation of a 3D array can be traced to difficulties in the process of units-locating (Battista, 2003, 2004), with the consequence of applying double and triple counting. In the present study, we use the notion of perceptual clues (Battista, 2010) to take a closer look at how a spherical unit-representation, compared to a standard cubical unit-representation, can facilitate students’ strategies in enumerating 2D representations of 3D arrays.

We follow a purposive sampling strategy (Bryman, 2016) where we interview three groups of grade 3 students, selected on the basis of their written work. Grade 3 students have not yet been introduced to enumerating 3D arrays and are therefore more likely to apply informal strategies in solving enumeration tasks. The aim of comparing students' strategies in enumerating 3D arrays with spherical and cubical unit-representations at this stage is to provide rich opportunities to explore how spherical unit-representations may act as perceptual clues in the enumeration process. We follow a principle of variation theory (Marton et al., 2004) that states, “…in order to experience something, a person must experience something else to compare it with” (p. 16). Hence, in order to experience and understand the role of a spherical unit-representation, we compare it with a cubical unit-representation. We address the research question: How can spherical unit-representations, compared to cubical unit-representations, provide perceptual clues that support students in developing strategies for enumerating units in 3D arrays?

Before we explain the details of our method, we elaborate on previous research on students’ difficulties in enumerating cubes in 3D arrays and perceptual clues for facilitating the enumeration of 3D arrays. Then we present the five cognitive processes essential for enumerating units in a 3D array (Battista, 2004), which will later be used as a framework for organizing and guiding our analyses. After presenting the method and results, we conclude by discussing our findings and their implications for further research.

Previous research

Difficulties in enumerating cubes in 3D arrays

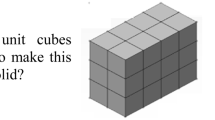
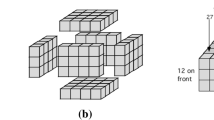
Enumerating unit cubes in 3D arrays is a challenging task for many students. A 3D array contains non-visible interior cubes that need to be taken into account. Student errors stem mostly from counting only what is visible in the 2D representation or from a more general lack of coordination of the units, including not counting interior cubes (Ben-Haim et al., 1985). As a result, a common erroneous strategy is to count 2D units, i.e., the visual faces of a unit cube, instead of 3D units. This will result in some cubes being double or triple counted (Fig. 1). Double and triple counting units are well documented in the research literature (Alstad et al., 2021; Battista, 1999; Battista & Clements, 1996; Ben-Haim et al., 1985; Finesilver, 2017; Kara et al., 2012; Tan Sisman & Aksu, 2016). Students who double and triple count have not been able to locate the 3D units in the array, due to various reasons. Ben-Haim et al. (1985) assumed that students in these cases perceived the pictures as 2D and failed to see the three-dimensionality of the array. Battista (2004) concluded that double and triple counting occurred because students were not capable of coordinating the different viewpoints of the array. Tan Sisman and Aksu (2016) and Finesilver (2017) argued that these counting strategies were connected to a lack of spatial visualization or spatial structuring, and that the students relied too much on counting. They found, like Ben-Haim et al. (1985), that the students were interacting with a 2D space instead of a 3D space. Similar findings have been made in research where students encountered physical objects that could be picked up and rotated (Finesilver, 2015, 2017).

Fig. 1

Double and triple counting cubes

Findings from previous research show that it is a demanding task for many students to mentally organize a 3D array, confirming the need for further explorations of how students’ abilities to enumerate 3D arrays can be facilitated.

Spatial abilities involved in enumerating cubes in 3D arrays

Research has shown that interpreting 2D representations of 3D arrays is difficult (Alstad et al., 2021; Battista & Clements, 1996; Ben-Haim et al., 1985). Several researchers have argued that these difficulties stem from a lack of some type of spatial ability. Spatial abilities are considered as “a form of mental activity that enables individuals to create spatial images and to manipulate them in solving various practical and theoretical problems.” (Pittalis & Christou, 2010, p. 191). Spatial abilities consist of several components, including spatial visualization and spatial structuring. Spatial visualization involves creating a mental image of an object which can be rotated and manipulated (Battista et al., 2018; Pittalis & Christou, 2010). Spatial structuring involves the mental act of constructing an organization or form for 3D objects (Battista, 1999; Battista & Clements, 1996). In tasks where pupils have to enumerate cubes in 3D arrays, spatial structuring can be explained as how one “sees” the organization of the cubes in the array. For instance: A 3 × 3 × 3 array of cubes might be spatially structured as three horizontal layers of nine cubes, or as nine columns of three cubes. To carry out this mental act, the students need to make sense of the task and the visual 2D representation in such a way that they can discern the structural and geometrical properties and mentally manipulate the object based on its 2D representation, which again involves an act of spatial visualization.

Perceptual clues for facilitating enumeration of 3D arrays

Battista (2010) brought forward the notion of perceptual clues. He suggested that using colored cubes or computer animation might provide visual hints that can support students in enumerating units in 3D arrays. Finesilver (2017) examined the hypothesis of perceptual clues by using layers of different colors in 3D arrays built of 1 cm³ multilink cubes. She found that students who tended to count only faces in enumeration tasks, moved toward a layer-based thinking when the arrays came in different colored layers. Vasilyeva et al. (2013) used grids as perceptual clues and presented students with both gridded and non-gridded 2D representations of 3D arrays. They found that students tended to apply more appropriate strategies when presented with gridded representations of 3D arrays. These findings suggest the possibility to support students’ enumeration strategies by implementing different perceptual clues in the design of both concrete models as well as 2D representations of 3D arrays.

In previous research, focus has mainly been on enumerating cubical units (Smith & Barrett, 2017). One recent exception is Alstad et al. (2021), where a grade 3 class was observed working on enumeration tasks with cubical and spherical unit-representations. Findings from the study revealed that students’ difficulties in enumerating units in 3D arrays can be connected to difficulties in the units-locating process. These difficulties led to students applying double and triple counting. In addition, the findings implied that spherical units may act as perceptual clues in the units-locating process and in assembling units into relevant composites. The present study builds on Alstad et al. (2021) in that it further explores how cubical and spherical unit-representations might support or hinder students’ enumeration processes. This study also adds an in-depth view on how children perceive 2D representations of 3D arrays built of different units and how this is connected to their spatial structuring of 3D arrays. More specifically we investigate how spherical units can act as perceptual clues to support the development of strategies for enumerating units in 3D arrays.

Analytical approach

Spatial structuring is regarded as one of the spatial abilities necessary for enumerating units in a 3D array. Spatial structuring is also formulated as one of the five essential cognitive processes underlying students’ enumeration strategies (Battista, 2004). The other four processes are units-locating, organizing-by-composites, forming and using mental models and abstraction. All five cognitive processes are elaborated on in the next section and will provide the basis for our analytical framework.

Five underlying cognitive processes

In order to identify how spherical representations can provide perceptual clues, we will use enumeration strategies to describe students’ interpretations of the 2D representations. An enumeration strategy is based on and considered to be the result of underlying cognitive processes (Battista, 2004). For instance, a student using the strategy of counting units on one side of the array and then using multiplication or repeated addition to find the total number of units in the array, can be described as spatially structuring the array in terms of layers. This again is a result of properly developed processes of units-locating and organizing-by-composites. Thus, strategies for enumerating 3D arrays and cognitive processes needed to enumerate 3D arrays are closely linked. Battista (2004, 2010) claimed that five cognitive processes are essential for enumerating units in 3D arrays: Units-locating, spatial structuring, organizing-by-composites, forming and using mental models and abstraction.

Units-locating involves discerning the basic unit of the array and coordinating the units’ locations along the dimensions that frame an array. Consequently, units-locating involves the coordination of different viewpoints of the array. In the spatial structuring process, students need to be able to locate the units and establish a relationship between them. They need to identify, interrelate, and organize the object’s components. Spatial structuring is how a student mentally perceives the structure of the object. For instance: a 3 × 3 × 3 array of cubes can be perceived as three horizontal or vertical layers of nine cubes, or as nine columns or rows of three cubes. Some ways of spatially structuring the array can be helpful in finding the correct number of cubes, while other ways of structuring might be obstructive. Organizing-by-composites involves the combination of basic units into a composite unit (a unit of units) that can be used to generate the complete array via iteration. In the process of forming and using mental models, students create and use mental images as representations of the perceived structure of the 3D figure. When a student has developed a proper mental model of a 3D figure, the model can be activated to visualize, comprehend, and reason about the figure at hand. Abstraction is “the process by which the mind selects, coordinates, unifies, and registers in memory a collection of mental items or acts that appear in the attentional field” (Battista, 2004, p. 186). In abstracting a 3D array, a student does not have to see the full picture of, or work concretely with, the 3D array to successfully enumerate it (Kim et al., 2017). Coming to see the three-dimensionality in a 2D representation of an array (Fig. 1) involves an act of abstraction.

In a previous study, we examined how unit-representations might be linked to one specific process, the units-locating process (Alstad et al., 2021). In the present study, we broaden our perspective, intending to contribute to research on enumerating 3D arrays, by exploring how spherical units may act as perceptual clues to support different cognitive processes, underlying students’ strategies for enumerating units in 3D arrays. Building on Battista’s theory, that students’ strategies can be explained with five cognitive processes, we have the expectation that a change in students’ enumeration strategies involves a change in the underlying cognitive processes and vice versa.

Method

Getting close to students’ underlying cognitive processes requires the need for in-depth data (Bryman, 2016). To this end, we adopted a purposive sampling strategy where we interviewed Norwegian grade 3 students who showed difficulties in the enumeration of 3D arrays. We chose to conduct the study on grade 3 students because they have not yet been introduced to enumerating 3D arrays, and are therefore more likely to apply informal strategies in the enumeration of the arrays.

Data collection

Data was collected in three rounds. Our first data collection was conducted during the spring of 2019 with a class of 22 grade 3 students from a small-town school in Norway. The students were organized in eleven pairs and asked to solve four tasks on worksheets (Fig. 2). We also asked students to clarify their thinking during the activity and video recorded two of the pairs. The findings from the first data collection provided the results in our previous study (Alstad et al., 2021). The findings are also included as part of the data in the present study, as they provide some important information regarding the current research question as well.

Fig. 2

Example of student worksheet from first data collection

The second data collection took place during spring 2021. A different class with 17 grade 3 students, organized in seven pairs and one group of three, participated in solving four similar enumeration tasks. Due to the local COVID19 situation at the time, we were not allowed to be present in the classroom during this data collection. Data in the second round consists of written answers on worksheets, in addition to audio recordings of each group. The second data collection laid the basis for the third data collection, where we interviewed two pairs and the group of three students from the second data collection to elaborate on their strategies in the four enumeration tasks. The two pairs and the group, which we will refer to as the groups, were purposively selected, according to our research question, and the interviews were video recorded. The selected groups had encountered difficulties when solving the tasks and showed differences in strategies between tasks with different unit-representations. In line with variation theory (Marton et al., 2004), it was conjectured that interviewing these students would provide information on how spherical unit-representations may have provided perceptual clues that facilitated the cognitive processes, underlying their enumeration strategies.

The tasks

Figure 3 shows the four tasks which were used in our first data collection (Alstad et al., 2021). The tasks were given one at a time in the following order: 1, 2, 3A and 4A. Before our second data collection, we decided to change two of the tasks. Task 2 and 3A were too similar, both in structure and units. Hence, we changed the arrays to be alternating in terms of unit-representations. In addition, we wanted to increase the dimensions on the fourth task to be > 2 since Task 4A did not have interior units. Task 3A, a 3 × 3 × 4 array of spheres, and Task 4A, a 2 × 5 × 4 array of cubes, was exchanged with Task 3B; a 4 × 4 × 3 array of cubes, and Task 4B; a 5 × 5 × 4 array of spheres. The second set of tasks (Fig. 4) was used in both the second and third round of data collection.

Fig. 3

Arrays for Task 1, 2, 3A, and 4A in the pilot study

Fig. 4

Arrays for Task 1, 2, 3B, and 4B in the 2^nd and 3^rd data collection

The arrays were represented with different units and different structures. The students were presented with Task 1 first. We chose to begin with a task with standard cubical units to see if any of the common misconceptions or erroneous strategies would occur. Task 2 has an identical structure to Task 1, but with spherical units. We wanted to alternate the cubical and spherical representations, thus Task 3B is represented with cubical units. The advantage of Task 1 and 2 being similar in structure is that it makes it easier to compare strategies across different unit representations. A possible disadvantage is that students can discover that the structures between two tasks are similar and therefore assume that the number of units is similar, without spatially analyzing or visualizing the structure of the array. Therefore, in Task 4B, we chose to introduce a new array, 4 × 4 × 5, which was not similar to any of the previous ones.

Method of analysis

Our analysis was organized in three steps. In the first step, we categorized the students’ enumeration strategies from the first and second data collection. Guided by Battista and Clements (1996) and Ben-Haim et al. (1985) we distinguished between successful strategies and unsuccessful strategies (Table 1). We found two successful strategies: grouping and iterating layers and grouping and iterating rows/columns. In the categorization of the unsuccessful strategies, we searched for and distinguished between students who enumerated 3D units and 2D units; students who counted only visible units and included hidden units; and students who counted only outer units and included interior units (cf. Battista & Clements, 1996; Ben-Haim et al., 1985). Details of the different strategies are provided in the results section.

Table 1 Range of enumeration strategies on each task

In the second step, we made connections between the different strategies and Battista’s cognitive processes (Battista, 2003, 2004). This analysis was based on students’ written answers from the first two rounds of data collection. In addition, we compared the students written work with audio recordings from the second data collection. This connection between strategies and underlying cognitive processes will be elaborated on in the results section. These two steps of the analysis provided an overview of enumeration strategies and underlying cognitive processes. In addition, they functioned as an instrument for the purposive sampling of the three interviews. They enabled us to locate students who had not yet developed proper enumeration strategies, and therefore would have problems with one or more of the underlying cognitive processes. In the third step, we transcribed and analyzed each video-recorded interview from the third data collection. The aim of this analysis was to scrutinize how spherical unit-representations can act as perceptual clues that support the cognitive processes, underlying students’ strategies for enumerating 3D arrays. The analysis was both chronological and thematic. Chronologically, we compared each group’s strategies between the four tasks, to account for differences in enumeration strategies between the cubical and spherical cases. As different strategies can be connected to different cognitive processes, this also provided information about what cognitive processes seemed to be activated in the enumeration of 3D arrays with either cubical or spherical unit-representations. Thematically, we looked for similarities or differences across the groups for each task to strengthen our claims on how the different unit-representations can facilitate underlying cognitive processes of students’ strategies for enumerating 3D arrays. The thematic analysis can be thought of as a synthesis.

Results

First, we will give a general overview of the enumeration strategies that we identified from the first and the second data collection (Table 1) and connect the strategies to underlying cognitive processes. Next, we use transcripts from the interviews to provide a more detailed analysis of the students’ enumeration strategies and underlying cognitive processes. Through this detailed analysis, we will compare how cubical and spherical unit-representations appeared to activate different cognitive processes connected to the enumeration of 3D arrays. Further, we will discuss how spherical unit-representations seemed to provide perceptual clues that facilitated the cognitive processes of units-locating, organizing-by-composites, and spatial structuring of the array.

An overview of students’ strategies on the written tasks

We identified eight categories of enumeration strategies from the first and second data collection (Table 1). The strategies were categorized based on Battista and Clements’ (1996) categories. Since Battista and Clements’ categories were based on students’ enumeration of cubes, we had to do some adjustments to give a more accurate description, which also fits the spherical units. In general, Battista and Clements categorized student strategies in five main categories, A-E: In strategy A the students perceive the units in the 3D array in terms of layers. In strategy B the students perceive the array as space-filling but not in terms of layers. Students who did not perceive the array as space-filling but counted outer units in one way or another were placed in category C. Strategies D and E were not evident in our study and will therefore not be elaborated in this paper. Further, Battista and Clements have several sub-categories (e.g., B1, B2 and so forth). The sub-categories relevant to our study are described in Table 1. Battista and Clements organized their categories based on whether the students were able to perceive the units in the array as space-filling or not. In clarifying the link to our research question, which deals with perceptual clues and cognitive processes, it was necessary to categorize the students' strategies more clearly linked to these processes. An adjustment of the categorization is seen through some new categories (strategy B5, F and G in Table 1).

One of the groups had a split decision and did not agree on an answer. In Table 1 this is seen as “ + 0.5”, indicating one person, but half a group. Table 1 contains strategies similar to the strategies presented in Alstad et al. (2021), but the description of the strategies is elaborated. By a more elaborated description of the strategies, we aim to clarify what each strategy entails to a greater extent. Of the eight strategies, we observed that only A1/A2 and B1 were strategies that might lead to a correct enumeration of the units in the arrays. The remaining strategies, B3 – H, were unsuccessful, but activated in various degrees the underlying cognitive processes. In our detailed analysis we will discuss how unsuccessful enumeration strategies can be a result of undeveloped underlying cognitive processes and how spherical units supported students’ underlying cognitive processes in developing successful enumeration strategies.

Connecting strategies to underlying cognitive processes

When using strategy A1/A2 or B1 (successful enumeration strategies), the students are able to locate the 3D unit, group the units into composite units that can be iterated to enumerate the array correctly, and are thus capable of spatially structuring the array properly. When using strategy B3, B4 and B5, the students are able to locate the 3D unit, and capable of grouping 3D units into composites. What makes strategy B3 unsuccessful is that students make mistakes when they try to iterate the composites to enumerate the units. This implies that the students either make some calculation errors, or that they are unsuccessful in their effort to coordinate the viewpoints of the array, thus ending up iterating the composite units incorrectly. What makes strategy B4 unsuccessful is that the students are adding an incorrect number of interior units. Using strategy B4, the students show that they are aware of interior units, but they do not know how to enumerate them. This suggests that the students are not able to establish a relationship between different units in the array, thus their spatial structuring is incoherent with the 2D representation of the 3D array. Strategy B5 is also unsuccessful. When applying this strategy, students spatially structure the array in terms of visible 3D units, not taking any hidden or interior units into account. When using strategy C2/C3 and F, students apply the process of organizing-by-composites by grouping units. However, these strategies lead to an incorrect enumeration since they are based on locating 2D units instead of 3D units. Moreover, when using strategy C2/C3, the students count hidden units, but do not take any interior units into account. This entails that they cannot establish a relationship between the units, as they spatially structure the array in terms of outer 2D units. In strategy F, the students show an awareness of interior units, but cannot enumerate these units properly. This implies that the students spatially structure the array as space-filling, but that they are not able to identify, interrelate, and organize the arrays components. In strategy G, the students count only visible 2D units, not taking hidden or interior units into account. This entails that the students are unable to locate and coordinate the units in the array, and that they spatially structure the array in terms of visible 2D units. Category H is a collection of unknown strategies that could not be categorized with any further descriptions.

In the detailed analysis that follows, we will offer extracts from the interviews that provide a more in-depth explanation of how the different strategies are connected to the underlying cognitive processes. This part of the analysis allows us to compare strategies and underlying cognitive processes across tasks with spherical and cubical unit-representations. On this basis, we discuss how spherical units can act as perceptual clues that support the development of enumeration strategies and cognitive processes.

Detailed analysis of students’ strategies and underlying cognitive processes

In this section we will take a closer look at how the three groups from our in-depth interview solved the tasks, and how their enumeration strategies were different when dealing with spherical and cubical units. First, we will give a short summary of the general findings from our analysis of the three groups. Second, some in-depth examples will be provided from each of the groups to substantiate our general findings. We will discuss how the differences in strategies can be connected to the underlying cognitive processes, and how the spherical units seemed to provide perceptual clues that facilitated the development of proper enumeration strategies.

In the transcripts, we meet seven people: the researcher (R), two students (L and K) in Group 1, two students (N and O) in Group 2, and three students in Group 3 (C, D and E. E was rather silent, and is not visible in the transcripts that follows). In Table 2 we describe how we label the various sides of the array.

Table 2 Description of different sides of an array

General findings from the three groups

An overview of the strategies of the three groups are seen in Table 3.

Table 3 Strategies of the groups during interview

As we can see in Table 3, all three groups used different strategies when working with spherical and cubical units. Group 1 used strategy B1 on three of the tasks, but their way of counting differed between the tasks with spherical and cubical units. The group used a less efficient counting strategy on Task 3B than Task 2 and 4B. Looking at the other groups, it is evident that Group 2 and 3 used more successful strategies when solving tasks with spherical units than with cubical units. These general findings imply that the spherical units somehow supported the students to make sense of and properly enumerate a 2D representation of a 3D array. The following sections will provide extracts from the conversations between the students working on some of the tasks to substantiate and elaborate on the implications of the general findings. In these sections we will shed light on the differences between the students’ strategies, how the strategies can be connected to underlying cognitive processes, and in what way the spherical units seemed to support the students in developing proper enumeration strategies.

Group 1: Spherical units as perceptual clues for spatially structuring arrays in columns

Group 1 developed a strategy of adding together composites in the form of columns for all tasks. Still, they ended up with different ways of counting the different arrays. With the cubical units they tried to enumerate the cubes by counting from the three visible sides of the array. Instead of counting columns from TL, which they did on both spherical tasks, they used a strategy in which they had to coordinate all three visible sides of the array.

• R: How many of these cubes do you need to build this figure? [giving students Task 1, Fig. 5]

Fig. 5

Task 1

• L: 3, 6, 9, 12, 15, 18 [counting in columns of three, ending up with enumerating the number of faces on RFL and LFL] (Fig. 6).

Fig. 6

L, in Group 1, counting faces on RFL and LFL in columns of three

• K: But this is the same block [pointing to the top cube in the front]. 1, 2, 3 (…) 17, 18, 19 [counting one cube at a time, not double counting the three cubes where RFL and LFL meet and counting 4 cubes in TL]. (Fig. 7)

Fig. 7

K, in Group 1, counting visible cubes

In this episode, we can see that L had some trouble in the units-locating process. She organized the units into composites, in terms of columns (Fig. 6). However, when counting the columns, she double counted some of the cubes. This indicates that she counted groups of 2D units instead of 3D units. K, on the other hand, expressed “But this is the same block”, showing that she was able to locate the 3D unit, thus avoiding double and triple counting. K did not seem to perceive the array as space-filling at first, as she did not take any of the hidden and interior units into account. She spatially structured the array in terms of 19 single, visible cubes (Fig. 7).

After a discussion, the students seemed to agree that some of the faces were connected to the same cube and that they had to consider hidden and interior units, but they had difficulties in finding a structured way of counting the cubes:

• K: Then it is… two threes…

• L: six.

• K: And this? [pointing at the back of left side of TL]. Are there six there as well?

• L: six plus six. Twelve. Plus six.

• K: Twenty-four. No. Minus three. Because these are the same [pointing towards the four cubes already marked on TL]. So, twenty-one.

• L: 21…, 22, 23, 24, 25, 26, 27.

• (…)

• L: 28, 29, 30.

The group used strategy B4, unsystematic counting. L used a pencil and tapped three times on the same cube face as she counted 22, 23, 24. This indicated that she counted in columns of three cubes from TL. She moved to another cube face and repeated the action, counting 25, 26, 27. After some discussion the two students ended up pointing three times to the center cube face on TL, counting 28, 29, 30.

Their answer was incorrect, but the group ended up showing that they perceived the array as space-filling, and they succeeded to locate the 3D unit. They struggled to count the different cubes, especially those that were hidden. Even if L and K eventually agreed that some cube faces were connected to the same cube, it was difficult for them to use this information to infer the number of cubes at the back and bottom of the array.

In Task 2 (Fig. 8) L further developed the iterative grouping strategy she used in Task 1 and found a more efficient method of counting the spherical units.

Fig. 8

Task 2

• L: 3, 6, 9, 12, 18, 21, 24, 27, 30! [pointing to the nine units on TL]

L counted in groups of three, spatially structuring the array in columns and pointing to the units in the TL for each group she counted. She made a composite unit of three units, which, when iterated, could give her the correct number of unit spheres in the array. She made a calculation error, jumping from 12 to 18, which left her with 30 instead of 27. L used a similar strategy on Task 1, where she also counted in groups of three units in a column. The difference between the enumeration processes is that she did not double or triple count any spheres in Task 2, as she did with the cubes in Task 1. In the spherical case, the group spatially structured the array as nine columns that were all counted from the TL. In the cubical case however, they also seemed to spatially structure the array in columns, but counted the cubes from all three visible sides. Looking beyond the calculation error, the group had more difficulties in enumerating the cubical 3 × 3 × 3 array than the spherical 3 × 3 × 3 array.

Group 1 seemed to have challenges with coordinating the different viewpoints of the cubical arrays and seeing which cube faces belonged to the same unit cube. On tasks with spherical units (2 and 4B, see Table 3), students did not apply double counting of units. It was interesting to observe that even though the students used the same spatial structuring for all four tasks (organizing the array in columns), they fell back to the strategy of counting units on all three sides when presented with another array with cubical units (Task 3B) and thus, making the enumeration process more complicated. Battista and Clements (1996) reported that some students see the cube faces as a medley of uncoordinated faces. Though Group 1 did show some sign of coordination with the cubical units, they had problems with interpreting the 2D cubical faces and how they relate to the unit cube. We interpret the cube faces to be an obstacle to this group for their spatial structuring. When encountering the spherical units, this obstacle was absent, and the group succeeded in the enumeration. Hence, the spherical units seemed to provide perceptual clues facilitating the cognitive processes of units-locating, organizing-by-composites, and spatial structuring of the array.

Group 2: Spherical units as perceptual clues for spatially structuring arrays in layers

Group 2 used two different strategies when solving tasks with cubical or spherical units. The group used strategy C2/C3 (counting outer 2D units) with the cubical tasks and strategy A1/A2 (repeated addition of horizontal layers) with the spherical tasks.

In Task 1, Group 2 organized composites of visible faces, which came to include double and triple counting.

• R: How many of these cubes do you need to build this figure?

• N: [counting and pointing with her fingers] … 54.

• R: That was fast. How did you think?

• N: I was thinking in nines.

• R: In nines?

• N: I did like this; 9, 18, 27 [pointing at LFL, RFL and TL] and then I took those behind, 30, eh… wait… 9, 18, 27, 36, 45, 54 under.

• R: Ok. So, you were thinking that there are nine here [pointing to LFL] and nine here [RFL] and there [TL]. And then there are three more nines that you don’t see on the back, on the side and under?

• N: Yes.

Expressing: “I took those behind”, N made the abstraction that the 2D picture is a representation of a 3D array, made up of six sides. Based on this abstraction, N counted all the visible faces of the unit cubes and discovered that there were nine faces on each side. She organized the faces into composites of nine, and added together the nines of each side, ending up in the addition, “9, 18, 27, 36, 45, 54”. However, what N did not consider was that there are interior cubes and that different faces can belong to the same unit cube. Consequently, the strategy of counting composites of faces, came to involve double and triple counting (cf. Battista, 2004).

Working on Task 2, N developed an additive layer-based strategy. Initially, the group’s first guess was that the answer was 54: the girls expressed that the task was similar to Task 1, and that the answer therefore had to be the same. Then N noticed:

• N: Wait, maybe there are only 27 because there are 9 on top [pointing on TL] and we have counted them, so those on the side don’t count [pointing to the top row of RFL]. Then it might be nine under here, and nine there. It could be that too.

• R: Ok, so do I understand you correctly that you think there might be nine in the top one here? [pointing to TL]

• N: Yes, and in the middle [pointing to Centre Horizontal Layer, CHL] and at the bottom [pointing to BL].

In this episode N spatially structured the array in terms of horizontal layers. She counted the units in the TL, grouped them into a composite unit and then used this composite unit to infer the number of spheres needed in the next two horizontal layers. The strategy is additive in that she adds the three layers together, ending up with 9 + 9 + 9 = 27.

Group 2 used strategy C2/C3 on both tasks with cubical units. When using strategy C2/C3, students are counting 2D units, in terms of cube faces located on the outside of the array. Using this strategy suggests that the cubical unit-representation did not support the students in locating the units as 3D objects, separated from each other. In addition, the students did not take into account interior units, i.e., they experienced problems with the process of spatially structuring the array. On both tasks with spherical units, the group used strategy A1/A2. Using this strategy, the students spatially structured the array in terms of layers. According to Battista, this indicates that the students show a “complete development and coordination of both the units-locating and the organizing-by-composites processes” (Battista, 2004, p. 199).

Group 3: Struggling with space-filling of 3d arrays

Group 3 used strategy F, which has similar characteristics to strategy C2/C3, in that they count the outside faces, however, the distinguishing feature is that they also considered that there were units on the inside of the array. However, as the group came to consider the interior of the 3D array, they also made visible how difficult it can be for students to visualize the interior structure of a 3D array. Group 3 struggled with this in both the cubical and spherical cases, despite that the spherical unit-representations seemed to act as perceptual clues in the students’ enumeration of units located on the outside of the 3D array. In Task 1, with the cubical units, the interior units were counted as faces:

• R: How many cubes do I need to build this figure?

• C: OK, if we take around the whole figure, then we have 18 + 18, which is 36. Because 9 + 9 is 18, and we have the ones on the backside, so then it will be 18. Then it will be … we also need the ones which are under!

• D: There is one inside!

• C: 36 … 36 + 18, that is … 46 … 54! 54, and then we have one inside with 6.

• R:One inside with 6 cubes?

• C: Yes, so then we have 60 of these [pointing to a face on LFL].

We note a difference between D and C, according to the interior of the 3D array. D claimed it is one inside the array. C acknowledged that there is one inside the array with 6. What is not clear is what C refers to as “one” and “6”. We interpreted C's lack of reaction to the impossibility involved in R's question as a sign that he did not consider the interior of the array to include one cube with six faces and, that it is the number of faces he enumerates. D did not argue against C on this enumeration. Hence, we interpret the students as structuring the 3D array as space-filling but, instead of counting cubes they are counting faces of cubes.

With Task 2 the students grouped the visible spheres into different composites (colored in Fig. 9) and added them together:

• C: The thing about this one, is that it is a bit more strange, because it doesn’t have as many as the last one. (…) We have 6, plus 4, that is 10, plus 9 is 19.

Fig. 9

Visualization of C's counting strategy

To enumerate the whole array, C then added 10 to 19. R asked him to explain:

• C: Yes, here we have 6, these ones [putting a circle around the 6 spheres in RFL]. Here we have 4 [drawing a circle around the 4 spheres in LFL, not including those already counted in the RFL]. That is 10. And we have 9 up here [drawing a circle around the TL, Fig. 9], plus 10, that is 19. And we have 6 back there and 4 back there [pointing to RBL and LBL], which is 10, then we have 29. And then there are 4 in the middle, so then it is 33.

• R: How do you know how many are on the back?

• C: Because it is the same here [pointing to the RFL and LFL] on the back as well.

C did not double or triple count any of the visible spheres and was aware of hidden units, which implies that the spheres supported him in discerning and locating the 3D units. He grouped the units into composites and calculated the number of visible units. When trying to find out how many units that were non-visible, he copied the number of units on RFL and LFL: “And we have 6 back there and 4 back there”. This led to double counting of four spheres. C thought there were four units in the middle of the figure, expressing: “And then there are 4 in the middle”. It is not clear why he believed this, and we were not able to make sense of it through the interview.

Group 3 used strategy F on both tasks with cubical units, and strategy B4 on both tasks with spherical units. Using strategy F entails that the students counted outer 2D units and added a number for some interior units. Similar to Group 2, the students in Group 3 experienced trouble in the units-locating process when working on tasks with cubical units, as they could not discern the three-dimensional cube. When using strategy B4, the students counted 3D units unsystematically, and added an incorrect number for the hidden units. This shows that the students were able to locate the 3D unit when working on tasks with spherical units. In addition, the spherical units supported the process of organizing-by-composites, as the students were able to make composite units of spheres that, if iterated correctly, could lead to a correct enumeration of the array. Even though the spherical units seemed to provide perceptual clues to support this group in the units-locating process and organizing-by-composites process, they did not seem to provide support to parts of the spatial structuring process; structuring the interior units of the array. The group struggled with the process of spatial structuring in both the cubical and spherical tasks.

Discussion and conclusion

The present study continued the investigation of spherical units as perceptual clues, exploring how a spherical unit-representation compared to a cubical unit-representation can provide further affordance to support the development of essential cognitive processes for enumerating 3D arrays, in addition to units-locating. We addressed the research question: How can spherical unit-representations, compared to cubical unit-representations, provide perceptual clues that support students in developing strategies for enumerating units in 3D arrays?

Our results confirm results from a previous study (Alstad et al., 2021) in showing how spherical units can facilitate the units-locating process and turn students away from double and triple counting units. In addition, this study shed new light on how spherical units can act as perceptual clues and support the cognitive processes of organizing-by-composites and spatial structuring. Our detailed analysis points to three examples of how such processes are supported by spherical unit-representations. First, we found that some students in all three groups double and triple counted units in the arrays built of cubes. In general, students expressed less double and triple counting in tasks with spherical units compared to tasks with cubical units. This example indicates that the spherical units facilitated the cognitive process of units-locating for the students. Second, Group 1 and 2 used strategies on the spherical tasks implying that they perceived the spherical arrays as space-filling, while they struggled with this perception in the tasks with cubical unit-representations (e.g., counting only outer 2D units). However, the analysis of Group 3 shows that spherical units may provide insufficient clues to support students to structure and enumerate units located in the interior of a 3D array, i.e. to perceive the 3D arrays as space filling. Third, with the spherical units, the students in Group 1 and 2 not only perceived the arrays as space-filling, but also utilized strategies that involve iterating composite units which, if used correctly, would lead to a correct answer.

There are clear distinctions between the strategies of the three groups. Group 1 used an enumeration strategy of counting in columns on all four tasks, while Group 2 started all four tasks by counting units on one side of the array. Group 1 and 2 only succeeded in spatially structuring the array in terms of columns and layers, respectively, in tasks with spherical units. The second and third example implies that the spherical units supported the development of the cognitive processes of organizing-by-composites and spatial structuring for some of the students.

All three groups used a more sophisticated strategy on Task 2 (spheres) than Task 1 (cubes), even though both tasks were 3 × 3 × 3 arrays. It is tempting to think that Task 1 was perceived as more difficult because it was the first task. One might think that the students needed a “warm-up-task”, and that Task 2 was easier because they now “saw” the three-dimensionality of the arrays. But it seems that this was not the case, as the students went back to a less efficient strategy when they encountered Task 3, which was another task with cubical units (Table 3). When faced with Task 3, Group 2 and 3 went back to the same strategy as they used on the first task with cubical units. Group 1 used the same enumeration strategy on Task 3 and Task 2 but counted in a less efficient manner on the cubical units; counting from all three sides which made their enumeration more difficult since they had to coordinate units counted from all three sides.

Our previous study implied that there might be a link between unit-representations and the units-locating process (Alstad et al., 2021). The present study sheds new light on how the process of organizing-by-composites relates to the process of units-locating (Battista, 2004). Alstad et al. (2021) found that cubical units led to double and triple counting in a greater extent than spherical units. Our study implies that cubical units might be obstructive for some students in the processes of creating composite units and developing a correct spatial structuring of the 3D array. The cubical units might act as a perceptual obstacle for some students in discovering the underlying 3D structure of the arrays. As our study is a small-scale study, it is not possible to draw a definite and unambiguous conclusion based on our findings, but we note how essential it is to locate the unit in an array to be able to assemble composite 3D units. When the students had trouble locating the 3D unit, they consequently had a hard time constructing composites of 3D units, which they could iterate to find the total number of units in the array.

To make sense of a 2D representation of a 3D array, students’ need the abilities of spatial visualization and spatial structuring (Battista et al., 2018; Pittalis & Christou, 2010). Successful enumeration of units in such a 2D representation involves being able to locate the 3D unit, organize the units into composite units, and use repeated addition or multiplication to iterate the composite unit to generate the whole array. Vasilyeva et al. (2013) found that gridded representations acted as perceptual clues for students to perceive objects as three dimensional. Finesilver (2017) showed that colored layers on 3D arrays acted as perceptual clues in supporting students in spatially structuring the arrays in terms of horizontal layers and using more appropriate enumeration strategies. In contrast to these earlier studies our study shows how spherical units can act as perceptual clues for students in the development of essential cognitive processes for enumerating units in a 3D array. The findings of the present study show how the spherical units supported students in the units-locating process, the organizing-by-composites process, and the spatial structuring process. These findings became evident through the students’ enumeration processes, where students were avoiding double and triple counting, organizing units into relevant composites, and perceiving the arrays as three dimensional and space-filling when working on tasks with spherical units.

One of the differences between unit-spheres and unit-cubes is that, while unit-spheres are visually separate, the unit-cubes are visually conjoined. Battista (2010) hypotheses about using different colored cubes as perceptual clues can be connected to our use of spherical units as perceptual clues, as both offer the possibility of visually separating the units from each other. In the present study, students from all three groups had more trouble locating the unit in the arrays built of cubes. The drawing seemed to mask the 3D shape of the unit cubes. Conversely, when enumerating arrays with spheres, the students were more able to locate the units and assemble composite units, which again led to the development of a more correct spatial structuring of the array. We argue that these findings contribute to the understanding of the role of perceptual clues in enumerating processes. In particular, our study indicates that spherical units provide students with perceptual clues for cognitive processes, needed for the development of efficient strategies for enumerating 3D arrays. However, it may not be the spherical shape per se that provides perceptual clues, but the fact that the units are visually separated in the spherical case, compared to the cubical case. We therefore invite future research to further explore the role of separated and conjoined units in how students succeed in enumerating 3D arrays.

In addition, our study supports the need for further investigations on how students’ space-filling reasoning in tasks with 2D representation of 3D arrays can be supported. Since the present study shows how spherical units can provide perceptual clues to many students in the enumeration of 3D arrays, one proposal of such an investigation could be to design task sequences where students compare concrete 3D arrays with spherical units with 2D representations of 3D arrays with spherical units.