Skip to main content
SpringerLink
Log in

The Nonlinear Instability of a Cylindrical Interface Between Two Hydromagnetic Darcian Flows

  • Research Article - Physics
  • Published:
Arabian Journal for Science and Engineering Aims and scope Submit manuscript

Abstract

This paper investigates the nonlinear instability of an interface between two magnetic fluids separated by a cylindrical interface in porous media. The system is influenced by a uniform axial magnetic field. The magnetic field intensities allow a presence of surface currents at the interface. The transfer of mass and heat across the interface is considered. The solutions of linearized equations of motion, under the appropriate nonlinear boundary conditions, lead to a nonlinear characteristic equation that is governed the behavior of the interface deflection. Drawing on the linear stability theory, Routh–Hurwitz’s criteria are utilized to judge the stability criteria. The coupling of Laplace transforms and Homotopy perturbation techniques are adopted to obtain an approximate analytical solution of the interface profile. The nonlinear stability analysis resulted in two levels of solvability conditions. By means of these conditions, a Ginzburg–Landau equation is conducted. The latter equation represented the nonlinear stability configuration. The magnetic field intensity was plotted versus the wave number of the surface waves. Therefore, the stability picture was divided into stable and as well as unstable regions. Subsequently, the influence of the various physical parameters was addressed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Rosensweig, R.E.: Ferrohydrodynamics. Cambridge University Press, Cambridge (1985)

    Google Scholar 

  2. Lübbe, A.S.; Alexiou, C.; Bergemann, C.: Clinical applications of magnetic drug targeting. J. Surg. Res. 95, 200–206 (2001)

    Article  Google Scholar 

  3. Elhefnawy, A.R.E.; Moatimid, G.M.: Nonlinear dynamics and stability of two streaming magnetic fluids. Chaos Solitons Fractals 12, 1207–1216 (2001)

    Article  Google Scholar 

  4. Yecko, P.: Stability of layered channel flow of magnetic fluids. Phys. Fluids 21, 034102 (2009)

    Article  Google Scholar 

  5. Rheinländer, T.; Kötitz, R.; Weitschies, W.; Semmler, W.: Magnetic fluids: biomedical applications and magnetic fractionation. Magn. Electr. Separ. 10, 179–199 (2000)

    Article  Google Scholar 

  6. El-Dib, Y.O.; Mady, A.A.: Nonlinear stability of rotating two superposed magnetized fluids with the homotopy perturbation technique. JCAMEH 49(2), 261–273 (2018)

    Google Scholar 

  7. Lakehal, D.; Meier, M.; Fulgosi, M.: Interface tracking towards the direct simulation of heat and mass transfer in multiphase flows. Int. J. Heat Fluid Flow 23, 242–257 (2002)

    Article  Google Scholar 

  8. Hsieh, D.Y.: Effect of heat and mass transfer on Rayleigh–Taylor instability. J. Basic Eng. 94D, 156–160 (1972)

    Article  Google Scholar 

  9. Hsieh, D.Y.: Interfacial stability with mass and heat transfer. Phys. Fluids 21, 745–748 (1978)

    Article  Google Scholar 

  10. Tiwari, D.K.; Awasthi, M.K.; Agrawal, G.S.: Viscous potential flow analysis of magnetohydrodynamic capillary instability with heat and mass transfer. Ain Shams Eng. J. 6(3), 1113–1120 (2015)

    Article  Google Scholar 

  11. Awasthi, M.K.; Asthana, R.; Agrawal, G.S.: Viscous corrections for the viscous potential flow analysis of magnetohydrodynamic Kelvin–Helmholtz instability with heat and mass transfer. Eur. Phys. J. A 48, 174 (2012). https://doi.org/10.1140/epja/i2012-12174-6

    Article  Google Scholar 

  12. Awasthi, M.K.: Three-dimensional magnetohydrodynamic Kelvin–Helmholtz instability of cylindrical flow with permeable boundaries. Phys. Plasmas 21, 032124 (2014)

    Article  Google Scholar 

  13. Moatimid, G.M.: On the stability of two rigidly rotating magnetic fluid columns in zero gravity in the presence of mass and heat transfer. J. Colloid Interface Sci. 250, 108–120 (2002)

    Article  Google Scholar 

  14. Lee, D.S.: Nonlinear stability in magnetic fluids of cylindrical interface with mass and heat transfer. Eur. Phys. J. B 28, 495–503 (2002)

    Article  Google Scholar 

  15. El-Sayed, M.F.; Moatimid, G.M.; Metwaly, T.M.N.: Three dimensional nonlinear instability analysis of electroconvective finite dielectric fluids. Int. J. Pure Appl. Math. 118(4), 895–920 (2018)

    Google Scholar 

  16. Sondergeld, C.H.; Turcotte, D.L.: An experimental study of two-phase convection in a porous medium with applications to geological problems. J. Geophys. Res. 82, 2045–2053 (1977)

    Article  Google Scholar 

  17. Bau, H.H.: Kelvin–Helmholtz instability for parallel flow in porous media: a linear theory. Phys. Fluids 25(10), 1719–1722 (1982)

    Article  Google Scholar 

  18. Zakaria, K.; Sirwah, M.A.; Alkharashi, S.A.: Temporal stability of superposed magnetic fluids in porous media. Phys. Scr. 77, 1–20 (2008)

    Article  Google Scholar 

  19. Obied Allah, M.H.: Viscous potential flow analysis of electrified miscible finitely conducting fluid through porous media. Phys. Plasmas 20, 042104 (2013)

    Article  Google Scholar 

  20. Moatimid, G.M.; El-Dib, Y.O.; Zekry, M.H.: Stability analysis using multiple scales homotopy approach of coupled cylindrical interfaces under the influence of periodic electrostatic fields. Chin. J. Phys. 56, 2507–2522 (2018)

    Article  Google Scholar 

  21. Moatimid, G.M.; El-Dib, Y.O.; Zekry, M.H.: Instability analysis of a streaming electrified cylindrical sheet through porous media. Pramana J. Phys. 92, 22 (2019)

    Article  Google Scholar 

  22. He, J.H.: Homotopy perturbation technique. Comput. Methods Appl. Mech. Eng. 178, 257–262 (1999)

    Article  MathSciNet  Google Scholar 

  23. He, J.H.: A coupling method of a homotopy technique and a perturbation technique for non-linear problems. Int. J. Non-Linear Mech. 35(1), 37–43 (2000)

    Article  MathSciNet  Google Scholar 

  24. Sajid, M.; Hayat, T.; Asghar, S.: Comparison between the HAM and HPM solutions of thin film flows of non-Newtonian fluids on a moving belt. Nonlinear Dyn. 50, 27–35 (2007)

    Article  MathSciNet  Google Scholar 

  25. Biazar, J.; Ghazvini, H.: Convergence of the homotopy perturbation method for partial differential equations. Nonlinear Anal. Real World Appl. 10, 2633–2640 (2009)

    Article  MathSciNet  Google Scholar 

  26. Ayati, Z.; Biazar, J.: On the convergence of Homotopy perturbation method. J. Egypt. Math. Soc. 23, 424–428 (2015)

    Article  MathSciNet  Google Scholar 

  27. El-Dib, Y.O.; Moatimid, G.M.: On the coupling of the homotopy perturbation and Frobeninus method for exact solutions of singular nonlinear differential equations. Nonlinear Sci. Lett. A 9(3), 220–230 (2018)

    Google Scholar 

  28. Moatimid, G.M.: Non-linear electrorheological instability of two streaming cylindrical fluids. J. Phys. A Math. Gen. 36, 11343–11365 (2003)

    Article  MathSciNet  Google Scholar 

  29. Chandrasekhar, S.: Hydrodynamic and Hydrodynamic Stability. Oxford University Press, Oxford (1961)

    MATH  Google Scholar 

  30. El-Sayed, M.F.; Moatimid, G.M.; Metwaly, T.M.N.: Nonlinear electrohydrodynamic stability of two superposed streaming finite dielectric fluids in porous medium with interfacial surface charges. Transp. Porous Media 86, 559–578 (2011)

    Article  MathSciNet  Google Scholar 

  31. Melcher, J.R.: Field Coupled Surface Waves. MIT Press, Cambridge (1963)

    Google Scholar 

  32. Zahreddin, Z.; El-Shehawey, E.F.: On the stability of system of differential equations with complex coefficients. Indian J. Pure Appl. Math. 19(10), 963–972 (1988)

    MathSciNet  Google Scholar 

  33. El-Dib, Y.O.; Moatimid, G.M.: Stability configuration of a rocking rod over a circular surface using the homotopy perturbation method and Laplace transform. Arab. J. Sci. Eng. 44, 6581–6591 (2019)

    Article  Google Scholar 

  34. Tripathi, R.; Mishra, H.K.: Homotopy perturbation method with Laplace transform (LT-HPM) for solving Lane–Emden type differential equations (LETDEs). Springer Plus 2016, 1–21 (2016)

    Google Scholar 

  35. Moatimid, G.M.; El-Dib, Y.O.: Nonlinear Kelvin–Helmholtz instability of Oldroydian viscoelastic fluid in porous media. Phys. A 333, 41–64 (2004)

    Article  Google Scholar 

  36. Moatimid, G.M.: Nonlinear Kelvin–Helmholtz instability of two miscible ferrofluids in porous media. ZAMP 57, 133–159 (2006)

    MathSciNet  MATH  Google Scholar 

  37. Lange, C.G.; Newell, A.C.: A stability criterion for envelope equation. SIAM J. Appl. Math. 27(3), 441–456 (1974)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Education, Ain Shams University, Roxy, Cairo, Egypt

    Galal M. Moatimid & Yusry O. El-Dib

  2. Department of Mathematics and Computer Science, Faculty of Science, Beni-Suef University, Beni Suef, Egypt

    Marwa H. Zekry

Authors
  1. Galal M. Moatimid
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yusry O. El-Dib
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Marwa H. Zekry
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Marwa H. Zekry.

Appendices

Appendix A

The coefficients that appearing in Eq. (2.24) may be listed as follows:

$$\begin{aligned}\alpha_{1} &= \frac{G}{RL}\left( {\frac{1}{{\ln \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)}} - \frac{1}{{\ln \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)}}} \right),\,\,\,\,\\ \alpha_{2} &= \frac{G}{{R^{2} L}}\left( {\frac{1}{{\ln \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)}} - \frac{1}{{\ln \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)}}} \right)\\ &\quad\times\left( { - 3 + 2\frac{{\left( {\ln^{2} \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right) - \ln^{2} \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)} \right)}}{{\ln \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)\ln \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)\ln \left( {{a \mathord{\left/ {\vphantom {a b}} \right. \kern-0pt} b}} \right)}}} \right) \end{aligned}$$

and

$$\begin{aligned} \alpha_{3} & = \frac{G}{{R^{3} L}}\left( {\frac{1}{{\ln \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)}} - \frac{1}{{\ln \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)}}} \right)\\ &\quad\times \left( {11 + 12\frac{{\left( {\ln^{2} \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right) - \ln^{2} \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)} \right)}}{{\ln \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)\ln \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)\ln \left( {{a \mathord{\left/ {\vphantom {a b}} \right. \kern-0pt} b}} \right)}}} \right.\\ &\quad\left.{- 6\frac{{\left( {\ln^{3} \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right) - \ln^{3} \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)} \right)}}{{\ln^{3} \left( {{b \mathord{\left/ {\vphantom {b R}} \right. \kern-0pt} R}} \right)\ln^{3} \left( {{a \mathord{\left/ {\vphantom {a R}} \right. \kern-0pt} R}} \right)\ln^{3} \left( {{a \mathord{\left/ {\vphantom {a b}} \right. \kern-0pt} b}} \right)}}} \right). \end{aligned}$$

In formulating Eqs. (2.28)–(2.31) and (2.34), the these coefficients may be listed as:

$$\begin{aligned} L(A_{1} ,A_{2} ) & = I_{1} (kA_{1} )K_{1} (kA_{2} ) - I_{1} (kA_{2} )K_{1} (kA_{1} ),\quad M(A_{1} ,A_{2} ) = I_{1} (kA_{1} )K_{0} (kA_{2} ) - I_{0} (kA_{2} )K_{1} (kA_{1} ), \\ N(A_{1} ,A_{2} ) & = I_{0} (kA_{1} )K_{1} (kA_{2} ) + I_{1} (kA_{2} )K_{0} (kA_{1} ),\quad W(A_{1} ,A_{2} ) = I_{0} (kA_{1} )K_{0} (kA_{2} ) - I_{0} (kA_{2} )K_{0} (kA_{1} ), \\ Q(A_{1} ,A_{2} ) & = I_{0} (kA_{1} )K_{0} (kA_{2} ) + I_{0} (kA_{2} )K_{0} (kA_{1} ),\quad F_{xy}^{cd} = I_{0} (kx)I_{0} (ky)K_{0} (kc)K_{0} (kd), \\ P_{x}^{c} & = I_{0} (kx)K_{0} (kc),\quad X_{xy}^{cd} = I_{0} (kx)I_{0} (ky)K_{0} (kc)K_{1} (kd), \\ Y_{xy}^{cd} & = I_{0} (kx)I_{1} (ky)K_{0} (kc)K_{0} (kd),\quad P_{x}^{c} = I_{0} (kx)K_{0} (kc),\quad V_{x}^{c} = I_{1} (kx)K_{1} (kc), \\ E_{xy}^{cd} & = I_{1} (kx)I_{1} (ky)K_{1} (kc)K_{1} (kd),\quad G_{xy}^{cd} = I_{0} (kx)I_{1} (ky)K_{1} (kc)K_{1} (kd), \\ Z_{xy}^{cd} & = I_{1} (kx)I_{1} (ky)K_{0} (kc)K_{1} (kd),\quad U_{xy}^{cd} = I_{0} (kx)I_{0} (ky)K_{1} (kc)K_{1} (kd), \\ D_{xy}^{cd} & = I_{1} (kx)I_{1} (ky)K_{0} (kc)K_{0} (kd), \\ \end{aligned}$$

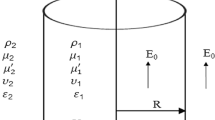
where \((A_{1} ,A_{2} ,x,y,c,d)\,\) are the same as \((\,a,\,b,\,R,\,r).\)

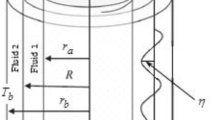
$$\begin{aligned} \varLambda & = 2ik\left( {\mu_{1} W(b,R)N(a,R) + \mu_{2} W(R,a)N(b,R)} \right) \\ & \quad - 2k(\mu_{1} - \mu_{2} )\left( {F_{RR}^{ab} + F_{ab}^{RR} - P_{R}^{R} Q(a,b)} \right.\left. {N(a,R)N(b,R)} \right)\,\eta_{z} \\ & \quad - ik\left( {(\mu_{1} - \mu_{2} )(X_{ab}^{RR} - Y_{RR}^{ab} ) + (\mu_{1} + 2\mu_{2} )(X_{aR}^{bR} - Y_{bR}^{aR} ) + (2\mu_{1} + \mu_{2} )(Y_{ab}^{RR} - X_{bR}^{aR} )} \right)\eta_{z}^{2} . \\ \end{aligned}$$

Appendix B

The coefficients that are appeared in Eq. (2.34) may be listed as follows:

$$\varpi^{2} = \frac{{\alpha_{1} \left( {v_{1} m_{1} \rho_{2} N(R,a)L(R,a) + v_{2} m_{2} \rho_{1} N(R,b)L(a,R)} \right)}}{{\rho_{1} \rho_{2} \left( {\rho_{1} N(R,a)L(R,a) - \rho_{2} N(R,b)L(a,R)} \right)}},$$
$$l_{1} = \frac{{\alpha_{1} L(b,a) - kR\left( {v_{1} m_{1} N(a,R)L(b,R) - v_{2} m_{2} N(b,R)L(a,R)} \right)}}{{ - kR\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$l_{2} = \frac{{V_{1} (\alpha_{1} + v_{1} m_{1} )m_{2} L(R,b)N(R,a) - V_{2} (\alpha_{1} + v_{2} m_{2} )m_{1} L(R,a)N(R,b)}}{{m_{1} m_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$l_{3} = \frac{{2V_{1} \rho_{1} m_{2} L(R,b)N(R,a) - 2V_{2} \rho_{2} m_{1} L(R,a)N(R,b)}}{{m_{1} m_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$l_{4} = T + \frac{{H^{2} }}{k}\left( {\left. {\frac{{h_{1} \mu_{1} (h_{1} \mu_{1} - h_{2} \mu_{2} )^{2} \left( {Q(R,a)W(R,b) - Q(R,b)W(R,a)} \right)}}{{\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)}}} \right) + \frac{{\rho_{1} V_{1}^{2} N(a,R)}}{{km_{1}^{2} L(R,a)}} + \frac{{\rho_{2} V_{2}^{2} N(R,b)}}{{km_{2}^{2} L(b,R)}},} \right.$$
$$s_{1} = \frac{{2m_{1} V_{2} L(R,a)\left( {k\alpha_{1} L(R,b) - \alpha_{2} N(R,b)} \right) - 2m_{2} V_{1} L(R,b)\left( {k\alpha_{1} L(R,a) - \alpha_{2} N(R,a)} \right)}}{{m_{1} m_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\beta_{1} = \frac{{\alpha_{1} \left( {v_{2} m_{2} \rho_{1}^{2} - v_{1} m_{1} \rho_{2}^{2} } \right)N(R,a)N(R,b)N(R,b)N(R,R)L(a,b)}}{{\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$s_{2} = \frac{{k\left( {V_{2} m_{1} \rho_{2} - V_{1} m_{2} \rho_{1} } \right)L(R,a)L(R,b)}}{{m_{1} m_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\beta_{2} = \frac{{\left( {v_{2} m_{2} \rho_{1} + \alpha_{1} (\rho_{1} - \rho_{2} ) - v_{1} m_{1} \rho_{2} } \right)N(R,a)N(R,b)N(R,b)N(R,R)L(a,b)}}{{\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)^{2} }},$$
$$\begin{aligned} s_{3} & = \frac{1}{2}\left( {H^{2} (\mu_{1} h_{1}^{2} - \mu_{2} h_{2}^{2} ) - } \right.\frac{{4\rho_{1} V_{1}^{2} e_{aR}^{aR} }}{{m_{1}^{2} L(a,R)L(R,a)}} + \frac{{4\rho_{2} V_{2}^{2} e_{bR}^{bR} }}{{m_{2}^{2} L(b,R)L(R,b)}} - \frac{{2\rho_{1} V_{1}^{2} \left( {e_{aa}^{RR} + e_{RR}^{aa} } \right)}}{{m_{1}^{2} L(R,a)^{2} }} + \frac{{2\rho_{2} V_{2}^{2} \left( {e_{bb}^{RR} + e_{RR}^{bb} } \right)}}{{m_{2}^{2} L(R,b)^{2} }} \\ & \quad - H^{2} (h_{1} \mu_{1} - h_{2} \mu_{2} )^{2} \left( {\left. {\frac{{\mu_{2} W(R,a)^{2} \left( {D_{RR}^{bb} + U_{bb}^{RR} } \right) + \mu_{2} W(R,a)^{2} V_{R}^{R} \left( {P_{a}^{a} + P_{b}^{b} } \right) - \mu_{1} W(R,b)^{2} \left( {D_{RR}^{aa} + U_{aa}^{RR} } \right)}}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }}} \right)} \right. \\ & \quad + 4H^{2} (h_{1} \mu_{1} - h_{2} \mu_{2} )\left( {\left. {\frac{{h_{1} \mu_{1} W(R,b)N(a,R) + h_{2} \mu_{2} W(R,b)N(a,R)}}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)}}} \right)} \right., \\ \end{aligned}$$
$$\beta_{3} = \frac{{\left( {V_{2} m_{1} \rho_{1} (\alpha_{1} + v_{2} m_{2} ) - V_{1} m_{2} \rho_{2} (\alpha_{1} + v_{1} m_{1} )} \right)N(R,a)N(R,b)N(R,b)N(R,R)L(a,b)}}{{\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)^{2} }},$$
$$s_{4} = \frac{{k\alpha_{1}^{2} (\rho_{1} - \rho_{2} )L(R,a) - \alpha_{2} v_{2} m_{2} \rho_{1} L(R,a)N(R,b) + \alpha_{2} v_{1} m_{1} \rho_{2} L(R,b)N(R,a)}}{{\rho_{1} \rho_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$s_{5} = \frac{{ - 2\alpha_{2} L(a,b)}}{{kR\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$s_{6} = \frac{{H^{2} (\mu_{1} h_{1}^{2} - \mu_{2} h_{2}^{2} )}}{{2k^{2} }}\left( {\frac{{\mu_{2} W(R,a)^{2} W(R,b)^{2} }}{{\left( {\mu_{2} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }} - \left. {\frac{{\mu_{1} W(R,a)^{2} W(R,b)^{2} }}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }}} \right)} \right.,$$
$$\beta_{4} = \frac{{\alpha_{1} \left( {V_{1} m_{2} N(R,a)^{2} L(R,b)^{2} - V_{2} m_{1} N(R,b)^{2} L(R,a)^{2} } \right)}}{{m_{1} m_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\beta_{5} = \frac{{\alpha_{1} L(a,b)\left( {Z_{Rb}^{Ra} + Z_{Rb}^{Rb} + 2G_{RR}^{ab} - G_{Rb}^{aR} + 2Z_{ab}^{RR} + G_{Ra}^{bR} } \right)}}{{kRL(R,a)L(R,b)\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\begin{aligned} \beta_{6} & = \frac{{2\left( {\rho_{1} m_{2}^{2} V_{1}^{2} L(R,b)^{2} N(R,a)^{2} - \rho_{2} m_{1}^{2} V_{2}^{2} N(R,b)^{2} L(R,a)^{2} } \right)}}{{km_{1}^{2} m_{2}^{2} L(R,a)^{2} L(R,b)^{2} }} \\ & \quad + \frac{{h_{2} \mu_{2} H^{2} W(b,R)}}{{k\left( {\mu_{2} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)}} \\ & \quad \left( {(h_{2} (\mu_{1} + 2\mu_{2} ) - 3h_{1} \mu_{1} )N(a,R)} \right. \\ & \quad + \left. {\frac{{2(\mu_{1} - \mu_{2} )(h_{1} \mu_{1} - h_{2} \mu_{2} )W(R,a)\left( { - P_{R}^{R} Q(b,a) + V_{R}^{R} Q(b,a) + D_{RR}^{ab} + F_{ab}^{RR} + U_{ab}^{RR} + X_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right) \\ & \quad - \frac{{h_{1} \mu_{2} H^{2} W(a,R)}}{{k\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)}} \\ & \quad \left( {(h_{1} (2\mu_{1} + \mu_{2} ) - 3h_{2} \mu_{2} )N(b,R)} \right. \\ & \quad - \left. {\frac{{2(\mu_{1} - \mu_{2} )(h_{1} \mu_{1} - h_{2} \mu_{2} )W(R,b)\left( { - P_{R}^{R} Q(a,b) + V_{R}^{R} Q(a,b) + D_{RR}^{ab} + F_{ab}^{RR} + F_{RR}^{ab} + U_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right), \\ \end{aligned}$$
$$\beta_{7} = \frac{{3\left( {V_{1} \rho_{1} m_{2} N(R,a)^{2} L(R,b)^{2} - V_{2} \rho_{2} m_{1} N(R,b)^{2} L(R,a)^{2} } \right)}}{{km_{1} m_{2} L(R,a)^{2} L(R,b)^{2} }},$$
$$\beta_{8} = \frac{{V_{1} \rho_{1} m_{2} N(R,a)^{2} L(R,b)^{2} - V_{2} \rho_{2} m_{1} N(R,b)^{2} L(R,a)^{2} }}{{m_{1} m_{2} L(R,a)L(R,b)\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\beta_{9} = \frac{{\rho_{1} N(R,a)^{2} L(R,b)^{2} - \rho_{2} N(R,b)^{2} L(R,a)^{2} }}{{L(R,a)L(R,b)\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$n_{1} = \frac{{\alpha_{1} }}{k}\left( {\frac{{2\alpha_{1} N(R,a)}}{{\rho_{1} L(R,a)}} - \frac{{2\alpha_{1} N(R,b)}}{{\rho_{2} L(R,b)}}} \right. - \frac{{v_{1} m_{1} N(R,a)\left( {N(R,a)^{2} - L(R,a)^{2} } \right)}}{{\rho_{1} L(R,a)^{3} }} + \left. {\frac{{v_{2} m_{2} N(R,b)\left( {N(R,b)^{2} - L(R,b)^{2} } \right)}}{{\rho_{2} L(R,b)^{3} }}} \right),$$
$$\gamma_{1} = \frac{{2\alpha_{1} }}{k}\left( {\frac{{V_{1} N(R,a)\left( {\alpha_{2} N(R,a) + 2kL(a,R)} \right)}}{{m_{1} L(R,a)^{2} }} + \frac{{V_{2} N(R,b)\left( {\alpha_{2} N(R,b)^{2} + 2kL(b,R)^{2} } \right)}}{{m_{2} L(R,b)^{2} }}} \right),$$
$$\begin{aligned} n_{2} & = L(a,b)\left( {(\rho_{1} - \rho_{2} )(m_{1} v_{1} \rho_{2} - m_{2} v_{2} \rho_{1} )} \right.N(R,a)^{2} N(R,b)^{2} - \alpha_{1} \left( {\rho_{1}^{2} N(R,a)\left( {U_{RR}^{bb} - E_{RR}^{bb} + 2V_{b}^{b} (P_{R}^{R} + V_{R}^{R} )} \right.} \right. \\ & \left. {\quad + D_{bb}^{RR} - E_{bb}^{RR} } \right) - 2\rho_{1} \rho_{2} N(R,a)N(R,b)\left( {V_{b}^{a} P_{R}^{R} + U_{RR}^{ab} - E_{RR}^{ab} + E_{Ra}^{Rb} + V_{a}^{b} P_{R}^{R} + E_{Ra}^{Rb} + D_{ab}^{RR} - E_{ab}^{RR} } \right) + \rho_{2}^{2} N(R,b)^{2} \\ & \quad {{\left. {\left. {\left( {U_{RR}^{aa} - E_{RR}^{aa} + 2V_{a}^{a} P_{R}^{R} + 2E_{Ra}^{Ra} + D_{aa}^{RR} - E_{aa}^{RR} } \right)} \right)} \right)} \mathord{\left/ {\vphantom {{\left. {\left. {\left( {U_{RR}^{aa} - E_{RR}^{aa} + 2V_{a}^{a} P_{R}^{R} + 2E_{Ra}^{Ra} + D_{aa}^{RR} - E_{aa}^{RR} } \right)} \right)} \right)} {\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)^{3} ,}}} \right. \kern-0pt} {\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)^{3} ,}} \\ \end{aligned}$$
$$\begin{aligned} \gamma_{2} & = - km_{1} V_{2} \rho_{2} (\rho_{1} N(R,a)L(R,b)\left. {\left( {3G_{Ra}^{Rb} + 2Z_{ab}^{RR} + Z_{Ra}^{Rb} - 3Z_{Ra}^{Rb} - 2G_{RR}^{ab} - G_{Rb}^{Ra} } \right) + 2\rho_{2} N(R,b)^{2} L(R,a)^{2} } \right) \\ & \quad - {{km_{2} V_{1} \rho_{1} (\rho_{2} N(R,b)L(R,a)\left. {\left( {3G_{Rb}^{Ra} + 2Z_{ab}^{RR} + Z_{Rb}^{Ra} - 3Z_{Ra}^{Rb} - 2G_{RR}^{ab} - G_{Ra}^{Rb} } \right) + 2\rho_{1} N(R,a)^{2} L(R,b)^{2} } \right)} \mathord{\left/ {\vphantom {{km_{2} V_{1} \rho_{1} (\rho_{2} N(R,b)L(R,a)\left. {\left( {3G_{Rb}^{Ra} + 2Z_{ab}^{RR} + Z_{Rb}^{Ra} - 3Z_{Ra}^{Rb} - 2G_{RR}^{ab} - G_{Ra}^{Rb} } \right) + 2\rho_{1} N(R,a)^{2} L(R,b)^{2} } \right)} {m_{1} m_{2} }}} \right. \kern-0pt} {m_{1} m_{2} }} \\ & \quad \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)^{2} , \\ \end{aligned}$$
$$\begin{aligned} n_{3} & = \frac{{V_{2} N(R,b)}}{{km_{2} L(R,b)^{3} }}\left( {m_{2} v_{2} \left( {2V_{b}^{b} \left( {P_{R}^{R} + V_{R}^{R} } \right) + D_{bb}^{RR} + U_{RR}^{bb} - E_{RR}^{bb} - E_{bb}^{RR} } \right)} \right. \\ & \quad + \left. {\alpha_{1} \left( {2V_{b}^{b} \left( {P_{R}^{R} + 3V_{R}^{R} } \right) + D_{RR}^{bb} + U_{RR}^{bb} - 3E_{RR}^{bb} - 3E_{bb}^{RR} } \right)} \right) \\ & \quad - \frac{{V_{1} N(R,a)}}{{km_{1} L(R,a)^{3} }}\left( {m_{1} v_{1} \left( {2V_{a}^{a} \left( {P_{R}^{R} + V_{R}^{R} } \right) + D_{aa}^{RR} + U_{RR}^{aa} - E_{RR}^{aa} - E_{aa}^{RR} } \right)} \right. \\ & \quad + \left. {\alpha_{1} \left( {2V_{a}^{a} \left( {P_{R}^{R} + 3V_{R}^{R} } \right) + D_{aa}^{RR} + U_{RR}^{aa} - 3E_{RR}^{aa} - 3E_{aa}^{RR} } \right)} \right), \\ \end{aligned}$$
$$\begin{aligned} \gamma_{3} & = \frac{1}{2}\left( {\frac{{4\rho_{1} V_{1}^{2} N(R,a)\left( {2E_{aR}^{aR} - E_{RR}^{aa} - E_{aa}^{RR} } \right)}}{{m_{1}^{2} L(R,a)^{3} }}} \right. - \frac{{4\rho_{2} V_{2}^{2} N(R,b)\left( {2E_{bR}^{bR} - E_{RR}^{bb} - E_{bb}^{RR} } \right)}}{{m_{2}^{2} L(R,b)^{3} }} \\ & \quad - \frac{{(h_{1} \mu_{1} - h_{2} \mu_{2} )\mu_{2} H^{2} \left( {D_{RR}^{bb} + U_{bb}^{RR} + V_{R}^{R} P_{b}^{b} } \right)W(a,R)}}{{\left( {\mu_{2} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }} \\ & \quad \left( {\frac{{2(h_{1} \mu_{1} - h_{2} \mu_{2} )(\mu_{1} - \mu_{2} )W(R,a)\left( { - P_{R}^{R} Q(b,a) + V_{R}^{R} Q(b,a) + D_{RR}^{ab} + F_{RR}^{ab} + F_{ab}^{RR} + U_{ab}^{RR} + X_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right. \\ & \quad + \left. {(h_{2} (\mu_{1} + 2\mu_{2} ) - 3h_{1} \mu_{1} )N(a,R)} \right) - \frac{{(h_{1} \mu_{1} - h_{2} \mu_{2} )\mu_{1} H^{2} \left( {D_{RR}^{aa} + U_{aa}^{RR} + V_{R}^{R} P_{a}^{a} } \right)W(R,b)}}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }}\left( {(h_{1} (2\mu_{1} + \mu_{2} ) - 3h_{2} \mu_{2} )N(b,R)} \right. \\ & \quad - \left. {\frac{{2(h_{1} \mu_{1} - h_{2} \mu_{2} )(\mu_{1} - \mu_{2} )W(R,b)\left( { - P_{R}^{R} Q(a,b) + V_{R}^{R} Q(a,b) + D_{RR}^{ab} + F_{RR}^{ab} + F_{ab}^{RR} + U_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right), \\ \end{aligned}$$
$$n_{4} = \frac{{V_{2} m_{1} L(R,a)\left( {2k\alpha_{2} L(R,b) - 3\alpha_{3} N(R,b)} \right) - V_{1} m_{2} L(R,b)\left( {2k\alpha_{2} L(R,a) - 3\alpha_{3} N(R,a)} \right)}}{{m_{1} m_{2} L(R,a)L(R,b)\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\gamma_{4} = \frac{{\alpha_{1} }}{k}\left( {\frac{{N(R,a)\left( {\alpha_{2} m_{1} v_{1} N(R,a) + 2k\alpha_{1} L(a,R)} \right)}}{{\rho_{1} L(R,a)^{2} }} - \frac{{N(R,b)\left( {\alpha_{2} m_{2} v_{2} N(R,b) - 2k\alpha_{1} L(R,b)} \right)}}{{\rho_{2} L(R,b)^{2} }}} \right),$$
$$n_{5} = \frac{4}{k}\left( {\frac{{V_{2} \rho_{2} N(R,b)\left( {N(R,b) + L(R,b)} \right)\left( {N(R,b) + L(b,R)} \right)}}{{m_{2} L(R,b)^{3} }} - \frac{{V_{1} \rho_{1} N(R,a)\left( {N(R,a) + L(R,a)} \right)\left( {N(R,a) + L(a,R)} \right)}}{{m_{1} L(R,a)^{3} }}} \right),$$
$$\begin{aligned} n_{6} & = \frac{{(h_{1} \mu_{1} - h_{2} \mu_{2} )H^{2} W(R,b)}}{k}\left( { - \frac{{\mu_{2} W(R,a)\left( {(h_{2} \mu_{2} - 2h_{1} \mu_{1} )W(R,a)N(b,R) - 2h_{2} \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)}}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }}} \right. \\ & \quad + \left. {\frac{{\mu_{1} W(a,R)\left( {h_{1} \mu_{2} P_{R}^{a} W(R,a)N(b,R) + N(a,R)\left( {h_{1} \mu_{1} \left( {P_{a}^{R} W(b,R) + 2W((R,b))} \right) - 2h_{2} \mu_{2} W(R,b)} \right)} \right)}}{{\left( {\mu_{2} P_{R}^{a} W(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)} \right)^{2} }}} \right) \\ & \quad - \frac{T}{2} - \frac{{3\rho_{1} V_{1}^{2} N(R,a)\left( {N(R,a) + L(R,a)} \right)\left( {N(R,a) + L(a,R)} \right)}}{{km_{1}^{2} L(R,a)^{3} }} \\ & \quad + \frac{{3\rho_{2} V_{2}^{2} N(R,b)\left( {N(R,b) + L(R,b)} \right)\left( {N(R,b) + L(b,R)} \right)}}{{km_{2}^{2} L(R,b)^{3} }}, \\ \end{aligned}$$
$$n_{7} = \frac{{2\alpha_{1} L(a,b)}}{{k^{2} RL(R,a)L(R,b)}} + \frac{{2\alpha_{1} N(R,a)^{3} }}{{kL(a,R)^{3} }} + \frac{{2\alpha_{1} N(R,b)^{3} }}{{kL(R,b)^{3} }},$$
$$n_{8} = \frac{{\rho_{2} N(R,b)\left( {L(R,b)^{2} - 2N(R,b)^{2} } \right)}}{{kL(R,b)^{3} }} - \frac{{\rho_{1} N(R,a)\left( {L(R,a)^{2} - 2N(R,a)^{2} } \right)}}{{kL(R,a)^{3} }},$$
$$n_{9} = \frac{{\rho_{2} V_{2} N(R,b)\left( {2N(R,b)^{2} - L(R,b)^{2} } \right)}}{{km_{2} L(R,b)^{3} }} - \frac{{\rho_{1} V_{1} N(R,a)\left( {2N(R,a)^{2} - L(R,a)^{2} } \right)}}{{km_{1} L(R,a)^{3} }},$$
$$n_{10} = \frac{{2\alpha_{1} }}{k}\left( {\frac{{V_{2} N(R,b)\left( {N(R,b)^{2} - L(R,b)^{2} } \right)}}{{m_{2} L(R,b)^{3} }} - \frac{{V_{1} N(R,a)\left( {N(R,b)^{2} - L(R,b)^{2} } \right)}}{{m_{1} L(R,a)^{3} }}} \right),$$
$$n_{11} = \frac{{3\alpha_{3} L(a,b)N(R,R)}}{{\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)}},$$
$$n_{12} = \frac{{2k\alpha_{1} \alpha_{2} (\rho_{1} - \rho_{2} )L(R,a)L(R,b) + m_{1} v_{1} \rho_{2} \alpha_{3} N(R,a)L(R,b) - m_{2} v_{2} \rho_{1} \alpha_{3} N(R,b)L(R,a)}}{{\rho_{1} \rho_{2} \left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\gamma_{5} = \alpha_{1} L(a,b)N(R,R)(\rho_{1} N(R,a)(3L(b,R) + 2\alpha_{2} N(R,b)) - \rho_{2} N(R,b)(3L(a,R) + 2\alpha_{2} N(R,a))),$$
$$\gamma_{6} = \frac{{\rho_{1} N(R,a)}}{L(a,R)} + \frac{{\rho_{2} N(R,b)}}{L(R,b)},$$
$$\begin{aligned} \gamma_{7} & = \frac{{(h_{1} \mu_{1} - h_{2} \mu_{2} )\mu_{2} H^{2} W(R,a)W(R,b)^{2} }}{{\left( {\mu_{1} P_{b}^{R} W(b,R)N(a,R) + \mu_{2} P_{R}^{a} W(R,a)N(b,R)} \right)^{2} }}\left( {(h_{2} (\mu_{1} + 2\mu_{2} ) - 3h_{1} \mu_{1} )N(a,R)} \right. \\ & \quad + \left. {\frac{{2(h_{1} \mu_{1} - h_{2} \mu_{2} )(\mu_{1} - \mu_{2} )W(R,a)\left( {\left( {V_{R}^{R} - P_{R}^{R} } \right)Q(b,a) + D_{RR}^{ab} + F_{ab}^{RR} + F_{RR}^{ab} + U_{ab}^{RR} + X_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right) \\ & \quad + \frac{{(h_{1} \mu_{1} - h_{2} \mu_{2} )\mu_{1} H^{2} W(R,b)W(R,a)^{2} }}{{\left( {\mu_{1} P_{b}^{R} W(b,R)N(a,R) + \mu_{2} P_{R}^{a} W(R,a)N(b,R)} \right)^{2} }}\left( {(h_{1} (2\mu_{1} + \mu_{2} ) - 3h_{2} \mu_{2} )N(b,R)} \right. \\ & \quad - \left. {\frac{{2(h_{1} \mu_{1} - h_{2} \mu_{2} )(\mu_{1} - \mu_{2} )W(R,\gamma )\left( {\left( {V_{R}^{R} - P_{R}^{R} } \right)Q(a,b) + D_{RR}^{ab} + F_{ab}^{RR} + F_{RR}^{ab} + U_{ab}^{RR} + X_{ab}^{RR} } \right)}}{{\mu_{1} W(R,b)N(a,R) - \mu_{2} W(R,a)N(b,R)}}} \right), \\ \end{aligned}$$
$$\gamma_{8} = \frac{{\alpha_{1} \alpha_{2} L(a,b)\left( {Z_{Rb}^{Ra} + Z_{Ra}^{Rb} + 2G_{RR}^{ab} - G_{Rb}^{Ra} + 2Z_{ab}^{RR} + 2G_{Ra}^{Rb} } \right)}}{{kRL(R,a)L(R,b)\left( {\rho_{1} N(R,a)L(R,b) - \rho_{2} N(R,b)L(R,a)} \right)}},$$
$$\gamma_{9} = \frac{{\alpha_{1} \alpha_{2} }}{k}\left( {\frac{{V_{1} N(R,a)^{2} }}{{m_{1} L(R,a)^{2} }} - \frac{{V_{2} N(R,b)^{2} }}{{m_{2} L(R,b)^{2} }}} \right),$$

Appendix C

The coefficients that are appeared in Eqs. (3.8), (4.3) and (4.16) may be listed as follows:

$$\varGamma = - \frac{{k^{3} l_{3}^{2} }}{k}\left( {\frac{{h_{1} \mu_{1} (h_{1} \mu_{1} - h_{2} \mu_{2} )Q(R,a)W(R,b)}}{{\mu_{2} P_{{_{R} }}^{a} Q(R,a)N(b,R) + \mu_{1} P_{b}^{R} W(b,R)N(a,R)}} + \left. {\frac{{h_{2} \mu_{2} (h_{2} \mu_{2} - h_{1} \mu_{1} )W(R,a)Q(R,b)}}{{\mu_{1} P_{b}^{R} W(b,R)N(a,R) + \mu_{2} P_{a}^{R} W(R,a)N(b,R)}}} \right)} \right.,$$
$${\rm K} = kl_{3}^{2} \varpi^{2} - kl_{2}^{2} - k^{3} l_{3}^{2} \left( {T + \frac{{\rho_{1} V_{1}^{2} N(a,R)}}{{km_{1}^{2} L(R,a)}} + \frac{{\rho_{2} V_{2}^{2} N(R,b)}}{{km_{2}^{2} L(b,R)}}} \right),$$
$$\begin{aligned} a_{1} & = s_{1} - \frac{{\beta_{1} n_{3} }}{{\gamma_{3} }},\,\;\;a_{2} = s_{2} - \frac{{\beta_{2} n_{3} }}{{\gamma_{3} }},\,\;\;a_{3} = - \frac{{\beta_{4} n_{3} }}{{\gamma_{3} }},\;\;\,a_{4} = s_{1} - \frac{{\beta_{5} n_{3} }}{{\gamma_{3} }}, \\ a_{5} & = - \frac{{\beta_{6} n_{3} }}{{\gamma_{3} }},\;\;\,a_{6} = - \frac{{\beta_{7} n_{3} }}{{\gamma_{3} }},\;\;\,a_{7} = - \frac{{\beta_{8} n_{3} }}{{\gamma_{3} }},\, \\ \end{aligned}$$
$$\begin{aligned} a_{8} & = s_{1} - \frac{{\beta_{9} n_{3} }}{{\gamma_{3} }},\;\;\,a_{9} = s_{3} - \frac{{\beta_{3} n_{3} }}{{\gamma_{3} }},\;\;\,a_{10} = n_{4} - \frac{{n_{3} \gamma_{4} }}{{\gamma_{3} }},\;\;\,a_{11} = - \frac{{n_{3} \gamma_{5} }}{{\gamma_{3} }}, \\ a_{12} & = - \frac{{n_{3} \gamma_{6} }}{{\gamma_{3} }},\;\;\,a_{13} = - \frac{{n_{3} \gamma_{8} }}{{\gamma_{3} }},\;\;\,a_{14} = - \frac{{n_{3} \gamma_{9} }}{{\gamma_{3} }}, \\ \end{aligned}$$
$$a_{15} = - \frac{{n_{3} \gamma_{7} }}{{\gamma_{3} }},\quad a_{16} = n_{1} - \frac{{n_{3} \gamma_{1} }}{{\gamma_{3} }},\quad a_{17} = n_{2} - \frac{{n_{3} \gamma_{2} }}{{\gamma_{3} }},\quad \sigma^{2} = - \frac{{3l_{1} n_{12} }}{{l_{4} n_{11} }},\quad b_{1} = \frac{{l_{2} n_{11} - 3l_{3} n_{12} }}{{l_{4} n_{11} }},$$
$$\begin{aligned} b_{2} & = \frac{{\varpi^{2} n_{11} a_{12} + 3n_{11} a_{10} - 3n_{12} a_{11} - 3n_{12} a_{13} }}{{4l_{4} n_{11} }},\,\;\;b_{3} = \frac{{\varpi^{2} n_{8} n_{11} - 3n_{7} n_{11} + 3n_{11} a_{16} - 3n_{12} a_{17} }}{{4l_{4} n_{11} }},\,\;\;b_{4} = \frac{{3n_{5} n_{12} }}{{4l_{4} n_{11} }}, \\ b_{5} & = \frac{{3a_{14} }}{{4l_{4} }},\;\;\,b_{6} = \frac{{3n_{10} n_{11} - 3n_{9} n_{12} }}{{4l_{4} n_{11} }},\,\;\;b_{7} = \frac{{3n_{10} n_{11} - 3n_{9} n_{12} }}{{4l_{4} n_{11} }}\;\;{\text{and}}\;\;b_{8} = \frac{{3a_{15} }}{{4l_{4} }}. \\ \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Moatimid, G.M., El-Dib, Y.O. & Zekry, M.H. The Nonlinear Instability of a Cylindrical Interface Between Two Hydromagnetic Darcian Flows. Arab J Sci Eng 45, 391–409 (2020). https://doi.org/10.1007/s13369-019-04192-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13369-019-04192-z

Keywords

Search

Navigation