Introduction

Despite the social controversy surrounding them, shale reservoirs have been exploited in several countries around the world. This has contributed to hydrocarbon self-sufficiency in the USA. Therefore, it is important to account practical characterization techniques to understand the properties of shale formations for an adequate reservoir management. Due their ultralow permeability, shaly formations must be subjected to hydraulic fracturing, which, in some cases, may lead to the communication between neighboring wells. Such connectivity can be evaluated using pressure interference test interpretation techniques. Interference testing is a helpful tool for reservoir characterization, and it is also useful to find properties such as horizontal anisotropy, reservoir connectivity, and fracture orientation.

It is important to observe that for exploiting shale reservoirs, a stimulated reservoir volume (SRV) is created once massive hydraulic fracturing has been performed. Normally, the permeability outside the SRV boundaries is so low that fluid flow is not expected in such regions. However, as time goes by, it is possible to have an interference between wells in shale plays, as noticed by authors such as Pang et al. (2015). Nonetheless, the best and interpretable interference is obtained between the connected fractures.

There has been research on the interference between wells connected by a hydraulic fracture. Wu et al. (2017) performed a numerical study to find that lesser fracturing spacing helps avoiding fracture connection. Chen et al. (2017) determined the spacing between horizontal hydraulically fractured wells through the interference tests, and they used integrated analysis to determine the spacing and production rates from the alternating shut-in periods of an interference test. Wang et al. (2018) proposed a semi-analytical Laplacian model to simulate pressure interference response in multi-well systems. Their model overcame the difficulties in handling discontinuities when the flow rate changed abruptly. Although, they studied pressure response, no interpretation technique was provided. Thompson (2018) suggested a semi-analytical superposition model to analyze the pressure and rate data behavior from wells with important characteristics, especially skin factor and flow capacity that are affected because of an interference fracture. However, he did not consider the gas compressibility effects in his equation. He et al. (2018) developed a new model for interference testing using the analytical solutions of a multi-segment horizontal well to obtain the pressure and pressure derivate behavior. Because of the interference tests, the model was able to evaluate the communication between wells. Wang and Yu (2019) conducted a numerical research on gas injection using compositional reservoir simulation to include connected natural fractures between wells. Their work considered the well interference effect and optimal well spacing. However, they did not elaborate on the behavior of pressure interference. He et al. (2019) developed a mathematical model to analyze multi-well interference testing to obtain the effect of nearly multiple horizontal wells with injectors wells has from the pressure data. They determined that the total injection and well spacing are the main properties to be considered for middle and late pressure behaviors. Kumar et al. (2020) adopted an approach based on horizontal well pressure data and tracer analysis to identify the relationship between these two procedures. They suggested that understanding those factors can contribute to optimizing well drilling, well spacing, etc. Haghshenas et al. (2021) devised a practical analytical tool considering the hydraulic fractures and matrix systems in interference tests with a Laplace transform combined with pseudo-time and pseudo-pressure functions. Seth et al. (2021) created a model to study the interference pressure behavior between horizontal wells. Using this model, they could determine fracture geometry and other characteristics. Luo et al. (2021) developed a numerical model that predicts the productivity of shale gas horizontal wells, in which, fractures present an interference between them and could register and analyze the azimuth and fracture conductivity.

Chu et al. (2020) conducted a study on the pressure interference between wells connected by a fracture using an analytical model proposed by Chen and Raghavan (2015). Chu et al. (2020) found that the Chow pressure group (CPG) is a quantitative indicator of connectivity when anomalous diffusion is observed. They, however, did not provide equations to estimate fracture conductivity, fracture permeability, or any other parameter. Han et al. (2019) presented two analytical solutions to study the pressure behavior between two wells connected by a large fracture. One model considered the crossflow between the matrix and fracture, whereas the other one did not, but they did not provide a direct analytical interpretation technique. Therefore, the current study is based on the two models proposed by Han et al. (2019) to provide two interpretation techniques: (1) conventional analysis and (2) Tiab’s direct synthesis (TDS) technique, Tiab (1993), which were tested with simulated examples. On the one hand, as it is well-known, the straight-line conventional analysis method uses a plot of pressure against a given function of time from which the slope reservoir parameters can be estimated. On the other hand, the TDS technique uses characteristic features found concerning the pressure and pressure derivative versus time log–log plot to develop analytical and direct expressions to find the reservoir parameters.

Some applications of the TDS technique in interference testing were presented by Escobar et al. (2008, 2018, 2020) for radial systems, linear and spherical systems, and naturally fractured reservoirs in radial systems, respectively. For further information on the TDS technique, refer to the works by Escobar (2015, 2018a, b, c) and Escobar et al. (2018a),

Mathematical formulation

Han et al. (2019) introduced two models to be used with shale formations to account for the interference of two multistage hydraulically fractured wells that communicate by a large fracture. One model includes the crossflow from the reservoir matrix to the fracture, while the other one, referred to as the simple model here, does not. The simple model with dimensionless pressure is given by

$$ P_{D} = \frac{{2\sqrt {t_{Dwf} } }}{\sqrt \pi }e^{{ - \frac{{x_{D}^{2} }}{{4t_{Dwf} }}}} + x_{D} erf\left( {\frac{{x_{D} }}{{2\sqrt {t_{Dwf} } }}} \right) - x_{D} $$
(1)

The pressure derivative estimated in this work was determined by

$$ t_{D} *P_{D} ^{\prime} = t_{Dwf} e^{{ - \frac{{x_{D}^{2} }}{{4t_{Dwf} }}}} \left[ {\frac{1}{\sqrt \pi } - \left( {\frac{{x_{D}^{2} }}{{2\sqrt \pi \sqrt[3]{{t_{Dwf}^{2} }}}}} \right)} \right] $$
(2)

The dimensionless time based on fracture width is given below:

$$ t_{Dwf} = \frac{{0.000263k_{f} t}}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }} $$
(3)

When dealing with gas wells, both compressibility and viscosity are evaluated at initial conditions.

The quantities of dimensionless pressure and pressure derivative for oil reservoirs are evaluated using:

$$ P_{D} = \frac{{k_{f} h\Delta P}}{141.2q\mu B} $$
(4)
$$ t_{D} *P_{D} ^{\prime} = \frac{{k_{f} h(t*\Delta P^{\prime})}}{141.2q\mu B} $$
(5)

For gas flow, the dimensionless pseudopressure and dimensionless pseudopressure derivative are defined by

$$ m(P)_{D} = \frac{{k_{f} h[m(P_{i} ) - m(P)]}}{{1424.52q_{sc} T}} $$
(6)
$$ t_{D} *m(P)_{D} ^{\prime} = \frac{{k_{f} h[t*\Delta m(P)^{\prime}]}}{{1424.52q_{sc} T}} $$
(7)

Han et al. (2019) also defined dimensionless fracture length as:

$$ x_{D} = \frac{{x_{f} }}{{w_{f} }} $$
(8)

Since the fracture connects the two wells, the fracture length can be taken as the distance between the wells.

The other model, with a crossflow from the matrix to large fracture, was also proposed by Han et al. (2019) in the Laplacian domain to be

$$ \overline{P}_{D} (s,x_{D} ,0) = \frac{1}{{s\sqrt {s + 2F_{D} \sqrt {sC_{f} } } }}e^{{ - \sqrt {s + 2F_{D} \sqrt {sC_{f} } } x_{D} }} $$
(9)

Laplacian pressure derivative is estimated by the Laplace properties and is determined by:

$$ \overline{P}_{D} ^{\prime}(s,x_{D} ,0) = \frac{1}{{\sqrt {s + 2F_{D} \sqrt {sC_{f} } } }}e^{{ - \sqrt {s + 2F_{D} \sqrt {sC_{f} } } x_{D} }} $$
(10)

Han et al. (2019) also defined the diffusivity ratio, Cf, and permeability ratio, FD, as follows:

$$ C_{f} = \frac{{\eta_{f} }}{{\eta_{m} }} $$
(11)
$$ F_{D} = \frac{{k_{m} }}{{k_{f} }} $$
(12)

Interpretation methodology

The input parameters for both Eqs. (1) and (9) are presented in Table 1 for all the simulations. For both equations, a computer program was written, and then the dimensionless pressure and pressure derivative versus dimensionless curves were obtained. Starting with the simple model, it does not consider the flow from the matrix to the hydraulic fracture, so only a linear flow is expected to occur along the connecting fracture. The pressure and pressure derivative response for different fracture lengths are shown in Fig. 1. As depicted in the figure, different pressure and pressure derivative trends are obtained as a function of the fracture length. There is a need for obtaining a unified pressure behavior for the purpose of establishing an interpretation technique. A process to this end has been outlined by Escobar, Jongkittnarukorn, and Hernandez (2018). The unified behavior, shown in Fig. 2, helps develop to obtain the following governing equations during the linear flow regime:

$$ \frac{{P_{D} }}{{x_{D} }} = 1.1054\left( {\frac{{t_{Dwf} }}{{x_{D}^{2} }}} \right)^{0.5} $$
(13)
$$ \frac{{t_{D} *P_{D} ^{\prime}}}{{x_{D} }} = 0.5527\left( {\frac{{t_{Dwf} }}{{x_{D}^{2} }}} \right)^{0.5} $$
(14)
Table 1 Input data for the simulations
Full size table
Fig. 1
figure 1

Dimensionless pressure and pressure derivative versus dimensionless time for the simple model with wf = 0.008 ft and different values of fracture length

Full size image
Fig. 2
figure 2

Unified dimensionless pressure and pressure derivative versus dimensionless time divided by the dimensionless squared fracture length for the simple model with wf = 0.008 ft and different values of fracture length

Full size image

Pressure derivative analysis for the simple model

The TDS technique devised by Tiab (1993) requires the use of characteristic points and lines observed in the pressure and pressure derivative versus time log–log plot. If the fracture width is known, then the fracture permeability can be found by reading an arbitrary point during the linear flow regime once the dimensionless quantities given by Eqs. (3) and (5) are replaced in Eq. (14):

$$ k_{f} = 1.606\left( {\frac{q\mu B}{{h(t*\Delta P^{\prime})_{L} }}} \right)^{2} \frac{{t_{L} }}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }} $$
(15)

It is recommended to read the pressure derivative at a time of 1 h so that an averaged and representative value is achieved. Thus, Eq. (15) becomes:

$$ k_{f} = \frac{1.606}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }}\left( {\frac{q\mu B}{{h(t*\Delta P^{\prime})_{L1} }}} \right)^{2} $$
(16)

For gas systems, replace Eq. (7) instead of Eq. (5) in Eq. (14). It is applicable for both oil and gas wells:

$$ k_{f} = 163.465\left( {\frac{{q_{sc} T}}{{h[t*\Delta m(P^{\prime})]_{L} }}} \right)^{2} \frac{{t_{L} }}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }} $$
(17)
$$ k_{fb} = 163.465\left( {\frac{{q_{sc} T}}{{h[t*\Delta m(P^{\prime})]_{L1} }}} \right)^{2} \frac{1}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }} $$
(18)

The intercept between the pressure and the pressure derivative is unique, and their coordinates are 4.61315 and 1.0306, respectively. Using the definition of the dimensionless quantities given by Eqs. (3), (5), and (7), this point allowed to develop a couple of expressions to find fracture permeability:

$$ k_{f} = \frac{{145.52q\mu B\;x_{f} }}{{h(t*\Delta P^{\prime})_{{\text{int}}} w_{f} }} $$
(19)
$$ k_{f} = \frac{{17439.9325(\phi c_{t} )_{f} \mu x_{f}^{2} }}{{t_{{\text{int}}} }} $$
(20)

For gas wells,

$$ k_{f} = \frac{{1462.22qT\;x_{f} }}{{h[t*\Delta m(P)^{\prime}]_{{\text{int}}} w_{f} }} $$
(21)

Equation (20) works for either gas or oil wells.

Conventional analysis for the simple model

Substituting into the dimensionless parameters provided by Eqs. (3) and (4) into Eq. (13) and solving for the pressure drop, we get,

$$ \Delta P = m_{L} \sqrt t , $$
(22)

where

$$ m_{L} = 2.5346\frac{q\mu B}{{\sqrt {k_{f} } h}}\left( {\frac{1}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }}} \right)^{0.5} $$
(23)

Equation (22) indicates that a Cartesian plot of either pressure or pressure drop versus the square root of time yielding a straight-line whose slope was used to find an expression for the fracture permeability:

$$ k_{f} = \frac{6.4242}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }}\left( {\frac{q\mu B}{{m_{L} h}}} \right)^{2} $$
(24)

For gas wells, Eq. (6) is used instead of Eq. (4), so the permeability can be determined using

$$ k_{f} = \frac{652.05}{{(\phi c_{t} )_{f} \mu w_{f}^{2} }}\left( {\frac{qT}{{m_{L} h}}} \right)^{2} $$
(25)

The use of both Eqs. (24) and (25) can be extended to buildup tests if the Cartesian plot of either ΔP or Δm(P) is plotted against the tandem square root of time, (tp + Δt)0.5 – Δt0.5.

Pressure derivative analysis for the crossflow model

Equations (9) and (10) were used to generate the plots depicted in Figs. 3, 4, 5 for different scenarios, in all of which the late bilinear flow regime was developed because of the simultaneous linear flow from the matrix to fracture as well as the linear flow along the fracture. The unification of these is shown in Fig. 6 which led to the following governing equations:

Fig. 3
figure 3

Dimensionless pressure and pressure derivative versus dimensionless time for the crossflow model with wf = 0.008 ft, Cf = 0.5 and FD = 0.5, and different values of fracture length

Full size image
Fig. 4
figure 4

Dimensionless pressure and pressure derivative versus dimensionless time for the crossflow model with wf = 0.008 ft, xf = 200 ft and FD = 0.5, and different values of diffusivity ratios

Full size image
Fig. 5
figure 5

Dimensionless pressure and pressure derivative versus dimensionless time for the crossflow model with wf = 0.008 ft, xf = 200 ft and Cf = 2, and different values of permeability ratios

Full size image
$$ \frac{{P_{D} }}{{\sqrt {x_{D} } }} = 0.79\left( {\frac{{t_{Dwf} }}{{x_{D}^{2} C_{f} F_{D}^{2} }}} \right)^{0.25} $$
(26)
$$ \frac{{t_{D} *P_{D} ^{\prime}}}{{\sqrt {x_{D} } }} = 0.18\left( {\frac{{t_{Dwf} }}{{x_{D}^{2} C_{f} F_{D}^{2} }}} \right)^{0.25} $$
(27)

As done in the simple model, the dimensionless quantities given by Eqs. (3) and (5) were plugged into Eq. (27), and solving for the fracture permeability led to:

$$ k_{f} = 4.792\left( {\frac{q\mu B}{{h(t*\Delta P^{\prime})_{BL} \sqrt {w_{f} } }}} \right)^{4/3} \sqrt[3]{{\frac{{t_{BL} }}{{(\phi c_{t} )_{f} \mu C_{f} F_{D}^{2} }}}} $$
(28)

Again, reading the pressure derivative value at the time t = 1 h allowed to obtain the following:

$$ k_{f} = 4.792\left( {\frac{q\mu B}{{h(t*\Delta P^{\prime})_{BL1} \sqrt {w_{f} } }}} \right)^{4/3} \sqrt[3]{{\frac{1}{{(\phi c_{t} )_{f} \mu C_{f} F_{D}^{2} }}}} $$
(29)

Using Eq. (6) instead of Eq. (4) for gas wells,

$$ k_{f} = 104.462\left( {\frac{qT}{{h[t*\Delta m(P^{\prime})]_{BL} \sqrt {w_{f} } }}} \right)^{4/3} \sqrt[3]{{\frac{{t_{BL} }}{{(\phi c_{t} )_{f} \mu C_{f} F_{D}^{2} }}}} $$
(30)
$$ k_{f} = 104.462\left( {\frac{qT}{{h[t*\Delta m(P^{\prime})]_{BL1} \sqrt {w_{f} } }}} \right)^{4/3} \sqrt[3]{{\frac{1}{{(\phi c_{t} )_{f} \mu C_{f} F_{D}^{2} }}}} $$
(31)

As for the simple model, there exists a unique intersection point, as shown in Fig. 6, which possesses the coordinates 0.075 and 0.0269, thus allowing to find expressions to calculate either the fracture permeability or the product Cf FD2 once the dimensionless quantities have been equalized to the mentioned values, so:

$$ k_{f} = \frac{{3.7983q\mu B\sqrt {x_{f} /w_{f} } }}{{h(t*\Delta P^{\prime})_{{\text{int}}} }} $$
(32)
$$ C_{f} F_{D}^{2} = \frac{{k_{f} t_{{{\text{int}}}} }}{{284.41(\phi c_{t} )_{f} \mu x_{f}^{2} }} $$
(33)
Fig. 6
figure 6

Unified dimensionless pressure divided by the square root of dimensionless fracture and pressure derivative divided by the square root of dimensionless fracture versus dimensionless time divided by the dimensionless squared fracture length times, the diffusivity ratio times, the squared permeability ratio for the simple model with wf = 0.008 ft and different values of fracture length

Full size image

If the matrix permeability km is known, use Eq. (12) to calculate FD and replace that value to calculate Cf from the product Cf FD2:

$$ C_{f} = \frac{{k_{f} t_{{{\text{int}}}} }}{{284.41(\phi c_{t} )_{f} \mu x_{f}^{2} F_{D}^{2} }} $$
(34)

Equation (33) is relevant for gas wells but not Eq. (32). So, for gas wells, the definition given by Eq. (7) is used:

$$ k_{f} = \frac{{38.32qT\sqrt {x_{f} /w_{f} } }}{{h[t*\Delta m(P)^{\prime}]_{{\text{int}}} }} $$
(35)

Conventional analysis for the crossflow model

Substituting the dimensionless quantities defined by Eqs. (3) and (4) into Eq. (26) and solving for the pressure drop, we get:

$$ \Delta P = m_{BL} \sqrt[4]{t}, $$
(36)

where

$$ m_{BL} = \frac{14.215q\mu B}{{k_{f} h}}\left( {\frac{{k_{f} }}{{(\phi c_{t} )_{f} \mu w_{f}^{2} C_{f} F_{D}^{2} }}} \right)^{0.25} $$
(37)

A Cartesian plot of either pressure or pressure drop versus the fourth root of time or (tp + Δt)0.25 – Δt0.25- for buildup tests yields a straight line whose slope leads to finding the fracture permeability:

$$ k_{f} = 34.434\left( {\frac{q\mu B}{{hm_{BL} }}} \right)^{4/3} \sqrt[3]{{\frac{1}{{(\phi c_{t} )_{f} \mu w_{f}^{2} C_{f} F_{D}^{2} }}}} $$
(38)

Again, for gas wells, Eq. (6) is used instead of Eq. (4) into Eq. (26); it then becomes:

$$ k_{f} = 749.25\left( {\frac{qT}{{hm_{BL} }}} \right)^{4/3} \sqrt[3]{{\frac{1}{{(\phi c_{t} )_{f} \mu w_{f}^{2} C_{f} F_{D}^{2} }}}} $$
(39)

By comparing Eq. (33) with Eq. (39), we can observe that the conventional analysis has a drawback using a more complete reservoir characterization since the analysis requires the product Cf FD2 to be known, while the TDS technique can be used to estimate the parameter product. This is very important since knowing the diffusivity ratio allows finding the permeability ratio and vice versa.

Examples

Synthetic example 1

Synthetic test data were generated using data from the first column of Table 2 and reported in Fig. 7. The interpretation of this test using both the TDS technique and conventional analysis is requested.

Table 2 Input data for the examples
Full size table
Fig. 7
figure 7

Pseudopressure and pseudopressure derivative versus time log–log plot for synthetic example 1

Full size image

TDS technique

As shown in Fig. 7, only the bilinear flow is clearly observed. From its plot, the following information was read:

tBL = 9.665 h [t*Δm(P’)]BL = 146,096,927.4 psi2/cp.

tint = 0.000182 h [t*Δm(P’)]int = 2,879,746.83 psi2/cp.

Use Eq. (35) to find the fracture permeability:

$$ k_{f} = \frac{{38.32(3000)(650)\sqrt {500/0.015} }}{600(2879746.83)} = 7.9\;{\text{md}} $$

Use Eq. (12) to find FD and replace that value in Eq. (34) to calculate Cf and find the product Cf FD2:

$$ F_{D} = \frac{0.1}{{7.896}} = 0.0013 $$
$$ C_{f} = \frac{39.41(0.00318)}{{284.41(0.05)(1 \times 10^{ - 4} )(0.75)(300^{2} )(0.013^{2} )}} = 78.77 $$
$$ C_{f} F_{D}^{2} = 78.77(0.013^{2} ) = 0.00013 $$

Now, we can find the product Cf FD2 using Eq. (33):

$$ C_{f} F_{D}^{2} = \frac{7.9(0.000182)}{{284.41(0.08)(0.0001)(0.02)(500^{2} )}} = 0.000126 $$

Both are closely compared to the value of 0.0001 from Table 2. However, the input value will be used further in the example.

Find the fracture permeability once again using Eq. (30):

$$ \begin{gathered} k_{f} = 104.462\left( {\frac{3000(650)}{{600(146096927.4)\sqrt {0.015} }}} \right)^{4/3} \hfill \\ \quad \sqrt[3]{{\frac{9.665}{{(0.08)(0.0001)(0.02)(0.0001)}}}} = 9.1\;{\text{md}} \hfill \\ \end{gathered} $$

Conventional analysis

Figure 8 presents the Cartesian plot of Δm(P) versus t0.25 whose straight line has the following slope.

Fig. 8
figure 8

Pseudopressure versus the fourth root of the time Cartesian plot for synthetic example 1

Full size image

mBL = 331,091,833.4 psi2/(cp h).

Contrary to the TDS technique’s procedure, in this case, Cf FD2 is assumed to be known. Thus, we can use Eq. (39) to find the fracture permeability:

$$ \begin{gathered} k_{f} = 749.25\left( {\frac{3000(650)}{{600(331091833.4)}}} \right)^{4/3} \hfill \\ \quad \sqrt[3]{{\frac{1}{{(0.08)(0.0001)(0.02)(0.015^{2} )(0.0001)}}}} = 10.3\;{\text{md}} \hfill \\ \end{gathered} $$

Synthetic example 2

Synthetic test data were generated using data from the second column of Table 2 and reported in Fig. 9. The interpretation of this test using both the TDS technique and conventional analysis is requested.

Fig. 9
figure 9

Pressure and pressure derivative versus time log–log plot for synthetic example 2

Full size image

TDS Technique

As shown in Fig. 9, only the linear flow is clearly observed. From the plot, the following information was read:

tL = 55.216 h [t*ΔP’]L = 2092.327 psi2/cp.

tint = 4.141 h [t*ΔP’]int = 491.875 psi2/cp.

Use Eq. (15), Eq. (19), and Eq. (20) to find the fracture permeability:

$$ \begin{gathered} k_{f} = 1.606\left( {\frac{300(0.5)(1.45)}{{500(2092.327)}}} \right)^{2} \frac{55.216}{{(0.03)(2 \times 10^{ - 4} )(0.5)(0.1^{2} )}} \hfill \\ = 127.76{\text{ md}} \hfill \\ \end{gathered} $$
$$ k_{f} = \frac{{145.52(300)(0.5)(1.45)\left( {100} \right)}}{500(491.875)(0.1)} = 128.69{\text{ md}} $$
$$ k_{f} = \frac{{17439.9325(0.03)(2 \times 10^{ - 4} )(0.5)(100^{2} )}}{4.141} = 126.36{\text{ md}} $$

Figure 10 presents the Cartesian plot of ΔP versus t0.5 whose straight line has the following slope.

Fig. 10
figure 10

Pressure versus the square root of the time Cartesian plot for synthetic example 2

Full size image

mBL = 573.907 psi/h.

Use Eq. (24) to find the fracture permeability:

$$ \begin{gathered} k_{f} = \frac{6.4242}{{(0.03)(2 \times 10^{ - 4} )(0.5)(0.1)^{2} }}\left( {\frac{300(0.5)(1.45)}{{500(573.907)}}} \right)^{2} \hfill \\ = 123.02{\text{ md}} \hfill \\ \end{gathered} $$

Synthetic example 3

Synthetic test data were generated using data from the third column of Table 2 and reported in Fig. 11. It is requested that the interpretation of this test be done using both the TDS technique and conventional analysis.

Fig. 11
figure 11

Pressure and pressure derivative versus time log–log plot for synthetic example 3

Full size image

TDS technique

As shown in Fig. 11, only the bilinear flow is clearly observed. From the plot, the following information was read:

tBL = 10.664 h [t*ΔP’]BL = 79.0503 psi.

tint = 0.00318 h [t*ΔP’]int = 2.8468 psi.

Use Eq. (32) to find the fracture permeability:

$$ k_{f} = \frac{{3.7983(120)(0.75)(1.34)\sqrt {300/0.02} }}{500(2.8468)} = 39.41{\text{ md}} $$

Use Eq. (12) to find FD and replace that value in Eq. (34) to calculate Cf and find the product Cf FD2:

$$ F_{D} = \frac{0.5}{{39.71}} = 0.0051 $$
$$ C_{f} = \frac{39.41(0.00318)}{{284.41(0.05)(1 \times 10^{ - 4} )(0.75)(300^{2} )(0.0127^{2} )}} = 50.77 $$
$$ C_{f} F_{D}^{2} = 50.77(0.051^{2} ) = 0.0013 $$

Now, find the product Cf FD2 using Eq. (33):

$$ C_{f} F_{D}^{2} = \frac{39.41(0.00318)}{{284.41(0.05)(1 \times 10^{ - 4} )(0.75)(300^{2} )}} = 0.0013 $$

Both are closely compared to the value of 0.0013 from Table 2.

Find the fracture permeability once again using Eq. (28):

$$ \begin{gathered} k_{f} = 4.792\left( {\frac{120(0.75)(1.34)}{{500(79.0503)\sqrt {0.02} }}} \right)^{4/3} \hfill \\ \sqrt[3]{{\frac{10.664}{{(0.05)(1 \times 10^{ - 4} )(0.75)(0.0013)}}}} = 37.29{\text{ md}} \hfill \\ \end{gathered} $$

Conventional analysis

Figure 12 presents the Cartesian plot of ΔP versus t0.25 whose straight line has the following slope.

Fig. 12
figure 12

Pressure versus the fourth root of the time Cartesian plot for synthetic example 3

Full size image

mBL = 178.962 psi h.

Contrary to the TDS technique’s procedure, in this case, Cf FD2 is assumed to be known. Thus, we can use Eq. (37) to find fracture permeability:

$$ \begin{gathered} k_{f} = 34.434\left( {\frac{120(0.75)(1.34)}{{500(178.962)}}} \right)^{4/3} \hfill \\ \sqrt[3]{{\frac{1}{{(0.05)(1 \times 10^{ - 4} )(0.75)(0.02^{2} )(0.0013)}}}} = 41.57{\text{ md}} \hfill \\ \end{gathered} $$

Comments on the results

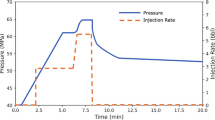
Figure 13 shows real-field data digitized from Chu et al. (2020) observed in Well 4H from testing Well 3H. The same information was reported in a dimensionless form by Han et al. (2019). Note that the linear flow regime is observed, so such information pertains to the simple model. However, there was no access to the reservoir and fluid properties; therefore, no interpretation could be performed. The equations developed in this work were tested using simulated examples and both the TDS technique and conventional analysis provided successful results. Also, the superiority of the TDS technique over conventional analysis was demonstrated. First, conventional analysis only provides one source of calculation, while the TDS technique provides two, so the product Cf FD2 can be found from the interception point between the pressure and the pressure derivative.

Fig. 13
figure 13

Actual field data reported by Han et al, (2019)

Full size image

Conclusions

  1. 1.

    An interpretation technique for interference data on two wells connected by a fracture in shaly formations is presented for the first time using both the TDS technique and conventional analysis. New equations have been developed for both the techniques for estimating the fracture permeability, and they were successfully tested using simulated examples.

  2. 2.

    Conventional analysis only provides fracture permeability, while the TDS technique provides both fracture permeability and the product Cf FD2. This is of importance because if the diffusivity ratio is known, then the permeability ratio can be estimated and vice versa. This is an advantage the TDS technique has over conventional analysis. However, the methods are meant to be used in combination instead of using them separately.