Abstract
This paper focus on the synthesis of a robust extended H∞ observer based on the combination of the mean value theorem and the sector non-linearity approach, which is applied to the estimation of all ordinary states of the Induction Motor (IM) and the rotor position under the Open Loop Field Oriented Control (OL-FOC). The main objective of this observer is to ensure a minimum disturbance attenuation level of the estimation error; at first, we introduce and formulate the problem of the robust extended observer that can be designed based on these approaches, secondly it will be applied to a class of Lipschitz nonlinear system of the IM. At this stage, it is possible to express the nonlinear error dynamics of the state observer error as a convex combination of known matrices with time varying coefficients as in linear parameter varying systems. Then, it is easy to use the Lyapunov theory such that the stability conditions are obtained and expressed in a form of Linear Matrix Inequalities (LMI’s), so, the extended observer gain is determined by solving the LMI’s through the YALMIP software. The effectiveness of the concept of the proposed approach is performed by measuring the two line currents and estimating all the IM drive states and the rotor position under the OL-FOC through an illustrative simulation to affirm the effectiveness of the proposed concept.








Similar content being viewed by others
Abbreviations
- x(t):
-
State vector
- \(\hat{x}\left( t \right)\) :
-
Estimated state vector
- x r(t):
-
Reference state vector
- e(t):
-
State estimation error
- u(t):
-
Input vector
- y(t):
-
Output vector
- w(t):
-
Disturbance vector
- w r, w s :
-
Rotor and stator speed
- w rr :
-
Rotor speed reference
- w sr :
-
Electrical stator speed reference
- \(\theta_{r}\) :
-
Rotor position
- \(\varPsi_{rd} , \varPsi_{rq}\) :
-
The (d,q) Rotor flux
- \(\varPsi_{r}\) :
-
Rotor flux reference
- i sd, i sq :
-
The (d,q) stator currents
- U ds, U qs :
-
The (d,q) stator voltages
- U dsr, U qsr :
-
The (d,q) open loop controls
- L r, L s :
-
Rotor and stator inductances
- R r, R s :
-
Rotor and stator resistances
- J :
-
Moment of inertia
- f :
-
Friction coefficient
- n p :
-
Pole pair number
- T L :
-
Load torque
- M :
-
Mutual inductance
- L 0 :
-
Observer gain
References
Ahrens JH, Khalil HK (2009) High-gain observers in the presence of measurement noise: a switched-gain approach. Automatica 45:936–943
Allag A, Benakcha A, Allag M, Zein I, Ayad MY (2015) Classical state feedback controller for nonlinear systems using mean value theorem: closed loop-FOC of PMSM motor application. Front Energy 9:413
Alonge F, Cirrincione M, Pucci M, Sferlazza A (2017) A nonlinear observer for rotor flux estimation of induction motor considering the estimated magnetization characteristic. IEEE Trans Ind Appl 53:5952–5965
Asseu O, Kouacou MA, Ori TR, Yéo Z, Koffi M, Lin-Shi X (2010) Nonlinear control of an induction motor using a reduced-order extended sliding mode observer for rotor flux and speed sensorless estimation. Engineering 2:813
Gacho J, Zalman M (2010) IM based speed servodrive with luenberger observer. J Electr Eng 61:149
Hammoudi MY, Allag A, Becherif M, Benbouzid M, Alloui H (2014) Observer design for induction motor: an approach based on the mean value theorem. Front Energy 8:426–433
Ichalal D, Marx B, Mammar S, Maquin D, Ragot J (2018) How to cope with unmeasurable premise variables in Takagi–Sugeno observer design: dynamic extension approach. Eng Appl Artif Intell 67:430–435
Kandoussi Z, Boulghasoul Z, Elbacha A, Tajer A (2017) Sensorless control of induction motor drives using an improved MRAS observer. J Electr Eng Technol 12:1456–1470
Manohar M, Das S (2017) Current sensor fault-tolerant control for direct torque control of induction motor drive using flux-linkage observer. IEEE Trans Ind Inf 13:2824–2833
Meziane S, Toufouti R, Benalla H (2008) Nonlinear control of induction machines using an extended kalman filter. Acta Polytech Hung 5:41–58
Park C-W, Lee S (2007) Nonlinear observer based control of induction motors. Electr Eng (Archiv fur Elektrotechnik) 90:107–113
Regaya CB, Farhani F, Zaafouri A, Chaari A (2017) An adaptive sliding-mode speed observer for induction motor under backstepping control. Int J Innov Comput I 11:763–771
Tanaka K, Ohtake H, Wang HO (2007) A descriptor system approach to fuzzy control system design via fuzzy Lyapunov functions. IEEE Trans Fuzzy Syst 15:333–341
Yin Z, Li G, Zhang Y, Liu J, Sun X, Zhong Y (2017) A Speed and flux observer of induction motor based on extended Kalman filter and Markov chain. IEEE Trans Power Electron 32:7096–7117
Zaidi S, Naceri F, Abdssamed R (2014) Input–output linearization of an induction motor using MRAS observer. Int J Adv Sci Technol 68:49–56
Zhao L, Huang J, Liu H, Li B, Kong W (2014) Second-order sliding-mode observer with online parameter identification for sensorless induction motor drives. IEEE Trans Ind Electron 61:5280–5289
Zina HB, Allouche M, Souissi M, Chaabane M, Chrifi-Alaoui L, Bouattour M (2016) Descriptor observer based fault tolerant tracking control for induction motor drive. Automatika 57:703–713
Zina HB, Allouche M, Souissi M, Chaabane M, Chrifi-Alaoui L (2017) Robust sensor fault-tolerant control of induction motor drive. Int J Fuzzy Syst 19:155–166
Zina HB, Allouche M, Souissi M, Chaabane M, Chrifi-Alaoui L, Bouattour M (2018) A Takagi–Sugeno fuzzy control of induction motor drive: experimental results. Int J Autom Control 12:44–61
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
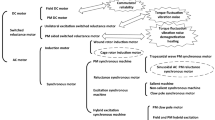
In the part, a flow chart about the different steps in order to obtain the robust extended observer gain for the class of strong nonlinear system that the IM drive:

Rights and permissions
About this article
Cite this article
Zeghib, O., Allag, A., Allag, M. et al. A robust extended H∞ observer based on the mean value theorem designed for induction motor drives. Int J Syst Assur Eng Manag 10, 533–542 (2019). https://doi.org/10.1007/s13198-019-00766-0
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13198-019-00766-0