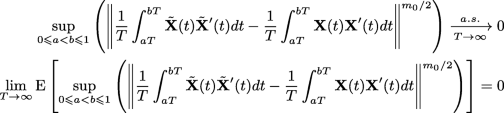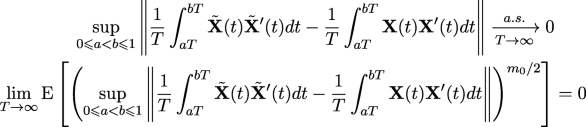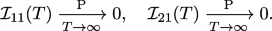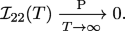Estimation and Testing in Multivariate Generalized Ornstein-Uhlenbeck Processes with Change-Points

Abstract

In this paper, we consider an inference problem about the drift parameter matrix of multivariate generalized Ornstein-Uhlenbeck processes with multiple unknown change-points in the case where the drift parameter matrix is suspected to satisfy some restrictions. The established results generalize in six ways some recent findings about univariate generalized Ornstein-Uhlenbeck processes. First, we consider a multivariate process with multiple change-points. Second, we weaken the assumptions underlying some recent findings and we derive the unrestricted estimator (UE) and the restricted estimator (RE). Third, we derive the asymptotic property of the UE and the RE. Fourth, we construct a test for testing the hypothesized constraint. Fifth, we establish the asymptotic power of the derived test and we prove that it is consistent. Sixth, we derive a class of shrinkage estimators (SEs) and its asymptotic distributional risk.

Appendices

Appendix A: Proofs of Some Fundamental Results

Proof 14 (Proof of Proposition 2.4).

We apply first the inequality $\left \|\displaystyle {\sum \limits _{j=1}^{m+1}}b_{j}\right \|^{m_{0}}\leqslant (m+1)^{m_{0}-1}\displaystyle {\sum \limits _{j=1}^{m+1}}\|b_{j}\|^{m_{0}}$, for all $b_{1}, b_{2}, \dots ,b_{m+1}$ d-column vectors. Thus, we have

$\displaystyle \text {E}[\|\tilde {X}(t)\|^{m_{0}}]\leqslant 2^{m_{0}-1}(m+1)^{m_{0}-1}\sum \limits _{j=1}^{m+1}\text {E}[\|\tilde {h}_{j}(t)\|^{m_{0}} +\|\tilde {Z}_{j}(t)\|^{m_{0}}].$ Then,

$$ \begin{array}{@{}rcl@{}} \text{E}[\|\tilde{X}(t)\|^{m_{0}}]&\leqslant& (2(m+1))^{m_{0}-1}\sum\limits_{j=1}^{m+1}\|\tilde{h}_{j}(t)\|^{m_{0}} \\&&+(2(m+1))^{m_{0}-1}\sum\limits_{j=1}^{m+1}\text{E}[\|\tilde{Z}_{j}(t)\|^{m_{0}}]. \end{array} $$

(A.1)

Note that, $\displaystyle \|\tilde {h}_{j}(t)\|^{m_{0}} =\displaystyle \Bigg \|e^{-A_{j}t}\sum \limits _{k=1}^{p}\mu _{k,j}{\int \limits }_{-\infty }^{t}e^{A_{j}s}\varphi _{k}(s)ds\Bigg \|^{m_{0}}.$ Then,

$$ \begin{array}{@{}rcl@{}} \sum\limits_{j=1}^{m+1}\|\tilde{h}_{j}(t)\|^{m_{0}}\leqslant (m+1)^{m_{0}-1} \displaystyle\sum\limits_{j=1}^{m+1}\left\|\sum\limits_{k=1}^{p}\mu_{k,j}{\int}_{-\infty}^{t}e^{-A_{j}(t-s)}\varphi_{k}(s)ds\right\|^{m_{0}}\\ \leqslant p^{m_{0}-1} (m+1)^{m_{0}-1}\displaystyle\sum\limits_{j=1}^{m+1} \sum\limits_{k=1}^{p}|\mu_{k,j}|^{m_{0}}\left( {\int}_{-\infty}^{t}\|e^{-A_{j}(t-s)}\|\|\varphi_{k}(s)\|ds\right)^{m_{0}}. \end{array} $$

This gives, $\displaystyle \sum \limits _{j=1}^{m+1}\|\tilde {h}_{j}(t)\|^{m_{0}}\!\leqslant \! p^{m_{0}-1}(m+1)^{m_{0}-1}K_{\mu }K_{\varphi }\displaystyle \sum \limits _{j=1}^{m+1}\left ({\int \limits }_{0}^{\infty }\|e^{-A_{j}s}\|ds\!\right )^{m_{0}}$ where K_μ and K_φ are strictly positive real numbers such that$\displaystyle \sum \limits _{j=1}^{m+1} \sum \limits _{k=1}^{p}|\mu _{k,j}$ $|^{m_{0}}\leqslant K_{\mu }$ and $\|\varphi _{k}(s)\|\leqslant K_{\varphi }$ for all s, $k=1,2,\dots ,m+1$. Then

$$ \begin{array}{@{}rcl@{}} \quad{ } {\sum}_{j=1}^{m+1}\|\tilde{h}_{j}(t)\|^{m_{0}}\leqslant p^{m_{0}-1}d^{m_{0}/2}(m+1)^{m_{0}-1}K_{\mu}K_{\varphi}\displaystyle\sum\limits_{j=1}^{m+1}\left( (\lambda_{1}(A_{j})/2)^{-1}\right)^{m_{0}},\!\!\!\!\!\!\!\!\\ \end{array} $$

(A.2)

$\forall \ t\geqslant 0$. Further, we have

$$ \begin{array}{@{}rcl@{}} &&\text{E}[\|\tilde{Z}_{j}(t)\|^{m_{0}}]\leqslant 2^{m_{0}-1}\text{E}\left[\Bigg\|e^{-A_{j}t}{{\int}_{0}^{t}}e^{A_{j}s}{\Sigma}^{1/2}dW^{(1)}_{s}\Bigg\|^{m_{0}}\right] \\ &&+2^{m_{0}-1}\text{E}\left[\Bigg\|e^{-A_{j}t}{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}dW^{(2)}_{-s}\Bigg\|^{m_{0}}\right]. \end{array} $$

Then, by using Proposition 2.4 and Lemma 2.4, we get

$$ \begin{array}{@{}rcl@{}} \text{E}\left( \sum\limits_{j=1}^{m+1}\|\tilde{Z}_{j}(t)\|^{m_{0}}\right)\leqslant 2^{m_{0}-1}\gamma_{1}(m_{0})\displaystyle\sum\limits_{j=1}^{m+1}\left( \text{trace}\left[ (A_{j} + A^{\prime}_{j})^{-1}\right]\right)^{m_{0}/2}\left( 1-e^{-t\lambda_{d}(A_{j})}\right)^{m_{0}/2},\\ + 2^{m_{0}-1}\gamma_{1}(m_{0})\displaystyle\sum\limits_{j=1}^{m+1}\left( \text{trace}\left[ (A_{j}+A^{\prime}_{j})^{-1}\right]\right)^{m_{0}/2} e^{-tm_{0}\lambda_{1}(A_{j})/2}\quad{ }\quad{ } \end{array} $$

and then,

$$ \begin{array}{@{}rcl@{}} \text{E}\left( \sum\limits_{j=1}^{m+1}\|\tilde{Z}_{j}(t)\|^{m_{0}}\right)\leqslant 2^{m_{0}-1}\gamma_{1}(m_{0}) \displaystyle\sum\limits_{j=1}^{m+1}\left( \text{trace}\left[ (A_{j}+A^{\prime}_{j})^{-1}\right]\right)^{m_{0}/2}, \forall t\geqslant0.\quad{ }\quad{ } \end{array} $$

(A.3)

Then, by combining Eqs. A.1, B.2 and A.3, we get

$$ \begin{array}{@{}rcl@{}} \sup_{t\geqslant 0}\text{E}[|\tilde{X}(t)|^{m_{0}}]&\leqslant& (2 (m+1)^{2}p)^{m_{0}-1}d^{m_{0}/2} K_{\mu}K_{\varphi}\displaystyle\sum\limits_{j=1}^{m+1}(\lambda_{1}(A_{j})/2)^{-m_{0}}\\ & & +(4(m+1))^{m_{0}-1} \gamma_{1}(m_{0}) \displaystyle\sum\limits_{j=1}^{m+1}\left( \text{trace}\left[ (A_{j}+A^{\prime}_{j})^{-1}\right]\right)^{m_{0}/2}<\infty, \end{array} $$

this completes the proof. □

Proof 15 (Proof of Proposition 2.5).

• (i) Recall that $\displaystyle (C_{0}(\gamma _{m}))^{m_{0}}=\displaystyle \iota _{1}\sum \limits _{j=1}^{m+1} \left ({\int \limits }_{-\infty }^{0}\|e^{A_js}\|ds\right )^{m_{0}}+\iota _{2} \sum \limits _{j=1}^{m+1} \left \|{\int \limits }_{-\infty }^{0}\right .$ $\left .e^{A_js}{\Sigma }^{1/2}d\tilde {W}_s\right \|^{m_{0}}+\iota _{3}\sum \limits _{j=1}^{m+1}\|X(\gamma _{j-1})\|^{m_{0}}$, with $\iota _{1}=(3p(m+1))^{m_{0}-1}K^{*}$ $d^{m_{0}/2}$, $\iota _{2}=d^{m_{0}/2} (3(m+1))^{m_{0}-1}$ and $\iota _{3}=(3(m+1))^{m_{0}-1}$. Then,

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left( \displaystyle (C_{0}(\gamma_{m}))^{m_{0}}\right)&=&\displaystyle\iota_{1}\sum\limits_{j=1}^{m+1} \left[\left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}}\right]+\iota_{2} \sum\limits_{j=1}^{m+1} \text{E}\left[ \left\|{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}d\tilde{W}_{s}\right\|^{m_{0}}\right]\\ &&+\iota_{3}\sum\limits_{j=1}^{m+1} \text{E}\left[\|X(\gamma_{j-1})\|^{m_{0}}\right] \end{array} $$

  and then, by combining Lemma 2.4 and Part (2) of Proposition 2.4, we have

  $$ \begin{array}{@{}rcl@{}} &&{{}}\text{E}\left( \displaystyle (C_{0}(\gamma_{m}))^{m_{0}}\right)\leqslant\displaystyle\iota_{1}\sum\limits_{j=1}^{m+1} \left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}}+\iota_{2} \sum\limits_{j=1}^{m+1} \gamma_{2}(m_{0})\left[\text{trace}\left( (A_{j}+A^{\prime}_{j})^{-1}\right)\right]^{m_{0}/2}\\ &&{\kern30pt}+\iota_{3} \text{E}\left[\|X(0)\|^{m_{0}}\right] + \iota_{3}\sum\limits_{j=2}^{m+1}3^{(m_{0}-1)(j-1)}\text{E}\left( \|\mathbf{X}(0)\|^{m_{0}}\right) + d^{m_{0}/2}\\ &&{\kern30pt}\left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}}\times \sum\limits_{i=0}^{j-2}3^{(m_{0}-1)(i+1)}\\&& {\kern30pt}\left( \left( \lambda_{\max}\left( A_{j-1-i}^{-1}\right)\right)^{m_{0}} +(\lambda_{\max}(A_{j-1-i}^{-1}))^{m_{0}/2}\right). \end{array} $$

  Hence,

  $$ \begin{array}{@{}rcl@{}} &&{{}}\text{E}\left( \displaystyle (C_{0}(\gamma_{m}))^{m_{0}}\right)\leqslant\displaystyle\iota_{1}\displaystyle\iota_{1}d^{m_{0}/2}2^{m_{0}}\sum\limits_{j=1}^{m+1} \left( \lambda_{\max}(A_{j}^{-1})\right)^{m_{0}}+\iota_{2} \sum\limits_{j=1}^{m+1} \gamma_{2}(m_{0})\left[\text{trace}\left( (A_{j}+A^{\prime}_{j})^{-1}\right)\right]^{m_{0}/2}\\ &&+\iota_{3} \text{E}\left[\|X(0)\|^{m_{0}}\right]+ \iota_{3}3^{m_{0}-1}\frac{3^{m(m_{0}-1)}-1}{3^{m_{0}-1}-1}\text{E}\left( \|\mathbf{X}(0)\|^{m_{0}}\right) \\&&+ d^{m_{0}/2} \left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}} \\ &&\times \sum\limits_{i=0}^{j-2}3^{(m_{0}-1)(i+1)}\left( \left( \lambda_{\max}\left( A_{j-1-i}^{-1}\right)\right)^{m_{0}} +(\lambda_{\max}(A_{j-1-i}^{-1}))^{m_{0}/2}\right)<\infty, \end{array} $$

  this proves Part (i).

• (ii) We recall first the inequality

  $$ \begin{array}{@{}rcl@{}} \left\|\displaystyle{\sum\limits_{j=1}^{m+1}}b_{j}\right\|^{m_{0}}\leqslant (m+1)^{m_{0}-1}\displaystyle{\sum\limits_{j=1}^{m+1}}\|b_{j}\|^{m_{0}}, \end{array} $$

  (A.4)

  for all $b_{1}, b_{2}, \dots ,b_{m+1}$ d-column vectors. From the inequality in Eq. A.4, we get

  $$ \begin{array}{@{}rcl@{}} \|\tilde{\mathbf{X}}(t)-\mathbf{X}(t)\|^{m_{0}} \leqslant \ 3^{m_{0}-1}(m+1)^{m_{0}-1}\displaystyle{\sum\limits_{j=1}^{m+1}}\|\tilde{h}_{j}(t)-h_{j}(t)\|^{m_{0}}\\ +3^{m_{0}-1}(m+1)^{m_{0}-1}\displaystyle{\sum\limits_{j=1}^{m+1}}\|\tilde{Z}_{j}(t)-{Z}_{j}(t)\|^{m_{0}}\\ +3^{m_{0}-1}(m+1)^{m_{0}-1}\displaystyle{\sum\limits_{j=1}^{m+1}}\|e^{-A_{j}(t-\gamma_{j-1})}X(\gamma_{j-1})\|^{m_{0}}. \end{array} $$

  (A.5)

  Further, from Cauchy-Schwarz’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} &&\displaystyle\|\tilde{h}_{j}(t)-h_{j}(t)\|^{m_{0}} =\left\|e^{-A_{j}(t-\gamma_{j-1})}\sum\limits_{k=1}^{p}\mu_{k,j}{\int}_{-\infty}^{0}e^{A_{j}s}\varphi(s)ds\right\|^{m_{0}}\\ &\leqslant& \left\|e^{-A_{j}(t-\gamma_{j-1})}\right\|^{m_{0}}\left\|\sum\limits_{k=1}^{p}\mu_{k,j}{\int}_{-\infty}^{0}e^{A_{j}s}\varphi(s)ds\right\|^{m_{0}}, \end{array} $$

  then, by Jensen’s inequality,

  $$ \begin{array}{@{}rcl@{}} &&\displaystyle\|\tilde{h}_{j}(t)-h_{j}(t)\|^{m_{0}}\leqslant p^{m_{0}-1}\|e^{-A_{j}(t-\gamma_{j-1})}\|^{m_{0}}\\ &&\times\sum\limits_{k=1}^{p}|\mu_{k,j}|^{m_{0}}\left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}-1}{\int}_{-\infty}^{0} \|e^{A_{j}s}\|\|\varphi(s)\|^{m_{0}}ds. \end{array} $$

  This gives,

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\|\tilde{h}_{j}(t)-h_{j}(t)\|^{m_{0}} &\!\!\leqslant& p^{m_{0}-1}d^{m_{0}/2} K_{\mu} K_{\varphi}e^{-\lambda_{1}(A_{j})(t-\gamma_{j-1})/2}\left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}}, \end{array} $$

  (A.6)

  where K_μ > 0 and K_φ > 0 such that $\|\mathbf {\varphi }(s)\|^{m_{0}}\!\leqslant \! K_{\varphi }$ and $\displaystyle \sum \limits _{k=1}^{p}|\mu _{k,j}|^{m_{0}}\leqslant K_{\mu }$. Further, $\displaystyle \|\tilde {Z}_j(t)-{Z}_{j}(t)\|^{m_{0}}=\left \| e^{-A_j(t-\gamma _{j-1})}{\int \limits }_{-\infty }^{0}e^{A_js}{\Sigma }^{1/2}d\tilde {W}_s\right \|^{m_{0}}$. Then,

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\|\tilde{Z}_{j}(t)-{Z}_{j}(t)\|^{m_{0}}\leqslant d^{m_{0}/2} e^{-\lambda_{1}(A_{j})(t-\gamma_{j-1}) m_{0}/2}\left\|{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}d\tilde{W}_{s}\right\|^{m_{0}}. \end{array} $$

  (A.7)

  From Eqs. A.6 and A.7, we get

  $$ \begin{array}{@{}rcl@{}} {~}\sum\limits_{j=1}^{m+1}\displaystyle|\tilde{h}_{j}(t)-h_{j}(t)|^{m_{0}}\leqslant p^{m_{0}-1}d^{m_{0}/2} K^{*} \sum\limits_{j=1}^{m+1} e^{-\lambda_{1}(A_{j})m_{0}(t-\gamma_{j-1})/2}\left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}}, \\ \sum\limits_{j=1}^{m+1}\displaystyle|\tilde{Z}_{j}(t)-{Z}_{j}(t)|^{m_{0}}\leqslant d^{m_{0}/2}\sum\limits_{j=1}^{m+1} e^{-\lambda_{1}(A_{j})m_{0}(t-\gamma_{j-1})/2}\left\|{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}d\tilde{W}_{s}\right\|^{m_{0}}, \end{array} $$

  $t\geqslant \gamma _{m}$, with K^∗ = K_μK_φ. Then,

  $$ \begin{array}{@{}rcl@{}} {{}}\sum\limits_{j=1}^{m+1}\displaystyle|\tilde{h}_{j}(t)-h_{j}(t)|^{m_{0}}\leqslant p^{m_{0}-1}d^{m_{0}/2} K^{*} e^{-a_{(1)}m_{0}(t-\gamma_{m})/2}\sum\limits_{j=1}^{m+1} \left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}}, \\ \sum\limits_{j=1}^{m+1}\displaystyle|\tilde{Z}_{j}(t)-{Z}_{j}(t)|^{m_{0}}\leqslant d^{m_{0}/2} e^{-a_{(1)}m_{0}(t-\gamma_{m})/2}\sum\limits_{j=1}^{m+1} \left\|{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}d\tilde{W}_{s}\right\|^{m_{0}}, \end{array} $$

  (A.8)

  $t\geqslant \gamma _{m}$. Then, by Eqs. A.5 and A.8, we have

  $$ \begin{array}{@{}rcl@{}} |\tilde{\mathbf{X}}(t)-\mathbf{X}(t)|^{m_{0}}\leqslant (C_{0}(\gamma_{m}))^{m_{0}}e^{-a_{(1)}m_{0}(t-\gamma_{m})/2}, \quad{ } t\geqslant\gamma_{m}, \end{array} $$

  (A.9)

  where

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle (C_{0}(\gamma_{m}))^{m_{0}} = \iota_{1}\sum\limits_{j=1}^{m+1} \left( {\int}_{-\infty}^{0}\|e^{A_{j}s}\|ds\right)^{m_{0}} + \iota_{2} \sum\limits_{j=1}^{m+1} \left\|{\int}_{-\infty}^{0}e^{A_{j}s}{\Sigma}^{1/2}d\tilde{W}_{s}\right\|^{m_{0}} + \iota_{3}\sum\limits_{j=1}^{m+1}\|X(\gamma_{j-1})\|^{m_{0}}, \end{array} $$

  with $\iota _{1}=(3p(m+1))^{m_{0}-1}K^{*}d^{m_{0}/2}$, $\iota _{2}=d^{m_{0}/2} (3(m+1))^{m_{0}-1}$ and $\iota _{3}=(3(m+1))^{m_{0}-1}$. Then, the proof of Part (ii) follows from the fact that, from Part (i), $\text {E}\left ((C_{0}(\gamma _{m}))^{m_{0}}\right )<\infty$.

• (iii). The convergence in $L^{m_{0}}$ follows directly from Part (ii) together with the fact that the process $\left \{\tilde {\mathbf {X}}(t)-\mathbf {X}(t):t\geqslant 0\right \}$ has continuous paths. Further, we from Part (ii), we have

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left\{\displaystyle{\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})-\mathbf{X}(t+\gamma_{m})\big\|^{m_{0}}\right\} \leqslant \text{E}\left( (C_{0}(\gamma_{m}))^{m_{0}}\right) e^{-a_{(1)}m_{0}2^{n}}, \end{array} $$

  and then

  $$ \begin{array}{@{}rcl@{}} \displaystyle{\sum\limits_{n=1}^{\infty}}\text{E}\left\{\displaystyle{\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})-\mathbf{X}(t+\gamma_{m})\big\|^{m_{0}}\right\}<\infty. \end{array} $$

  Therefore, together with the fact that $\left \{\tilde {\mathbf {X}}(t)-\mathbf {X}(t):t\geqslant 0\right \}$ is a process with continuous paths, the convergence almost surely follows from Borel-Cantelli’s lemma.

• (iv) We have

  $$ \begin{array}{@{}rcl@{}} &{}\big\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\big\|^{m_{0}/2}= \big\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\tilde{\mathbf{X}}^{\prime}(t)+\mathbf{X}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\big\|^{m_{0}/2}\\ &{}\leqslant 2^{m_{0}/2-1}\|\tilde{\mathbf{X}}(t)-\mathbf{X}(t)\|^{m_{0}/2}\|\tilde{\mathbf{X}}(t)\|^{m_{0}/2}+ 2^{m_{0}/2-1}\|\tilde{\mathbf{X}}(t)-\mathbf{X}(t)\|^{m_{0}/2}\|\mathbf{X}(t)\|^{m_{0}/2}. \end{array} $$

  Then, together with Eq. A.9, for all $t\geqslant \gamma _{m}$, we get

  $$ \big\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\big\|^{m_{0}/2}\leqslant (C_{0}(\gamma_{m}))^{m_{0}/2}e^{-a_{(1)}m_{0}(t-\gamma_{m})/2}\left( \|\tilde{\mathbf{X}}(t)\|^{m_{0}/2} + \|\mathbf{X}(t)\|^{m_{0}/2}\right). $$

  This gives

  $$ \begin{array}{@{}rcl@{}} &&{{}}\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})\tilde{\mathbf{X}}^{\prime}(t+\gamma_{m})-\mathbf{X}(t+\gamma_{m})\mathbf{X}^{\prime}(t+\gamma_{m})\big\|^{m_{0}/2} \leqslant (C_{0}(\gamma_{m}))^{m_{0}/2} e^{-a_{(1)}m_{0}2^{n-1}} \\ &&{{}}\quad{ } \quad{ }\quad{ } \times\left( \sup_{2^{n}\leqslant t\leqslant 2^{n+1}}\|\tilde{\mathbf{X}}(t+\gamma_{m})\|^{m_{0}/2}+\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}\|\mathbf{X}(t+\gamma_{m})\|^{m_{0}/2}\right). \end{array} $$

  (A.10)

  Then, since the processes $\{\mathbf {X}(t):t\geqslant 0\}$ and $\{\tilde {\mathbf {X}}(t):t\geqslant 0\}$ have continuous paths, we get

  $$ \begin{array}{@{}rcl@{}} &&{{}}\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})\tilde{\mathbf{X}}^{\prime}(t+\gamma_{m})-\mathbf{X}(t+\gamma_{m})\mathbf{X}^{\prime}(t+\gamma_{m})\big\|^{m_{0}/2} \leqslant (C_{0}(\gamma_{m}))^{m_{0}/2} e^{-a_{(1)}m_{0}2^{n-1}}\\ &&\times \left( \|\tilde{\mathbf{X}}(t_{n}+\gamma_{m})\|^{m_{0}/2}+\|\mathbf{X}(t_{n}^{*}+\gamma_{m})\|^{m_{0}/2}\right), \quad{ } \end{array} $$

  (A.11)

  $2^{n}\leqslant t_{n},t_{n}^{*}\leqslant 2^{n+1}$. Further, by Cauchy-Schwarz’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} &&{{}}\text{E}\left[(C_{0}(\gamma_{m}))^{m_{0}/2}(\|\tilde{\mathbf{X}}(t_{n}+\gamma_{m})\|^{m_{0}/2}+\|\mathbf{X}(t_{n}^{*}+\gamma_{m})\|^{m_{0}/2})\right] \leqslant\left[\text{E}\left( (C_{0}(\gamma_{m}))^{m_{0}}\right)\right]^{1/2}\\ &&{}\quad{ } \quad{ } \quad{ } \quad{ } \quad{ } \quad{ } \quad{ } \quad{ } \quad{ } \times \left\{\text{E}\left[(\|\tilde{\mathbf{X}}(t_{n}+\gamma_{m})\|^{m_{0}/2}+\|\mathbf{X}(t_{n}^{*}+\gamma_{m})\|^{m_{0}/2})^{2}\right]\right\}^{1/2}\\ && {{}}\quad{ } \leqslant2^{1/2}\left[\text{E}\left( (C_{0}(\gamma_{m}))^{m_{0}}\right)\right]^{1/2} \left\{\text{E}\left[\|\tilde{\mathbf{X}}(t_{n}+\gamma_{m})\|^{m_{0}}\right]+\text{E}\left[\|\mathbf{X}(t_{n}^{*}+\gamma_{m})\|^{m_{0}}\right]\right\}^{1/2}. \end{array} $$

  As established in the proof of Part (i), $\text {E}\left ((C_{0}(\gamma _{m}))^{m_{0}}\right )<\infty$. Further, from Propositions 2.4 and 2.4, $\displaystyle \sup \limits _{t\geqslant 0} \text {E}[\|\mathbf {X}(t)\|^{m_{0}}]<\infty$ and $\displaystyle \sup \limits _{t\geqslant 0} \text {E}[\|\tilde {\mathbf {X}}$ $(t)\|^{m_{0}}]<\infty$ and then, there exists K₂ > 0 such that

  $$ \begin{array}{@{}rcl@{}} &&{{}}\text{E}\left[(C_{0}(\gamma_{m}))^{m_{0}/2}(\|\tilde{\mathbf{X}}(t_{n}+\gamma_{m})\|^{m_{0}/2}+\|\mathbf{X}(t_{n}^{*}+\gamma_{m})\|^{m_{0}/2})\right] \leqslant 2^{1/2}\left[\text{E}\left( (C_{0}(\gamma_{m}))^{m_{0}}\right)\right]^{1/2}\\ &&{\kern98pt}\times\sup\limits_{t\geqslant 0}\{\left( \text{E}[\|\mathbf{X}(t)\|^{m_{0}}]+\text{E}[\|\tilde{\mathbf{X}}(t)\|^{m_{0}}]\right)^{\frac{1}{2}}\}\leqslant K_{2}<\infty. \end{array} $$

  Then

  $$ \begin{array}{@{}rcl@{}} &&{{}}\text{E}\left\{\displaystyle{\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})\tilde{\mathbf{X}}^{\prime}(t+\gamma_{m})\!-!\mathbf{X}(t+\gamma_{m})\mathbf{X}^{\prime}(t+\gamma_{m})\big\|^{m_{0}/2}\right\} \!\leqslant\! K_{0}^{*} K_{2}^{*} e^{-a_{(1)}m_{0}2^{n-1}}, \end{array} $$

  for some $K_{0}^{*}>0$, $K_{2}^{*}>0$, and then

  $$ \begin{array}{@{}rcl@{}} &&{{}}\displaystyle{\sum\limits_{n=1}^{\infty}}\text{E}\left\{\displaystyle{\sup_{2^{n}\leqslant t\leqslant 2^{n+1}}}\big\|\tilde{\mathbf{X}}(t+\gamma_{m})\tilde{\mathbf{X}}^{\prime}(t+\gamma_{m})-\mathbf{X}(t+\gamma_{m})\mathbf{X}^{\prime}(t+\gamma_{m})\big\|^{m_{0}/2}\right\}<\infty. \end{array} $$

  Therefore, together with the fact that $\left \{\tilde {\mathbf {X}}(t)\tilde {\mathbf {X}}^{\prime }(t)-\mathbf {X}(t)\mathbf {X}^{\prime }(t):t\geqslant 0\right \}$ is a process with continuous paths, Part (iv) follows from Borel-Cantelli’s lemma.

• (v) For $0\leqslant a<b\leqslant 1$, by using the triangle inequality along with Cauchy-Schwarz and Jensen’s inequalities, we have

  $$ \begin{array}{@{}rcl@{}} &&\left\|{\int}_{aT}^{bT}\tilde{\mathbf{X}}(t)\varphi^{\prime}(t)dt-{\int}_{aT}^{bT}\mathbf{X}(t)\varphi^{\prime}(t)dt\right\|^{m_{0}} \\&\leqslant& K_{\varphi}((b-a)T)^{m_{0}-1} {\int}_{aT}^{bT}\|\tilde{\mathbf{X}}(t)-\mathbf{X}(t)\|^{m_{0}}dt. \end{array} $$

  (A.12)

  Then, since 0 < (b − a)^m− 1 < 1 for all $0\leqslant a<b\leqslant 1$, we have

  $$ \begin{array}{@{}rcl@{}} &&\sup_{0\leqslant a<b\leqslant 1}\left\|\frac{1}{T}{\int}_{aT}^{bT}\tilde{\mathbf{X}}(t)\varphi^{\prime}(t)dt-\frac{1}{T}{\int}_{aT}^{bT}\mathbf{X}(t)\varphi^{\prime}(t)dt\right\|^{m_{0}}\\ &\leqslant& \frac{K_{\varphi}}{T} {{\int}_{0}^{T}}\|\tilde{\mathbf{X}}(t)-\mathbf{X}(t)\|^{m_{0}}dt, \end{array} $$

  (A.13)

  and then, by using Part (iii) along with the continuous version of Kronecker’s lemma,

  

  then, by combining this last relation with Eq. A.13, we get

  

  and then,

  

  this proves the statement in Part (v).

• (vi) For $0\leqslant a<b\leqslant 1$, by the triangle inequality, we have

  $$ \begin{array}{@{}rcl@{}} \left\|\frac{1}{T}{\int}_{aT}^{bT}\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)dt-\frac{1}{T}{\int}_{aT}^{bT}\mathbf{X}(t)\mathbf{X}^{\prime}(t)dt\right\|^{m_{0}/2}\\ \leqslant \frac{1}{T^{m_{0}/2}}\left( {\int}_{aT}^{bT}\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\|dt\right)^{m_{0}/2}. \end{array} $$

  Then, by Jensen’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} \left\|\frac{1}{T}{\int}_{aT}^{bT}\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)dt-\frac{1}{T}{\int}_{aT}^{bT}\mathbf{X}(t)\mathbf{X}^{\prime}(t)dt\right\|^{m_{0}/2}\\ \leqslant \frac{1}{T}(b-a)^{m_{0}/2-1}{\int}_{aT}^{bT}\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\|^{m_{0}/2}dt, \end{array} $$

  then, since $0< (b-a)^{m_{0}/2-1}<1$ for all $0\leqslant a<b\leqslant 1$ whenever $m_{0}\geqslant 2$, we get

  $$ \begin{array}{@{}rcl@{}} &&\displaystyle \sup_{0\leqslant a<b\leqslant 1}\left( \Bigg\|\frac{1}{T}{\int}_{aT}^{bT}\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)dt-\frac{1}{T}{\int}_{aT}^{bT}\mathbf{X}(t)\mathbf{X}^{\prime}(t)dt\Bigg\|^{m_{0}/2}\right)\\ &&{}\leqslant \frac{1}{T}{{\int}_{0}^{T}}\|\tilde{\mathbf{X}}(t)\tilde{\mathbf{X}}^{\prime}(t)-\mathbf{X}(t)\mathbf{X}^{\prime}(t)\|^{m_{0}/2}dt. \end{array} $$

  (A.14)

  Therefore, from Part (iv) along with the continuous version of Kronecker’s lemma, we get

  

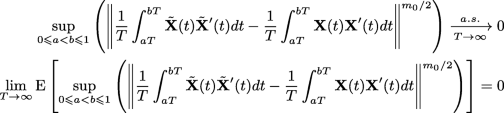
  and then,

  

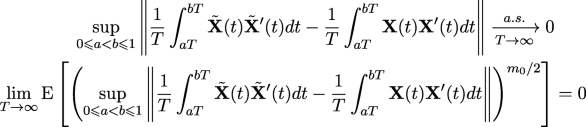
  this completes the proof.

□

Proof 16 (Proof of Lemma 4.1).

• (1). Let $\mathcal {C}_{T}(a, b)=\frac {1}{T}\displaystyle {{\int \limits }_{0}^{a T}}Y(t)dt-\frac {1}{T}\displaystyle {{\int \limits }_{0}^{b T}}Y(t)dt$. We have

  $$ \begin{array}{@{}rcl@{}} \left\|\frac{1}{T}\displaystyle{{\int}_{\hat{a}T}^{\hat{b} T}}Y(t)dt-\frac{1}{T}\displaystyle{{\int}_{a T}^{b T}}Y(t)dt\right\| \leqslant \|\mathcal{C}_{T}(\hat{b}, b)\|+\|\mathcal{C}_{T}(\hat{a}, a)\|. \end{array} $$

  (A.15)

  It suffices to prove that $\displaystyle \lim _{T\rightarrow \infty }\text {E}\left (\|\mathcal {C}_{T}(\hat {b}, b)\|\right )=0$. Let 𝜖 > 0, we have

  $$ \begin{array}{@{}rcl@{}} \lim_{T\rightarrow\infty}P\left( |\hat{b}-b|\geqslant \epsilon\right)=0. \end{array} $$

  (A.16)

  Further, one can verify that

  $$ \begin{array}{@{}rcl@{}} \|\mathcal{C}_{T}(\hat{b}, b)\|\leqslant \mathcal{I}_{11}(T)+\mathcal{I}_{12}(T)+\mathcal{I}_{21}(T)+\mathcal{I}_{22}(T) \end{array} $$

  (A.17)

  where

  $$ \begin{array}{@{}rcl@{}} &&{{}}\mathcal{I}_{11}(T)=\frac{1}{T}\displaystyle{{\int}_{bT}^{\hat{b} T}}\|Y(t)\|dt \mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{|\hat{b}-b|\geqslant \epsilon\right\}}, \mathcal{I}_{12}(T)=\frac{1}{T}\displaystyle{{\int}_{bT}^{\hat{b} T}}\|Y(t)\|dt \mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{|\hat{b}-b|< \epsilon\right\}}\\ &&{{}}\mathcal{I}_{21}(T)=\frac{1}{T}\displaystyle{{\int}_{bT}^{\hat{b} T}}\|Y(t)\|dt \mathbb{I}_{\{b\geqslant \hat{b}\}}\mathbb{I}_{\left\{|\hat{b}-b|\geqslant \epsilon\right\}}, \mathcal{I}_{22}(T)=\frac{1}{T}\displaystyle{{\int}_{bT}^{\hat{b} T}}\|Y(t)\|dt \mathbb{I}_{\{b\geqslant \hat{b}\}}\mathbb{I}_{\left\{|\hat{b}-b|< \epsilon\right\}}. \end{array} $$

  We have

  $$ \begin{array}{@{}rcl@{}} \text{ E}\left( \mathcal{I}_{11}(T)\right)\leqslant\frac{1}{T}\displaystyle{{\int}_{b T}^{T}}\text{E}\left[\|Y(t)\|\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right]dt. \end{array} $$

  (A.18)

  Then, by Hölder’s inequality

  $$ \begin{array}{@{}rcl@{}} &&\text{ E}\left( \mathcal{I}_{11}(T)\right)\leqslant\frac{1}{T}\displaystyle{{\int}_{b T}^{T}}\left[\text{E}\left( \|Y(t)\|^{p}\right)\right]^{1/p} \left[\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right)\right]^{1/q}dt\\ &&{}\leqslant \frac{1}{T}\left[\displaystyle{{\int}_{b T}^{T}}\text{E}\left( \|Y(t)\|^{p}\right)dt\right]^{1/p} \left[\displaystyle{{\int}_{b T}^{ T}}\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right)dt\right]^{1/q} \end{array} $$

  with 1/q = 1 − 1/p, and since $\left \{Y(t):t\geqslant 0\right \}$ is L^p-bounded, there exists K₀ > 0 such that

  $$ \begin{array}{@{}rcl@{}} \text{ E}\left( \mathcal{I}_{11}(T)\right)&\leqslant&\frac{K_{0}^{1/p}(1-b)^{1/p}T^{1/p}}{T}\left\{\text{E}\left[\displaystyle{{\int}_{b T}^{T}} \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}dt\right]\right\}^{1/q}\\ & & \leqslant\frac{K_{0}^{1/p}(1-b)^{1/p}T^{1/p}}{T}T^{1/q} \left[\text{E}\left( |\hat{b}-b|\right)\right]^{1/q} \end{array} $$

  this gives

  $$ \begin{array}{@{}rcl@{}} \text{ E}\left( \mathcal{I}_{11}(T)\right)\leqslant K_{0}^{1/p}(1-b)^{1/p}\left[\text{E}\left( |\hat{b}-b|\right)\right]^{1/q}, \end{array} $$

  (A.19)

  and then, by combining Eqs. A.16 and A.19 along with Lebesgue dominated convergence theorem, we get $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{11}(T)\right )=0$. Further, we have

  $$ \begin{array}{@{}rcl@{}} \text{E}\left( \mathcal{I}_{12}(T)\right)\leqslant \frac{1}{T}\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\text{E}\left[\|Y(t)\|\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right]dt. \end{array} $$

  Then, by Hölder’s inequality

  $$ \begin{array}{@{}rcl@{}} &&{}\text{ E}\left( \mathcal{I}_{12}(T)\right)\leqslant\frac{1}{T}\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\left[\text{E}\left( \|Y(t)\|^{p}\right)\right]^{1/p} \left[\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right)\right]^{1/q}dt\\ &&{}\leqslant \frac{1}{T}\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\text{E}\left( \|Y(t)\|^{p}\right)dt\right]^{1/p} \left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right)dt\right]^{1/q} \end{array} $$

  with 1/q = 1 − 1/p, and since $\left \{Y(t):t\geqslant 0\right \}$ is L^p-bounded, there exists K₀ > 0 such that

  $$ \begin{array}{@{}rcl@{}} {}\text{E}\left( \mathcal{I}_{12}(T)\right)&&\!\!\!\!\leqslant\frac{K_{0}^{1/p}\epsilon^{1/p}T^{1/p}}{T}\left\{\text{\!E\!}\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}} \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}dt\right]\right\}^{1/q}\\ &&\!\!\!\leqslant\frac{K_{0}^{1/p}\epsilon^{1/p}T^{1/p}}{T}T^{1/q} \left[\text{E}\left( |\hat{b}-b|\mathbb{I}_{\left\{|\hat{b}-b|<\epsilon\right\}}\right)\right]^{1/q}<K_{0}^{1/p}\epsilon, \end{array} $$

  this proves that $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{12}(T)\right )=0$. By using the similar techniques, one can prove that $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{21}(T)\right )=\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{22}(T)\right )=0$, this proves the first statement.

• (2). Obviously,

  

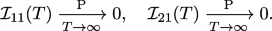  (A.20)

  Further, we have

  $$ \begin{array}{@{}rcl@{}} &&{}\text{E}\left( \mathcal{I}^{p}_{12}(T)\right)\leqslant \frac{1}{T^{p}}\text{E}\left\{\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\left( \displaystyle{\sup_{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)\right)\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}dt\right]^{p}\right\}\\ &&\leqslant \frac{1}{T^{p}}\text{E}\left\{\left( \displaystyle{\sup_{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)\right)^{p}\left( \displaystyle{{\int}_{b T}^{bT+\epsilon T}}\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}dt\right)^{p}\right\}\\ &&\leqslant \frac{1}{T^{p}}\text{E}\left\{\left( \displaystyle{\sup_{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)\right)^{p}T^{p}|\hat{b}-b|^{p}\mathbb{I}_{\left\{|\hat{b}- b|<\epsilon\right\}}\right\}\\&& \leqslant b^{p}\epsilon^{p}\text{E}\left\{\left( \displaystyle{\sup_{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)\right)^{p}\right\}, \end{array} $$

  and since the process $\left \{\|Y(t)\|:t\geqslant 0\right \}$ has continuous paths, there exists $t_{0}^{*}\in [bT, bT+T\epsilon ]$ such that $\displaystyle {\sup _{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)=\|Y(t_{0}^{*})\|$ then $\text {E}\left \{\left (\displaystyle {\sup _{bT\leqslant t \leqslant bT+\epsilon T}}(\|Y(t)\|)\right )^{p}\right \}=\text {E}\left (\|Y(t_{0}^{*}\|^{p})\right ) \leqslant \displaystyle \sup _{t\geqslant 0}\text {E}\left (\|Y(t)\|^{p}\right )$ $<\infty$. Therefore, $\displaystyle \text {E}\left (\mathcal {I}^{p}_{12}(T)\right ) \leqslant b^{p}\epsilon ^{p}\sup _{t\geqslant 0}\text {E}\left (\|Y(t)\|^{p}\right )$, this proves that $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}^{p}_{12}(T)\right )=0$ and then

  

  (A.21)

  In the similar way, one can prove that

  

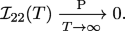  (A.22)

  Therefore, the proof of the second statement is completed by combining the relations Eqs. A.15, A.17, A.20, A.21 and A.22.

• (3). By Jensen’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} \mathcal{I}_{11}^{p/2}(T)\leqslant\frac{1}{T}\displaystyle{{\int}_{0}^{T}}\left[\|Y(t)\|^{p/2}\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right]dt. \end{array} $$

  (A.23)

  Then, by Cauchy-Schwarz’s inequality

  $$ \begin{array}{@{}rcl@{}} {}\text{ E}\left( \mathcal{I}_{11}^{p/2}(T)\right)&&{}\leqslant\frac{1}{T}\displaystyle{{\int}_{0}^{T}}\left[\text{E}\left( \|Y(t)\|^{p}\right)\right]^{1/2} \left[\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right)\right]^{1/2}dt\\ &&{}\leqslant \frac{1}{T}\left[\displaystyle{{\int}_{0}^{T}}\text{E}\left( \|Y(t)\|^{p}\right)dt\right]^{1/2} \left[\displaystyle{{\int}_{0}^{ T}}\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}\right)dt\right]^{1/2} \end{array} $$

  and since $\left \{Y(t):t\geqslant 0\right \}$ is L^p-bounded, there exists K₀ > 0 such that

  $$ \begin{array}{@{}rcl@{}} \text{ E}\left( \mathcal{I}_{11}^{p/2}(T)\right) &&\!\!\leqslant \frac{K_{0}^{1/p}T^{1/2}}{T}\left\{\text{E}\left[\displaystyle{{\int}_{0}^{T}} \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{\hat{b}\geqslant b+\epsilon\right\}}dt\right]\right\}^{1/2} \\ &&\!\!\leqslant\frac{K_{0}^{1/p}T^{1/2}}{T}T^{1/2} \left[\text{E}\left( |\hat{b}-b|\right)\right]^{1/2}, \end{array} $$

  and then, together with Lebesgue dominated convergence theorem, we get $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{11}^{p/2}(T)\right )=0$. Further, by Jensen’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left( \mathcal{I}_{12}^{p/2}(T)\right)\leqslant \frac{(\epsilon T)^{p/2}}{T^{p/2}}\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\frac{1}{\epsilon T}\text{E}\left[\|Y(t)\|^{p/2}\mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right]dt. \end{array} $$

  Then, together with Cauchy-Shwarz’s inequality, we have

  $$ \begin{array}{@{}rcl@{}} \!\!\!\!\!\!\!\!\!\!\!\!\!\!\text{ E}\left( \mathcal{I}_{12}^{p/2}(T)\right)\!\!\!\!\!&\leqslant&\!\!\!\!\!\frac{\epsilon^{p/2-1} }{T}\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\left[\text{E}\left( \|Y(t)\|^{p}\right)\right]^{1/2} \left[\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right)\right]^{1/2}dt\\ \!\!\!\!\!&\leqslant&\!\!\!\!\! \frac{\epsilon^{p/2-1}}{T}\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\text{E}\left( \|Y(t)\|^{p}\right)dt\right]^{1/2}\\&& {\kern22pt}\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}}\text{E}\left( \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}\right)dt\right]^{1/2}, \end{array} $$

  and since $\left \{Y(t):t\geqslant 0\right \}$ is L^p-bounded, we have

  $$ \begin{array}{@{}rcl@{}} &&{}\text{ E}\left( \mathcal{I}_{12}^{p/2}(T)\right)\leqslant\frac{K_{0}^{1/2}\epsilon^{p/2-1/2}T^{1/2}}{T}\left\{\text{E}\left[\displaystyle{{\int}_{b T}^{bT+\epsilon T}} \mathbb{I}_{\{bT<t<\hat{b}T\}}\mathbb{I}_{\{b<\hat{b}\}}\mathbb{I}_{\left\{b<\hat{b}< b+\epsilon\right\}}dt\right]\right\}^{1/2}dt\\ &&{}\leqslant\frac{K_{0}^{1/p}\epsilon^{(p-1)/2}T^{1/2}}{T}T^{1/2} \left[\text{E}\left( |\hat{b}-b|\mathbb{I}_{\left\{|\hat{b}-b|<\epsilon\right\}}\right)\right]^{1/2}<K_{0}^{1/p}\epsilon^{(p-1)/2} \left[\text{E}\left( |\hat{b}-b|\right)\right]^{1/2}. \end{array} $$

  Hence, by Lebesgue-dominated convergence theorem, we get $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}$ $\left (\mathcal {I}_{12}(T)\right )=0$. By using the similar techniques, one can prove that $\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{21}^{p/2}(T)\right )=\displaystyle {\lim _{T\rightarrow \infty }}\text { E}\left (\mathcal {I}_{22}^{p/2}(T)\right )=0,$ this proves the third statement.

□

Proof 17 (Proof of Lemma 4.2).

We have

$$ \begin{array}{@{}rcl@{}} \frac{1}{\sqrt{T}}\left( {\int}_{\hat{a}T}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{aT}^{bT}Y_{t}dW_{t}\right) =\frac{1}{\sqrt{T}}\left( {\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right) \\ -\frac{1}{\sqrt{T}}\left( {\int}_{0}^{\hat{a}T}Y_{t}dW_{t}-{\int}_{0}^{aT}Y_{t}dW_{t}\right). \end{array} $$

Then, it suffices to prove that . Let 𝜖 > 0, we have $\displaystyle \lim _{T\rightarrow \infty }\text {P}\left (|\hat {b}-b|\geqslant \epsilon \right )=0$. Further, let

$$ \begin{array}{@{}rcl@{}} \displaystyle \mathrm{I}_{11}(p,T)&=&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{\hat{b}>b\right\}} \mathbb{I}_{\left\{|\hat{b}-b|<\epsilon\right\}}\right],\\ \displaystyle \mathrm{I}_{12}(p,T)&=&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p/2} \mathbb{I}_{\left\{\hat{b}> b\right\}}\mathbb{I}_{\left\{|\hat{b}-b|\geqslant\epsilon\right\}}\right],\\ \displaystyle \mathrm{I}_{21}(p,T)&=&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p/2} \mathbb{I}_{\left\{\hat{b}\leqslant b\right\}} \mathbb{I}_{\left\{|\hat{b}-b|<\epsilon\right\}}\right],\\ \displaystyle \mathrm{I}_{22}(p,T)&=&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{\hat{b}\leqslant b\right\}}\mathbb{I}_{\left\{|\hat{b}-b|\geqslant\epsilon\right\}}\right].\\ \end{array} $$

(A.24)

One can verify that

$$ \frac{1}{T^{p/2}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p/2}\right]=\mathrm{I}_{11}(p,T)+\mathrm{I}_{12}(p,T) +\mathrm{I}_{21}(p,T) +\mathrm{I}_{22}(p,T). $$

(A.25)

Hence, the proof is completed if we prove that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{11}(p,T)=\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{12}$ $(p,T)=\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{21}(p,T) =\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{22}(p,T)=0$. To this end, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T)=\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{b T}^{\hat{b}T}Y_{t}dW_{t}\right\|^{p/4}\mathbb{I}_{\left\{\hat{b}>b\right\}}\mathbb{I}_{\left\{|\hat{b}-b|<\epsilon\right\}}\right] =\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{bT}^{\hat{b}T}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{0<\hat{b}-b< \epsilon\right\}}\right], \end{array} $$

then

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T)=\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{bT}^{\hat{b}T}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{bT<T\hat{b}< bT+ \epsilon T\right\}}\right]. \quad{ } \end{array} $$

(A.26)

From Eq. A.26, we get $\mathrm {I}_{11}(p,T) \leqslant \displaystyle \frac {1}{T^{p/4}}\mathrm {E}\left [\left (\sup _{bT\leqslant t\leqslant bT+\epsilon T}\left \|{\int \limits }_{bT}^{t}Y_{u}dW_{u}\right \|\right )^{p/2}\right .$ $\left .\mathbb {I}_{\left \{b T<T\hat {b}\leqslant bT+ \epsilon T\right \}}\right ]$, then, by Cauchy-Schwartz’s inequality, we get

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T) \leqslant\frac{1}{T^{p/4}}\mathrm{E}^{1/2}\left[\left( \sup_{bT\leqslant t\leqslant bT+\epsilon T}\left\|{\int}_{bT}^{t}Y_{u}dW_{u}\right\|\right)^{p}\right]\text{E}^{1/2}\left[\mathbb{I}_{\left\{0<|\hat{b}-b|\leqslant \epsilon \right\}}\right].\!\!\!\\ \end{array} $$

(A.27)

Note that, since $\left \{Y_{u}: u\geqslant 0\right \}$ is a L^p-bounded process, the process $\left \{\displaystyle {\int \limits }_{Tb}^{t}\right .$ $\left .Y_{u}dW_{u}: t\geqslant Tb\right \}$ is a martingale with respect to the natural filtration generated by $\left \{W_{t}:t\geqslant Tb\right \}$. Then, by combining Eq. A.27 with Burkholder-Davis-Gundy’s inequality, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T) \leqslant \displaystyle c_{p}\frac{1}{T^{p/4}}\mathrm{E}^{1/2}\left\{\left[{\int}_{bT}^{Tb+\epsilon T}\left\|Y_{u}\right\|^{2}du\right]^{p/2}\right\}. \end{array} $$

(A.28)

for some c_p > 0. Then, by Jensen’s inequality

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T) \leqslant \displaystyle c_{p}\frac{1}{T^{p/4}}(Tb+\epsilon T-bT)^{p/4-1/2}\mathrm{E}^{1/2}\left\{\left[{\int}_{bT}^{Tb+\epsilon T}\left\|Y_{u}\right\|^{p}du\right]\right\}. \end{array} $$

(A.29)

Since $\left \{Y_{u}: u\geqslant 0\right \}$ is a L^p-bounded process, there exits K₃ > 0 such that $\text {E}\left (\|Y_{t}\|^{p}\right )\leqslant K_{3},$ for all $t\geqslant 0$. Then, together with Eq. A.29

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(p,T) \leqslant \displaystyle c_{p}\frac{1}{T^{p/4}}\left( Tb+\epsilon T-bT\right)^{p/4-1/2}\left[{\int}_{bT}^{Tb+\epsilon T}K_{3}du\right]^{1/2} =c_{p}b^{p/4}\epsilon^{p/4},\\ \end{array} $$

(A.30)

for all 𝜖 > 0. This proves that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{11}(p,T)=0$. Further, since 0 < b < 1, $0<\hat {b}\leqslant 1$ a.s., we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{12}(p,T)&=&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{b T}^{\hat{b}T}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{1\geqslant \hat{b}>b>0\right\}}\mathbb{I}_{\left\{|\hat{b}-b|\geqslant\epsilon\right\}}\right]\\ & =&\frac{1}{T^{p/4}}\mathrm{E}\left[\left\|{\int}_{bT}^{\hat{b}T}Y_{t}dW_{t}\right\|^{p/2}\mathbb{I}_{\left\{(\hat{b}-b)> \epsilon\right\}}\mathbb{I}_{\left\{T\geqslant T(\hat{b}-b)> 0\right\}}\right]. \end{array} $$

Then, together with Cauchy-Schwarz’s inequality, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{12}(p,T) \leqslant\frac{1}{T^{p/4}}\left\{\mathrm{E}\left[\left\|{\int}_{bT}^{\hat{b}T}Y_{u}dW_{u}\right\|^{p}\mathbb{I}_{\left\{T\geqslant T(\hat{b}-b)> 0\right\}}\right]\right\}^{1/2}\left\{\mathrm{E}\left[\mathbb{I}_{\left\{(\hat{b}-b)> \epsilon\right\}}\right]\right\}^{1/2}. \end{array} $$

Hence, $\mathrm {I}_{12}(p,T) \leqslant \displaystyle \frac {1}{T^{p/4}}\left \{\mathrm {E}\left [\left [\sup _{0\leqslant t\leqslant T}\left \|{{\int \limits }_{0}^{t}}Y_{u}dW_{u}\right \|\right ]^{p}\mathbb {I}_{\left \{T\geqslant T(\hat {b}-b)> 0\right \}}\right ]\right \}^{1/2}$ $\left \{\!\mathrm {P}\!\!\left [\hat {b} - b\!>\! \epsilon \right ]\!\right \}^{1/2}$, and this gives, $\mathrm {I}_{12}(p,T)\! \!\leqslant \!\displaystyle \!\frac {1}{T^{p/4}}\left \{\!\mathrm {E}\!\left [\!\left [\sup _{0\leqslant t\leqslant T}\left \|{{\int \limits }_{0}^{t}}\!Y_{u}dW_{u}\right \|\right ]^{p}\right ]\!\right \}^{1/2}$ $\left \{\mathrm {P}\left [\hat {b}-b> \epsilon \right ]\right \}^{1/2}$. Then, by Burkholder-Davis-Gundy’s inequality, I₁₂(p,T) $\leqslant \displaystyle \frac {C_{p}}{T^{p/4}}\left \{\mathrm {E}\left [\left [{\int \limits }_{0}^{T}\|Y_{u}\|^{2}du\right ]^{p/2}\right ]\right \}^{1/2} \left \{\mathrm {P}\left [\hat {b}\!\!-b\!>\! \epsilon \right ]\right \}^{1/2}$, where C_p is a strictly positive real number. Then, by Jensen’s inequality, we get

$\mathrm {I}_{12}(p,T) \leqslant \displaystyle \frac {C_{p}}{T^{p/4}}T^{p/4-1/2}\left [{\int \limits }_{0}^{T}K_{3}du\right ]^{1/2} \left \{\mathrm {P}\left [\hat {b}-b> \epsilon \right ]\right \}^{1/2}$. Then,

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{12}(p,T) \leqslant C_{p}K_{3}^{1/2} \left\{\mathrm{P}\left[\hat{b}-b> \epsilon\right]\right\}^{1/2}, \end{array} $$

this proves that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{12}(p,T)=0$. By using the similar techniques as for I₁₁(p,T) and I₁₂(p,T), we prove that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{21}(p,T)=\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{22}(p,T)=0$, this completes the proof. □

Proof 18 (Proof of Lemma 4.3).

Let I₁₁(2p,T), I₁₂(2p,T), I₂₁(2p,T) and I₂₂(2p, T) be as defined in Eq. A.24 by replacing p by 2p. As in Eq. A.25, we have

$$ \frac{1}{T^{p/2}}\mathrm{E}\left[\left\|{\int}_{0}^{\hat{b}T}Y_{t}dW_{t}-{\int}_{0}^{bT}Y_{t}dW_{t}\right\|^{p}\right]=\mathrm{I}_{11}(2p,T)+\mathrm{I}_{12}(2p,T) +\mathrm{I}_{21}(2p,T) +\mathrm{I}_{22}(2p,T). $$

Then, it suffices to show that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{11}(2p,T)=\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{12}(2p,T)=\displaystyle \lim _{T\rightarrow \infty }$ $\mathrm {I}_{21}(2p,T) = \displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{22}(2p,T) = 0$. We have $\mathrm {I}_{11}(2p,T)=\displaystyle \frac {1}{T^{p/2}}\mathrm {E}\left [\left \|{\int \limits }_{b T}^{\hat {b}T} Y_tdW_t\right .\right \|$ $\left .{~}^{p}\mathbb {I}_{\left \{\hat {b}>b\right \}}\mathbb {I}_{\left \{|\hat {b}-b|<\epsilon \right \}}\right ] =\frac {1}{T^{p/2}}\mathrm {E}\left [\left \|{\int \limits }_{bT}^{\hat {b}T}Y_tdW_t\right \|^{p}\mathbb {I}_{\left \{0<\hat {b}-b\leqslant \epsilon \right \}}\right ]$, then, as in proof of Lemma 4.2, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(2p,T)&\leqslant &\frac{1}{T^{p/2}}\mathrm{E}\left[\left( \sup_{bT\leqslant t\leqslant bT+\epsilon T}\left\|{\int}_{bT}^{t}Y_{u}dW_{u}\right\|\right)^{p}\mathbb{I}_{\left\{b T<T\hat{b}\leqslant bT+ \epsilon T\right\}}\right], \end{array} $$

then, from Cauchy-Shwartz’s inequality, we get

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(2p,T) &\!\!\leqslant&\frac{1}{T^{p/2}}\mathrm{E}^{1/2}\left[\left( \sup_{bT\leqslant t\leqslant bT+\epsilon T}\left\|{\int}_{bT}^{t}Y_{u}dW_{u}\right\|\right)^{2p}\right]\text{E}^{1/2}\left[\mathbb{I}_{\left\{0<|\hat{b}-b|\leqslant \epsilon \right\}}\right]. \end{array} $$

(A.31)

Then, by combining Eq. A.31 with by Burkholder-Davis-Gundy’s inequality, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(2p,T) \leqslant \displaystyle c_{p}\frac{1}{T^{p/2}}\mathrm{E}^{1/2}\left\{\left[{\int}_{bT}^{Tb+\epsilon T}\left\|Y_{u}\right\|^{2}du\right]^{p}\right\}. \end{array} $$

(A.32)

for some c_p > 0. Then, since for some K_3∗ > 0, $\|Y_{t}\|^{2}\leqslant K_{3*}$ for all $t\geqslant 0$, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{I}_{11}(2p,T) \leqslant \displaystyle c_{p}\frac{1}{T^{p/2}}\left[{\int}_{bT}^{Tb+\epsilon T}K_{3*}du\right]^{p/2} =c_{p}b^{p/2}\epsilon^{p/2}, \end{array} $$

(A.33)

for all 𝜖 > 0. This proves that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{11}(2p,T)=0$. Further, as in the proof of Lemma 4.2, we prove that $\mathrm {I}_{12}(2p,T) \!\leqslant \!\displaystyle \frac {1}{T^{p/2}}\left \{\mathrm {E}\!\left [\left [\sup _{0\leqslant t\leqslant T}\left \|{{\int \limits }_{0}^{t}}Y_{u}dW_{u}\right \|\right ]^{2p}\right ]\!\right \}^{1/2}$ $\left \{\mathrm {P}\left [\hat {b}-b> \epsilon \right ]\right \}^{1/2}$. Then, by Burkholder-Davis-Gundy’s inequality, I₁₂(2p, $T) \leqslant \displaystyle \frac {C_{p}}{T^{p/2}}\left \{\mathrm {E}\left [\left [{\int \limits }_{0}^{T}\|Y_{u}\|^{2}du\right ]^{p}\right ]\right \}^{1/2} \left \{\mathrm {P}\left [\hat {b}-b> \epsilon \right ]\right \}^{1/2}$, where C_p is a strictly positive real number. Then, $\|Y_{t}\|^{2}\leqslant K_{3*}$ for all $t\geqslant 0$, we get $\mathrm {I}_{12}(2p,T) \leqslant C_{p}K_{3*}^{1/2} \left \{\mathrm {P}\left [\hat {b}-b> \epsilon \right ]\right \}^{1/2}$, this proves that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{12}(2p,T)=0$. Similarly, one can verify that $\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{21}(2p,T)=\displaystyle \lim _{T\rightarrow \infty }\mathrm {I}_{22}(2p,T)=0$, this completes the proof. □

Appendix B: Additional Proofs and Details

Proposition B.1.

Let A be a d × d-positive definite matrix A, let λ₁(A) be the smallest eigenvalue of $A^{\prime }+A$, let λ_d(A) be the largest eigenvalue of $A^{\prime }+A$ and let p > 0. Then, ${d^{p/2}e^{-tp\lambda _{d}(A)/2}}\leqslant \|e^{-At}\|^{p} \leqslant {d^{p/2}e^{-tp\lambda _1(A)/2}}$, for all $t\geqslant 0$. Further, $\displaystyle {\lim _{t \to \infty }} e^{-At} = 0.$

The proof follows from algebraic computations.

Proof 19 (Proof of Proposition 2.4).

• Let $\mathcal {I}_{t}^{*}=\displaystyle e^{-A_{j}t}{{\int \limits }_{0}^{t}}e^{A_{j}s}{\Sigma }^{1/2}d{W}_s$, $t\geqslant 0$. By Burkholder-Davis-Gundy’s inequality, there exists $c_{m_{0}}>0$ such that

  $$ \begin{array}{@{}rcl@{}} & &\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \leqslant c_{m_{0}} \left[\left( {\displaystyle{\int}_{0}^{t}}\|e^{-A_{j}(t-s)}{\Sigma}^{1/2}\|^{2}ds\right)^{\frac{m_{0}}{2}} \right], \end{array} $$

  then,

  $$ \begin{array}{@{}rcl@{}} {~}\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] &\leqslant& c_{m_{0}}(\lambda_{\max}({\Sigma}))^{m_{0}/2}d^{m_{0}/2}\left[\text{trace}{\displaystyle{\int}_{0}^{t}} e^{-(A_{j}+A^{\prime}_{j})(t-s)}ds\right]^{m_{0}/2} \end{array} $$

  and then

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \leqslant c_{m_{0}}(\lambda_{\max}({\Sigma}))^{m_{0}/2}d^{m_{0}/2}\left[\text{trace}\left[\left( A_{j}+A^{\prime}_{j}\right)^{-1} \left( I_{d}-e^{-(A_{j}+A^{\prime}_{j})t}\right)\right]\right]^{m_{0}/2}. \end{array} $$

  This gives

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \!\!\!&\leqslant&\!\!\! c_{m_{0}}(\lambda_{\max}({\Sigma}))^{m_{0}/2}d^{m_{0}/2}\left[\text{trace}\left( \left( A_{j}+A^{\prime}_{j}\right)^{-1}\right)\right]^{m_{0}/2}\\&& \left[\text{trace}\left[I_{d}-e^{-(A_{j}+A^{\prime}_{j})t}\right]\right]^{m_{0}/2}, \end{array} $$

  then

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \leqslant c_{m_{0}}(\lambda_{\max}({\Sigma}))^{m_{0}/2}d^{m_{0}/2}\left[\text{trace}\left( \left( A_{j}+A^{\prime}_{j}\right)^{-1}\right)\right]^{m_{0}/2} \left[d-de^{-\lambda_{d}(A_{j})t}\right]^{m_{0}/2}. \end{array} $$

  Hence,

  $$ \begin{array}{@{}rcl@{}} {{}}\displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \leqslant c_{m_{0}}(\lambda_{\max}({\Sigma}))^{m_{0}/2}d^{m_{0}}\left[\text{trace}\left( \left( A_{j}+A^{\prime}_{j}\right)^{-1}\right)\right]^{m_{0}/2} \left[1-e^{-\lambda_{d}(A_{j})t}\right]^{m_{0}/2}. \end{array} $$

  This gives

  $$ \displaystyle\text{E}\left[\left\|\mathcal{I}_{t}^{*}\right\|^{m_{0}}\right] \leqslant\gamma_{1}(m_{0}) \left[\text{trace}\left( (A_{j}+A^{\prime}_{j})^{-1}\right)\right]^{m_{0}/2}\left( 1-e^{-\lambda_{d}(A_{j})t}\right)^{m_{0}/2}, $$

  (B.1)

  this proves the statement in Part (1).

• (2). We have $\mathbf {X}(\gamma _{k})=e^{-A_{k}(\gamma _{k}-\gamma _{k-1})}\mathbf {X}(\gamma _{k-1})+h_{k}^{(\gamma _{k-1})}(\gamma _{k}-\gamma _{k-1}) +\mathbf {Z}_{k}^{(\gamma _{k-1})}$ (γ_k − γ_k− 1). Then,

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left[\|\mathbf{X}(\gamma_{k}) \|^{m_{0}}\right]&\leqslant& 3^{m_{0}-1}\left\{e^{-m_{0}A_{k}(\gamma_{k}-\gamma_{k-1})}\text{E}\left( \|\mathbf{X}(\gamma_{k-1})\|^{m_{0}}\right) +\left\|h_{k}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\right\|^{m_{0}}\right\}\\ &&+3^{m_{0}-1}\text{E}\left( \|\mathbf{Z}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\|^{m_{0}}\right). \end{array} $$

  (B.2)

  To simplify the second term in the right hand side of Eq. B.2, note that

  $$ \begin{array}{@{}rcl@{}} {{}}\left\|h_{k}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\right\|^{m_{0}}\leqslant\left( {\int}_{0}^{\gamma_{k}-\gamma_{k-1}} \|e^{-A_{k}(\gamma_{k}-\gamma_{k-1}-s)}\|\|\mu_{k}\mathbf{\varphi}(\gamma_{k-1}+s)\| ds\right)^{m_{0}}, \end{array} $$

  then, by combining Assumption 2 and Proposition B.1, we get

  $$ \begin{array}{@{}rcl@{}} {{}}\left\|h_{k}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\right\|^{m_{0}}\leqslant K_{2}^{m_{0}}d^{m_{0}}\left( {\int}_{0}^{\gamma_{k}-\gamma_{k-1}} \|e^{-\lambda_{1}(A_{k})(\gamma_{k}-\gamma_{k-1}-s)/2}\| ds\right)^{m_{0}}. \end{array} $$

  This gives

  $$ \begin{array}{@{}rcl@{}} {~}\left\|h_{k}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\right\|^{m_{0}}&\leqslant& K_{2}^{m_{0}}d^{m_{0}/2}\left( \frac{2}{\lambda_{1}(A_{k})}\right)^{m_{0}} \\&=&(2K_{2}d^{1/2})^{m_{0}}\left( \lambda_{\max}\left( A_{k}^{-1}\right)\right)^{m_{0}}. \end{array} $$

  (B.3)

  For the third term in the hand side of Eq. B.2 , by Burkholder-Davis-Gundy’s inequality,

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left( \|\mathbf{Z}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\|^{m_{0}}\right) \leqslant c_{m_{0}}\left( {\int}_{0}^{\gamma_{k}-\gamma_{k-1}}\left\|e^{-A_{k}(\gamma_{k}-\gamma_{k-1}-s)}\mathbf{\Sigma}^{1/2}\right\|^{2} ds\right)^{m_{0}/2}, \end{array} $$

  then, by using Proposition B.1, we get

  $$ \begin{array}{@{}rcl@{}} \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\text{E}\left( \|\mathbf{Z}^{(\gamma_{k-1})}(\gamma_{k}-\gamma_{k-1})\|^{m_{0}}\right) \!\!\!&\leqslant&\!\!\! c_{m_{0}}\frac{d^{m_{0}/2}\|\mathbf{\Sigma}^{1/2}\|^{m_{0}}}{(\lambda_{1}(A_{k}))^{m_{0}/2}}\\\!\!\!&=&\!\!\! c_{m_{0}}d^{m_{0}/2}\|\mathbf{\Sigma}^{1/2}\|^{m_{0}}(\lambda_{\max}(A_{k}^{-1}))^{m_{0}/2}. \end{array} $$

  (B.4)

  Then, by combining relations Eqs. B.2, B.3 and B.4, we get

  $$ \begin{array}{@{}rcl@{}} &&{}\text{E}\left[\|\mathbf{X}(\gamma_{k}) \|^{m_{0}}\right]\!\leqslant\! 3^{m_{0}-1} \left\{\text{E}\left( \|\mathbf{X}(\gamma_{k-1})\|^{m_{0}}\right) +(2K_{2}d^{1/2})^{m_{0}}\left( \lambda_{\max}\left( A_{k}^{-1}\right)\right)^{m_{0}}\right\}\\ &&{\kern65pt}+3^{m_{0}-1}c_{m_{0}}d^{m_{0}/2}\|\mathbf{\Sigma}^{1/2}\|^{m_{0}}(\lambda_{\max}(A_{k}^{-1}))^{m_{0}/2}. \end{array} $$

  This gives

  $$ \begin{array}{@{}rcl@{}} {~}\text{E}\left[\|\mathbf{X}(\gamma_{k}) \|^{m_{0}}\right]\leqslant 3^{m_{0}-1} \text{E}\left( \|\mathbf{X}(\gamma_{k-1})\|^{m_{0}}\right) &+&3^{m_{0}-1}d^{m_{0}/2}\left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}}\\ &&\times \left( \left( \lambda_{\max}\left( A_{k}^{-1}\right)\right)^{m_{0}} +(\lambda_{\max}(A_{k}^{-1}))^{m_{0}/2}\right), \end{array} $$

  for $k=1,2,\dots$. Then, by using induction, we get

  $$ \begin{array}{@{}rcl@{}} {{}}\text{E}\left[\|\mathbf{X}(\gamma_{k}) \|^{m_{0}}\right]\leqslant 3^{(m_{0}-1)k}\text{E}\left( \|\mathbf{X}(0)\|^{m_{0}}\right) + d^{m_{0}/2} \left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}}\\ \times {\sum}_{i=0}^{k-1}3^{(m_{0}-1)(i+1)}\left( \left( \lambda_{\max}\left( A_{k-i}^{-1}\right)\right)^{m_{0}} +(\lambda_{\max}(A_{k-i}^{-1}))^{m_{0}/2}\right), \end{array} $$

  for $k=1,2,\dots ,m+1$, this proves the statement in Part (2).

• (3). Further, for $k=1,2,\dots ,m+1$, $t\geqslant \gamma _{k-1}$, we have

  $$ \begin{array}{@{}rcl@{}} &&{}\mathrm{E}[\|\mathbf{X}_{k}(t)\|^{m_{0}}] \leqslant3^{m_{0}-1} \|e^{-A_{k}(t-\gamma_{k-1})}\|^{m_{0}}\mathrm{E}(\|\mathbf{X}(\gamma_{k-1})\|^{m_{0}})\\ &&{\kern34pt}+3^{m_{0}-1}\left\|{\int}_{0}^{t-\gamma_{k-1}}e^{-A_{k}(t-\gamma_{k-1}-s)}\mu_{k}\varphi(s+\gamma_{k-1}) ds\right\|^{m_{0}}\\ &&{\kern34pt}+3^{m_{0}-1}\left[\mathrm{E}\left( \left\|\mathbf{Z}_{k}^{(\gamma_{k-1})}(t-\gamma_{k-1})\right\|^{m_{0}}\right)\right]. \end{array} $$

  Then, by using the similar techniques as in Part (2), we get

  $$ \begin{array}{@{}rcl@{}} &&{}\mathrm{E}[\|\mathbf{X}_{k}(t)\|^{m_{0}}] \leqslant3^{m_{0}-1} \mathrm{E}(\|\mathbf{X}(\gamma_{k-1})\|^{m_{0}})\\ &&{\kern12pt}+3^{m_{0}-1}d^{m_{0}/2}(2K_{2})^{m_{0}}\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}} +3^{m_{0}-1}c_{m_{0}}d^{m_{0}/2}\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}/2}. \end{array} $$

  for all $t\geqslant \gamma _{k-1}$, $k=1,2,\dots ,m+1$. Therefore,

  $$ \begin{array}{@{}rcl@{}} &&{}\displaystyle{\sup_{t\geqslant 0}}\ \mathrm{E}(\|\mathbf{X}(t)\|^{m_{0}})\leqslant\displaystyle\sum\limits_{k=1}^{m+1}\displaystyle{\sup_{t\geqslant 0}}\left( \mathrm{E}(\|\mathbf{X}_{k}(t)\|^{m_{0}})\mathbb{I}_{\left\{\gamma_{k-1}\leqslant t< \gamma_{k}\right\}}\right) \leqslant\displaystyle\sum\limits_{k=1}^{m+1}3^{m_{0}-1} \mathrm{E}(\|\mathbf{X}(\gamma_{k-1})\|^{m_{0}})\\ {\kern25pt}&&+3^{m_{0}-1}d^{m_{0}/2}\max\left( 2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|\right)^{m_{0}}\\&&\sum\limits_{k=1}^{m+1}\left[\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}} +\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}/2}\right], \end{array} $$

  and then, using Part (2), we get

  $$ \begin{array}{@{}rcl@{}} &&{}\displaystyle{\sup_{t\geqslant 0}}\ \mathrm{E}(\|\mathbf{X}(t)\|^{m_{0}})\leqslant 3^{m_{0}-1}\mathrm{E}(\|\mathbf{X}(0)\|^{m_{0}})+ 3^{m_{0}-1}d^{m_{0}/2}\left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}} \\ &&{\kern20pt}\times \left( \left( \lambda_{\max}\left( A_{1}^{-1}\right)\right)^{m_{0}} + (\lambda_{\max}(A_{1}^{-1}))^{m_{0}/2}\right) + 3^{m_{0}-1}\!\displaystyle\sum\limits_{k=2}^{m+1} 3^{(m_{0}-1)(k-1)}\text{E}\left( \|\mathbf{X}(0)\|^{m_{0}}\right)\\ &&{\kern20pt}+d^{m_{0}/2} \left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}} \sum\limits_{k=2}^{m+1}\sum\limits_{i=0}^{k-2}3^{(m_{0}-1)(i+1)}\left( \left( \lambda_{\max}\left( A_{k-1-i}^{-1}\right)\right)^{m_{0}}\right.\\ &&\left.{\kern20pt}+(\lambda_{\max}(A_{k-1-i}^{-1}))^{m_{0}/2}\right)\\ &&{\kern20pt}+3^{m_{0}-1}d^{m_{0}/2}\left( \max\left( 2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|\right)\right)^{m_{0}} \\&&{\kern20pt}\times\sum\limits_{k=1}^{m+1}\left[\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}} +\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}/2}\right]. \end{array} $$

  Therefore,

  $$ \begin{array}{@{}rcl@{}} &&{}\displaystyle{\sup_{t\geqslant 0}}\ \mathrm{E}(\|\mathbf{X}(t)\|^{m_{0}})\leqslant 3^{m_{0}-1}\mathrm{E}(\|\mathbf{X}(0)\|^{m_{0}})+ 3^{m_{0}-1}3^{m_{0}-1}\frac{3^{m(m_{0}-1)}-1}{3^{m_{0}-1}-1}\text{E}\left( \|\mathbf{X}(0)\|^{m_{0}}\right)\\ &&{\kern14pt}+3^{m_{0}-1}d^{m_{0}/2}\!\left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)\!^{m_{0}}\!\! \left( \left( \lambda_{\max}\left( A_{1}^{-1}\right)\right)^{m_{0}} + (\lambda_{\max}(A_{1}^{-1}))^{m_{0}/2}\right) \\ &&{\kern14pt}+d^{m_{0}/2} \left( \max(2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|)\right)^{m_{0}} \sum\limits_{k=2}^{m+1}\sum\limits_{i=0}^{k-2}3^{(m_{0}-1)(i+1)}\left( \left( \lambda_{\max}\left( A_{k-1-i}^{-1}\right)\right)^{m_{0}} \right.\\ &&{\kern14pt}+\left.(\lambda_{\max}(A_{k-1-i}^{-1}))^{m_{0}/2}\right) +3^{m_{0}-1}d^{m_{0}/2}\left( \max\left( 2K_{2},c_{m_{0}}\|\mathbf{\Sigma}^{1/2}\|\right)\right)^{m_{0}} \\&&{\kern14pt}\times\sum\limits_{k=1}^{m+1}\left[\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}} +\left( \lambda_{\max}(A_{k}^{-1})\right)^{m_{0}/2}\right]<+\infty, \end{array} $$

this completes the proof. □

Proof 20 (Proof of Lemma 3.2).

We have

$$ \begin{array}{@{}rcl@{}} \frac{1}{T}{\int}_{\phi_{j-1}T}^{\phi_{j} T}\mathbf{Y}(t)dt&=& \frac{1}{ T}\sum\limits_{k=\lfloor \phi_{j-1} T\rfloor+1}^{\left \lfloor{\phi_{j} T}\right \rfloor}{\int}_{k-1}^{k}\mathbf{Y}_{j}(t)dt+\frac{1}{ T}{\int}_{\left \lfloor{\phi_{j} T}\right \rfloor}^{\phi_{j} T}\mathbf{Y}_{j}(t)dt \\&&-\frac{1}{T}{\int}_{\lfloor\phi_{j-1}T\rfloor}^{\phi_{j-1}T}\mathbf{Y}_{j-1}(t)dt. \end{array} $$

(B.5)

Since $\{\mathbf {Y}_{j}(t+k-1)\}_{k=1}^{\infty }$ is a stationary and ergodic process, we conclude that $\left \{\displaystyle {{\int \limits }_{k-1}^{k}}\mathbf {Y}_j(t)dt\right \}_{k=1}^{\infty }=\left \{\displaystyle {{{\int \limits }_{0}^{1}}}\mathbf {Y}_{j}(t+k-1)dt\right \}_{k=1}^{\infty }$ is a stationary and ergodic process. Then, by using Birkhoff’s ergodic theorem,

and then, together with Fubini’s theorem, we get

(B.6)

By the triangle inequality,

Hence,

(B.7)

Further, by the triangle inequality and Jensen’s inequality, we get

$$ \begin{array}{@{}rcl@{}} \mathrm{E}\left( \left\|\phi_{j}\frac{1}{\phi_{j} T}{\int}_{\left \lfloor{\phi_{j} T}\right \rfloor}^{\phi_{j} T}\mathbf{Y}_{j}(t)dt\right\|^{p}\right)\leqslant \left( \frac{\phi_{j}T-\left \lfloor{\phi_{j} T}\right \rfloor}{\phi_{j}T}\right)^{p-1}\phi_{j}\frac{1}{\phi_{j} T}{\int}_{\left \lfloor{\phi_{j} T}\right \rfloor}^{\phi_{j} T}\mathrm{E}\left( \|\mathbf{Y}_{j}(t)\|^{p}\right)dt. \end{array} $$

Then, since Y_j(t) is L^p-bounded, this implies that

(B.8)

Similarly, we have

(B.9)

The proof is completed by combining the relations Eqs. B.5–B.9. □

Proof 21 (Proof of Proposition 3.1).

(i). From Proposition 2.5, we have

for $j=1,2,\dots ,m+1$. Then, Part (i) will be established if we prove that

for $j = 1,2,\dots ,m+1$. To this end, note that the processes $\left \{\tilde {\mathbf {X}}(t)\tilde {\mathbf {X}}^{\prime }(t):t\!\geqslant \! 0\right \}$ and $\left \{\tilde {\mathbf {X}}_{j}(t)\tilde {\mathbf {X}}_{j}^{\prime }(t):t\geqslant 0\right \}$ are $\text {L}^{m_{0}/2}$-bounded. Then, set $\tilde {\mathbf {X}}(t)\tilde {\mathbf {X}}^{\prime }(t)\equiv \mathbf {Y}(t)$, and set $\tilde {\mathbf {X}}_{j}(t)\tilde {\mathbf {X}}_{j}^{\prime }(t)\equiv \mathbf {Y}_{j}(t)$, for $j=1,\dots ,m+1$. Hence, by using Lemma 3.2, we have

for $j=1,2,\dots ,m+1$. Then, the proof of Part (i) is completed if we prove that

$$ \begin{array}{@{}rcl@{}} \mathrm{E}\left( \tilde{\mathbf{X}}_{j}(t)\tilde{\mathbf{X}}_{j}^{\prime}(t)dt\right) =\tilde{h}_{j}(t)\tilde{h}^{\prime}_{j}(t)+\mathbf{V}_{j}(0), \end{array} $$

for $j=1,\dots ,m+1$. For $j=1,2,\dots ,m+1$. Indeed, we have

$$ \begin{array}{@{}rcl@{}} \mathrm{E}\left( \tilde{\mathbf{X}}_{j}(t)\tilde{\mathbf{X}}^{\prime}_{j}(t)\right) =\tilde{h}_{j}(t)\tilde{h}^{\prime}_{j}(t)+\tilde{h}_{j}(t)\text{E}\left( \tilde{Z}^{\prime}_{j}(t)\right) +\text{E}\left( \tilde{Z}_{j}(t)\right)\tilde{h}^{\prime}_{j}(t)+\text{E}\left( \tilde{Z}_{j}(t)\tilde{Z}^{\prime}_{j}(t)\right), \end{array} $$

and,

$$ \begin{array}{@{}rcl@{}} \mathrm{E}(\tilde{Z}_{j}(t))=e^{-A_{j}t}\left[\mathrm{E}\left( {{\int}_{0}^{t}}e^{A_{j}s}\mathbf{\Sigma}^{1/2}d{W}^{(1)}_{s}\right) +\mathrm{E}\left( {\int}_{-\infty}^{0}e^{A_{j}s}\mathbf{\Sigma}^{1/2}d{W}^{(2)}_{-s}\right)\right]=0. \end{array} $$

(B.10)

Further, by Proposition 2.3, for $j=1,\dots ,m+1$, $\mathbf {V}_{j}(0)=\text {E}\left (\tilde {Z}_{j}(t)\tilde {Z}^{\prime }_{j}(t)\right )$ does not depend on t. Then,

$$ \begin{array}{@{}rcl@{}} \mathrm{E}\left( \tilde{\mathbf{X}}_{j}(t)\tilde{\mathbf{X}}_{j}^{\prime}(t)\right) =\tilde{h}_{j}(t)\tilde{h}^{\prime}_{j}(t)+\mathbf{V}_{j}(0),\quad{ } j=1,2,\dots,m+1, \end{array} $$

this proves the statement in Part (i). The statements in (ii) and (iii) are established in the similar ways. □

Proposition B.2.

For j = 1,2,3, let {κ_jT : T > 0}, {ι_jT : T > 0}{α_jT : T > 0},{β_jT : T > 0}, {ϱ_jT : T > 0}, be collections of random matrices such that , , , , , where, for j = 1,2,3, κ_j, α_j, ι_j and β_j,ϱ_j, are non-random matrices. If a collection of p × q random matrices {Y_T : T > 0} is such that , where Λ is a pq × pq matrix. We have

with $\mathbf {{\varrho }}= \left (\begin {array}{c} {\varrho }_1\\ {\varrho }_2\\ {\varrho }_3 \end {array} \right )$, $\mathbf {A}= \left (\begin {array}{c c c} \mathbf {A}_{11} & \mathbf {A}_{12}& \mathbf {A}_{13}\\ \mathbf {A}_{21} & \mathbf {A}_{22}& \mathbf {A}_{23}\\ \mathbf {A}_{31} & \mathbf {A}_{32}& \mathbf {A}_{33} \end {array} \right )$, where $\quad { } \mathbf {A}_{ji}=(\mathbf {A}_{ij})^{\prime }, i,j=1,2,3$ and

$$ \begin{array}{@{}rcl@{}} \mathbf{A}_{ij}&=&(\kappa_{i}\otimes\iota^{\prime}_{i}){\Lambda}(\kappa^{\prime}_{j}\otimes\iota_{j})+(\kappa_{i}\otimes\iota^{\prime}_{i}){\Lambda}(\alpha^{\prime}_{j}\otimes \beta_{j}) +(\alpha_{i}\otimes \beta^{\prime}_{i})\mathbf{\Lambda}(\alpha^{\prime}_{j}\otimes \beta_{j}) \\ &&+(\alpha_{i}\otimes \beta^{\prime}_{i}){\Lambda}(\alpha^{\prime}_{j}\otimes \beta_{j}). \end{array} $$

The proof follows directly from the properties of multivariate distribution along with some algebraic computations.

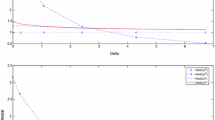
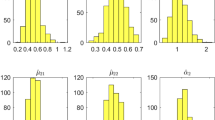
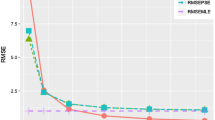
Appendix C: Additional Simulation Results