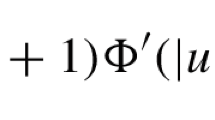Abstract
The existence of a solution to an important singular coagulation equation with a multiple fragmentation kernel has been recently proved in Jpn J Ind Appl Math 35(3):1283–1302, 2018. This paper proves the uniqueness of the solution to the same problem in the function space \(\varOmega _{.,r_2} (T) = \bigcup _{\lambda >0 }\varOmega _{\lambda , r_2} (T)\), where \(\varOmega _{\lambda , r_2} (T)\) is the space of all continuous functions f such that
and \(0< r_2 < 1\).
Similar content being viewed by others
References
Aizenman, M., Bak, T.A.: Convergence to equilibrium in a system of reacting polymers. Commun. Math. Phys. 65(3), 203–230 (1979)
Banasiak, J.: On a non-uniqueness in fragmentation models. Math. Methods Appl. Sci. 25(7), 541–556 (2002)
Banasiak, J., Lamb, W.: Global strict solutions to continuous coagulation-fragmentation equations with strong fragmentation. Proc. R. Soc. Edinb. Sect. A Math. 141(03), 465–480 (2011)
Camejo, C.C., Gröpler, R., Warnecke, G.: Regular solutions to the coagulation equations with singular kernels. Math. Methods Appl. Sci. 38(11), 2171–2184 (2015)
Costa, F.P.: Existence and uniqueness of density conserving solutions to the coagulation-fragmentation equations with strong fragmentation. J. Math. Anal. Appl. 192(3), 892–914 (1995)
Ding, A., Hounslow, M.J., Biggs, C.A.: Population balance modelling of activated sludge flocculation: investigating the size dependence of aggregation, breakage and collision efficiency. Chem. Eng. Sci. 61(1), 63–74 (2006)
Dubovskiǐ, P.B., Stewart, I.W.: Existence, uniqueness and mass conservation for the coagulation-fragmentation equation. Math. Methods Appl. Sci. 19(7), 571–591 (1996)
Ernst, M.H., Ziff, R.M., Hendriks, E.M.: Coagulation processes with a phase transition. J. Colloid Interface Sci. 97(1), 266–277 (1984)
Galkin, V.A., Dubovski, P.B.: Solutions of a coagulation equation with unbounded kernels. Differ. Uravn. 22(3), 504–509 (1986)
Ghosh, D., Kumar, J.: Existence of mass conserving solution for the coagulation-fragmentation equation with singular kernel. Jpn. J. Ind. Appl. Math. 35(3), 1283–1302 (2018)
Giri, A.K., Kumar, J., Warnecke, G.: The continuous coagulation equation with multiple fragmentation. J. Math. Anal. Appl. 374(1), 71–87 (2011)
Giri, A.K., Warnecke, G.: Uniqueness for the coagulation-fragmentation equation with strong fragmentation. Z. Angew. Math. Phys. 62(6), 1047–1063 (2011)
Hounslow, M.: The population balance as a tool for understanding particle rate processes. KONA Powder Part. J. 16, 179–193 (1998)
Kapur, P.C.: Kinetics of granulation by non-random coalescence mechanism. Chem. Eng. Sci. 27(10), 1863–1869 (1972)
Melzak, Z.A.: A scalar transport equation. Trans. Am. Math. Soc. 85(2), 547–560 (1957)
Müller, H.: Zur allgemeinen theorie ser raschen koagulation. Fortschr. Kolloide Polym. 27(6), 223–250 (1928)
Norris, J.R.: Smoluchowski’s coagulation equation: uniqueness, nonuniqueness and a hydrodynamic limit for the stochastic coalescent. Ann. Appl. Probab. 9(1), 78–109 (1999)
Peglow, M.: Beitrag zur modellbildung von eigenschaftsverteilten dispersen systemen am beispiel der wirbelschicht-sprühagglomeration. PhD Thesis, Otto-von-Guericke-Universität Magdeburg (2005)
Shiloh, K., Sideman, S., Resnick, W.: Coalescence and break-up in dilute polydispersions. Can. J. Chem. Eng. 51(5), 542–549 (1973)
Smoluchowski, M.V.: An experiment on mathematical theorization of coagulation kinetics of the colloidal solutions. Z. Phys. Chemie 92, 129–168 (1917)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
About this article
Cite this article
Ghosh, D., Kumar, J. Uniqueness of solutions to the coagulation–fragmentation equation with singular kernel. Japan J. Indust. Appl. Math. 37, 487–505 (2020). https://doi.org/10.1007/s13160-020-00412-4
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13160-020-00412-4
Keywords
- Coagulation–fragmentation equation
- Singular coagulation kernel
- Multiple fragmentation kernel
- Uniqueness result