Fuzzy multi-objective deterministic inventory model with time dependent demand and holding cost under Pythagorean fuzzy goal programming approach

Abstract

In this present study we have considered a deterministic inventory model in which we have taken deterioration as a function of time and demand function is considered initially linear for some time and next exponential. In the presence of uncertainty all the cost parameters are taken as generalized triangular fuzzy number. The proposed inventory model has been solved by fuzzy nonlinear programming approach and Pythagorean fuzzy goal programming approach. Finally we have taken numerical examples and sensitivity analysis for some cost parameters.

Appendix

The governing differential equations are given bellow.

$$\frac{{dQ_{1i} }}{dt} = - D_{i} = - a_{i} t,\quad 0 \le t \le t_{1i}$$

(23)

$$\frac{{dQ_{2i} }}{dt} = - D_{i} \left( t \right) - w_{i} .Q_{2i} \left( t \right) = - a_{i} e^{{\alpha_{i} t}} - \alpha_{i} t.Q_{2i} \left( t \right),\quad t_{1i} \le t \le t_{2i}$$

(24)

$$\frac{{dQ_{3i} }}{dt} = - {\text{b}}_{{\text{i}}} ,\quad t_{2i} \le t \le t_{3i}$$

(25)

We have the boundary condition $Q_{2i} \left( {t_{2i} } \right) = 0, Q_{1i} \left( 0 \right) = Q_{max}$ and a condition where $Q_{1i} \left( {t_{1i} } \right) = Q_{2i} \left( {t_{1i} } \right)$.

From (23)

$$dQ_{1i} = - a_{i} t ;with Q_{1i} \left( 0 \right) = Q_{max}$$

(26)

Integrating both sides of (4) we get

$$\begin{aligned} \smallint dQ_{1i} & = \smallint - a_{i} tdt \\ Q_{1i} \left( t \right) & = - \frac{{a_{i} t^{2} }}{2} + C_{1} \left( { integrating\;constant} \right) \\ \end{aligned}$$

(27)

Using the condition $Q_{1i} \left( 0 \right) = Q_{max}$ we get from (27)

$$Q_{1i} \left( t \right) = Q_{max} - \frac{{a_{i} t^{2} }}{2}$$

(28)

Now from (24) we have

$$\frac{{dQ_{2i} }}{dt} + \alpha_{i} t.Q_{2i} \left( t \right) = - a_{i} e^{{a_{i} t}}$$

This is a linear equation with integrating factor (I.F)$= e^{{\smallint \alpha_{i} .t.dt}} = e^{{\frac{{a_{i} t^{2} }}{2}}}$.

Therefore,

$$\begin{aligned} Q_{2i} \left( t \right) \cdot e^{{\frac{{a_{i} t^{2} }}{2}}} & = - a_{i} \smallint e^{{a_{i} t}} \cdot e^{{\frac{{a_{i} t^{2} }}{2}}} dt \\ & = - a_{i} \smallint e^{{a_{i} t + \frac{{a_{i} t^{2} }}{2}}} dt \\ & = - a_{i} \left[ {1 + a_{i} t + \frac{{a_{i} t^{2} }}{2} + \left( {a_{i} t + \frac{{a_{i} t^{2} }}{2}} \right)^{2} + \ldots } \right] + C_{2} \left( {{\text{integrating}}\;{\text{constant}}} \right) \\ \end{aligned}$$

(29)

Now using the condition $Q_{2i} \left( {t_{2i} } \right) = 0 in \left( {7^{\prime}} \right)and finding the value of C_{2}$ we get,

$$Q_{2i} \left( t \right) = a_{i} e^{{ - \frac{{\alpha_{i} t^{2} }}{2}}} \left\{ {\left( {t_{2i} - t} \right) + \frac{{a_{i} }}{2}\left( {t_{2i}^{2} - t^{2} } \right) + \frac{1}{3}\left( {\frac{{\alpha_{i} }}{{a_{i} }} + a_{i}^{2} } \right)\left( {t_{2i}^{3} - t^{3} } \right) + \frac{{a_{i} \alpha_{i} }}{4}\left( {t_{2i}^{4} - t^{4} } \right)} \right\}$$

(30)

From the Eq. (25) we have

$$\begin{aligned} \frac{{dQ_{3i} }}{dt} & = - {\text{b}}_{{\text{i}}} \\ dQ_{3i} & = - {\text{b}}_{{\text{i}}} \cdot dt\quad for\quad t_{2i} \le t \le t_{3i} \\ \end{aligned}$$

(31)

Integrating (31) we get,

$$Q_{3i} = - b_{i} t + C_{3} \left( { Integrating constant} \right)$$

(32)

Using the condition $Q_{3i} \left( {t_{2i} } \right) = 0$ we get from (31)

$$Q_{3i} = b_{i} \left( {t_{2i} - t_{3i} } \right)$$

(33)

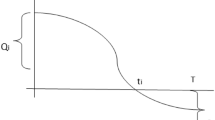
Equation (28) represents the inventory during the time interval $0 \le t \le t_{1i} .$

Equation (30) represents the inventory during the time interval $t_{1i} \le t \le t_{2i}$.

Equation (33) represents the negative inventory during the time interval $t_{2i} \le t \le t_{3i}$.