Unified killing mechanism in a single server queue with renewal input

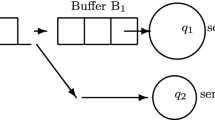
Abstract

Queueing systems experienced in real-life situations are very often influenced by negative arrivals which are independent of service process and cause the elimination of jobs from the system. Such a scenario occurs in computer network and telecommunication systems where an attack by a malicious virus results in the removal of some or all data files from the system. Along this direction many authors have proposed various killing processes in the past. This paper unifies different killing mechanisms into the classical single server queue having infinite capacity, where arrival occurs as renewal process with exponential service time distribution. The system is assumed to be affected by negative customers as well as disasters. The model is investigated in steady-state in a very simple and elegant way by means of supplementary variable and difference equation technique. The distribution of system-content for the positive customers is derived in an explicit form at pre-arrival and random epochs. The influence of different parameters on the system performance are also examined.

Appendix

Corollary 1

The equation$A^*(\mu - \mu z)p+ A^*(\mu - \mu z)q_1z^2-z=0$has only one root in (0, 1) iff$\frac{\lambda (p-q_1)}{\mu }<1$.

Proof

Let $f(z)=-z$ and $g(z)= A^*(\mu - \mu z)p+ A^*(\mu - \mu z)q_1z^2$. Let $H(z)=A^*(\mu - \mu z)$, it is analytic inside the unit circle and can be written as $H(z)=\sum _{i=0}^{\infty }h_iz^i$. The coefficients are such that $h_i \ge 0~~ \forall ~ i \ge 0$. Consider the circle $|z|=1- \epsilon$ where $\epsilon >0$ is a very small quantity. Now, $|f(z)|= 1- \epsilon$ and

$$\begin{aligned} |g(z)|= & {} |H(z)p+ H(z)q_1z^2| \nonumber \\\le & {} H(|z|)p+ H(|z|)q_1|z|^2 \nonumber \\= & {} H(1- \epsilon )p+ H(1- \epsilon )q_1(1- \epsilon )^2 \nonumber \\= & {} \left( 1- \frac{\epsilon \mu }{\lambda }+ o(\epsilon )\right) p+ \left( 1- \frac{\epsilon \mu }{\lambda }+ o(\epsilon )\right) (q_1-2q_1\epsilon + o(\epsilon )) \nonumber \\= & {} 1- \epsilon \left( \frac{\mu }{\lambda }+2q_1\right) + o(\epsilon ) \end{aligned}$$

(18)

From (18) it can be observed that $|g(z)|< |f(z)|$ iff $(\frac{\mu }{\lambda }+2q_1)>1$ i.e., $\frac{\lambda (p-q_1)}{\mu }<1$. Thus using Rouch$\acute{e}$’s theorem, f(z) and $f(z)+g(z)$ have equal number of zeroes inside $|z|=1$ and hence $f(z)+g(z)$ has only one root in the interval (0, 1) under the condition $\frac{\lambda (p-q_1)}{\mu }<1$. $\square$

Corollary 2

The equation$A^*(\mu - \mu z)p-z=0$has only one root in (0, 1) iff$\frac{\mu p}{\lambda }>1$.

Proof

As before let $f(z)=-z$ and $g(z)= A^*(\mu - \mu z)p$ and consider $|z|=1- \epsilon$. Since $|f(z)|= 1- \epsilon$ and $|g(z)|= p- \frac{\epsilon p \mu }{\lambda }< 1-\frac{\epsilon p \mu }{\lambda }$, this suggests that $f(z)+g(z)$ has only one root in (0, 1) under the condition $\frac{\mu p}{\lambda }>1$. $\square$

Corollary 3

The equation$A^*(\mu + \delta - \mu z) - z=0$has only one root in (0, 1) under the sufficient condition$\delta > 0$.

Proof

Let $f(z)=-z$ and $g(z)= A^*(\mu + \delta - \mu z)=H(z)$ as defined in Corollary 1. Consider $|z|=1- \epsilon$ such that $\epsilon > 0$ is a very small quantity. Now, $|f(z)|= 1- \epsilon$ and

$$\begin{aligned} |g(z)| = |H(z)| \le H(1- \epsilon )= A^*(\delta ) - \epsilon A^{*(1)}(\delta )(-\mu )+ o(\epsilon ) \end{aligned}$$

Since, $|g(z)|< |f(z)|$ implies that $\delta > 0$. Thus applying Rouch$\acute{e}$’s theorem it can be concluded that $A^*(\mu + \delta - \mu z) - z=0$ has only one root in (0, 1). $\square$