Skip to main content
SpringerLink
Log in

Canard Cycles and Homoclinic Orbit of a Leslie–Gower Predator–Prey Model with Allee Effect and Holling Type II Functional Response

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

We study dynamics of a fast–slow Leslie–Gower predator–prey system with Allee effect and Holling Type II functional response. More specifically, we show some sufficient conditions to guarantee the existence of two positive equilibria of the system and their location, and then we further fully determine their dynamics. Based on geometric singular perturbation theory and the slow–fast normal form, we determine the associated bifurcation curve and observe canard explosion. Besides, we also find a homoclinic orbit to a saddle with slow and fast segments, in which, the stable and unstable manifolds of the saddle are connected under explicit parameters conditions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Data Availability

No datasets were generated or analysed during the current study.

References

  1. Leslie, P.H.: Some further notes on the use of matrices in population mathematics. Biometrika 35(3/4), 213–245 (1948)

    Article  MathSciNet  Google Scholar 

  2. Leslie, P.: A stochastic model for studying the properties of certain biological systems by numerical methods. Biometrika 45(1–2), 16–31 (1958)

    Article  MathSciNet  Google Scholar 

  3. Hsu, S.B., Huang, T.W.: Global stability for a class of predator-prey systems. SIAM J. Appl. Math. 55(3), 763–783 (1995)

    Article  MathSciNet  Google Scholar 

  4. Hsu, S.B., Hwang, T.W.: Hopf bifurcation analysis for a predator–prey system of Holling and Leslie type. Taiwan. J. Math. 3(1), 35–53 (1999)

    Article  MathSciNet  Google Scholar 

  5. Korobeinikov, A.: A Lyapunov function for Leslie–Gower predator–prey models. Appl. Math. Lett. 14(6), 697–699 (2001)

    Article  MathSciNet  Google Scholar 

  6. Yuan, S., Song, Y.: Bifurcation and stability analysis for a delayed Leslie–Gower predator–prey system. IMA J. Appl. Math. 74(4), 574–603 (2009)

    Article  MathSciNet  Google Scholar 

  7. Holling, C.S.: The functional response of predators to prey density and its role in mimicry and population regulation. Mem. Entomol. Soc. Can. 97(S45), 5–60 (1965)

    Article  Google Scholar 

  8. Huang, J., Ruan, S., Song, J.: Bifurcations in a predator–prey system of Leslie type with generalized Holling type III functional response. J. Differ. Equ. 257(6), 1721–1752 (2014)

    Article  MathSciNet  Google Scholar 

  9. Dai, Y., Zhao, Y., Sang, B.: Four limit cycles in a predator–prey system of Leslie type with generalized Holling type III functional response. Nonlinear Anal. Real World Appl. 50, 218–239 (2019)

    Article  MathSciNet  Google Scholar 

  10. Tiwari, V., Tripathi, J.P., Mishra, S., Upadhyay, R.K.: Modeling the fear effect and stability of non-equilibrium patterns in mutually interfering predator–prey systems. Appl. Math. Comput. 371, 124948 (2020)

    MathSciNet  Google Scholar 

  11. Tripathi, J.P., Bugalia, S., Jana, D., Gupta, N., Tiwari, V., Li, J., Sun, G.Q.: Modeling the cost of anti-predator strategy in a predator-prey system: the roles of indirect effect. Math. Methods Appl. Sci. 45(8), 4365–4396 (2022)

    Article  MathSciNet  Google Scholar 

  12. Tripathi, J.P., Abbas, S., Thakur, M.: Dynamical analysis of a prey–predator model with Beddington–DeAngelis type function response incorporating a prey refuge. Nonlinear Dyn. 80, 177–196 (2015)

    Article  MathSciNet  Google Scholar 

  13. Tripathi, J.P., Abbas, S., Thakur, M.: A density dependent delayed predator-prey model with Beddington–DeAngelis type function response incorporating a prey refuge. Commun. Nonlinear Sci. Numer. Simul. 22(1–3), 427–450 (2015)

    Article  MathSciNet  Google Scholar 

  14. Allee, W., Bowen, E.S.: Studies in animal aggregations: mass protection against colloidal silver among goldfishes. J. Exp. Zool. 61(2), 185–207 (1932)

    Article  Google Scholar 

  15. Tripathi, J.P., Mandal, P.S., Poonia, A., Bajiya, V.P.: A widespread interaction between generalist and specialist enemies: the role of intraguild predation and Allee effect. Appl. Math. Model. 89, 105–135 (2021)

    Article  MathSciNet  Google Scholar 

  16. Aguirre, P., Gonzalez-Olivares, E., Sáez, E.: Two limit cycles in a Leslie–Gower predator-prey model with additive Allee effect. Nonlinear Anal. Real World Appl. 10(3), 1401–1416 (2009)

    Article  MathSciNet  Google Scholar 

  17. Aguirre, P., González-Olivares, E., Sáez, E.: Three limit cycles in a Leslie-Gower predator-prey model with additive Allee effect. SIAM J. Appl. Math. 69(5), 1244–1262 (2009)

    Article  MathSciNet  Google Scholar 

  18. Aguirre, P.: A general class of predation models with multiplicative Allee effect. Nonlinear Dyn. 78(1), 629–648 (2014)

    Article  MathSciNet  Google Scholar 

  19. Zhu, Z., Chen, Y., Li, Z., Chen, F.: Stability and bifurcation in a Leslie–Gower predator–prey model with Allee effect. Int. J. Bifurc. Chaos 32(03), 2250040 (2022)

    Article  MathSciNet  Google Scholar 

  20. Zu, J., Mimura, M.: The impact of Allee effect on a predator-prey system with Holling type II functional response. Appl. Math. Comput. 217(7), 3542–3556 (2010)

    MathSciNet  Google Scholar 

  21. Zu, J.: Global qualitative analysis of a predator-prey system with Allee effect on the prey species. Math. Comput. Simul. 94, 33–54 (2013)

    Article  MathSciNet  Google Scholar 

  22. Wang, C., Zhang, X.: Canards, heteroclinic and homoclinic orbits for a slow–fast predator–prey model of generalized Holling type III. J. Differ. Equ. 267(6), 3397–3441 (2019)

    Article  MathSciNet  Google Scholar 

  23. Su, W., Zhang, X.: Global stability and canard explosions of the predator–prey model with the Sigmoid functional response. SIAM J. Appl. Math. 82(3), 976–1000 (2022)

    Article  MathSciNet  Google Scholar 

  24. Chen, X., Zhang, X.: Dynamics of the predator-prey model with the Sigmoid functional response. Stud. Appl. Math. 147(1), 300–318 (2021)

    Article  MathSciNet  Google Scholar 

  25. Atabaigi, A.: Canard explosion, homoclinic and heteroclinic orbits in singularly perturbed generalist predator–prey systems. Int. J. Biomath. 14(01), 2150003 (2021)

    Article  MathSciNet  Google Scholar 

  26. Saha, T., Pal, P.J., Banerjee, M.: Slow-fast analysis of a modified Leslie–Gower model with Holling type I functional response. Nonlinear Dyn. 108(4), 4531–4555 (2022)

    Article  Google Scholar 

  27. Zhu, Z., Liu, X.: Canard cycles and relaxation oscillations in a singularly perturbed Leslie–Gower predator-prey model with Allee effect. Int. J. Bifur. Chaos 32(05), 2250071 (2022)

    Article  MathSciNet  Google Scholar 

  28. Zhao, L., Shen, J.: Canards and homoclinic orbits in a slow–fast modified May–Holling–Tanner predator-prey model with weak multiple Allee effect. Discrete Contin. Dyn. Syst. B 27(11), 6745–6769 (2022)

    Article  MathSciNet  Google Scholar 

  29. Shi, T., Wen, Z.: Canard cycles and their cyclicity of a fast–slow Leslie–Gower predator–prey model with Allee effect. In: International Journal of Bifurcation and Chaos, accepted

  30. Chowdhury, P.R., Banerjee, M., Petrovskii, S.: Canards, relaxation oscillations, and pattern formation in a slow–fast ratio-dependent predator-prey system. Appl. Math. Model. 109, 519–535 (2022)

    Article  MathSciNet  Google Scholar 

  31. Li, K., Li, S., Wu, K.: Relaxation oscillations and canard explosion of a Leslie–Gower predator-prey system with addictive Allee effect. Int. J. Bifurc. Chaos 32(11), 2250168 (2022)

    Article  MathSciNet  Google Scholar 

  32. Wen, Z., Shi, T.: Existence and uniqueness of a canard cycle with cyclicity at most two in a singularly perturbed Leslie-Gower predator-prey model with prey harvesting. Int. J. Bifurc. Chaos 34(03), 2450036 (2024)

    Article  MathSciNet  Google Scholar 

  33. Fenichel, N.: Geometric singular perturbation theory for ordinary differential equations. J. Differ. Equ. 31(1), 53–98 (1979)

    Article  MathSciNet  Google Scholar 

  34. Krupa, M., Szmolyan, P.: Relaxation oscillation and canard explosion. J. Differ. Equ. 174(2), 312–368 (2001)

    Article  MathSciNet  Google Scholar 

  35. Kuznetsov, Y.A., Muratori, S., Rinaldi, S.: Homoclinic bifurcations in slow–fast second order systems. Nonlinear Anal. Theory Methods Appl. 25(7), 747–762 (1995)

    Article  MathSciNet  Google Scholar 

  36. Shen, J.: Canard limit cycles and global dynamics in a singularly perturbed predator–prey system with non-monotonic functional response. Nonlinear Anal. Real World Appl. 31, 146–165 (2016)

    Article  MathSciNet  Google Scholar 

  37. Krupa, M., Szmolyan, P.: Extending geometric singular perturbation theory to nonhyperbolic points–fold and canard points in two dimensions. SIAM J. Math. Anal. 33(2), 286–314 (2001)

    Article  MathSciNet  Google Scholar 

  38. Lu, M., Huang, J.: Global analysis in Bazykin’s model with Holling II functional response and predator competition. J. Differ. Equ. 280, 99–138 (2021)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Huaqiao University, Quanzhou, 362021, People’s Republic of China

    Tianyu Shi & Zhenshu Wen

Authors
  1. Tianyu Shi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhenshu Wen
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

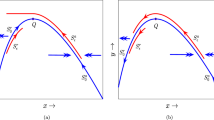
T.S. carried out the investigation, prepared figure 1 and wrote the original draft manuscript. Z.W. convinced the study, verified the investigation and wrote the main manuscript. All authors reviewed the manuscript.

Corresponding author

Correspondence to Zhenshu Wen.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work is partially supported by the National Natural Science Foundation of China (12071162), the Natural Science Foundation of Fujian Province (No. 2021J01302) and the Fundamental Research Funds for the Central Universities (No. ZQN–802).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shi, T., Wen, Z. Canard Cycles and Homoclinic Orbit of a Leslie–Gower Predator–Prey Model with Allee Effect and Holling Type II Functional Response. Qual. Theory Dyn. Syst. 23, 197 (2024). https://doi.org/10.1007/s12346-024-01059-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-024-01059-z

Keywords

Mathematics Subject Classification

Search

Navigation