A non-stationary relay-based 3D MIMO channel model with time-variant path gains for human activity recognition in indoor environments

Abstract

Extensive research showed that the physiological response of human tissue to exposure to low-frequency electromagnetic fields is the induction of an electric current in the body segments. As a result, each segment of the human body behaves as a relay, which retransmits the radio-frequency (RF) signal. To investigate the impact of this phenomenon on the Doppler characteristics of the received RF signal, we introduce a new three-dimensional (3D) non-stationary channel model to describe the propagation phenomenon taking place in an indoor environment. Here, the indoor space is equipped with a multiple-input multiple-output (MIMO) system. A single person is moving in the indoor space and is modelled by a cluster of synchronized moving point scatterers, which behave as relays. We derive the time-variant (TV) channel transfer function (CTF) with TV path gains and TV path delays. The expression of the TV path gains is obtained from the instantaneous total received power at the receiver side. This TV total received power is expressed as the product of the TV power of the RF signal initially transmitted and received by a body segment and the TV received power of the redirected signal. These TV powers are determined according the free-space path-loss model. Also, a closed-form approximate solution to the spectrogram of the TVCTF is derived. Here, we analyse the effect of the motion of the person and the validity of the relay assumption on the spectrogram, the TV mean Doppler shift (MDS), and the TV Doppler shift (DS) of the TVCTF. Simulation results are presented to illustrate the proposed channel model.

1 Introduction

According to the World Health Organization (WHO), the elderly population (ages 65+) is expected to triple in the next 30 years and reach 16% of the global population [2]. Thus, there has been a great focus on the study of home care systems based on human activity recognition, tracking, and classification techniques. This is of great interest as most older people prefer to live independently in their own homes [10]. But, living alone, albeit in a familiar environment, carries the risk of incidents like falls and unconsciousness [31]. For example, as reported in [34], approximately 30% of people over 65 and 50% of people over 85 fall at least once a year. This calls for robust in-home tracking and monitoring systems.

A review of the literature shows that human activity recognition systems utilize three major techniques: video or optical marker–based surveillance, wearable and context-aware inertial measurement units (IMUs), and radio frequency (RF) signal processing [14, 16, 21]. With the recent developments in computer vision and digital cameras, vision-based and/or marker-based techniques have been widely investigated in the context of in-home activity monitoring. Here, the markers are placed on the human body and must be clearly visible to the cameras. They are based on extensive image processing and/or the use of optical marker–based motion capture systems, e.g., [15, 17, 25]. Wearable and context-aware systems use IMUs which can be placed on the person (such as watch, smartphone, clothes, etc.) or deployed in the room (along the floor or on the walls). They employ the embedded accelerometers, gyroscopes, and magnetometers data to identify and classify human activities [24, 28]. Apart from privacy concerns, the main drawbacks of the first two categories are that the person may forget to wear the sensors-based devices or move outside the detection range of the non-wearable sensors/cameras [30]. This is of practical importance, especially for the elderly population. Non-wearable RF-based systems have been introduced as a solution to overcome the disadvantages of the aforementioned systems [21]. These RF-based systems track and monitor indoor human activities by analyzing and exploiting the effects of the human motion on the Doppler characteristics of the transmitted RF signals. Several parameters can be utilized for this underlying task such as the received signal strength, the TV phase of the received signal, the time-of-arrival, the time differences of arrival, and the angle-of-arrival. More papers on this topic can be found in [7, 12, 18, 20, 23, 32, 33].

The design, development, and implementation of efficient RF-based methods rely heavily on accurate indoor channel models to describe the non-stationary multipath propagation phenomenon. The majority of the aforementioned studies are based on scenario specific multipath channel models, where the moving person or object is modelled by a single point scatterer, and assume constant path gains when expressing the received RF signal. A fixed-to-fixed (F2F) single-input single-output (SISO) three-dimensional (3D) channel model with constant path gains has been reported in [3], where the body segments of the moving person have been modelled by a cluster of synchronized moving point scatterers. The authors analyzed the effects of the motion of the person on the spectrogram of the complex channel gain, the TV mean Doppler shift (MDS), and TV Doppler spread (DS). More recently, a F2F SISO 3D non-stationary channel model with a stochastic trajectory model has been presented in [6], where the person is modelled by a single moving scatterer. The expression of the complex channel gain of this model incorporates TV path gains that change with the total distance travelled by the moving person from the transmitter to the receiver. In [6], the TV path gains are determined according to the path loss model [29] by considering the sum of the TV distances between the body segments (moving scatterers) and the transmitter/receiver. In other words, diffuse reflection is assumed. This means that the incoming radio waves bounce back in all directions without absorption, redirection, or any physical alteration. This assumption is not consistent with the results of the studies reported in [1, 8, 9, 22], in which the effects of electromagnetic fields, particularly in the extreme low-frequency range, on the human body were investigated. As shown in [1, 8, 9, 22], when exposed to a low-frequency electromagnetic field, the human tissues (body) generate(s) an electric current. This current is generally referred to as “induced current.” In this case, the human body does not reflect the incoming radio waves, but it retransmits them. Thus, the different body segments of the moving person (moving point scatterers) are assumed to play the role of relays, which redirect the transmitted RF signal. This problem was initially addressed in [13], where a new channel model with TV path gains and TV path delays was introduced. In [13], each body segment is assumed to play the role of a moving relay. In this case, the TV path gain of each multipath component is computed in terms of the product of the mean power of the initially transmitted signal and the mean power of the redirected signal (received by the receive antennas). These mean powers can be modelled according to the free-space path loss model [29]. Here, the result depends on the product of the TV distance between the transmit antenna and the moving scatterer (body segment) and the TV distance between the same moving scatterer and the receive antenna. This problem was initially studied in [13]. In [13], relay properties are applied to compute the total received power, from which the expression of the TV path gains is derived.

With this paper, we extend the work in [13] to address this problem. To do so, we present a new generic F2F multiple-input multiple-output (MIMO) 3D channel model to describe the non-stationary behavior of indoor environments. Here, the different body segments of the moving person (modelled by a synchronized cluster of moving point scatterers) play the role of moving relays. The impacts of the fixed objects and the line-of-sight component (LOS) are taken into account. Since they do not experience Doppler effects, they can be modelled by fixed point scatterers with constant path gains and constant path delays. Here, we present an expression for the TV channel transfer function (CTF) of the received RF signal with TV path gains and TV path delays. The free-space path loss model [29] is applied to describe the total received power of the initially transmitted signal and the received power of the redirected radio signal. Later, a closed-form approximate solution to the spectrogram of the TVCTF is derived. The influence of introducing TV path gains and assuming the relay-like behavior of the different body segments on the Doppler characteristics of the channel is analyzed. The impact of considering TV path gains and multiple antenna elements at both the transmitter side and receiver side is also investigated. These tasks are achieved by means of the spectrogram of the TVCTF, the TV mean Doppler shift (MDS), and the TV Doppler spread (DS). Simulation results are presented to illustrate the proposed channel model.

The remaining of this paper is organised as follows. Section 2 discusses the indoor propagation scenario, where the person is modelled by a cluster of synchronized moving scatterers. Then, the total power of the received RF signal is determined in Section 3. The derivation of the TVCTF of the received RF signals is presented in Section 4 together with the corresponding spectrogram. Section 5 presents some numerical results and Section 6 concludes the paper.

2 Scenario description

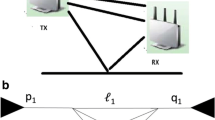
As illustrated in Fig. 1, we consider an example of a typical rectangular cuboid room. The room of length A, width B, and height H, is equipped with an N_T × N_R MIMO communication system. Here, N_T denotes the number of transmit antennas ${A_{j}^{T}}$ $(j=1,\dots ,N_{T})$ and N_R designates the number of receive antennas ${A_{i}^{R}}$ $(i=1,\dots ,N_{R})$. There are several stationary (fixed) objects (e.g., walls, furniture, and decoration items) and a single moving person. The moving person is modelled by a cluster of moving scatterers, where each single point scatterer () represents a segment of the human body such as head, shoulders, arms, etc.

Fig. 1

A single moving person (modelled by a cluster of moving scatterers) walks in a room equipped with a MIMO system and several fixed (stationary) objects [13]

The corresponding geometrical 3D channel model shown in Fig. 2 is the starting point for modelling the indoor propagation phenomenon. Each transmit (receive) antenna element ${A_{j}^{T}}$ (${A_{i}^{R}}$) is located at the fixed coordinates $({x_{j}^{T}},{y_{j}^{T}},{z_{j}^{T}})$ $(({x_{i}^{R}},{y_{i}^{R}},{z_{i}^{R}}))$, $j=1,2,\dots , N_{T}$ ($i=1,2,\dots , N_{R}$). Here, we assume the presence of LOS components.

Fig. 2

The 3D geometrical model for an N_T × N_R MIMO channel with fixed (∙) and moving scatterers () [13]

The fixed objects, which can be seen between the antennas ${A_{i}^{T}}$ and ${A_{j}^{R}}$, are modelled by K_ij fixed point scatterers (∙). These fixed scatterers are denoted by $S_{k_{ij}}^{F}$, $k_{ij}=1,2,\dots ,K_{ij}$. On the other hand, every body segment of the moving person is modelled by a single moving point scatterer (), denoted by ${S^{M}_{n}}$ ($n=1,2,\dots ,M$), which is located at the TV position (x_n(t),y_n(t),z_n(t)). Single-bounce scattering is assumed when modelling the fixed and moving scatterers.

3 Total received power

Together with considering TV path gains and TV path delays, the other novelty of this work is that the human body segments are assumed to play the role of relays, which redirect the transmitted radio wave. This is motivated by the fact that low-frequency electromagnetic fields have been shown to induce (in)perceivable electric currents in the human body. In turn, these “induced” currents can cause effects that vary from a dermal reaction to a tingling sensation depending on the frequency range [1, 8, 9, 22]. In other words, and as illustrated in Fig. 3, when the incoming (transmitted) RF signal () impinges on a body segment (moving scatterer ()), an electric current is induced () that retransmits the RF signal in all directions (). In the presence of these induced currents (), the human body segments (moving scatterers) play the role of relays.

Fig. 3

Behavior of human body segments in the presence of low-frequency electromagnetic fields

As can be seen in Fig. 3, the propagation phenomenon can be divided into three phases. In phase I, the RF signal is transmitted from the antenna element ${A_{j}^{T}}$ and received by the n th body segment ${S_{n}^{M}}$ with an instantaneous power $P^{T}_{jn}(t)$. In phase II, the electric current is induced, and the power of the received signal is amplified by a factor ${a_{n}^{2}}$, which describes the contribution of ${S_{n}^{M}}$ with respect to the entire human body and satisfies the condition ${\sum }_{n=1}^{M}{a_{n}^{2}}=1$. In relay systems, the quantity ${a_{n}^{2}}$ is commonly referred to as the “cross-section” parameter. Finally, in phase III, the signal is retransmitted from the scatterer ${S_{n}^{M}}$ and received at the receive antenna ${A_{i}^{R}}$ with an instantaneous power $P^{R}_{in}(t)$.

We denote by P_ijn(t) the total instantaneous received power of the RF signal transmitted from the j th transmit antenna ${A_{j}^{T}}$ and received at the i th receive antenna ${A_{i}^{R}}$ after being relayed by the n th moving scatterer ${S_{n}^{M}}$. Assuming that the body segments play the role of relays, the total TV received power P_ijn(t) can be expressed as the product of the TV powers $P^{T}_{jn}(t)$ and $P^{R}_{in}(t)$ and the cross-section parameter ${a_{n}^{2}}$, i.e.,

$$ \begin{array}{@{}rcl@{}} P_{ijn}(t)=P^{T}_{jn}(t)\times {a_{n}^{2}}\times P^{R}_{in}(t). \end{array} $$

(1)

The wave propagation phenomena in phases I and III are taking place in a free-space environment. Then, the free-space path loss model [11, 29] can be employed to express the TV received powers $P^{T}_{jn}(t)$ and $P^{R}_{in}(t)$ as $P^{R}_{in}(t)=C\left [ D_{jn}^{T}(t)\right ]^{-\gamma }$ and $P^{R}_{in}(t)=C\left [D_{in}^{R}(t)\right ]^{-\gamma }$, respectively. Here, the quantity C depends on the transmit/receive antenna gain, the transmission power, and the wavelength [29], while γ is the path loss exponent which is equal to 2 in free space and between 1.6 and 1.8 in indoor environments. Moreover, the quantity $D_{jn}^{T}(t)$ ($D_{in}^{R}(t)$) represents the TV distance between the j th (i th) transmit (receive) antenna ${A_{j}^{T}}$ (${A_{i}^{R}}$) and the n th moving scatterer ${S_{n}^{M}}$, $n=1,2,\dots ,M$. According to Fig. 2, the TV distances $D_{jn}^{T}(t)$ and $D_{in}^{R}(t)$ can be expressed as

$$ \begin{array}{@{}rcl@{}} D_{jn}^{T}(t){\kern-.07 cm}&=&{\kern-.15 cm} \left[ {\kern-.05 cm}\left( x_{n}(t) {\kern-.05 cm}-{\kern-.05 cm}{x_{j}^{T}} \right)^{{\kern-.05 cm}2}{\kern-.09 cm}+{\kern-.07 cm}\left( y_{n}(t) {\kern-.05 cm}-{\kern-.05 cm}{y_{j}^{T}} \right)^{{\kern-.05 cm}2} {\kern-.09 cm}+{\kern-.07 cm}\left( {\kern-.03 cm}z_{n}(t) {\kern-.05 cm}-{\kern-.05 cm}{z_{j}^{T}} \right)^{{\kern-.05 cm}2}{\kern-.05 cm} \right]^{{\kern-.05 cm}\frac{1}{2}} \end{array} $$

(2)

and

$$ \begin{array}{@{}rcl@{}} D_{in}^{R}(t)&=& \left[ \left( {x_{i}^{R}}-x_{n}(t) \right)^{2}+\left( {y_{i}^{R}}-y_{n}(t) \right)^{2} +\left( {z_{i}^{R}}-z_{n}(t) \right)^{2} \right]^{\frac{1}{2}} \end{array} $$

(3)

respectively. Finally, inserting the expressions of $P^{T}_{jn}(t)$ and $P^{R}_{in}(t)$ in Eq. 1 yields the following expression for the instantaneous received power P_ijn(t)

$$ \begin{array}{@{}rcl@{}} P^{R}_{in}(t)={a_{n}^{2}}C^{2}\left[D_{in}^{R}(t)\times D_{jn}(t)^{T}\right]^{-\gamma}. \end{array} $$

(4)

The total instantaneous power P_ijn(t) represents the power of the signal transmitted by the j th transmit antenna ${A_{j}^{T}}$ and received at the i th receive antenna ${A_{i}^{R}}$ after being relayed by the n th moving scatterer (body segment) ${S_{n}^{M}}$.

In the following section, the result in Eq. 4 will be employed to express the TV path gains of the moving scatterers.

4 3D non-stationary channel model

According to Fig. 2, the TVCTF H_ij(f^′,t) of the sub-channel between the j th transmit antenna ${A_{j}^{T}}$ and the i th receive antenna ${A_{i}^{R}}$ can be expressed as

$$ \begin{array}{@{}rcl@{}} H_{ij}(f^{\prime},t)&=& \sum \limits_{n=1}^{M} {\kern-.05 cm} c_{ijn}(t)\exp{\kern-.05 cm}\left( j{\kern-.05 cm}\left( \theta_{ijn}{\kern-.05 cm}-{\kern-.05 cm}2\pi (f_{c}+f^{\prime})\tau_{ijn}(t){\kern-.05 cm}\right){\kern-.05 cm} \right) {\kern-.05 cm} \\ &&+\sum \limits_{k=0}^{K_{ij}} {\kern-.05 cm}c_{k_{ij}}{\kern-.05 cm} \exp{\kern-.05 cm}\left( {\kern-.05 cm}j{\kern-.05 cm}\left( {\kern-.05 cm} \theta_{k_{ij}}{\kern-.08 cm}-{\kern-.05 cm}2\pi (f_{c}+f^{\prime})\tau_{k_{ij}} {\kern-.05 cm}\right) {\kern-.07 cm}\right){\kern-.05 cm} \end{array} $$

(5)

where f_c is the center frequency and t ($f^{\prime }$) refers to the time (frequency) domain. The first part of Eq. 5 describes the effects introduced by the moving point scatterers (modelling the centres of mass of the major segments of the human body). Here, the quantities c_ijn(t) and τ_ijn(t) are the TV path gain and TV path delay of the n th moving scatterer ${S_{n}^{M}}$ ($n=1,2,\dots ,M$), respectively. These parameters are given by

$$ \begin{array}{@{}rcl@{}} c_{ijn}(t)=\sqrt{P_{in}^{R}}=a_{n} C \left[D_{in}^{R}(t)\times D_{jn}^{T}(t)\right]^{-\gamma/2} \end{array} $$

(6)

and

$$ \begin{array}{@{}rcl@{}} \tau_{ijn}(t) = \frac{D_{jn}^{T}(t)+D_{in}^{R}(t)}{c_{0}} \end{array} $$

(7)

respectively, where the quantity c₀ denotes the speed of light. It is worth mentioning that the instantaneous Doppler frequencies $f_{ijn}(t,f^{\prime })$ of the n th moving scatterer ${S_{n}^{M}}$ ($n=1,2,\dots ,M$) can be obtained from the TV delays τ_ijn(t) according to the fundamental relationship $f_{ijn}(t)=-(f_{c}+f^{\prime })\tau _{ijn}(t)$ [27]. In the Appendix, mathematical details are presented showing that for short time intervals [t₀ − δt/2,t₀ + δt/2] centered at the time instant t₀ and of duration δt, the TV path gains c_ijn(t) can be assumed constant and expressed according to Eq. 24. The initial phases of the channel, denoted by 𝜃_ijn $(j=1,2,\dots ,N_{T},$ $i=1,2,\dots ,N_{R}$, and $n=1,2,\dots ,M)$, are modelled by independent and uniformly distributed (i.i.d.) random variables which are uniformly distributed over the interval [0,2π).

The second sum of Eq. 5 models the multipath propagation effect resulting from the fixed scatterers (objects) $S_{k_{ij}}^{F}$, $k_{ij}=1,2,\dots ,K_{ij}$. Each fixed scatterer $S_{k_{ij}}$ is described by a constant path gain $c_{k_{ij}}$, a constant path delay $\tau ^{\prime }_{k_{ij}}$, and a constant phase $\theta _{k_{ij}}$, $k=1,2,\dots , K_{ij}$.

The LOS component, which does not experience any Doppler effects, can be modelled by a fixed scatterer ${S_{0}^{F}}$ (k_ij = 0), with a constant path gain $c_{0_{ij}}$ and a constant delay $\tau _{0_{ij}}$. The phases $\theta _{k_{ij}}$ are modelled by i.i.d. random variables with a uniform distribution over the interval [0,2π). The aim of the present work is to investigate the impact of the TV path gains c_ijn(t) on the channel characteristics independently of the individual contributions of the fixed scatterers $S_{k_{ij}}$. Therefore, for simplicity, the overall effect of the K_ij fixed scatterers, i.e., the second sum of Eq. 5, is replaced by a single complex term $c_{ijF}(f^{\prime })\exp [j(\vartheta _{ijF}(f^{\prime }))]$, with a magnitude $c_{ijF}(f^{\prime })$ and an argument $\vartheta _{ijF}(f^{\prime })$. The quantities $c_{ijF}(f^{\prime })$ and $\vartheta _{ijF}(f^{\prime })$, $j=1,2,\dots ,N_{T},$ and $i=1,2,\dots ,N_{R}$, are expressed in terms of the fixed path gains $c_{k_{ij}}$, phases $\theta _{k_{ij}}$, and path delays $\tau _{k_{ij}}$ as

$$ \begin{array}{@{}rcl@{}} c_{ijF}(f^{\prime})&=&\bigg\{\bigg[\sum \limits_{k=0}^{K_{ij}}c_{k_{ij}}\cos(\theta_{k_{ij}}-2\pi f^{\prime}\tau '_{k_{ij}})\bigg]^{2} \\ &&+\bigg[\sum \limits_{k=0}^{K_{ij}}c_{k_{ij}}\sin(\theta_{k_{ij}}-2\pi f^{\prime}\tau '_{k_{ij}})\bigg]^{2}\bigg\}^{1/2} \end{array} $$

(8)

and

$$ \begin{array}{@{}rcl@{}} \vartheta_{ijF}(f^{\prime})&=&\text{atan}2 \bigg(\sum \limits_{k=0}^{K_{ij}}c_{k_{ij}}\sin(\theta_{k_{ij}}-2\pi f^{\prime}\tau '_{k_{ij}}), \\ && \sum \limits_{k=0}^{K_{ij}}c_{k_{ij}}\cos(\theta_{k_{ij}}-2\pi f^{\prime}\tau '_{k_{ij}})\bigg) \end{array} $$

(9)

$$ \begin{array}{@{}rcl@{}} S_{ij}^{(c)}(f^{\prime},f,t)&=&\frac{2}{\sigma_w\pi}\Bigg[\sum \limits_{n=1}^{M-1}\sum \limits_{l=n+1}^M c_{ijn}(t)c_{ijl}(t)\mathbf{Re}\bigg\{ G\left( f,-(f_c+f^{\prime})\dot \tau _n(t),\sigma_{ijn,2}^2(f^{\prime},t)\right)G^{*}\left( f,-(f_c+f^{\prime})\dot \tau _l(t),\sigma_{ijl,2}^2(f^{\prime},t)\right) \\ &&\cdot \exp \left( j\left( \theta_{ijn}-\theta_{ijl}-2\pi \left( f_c+f^{\prime} \right)\left( \tau_{ijn}(t)-\tau_{ijl}(t) \right) \right) \right)\bigg\} +\sum \limits_{n=1}^M c_{ijn}(t)c_{ijF}(f^{\prime})\hspace{.03 cm}G\left( f,0,\sigma_{F}^2\right) \\ &&\cdot \mathbf{Re}\bigg\{ G\left( f,-(f_c+f^{\prime})\dot \tau _n(t),\sigma_{ijn,2}^2(f^{\prime},t)\right) \exp \left( j\left( \theta_{ijn}-\vartheta_{ijF}(f^{\prime})-2\pi \left( f_c+f^{\prime} \right)\tau_{ijn}(t) \right) \right)\bigg\}\Bigg]. \end{array} $$

(10)

respectively, where atan2(⋅) is the inverse tangent function and returns a value in the interval [−π,π[ [26].

To visualize the influence of introducing TV path gains to describe the motion of the person (modelled by a cluster of synchronized moving scatterers), we consider the spectrogram $S_{ij}(f^{\prime },f,t)$ of the TVCTF $H_{ij}(f^{\prime },t)$ using a Gaussian window [3, 6].

According to [3], the spectrogram $S_{ij}(f^{\prime },f,t)$ of the TV- CTF $H_{ij}(f^{\prime },t)$ is given by the absolute square of the short-time Fourier transform $X_{ij}(f^{\prime },f,t)$ of the product of the TVCTF $H_{ij}(f^{\prime },t)$ and a sliding Gaussian window h(t) of spread σ_w. Therefore, the spectrogram $S_{ij}(f^{\prime },f,t)$ of the TVCTF $H_{ij}(f^{\prime },t)$ can be expressed as

$$ \begin{array}{@{}rcl@{}} S_{ij}(f^{\prime} , f , t)&=& \left|X_{ij}(f^{\prime},f,t)\right|^2 \\ &=& \Bigg|\int \limits_{-\infty}^{\infty} H_{ij}(f^{\prime} ,t^{\prime})h(t^{\prime} - t)\exp \left( -2\pi ft^{\prime}\right)\hspace{.0 cm} dt^{\prime}\Bigg|^2 \end{array} $$

(11)

where the Gaussian window is given by $h(t)=1/(\sqrt {\sigma _w \sqrt {\pi }})$ $\exp (-t^2/(2\sigma _w^2))$ is a real-valued, positive, and even function and has a normalized energy, i,e., ${\int \limits }_{-\infty }^{\infty } h(t)dt=1$. In Eq. 11, the quantity $H_{ij}(f^{\prime }\hspace {-.07 cm} ,t^{\prime })h(t^{\prime }\hspace {-.07 cm} -\hspace {-.07 cm} t)$ is referred to as the short-time TVCTF [5, Eq. (2.3.1)], in which $t^{\prime }$ (t) is the running (local) time.

As described in the appendix, for short time intervals of duration δt, the TV path gain c_ijn(t) corresponding to the n th moving scatterers $S_n^M$ ($n=1,2,\dots , M$) can be assumed to be constant and are expressed as in Eeq. 24. In this case, and by following the same steps as in [4, Section IV], a closed-form approximate solution to the spectrogram can be determined as

$$ \begin{array}{@{}rcl@{}} S_{ij}(f^{\prime},f,t)=S_{ij}^{(a)}(f^{\prime},f,t)+S_{ij}^{(c)}(f^{\prime},f,t). \end{array} $$

(12)

Here, the auto-term $S_{ij}^{(a)}(f^{\prime },f,t)$ is expressed as

$$ \begin{array}{@{}rcl@{}} S_{ij}^{(a)}(f^{\prime},f,t)& = & \sum \limits_{n=1}^M c_{ijn}^2(t) G\left( f,-(f_c+f^{\prime})\dot \tau _n(t),\sigma_{n,1}^2(f^{\prime},t)\right) \\ &&+c_{ijF}^2(f^{\prime})G\left( f,0,\frac{\sigma_F^2 }{2}\right) \end{array} $$

(13)

while the cross-term $S_{ij}^{(c)}(f,t)$ is given in Eq. 10 (see the top of this page), where the operators Re{⋅} and (⋅)^∗ return the real part and the complex conjugate of a complex number, respectively. In Eqs. 13 and 10, $\sigma _F^2=1/(2\pi \sigma _w)^2$, $\sigma _{ijn,1}^2(f^{\prime },t)=[\sigma _F^2+\sigma _w^2k_{ijn}^2(f^{\prime },t)]/2$, and $\sigma _{ijn,2}^2(f^{\prime },t)=\sigma _F^2-j k_{ijn}(f^{\prime },t)/$ (2π), in which $k_{ijn}(f^{\prime },t)=-(f_c+f^{\prime })\ddot {\tau }(t)$, with $\ddot {\tau }(t)$ being the second-order time derivative of the TV delays τ_ijn(t). By exploiting the results reported in [6, Eqs. (20) and (21)], the TV MDS $B_{ij}^{(1)}(f^{\prime },t)$ and the TV DS $B_{ij}^{(2)}(f^{\prime },t)$ can be determined from the spectrogram $S_{ij}(f^{\prime },f,t)$ as

$$ \begin{array}{@{}rcl@{}} B_{ij}^{(1)}(f^{\prime},t)=\frac{\int \limits_{-\infty}^{\infty} fS_{ij}(f^{\prime},f,t)\hspace{.05 cm}df}{\int \limits_{-\infty}^{\infty} S_{ij}(f^{\prime},f,t)\hspace{.05 cm}df} \end{array} $$

(14)

and

$$ \begin{array}{@{}rcl@{}} B_{ij}^{(2)}(f^{\prime},t)&=&\sqrt{\frac{\int \limits_{-\infty}^{\infty} f^2S_{ij}(f^{\prime},f,t)\hspace{.05 cm}df}{\int \limits_{-\infty}^{\infty} S_{ij}(f^{\prime},f,t)\hspace{.05 cm}df}-\left( B_{ij}^{(1)}(f^{\prime},t) \right)^2} \end{array} $$

(15)

respectively.

5 Simulation results

In this section, we explore the propagation scenario described in Section 4 by studying the effect of introducing the concept of TV path gains on the spectrogram $S_{ij}(f^{\prime },f,t)$, the TV MDS $B_{ij}^{(1)}(f^{\prime },t)$, and the TV DS $B_{ij}^{(2)}(f^{\prime },t)$ of the TVCTF $H_{ij}(f^{\prime },t)$ of the received RF signal.

As illustrated in Fig. 4, we consider a room of length A = 5 m, width B = 10 m, and height H = 2.4 m. Here, the transmitter T_X and the receiver R_X consist of three transmit antennas $A_j^T$ (j = 1,2,3) and three receive antennas $A_i^R$ (i = 1,2,3), respectively. All omnidirectional antenna elements are mounted in pairs $(A_i^T,A_i^R)$ on the ceiling, where the elements $A_i^T$ and $A_i^R$ are separated by 0.5 m. The fixed coordinates of the antennas $A_1^T$, $A_2^T$, $A_3^T$, $A_1^R$, $A_2^R$, and $A_3^R$ are (0, − 0.25, 2.4), (2.5, -0.25, 2.4), (5, -0.25, 2.4), (0, 0.25, 2.4), (2.5, 0.25, 2.4), and (5, 0.25, 2.4), respectively.

Fig. 4

3D simulation scenario with M = 6 moving scatterers and a 3 × 3 MIMO system

A single walking person is modelled by 6 moving point scatterers, i.e., M = 6. The scatterers represent the centre of mass of the major segments of the human body, namely, the head, ankles, wrists, and trunk (see Fig. 4). The selection of these specific body segments has been made in accordance with the analysis conducted in [16] and [19]. The authors of [16] and [19] studied the individual contribution of each segment to the overall human motor activities. The motion of the person is observed for a period of 10 s. The trajectories of the different moving scatterers (body segments) are simulated according to [3, Section V, Eqs. (24)−(29)]. As shown in Fig. 4, the person is first at one end of the room under antennas ${A_{1}^{T}}$ and ${A_{1}^{R}}$ and then moves to the other end of the room under antennas ${A_{3}^{T}}$ and ${A_{3}^{R}}$. The parameter C and the path loss component γ are set to 1000 and 1.6, respectively. The center frequency f_c was chosen to be 5.9 GHz. For clarity and without loss of generality, the figures presented are obtained for $f^{\prime }=0$ Hz. For comparison, the results for constant path gains and TV path gains are contrasted. For the case where the path gains of the moving scatterers are constant, the path gains are obtained by c_ijn(t) = c_ijn = 1. The path gains of the fixed scatterers are given by $c_{k_{ij}}=\sqrt {2/K_{ij}}$. The contribution of the fixed scatterers $S_{k_{ij}}$ is removed by applying a high-pass filtering. For the remaining parameters as well as the scenario with constant path gains, we consider the same simulation parameters as described in [3, Section V].

Figures 5, 6, and 7 depict the spectrograms S₁₁(0,f,t), S₂₂(0,f,t), and S₃₃(0,f,t), respectively. For comparison, we included here the spectrograms with constant path gains (Figs. 5a, 6a, and 7a) and the spectrograms with TV path gains (Figs. 5b, 6b, and 7b). From Figs. 5a, 6a, and 7a, it can be observed that the average power of the spectrogram, which is proportional to the sum of the squared constant gains ${\sum }_{n=1}^{M} c_{ijn}^{2}$, remains constant along the observation time of 10 s. The yellow parts of the spectrogram correspond to the superposition of the Gaussian function G(a,b,c) (see Eqs. 13 and 10) and provide neither information about the motion of the body segments (moving point scatterers) nor the relative position of the person with respect to the transmit/receive antenna elements. This contrasts with Figs. 5b, 6b, and 7b, where the TV average power, which is proportional to the sum of the squared TV gains ${\sum }_{n=1}^{M} c_{ijn}^{2}(t)$, of the moving scatterers changes with time t. This stems from the fact that the TV path gains c_ijn(t) are inversely proportional to the product of the TV distances $D_{1n}^{T}(t)$ and $D_{1n}^{R}(t)$ to the power of γ/2. For example, in Fig. 5b, the person (modelled by a cluster of M = 6 point scatterers) is moving away from the antenna pair $({A_{1}^{T}},{A_{1}^{R}})$. Here, the TV distances $D_{1n}^{T}(t)$ and $D_{1n}^{R}(t)$ increase with respect to time t, which, in turn, results in decreasing TV average powers $c_{ijn}^{2}(t)$. In Fig. 6b, the person starts moving towards the antennas ${A_{2}^{T}}$ and ${A_{2}^{R}}$ and then moves away from this antennas pair. In this case, the TV power of the spectrogram increases until the person is located below $({A_{2}^{T}},{A_{2}^{R}})$ (at approximately t = 4.5 s) and then begins to decrease until the person reaches its final position. Finally, Fig. 7b depicts the spectrogram S₃₃(0,f,t) of the TVCTF H₃₃(0,t), where the person walks towards the antennas ${A_{3}^{T}}$ and ${A_{3}^{R}}$.

Fig. 5

Spectrogram S₁₁(f,t) with a constant path gains and b TV path gains

Fig. 6

Spectrogram S₂₂(0,f,t) with a constant gains and b TV path gains

Fig. 7

Spectrogram S₃₃(f,t) with a constant path gains and b TV path gains

In Figs. 5a, 6a, and 7a, the constant power of the spectrograms can be physically interpreted by the fact that the range of the RF system is infinite. This means that the motion of the person can be observed regardless of how far or how close it is positioned with respect to the transmit and receive antennas. In reality, this is not the case, especially for indoor wireless communications. By introducing instantaneous path gains for the moving scatterers ${S_{n}^{M}}$ ($n=1,2,\dots ,M$) (see Figs. 5b, 6b, and 7b), the TV behavior of the power of the spectrograms can be explained by the fact that by moving away from (towards) the T_X antennas and the R_X antennas, the person is walking out of (into) the range of the RF system. This is the behavior observed in practice, which confirms the use of TV path gains to describe the motion of the different human body segments.

To further investigate the effect of introducing TV path gains to describe the moving scatterers S_n, $n=1,2,\dots ,M$, in the expression of the TVCTF $H_{ij}(f^{\prime },t)$, on the Doppler characteristics of the fading channel model, we study the TV MDS $B_{ij}^{(1)}(0,t)$ and TV DS $B_{ij}^{(2)}(0,t)$. Let us denote by T₁, T₂, and T₃ the time intervals during which the person in moving close to the antenna elements $({A_{1}^{T}},{A_{1}^{R}})$, $({A_{2}^{T}},{A_{2}^{R}})$, and $({A_{3}^{T}},{A_{3}^{R}})$, respectively. We present in Fig. 9a, b, and c a comparison of the TV MDSs $B_{ij}^{(1)}(0,t)$ using constant path gains and TV path gains for the different pairs of antennas $({A_{1}^{T}},{A_{1}^{R}})$, $({A_{2}^{T}},{A_{2}^{R}})$, and $({A_{3}^{T}},{A_{3}^{R}})$, respectively. The TV MDSs $B_{ij}^{(1)}(0,t)$ were numerically computed according to Eq. 14. As can be seen, the introduction of TV path gains only slightly influences the trend of the TV MDS. However, it strongly affects the values of $B_{ij}^{(1)}(0,t)$, especially when the person is not in close proximity to the antenna pairs, i.e., outside the intervals T₁, T₂, and T₃. The TV DSs $B_{ij}^{(2)}(0,t)$ were computed from the spectrograms S_ij(0,f,t) utilizing (15). It is shown in Fig. 8a, b, and c that the consideration of the TV path gains c_ij(t) influences both the trend and the values of the TV DSs $B_{ij}^{(2)}(0,t)$. We also observe that the TV DSs $B_{1}^{(2)}(0,t)$, $B_{22}^{(2)}(0,t)$, and $B_{33}^{(2)}(0,t)$ remain almost constant during the time durations T₁, T₂, and T₃, receptively. This means that the Doppler frequencies do not deviate much from the MDS $B_{ij}^{(1)}(0,t)$. In other words, during T₁, T₂, and T₃ (see Fig. 8a, b, and c), the moving scatterers (segments) modelling the human body can be replaced by a single moving scatterer, whose corresponding Doppler frequency can be approximated by the TV MDS $B_{11}^{(1)}(0,t)|_{t\in T_{1}}$, $B_{22}^{(1)}(0,t)|_{t\in T_{2}}$, and $B_{33}^{(1)}(0,t)|_{t\in T_{3}}$ (see Fig. 9). This can be explained by the fact that as the person gets closer to the antennas, the main body segment describing the motion of the person is the head. All other body parts are obstructed because the antennas are attached to the ceiling.

Fig. 8

TV mean Doppler shift (MDS) $B_{ij}^{(1)}(0,t)$ observed between the antennas a ${A_{1}^{T}}$ and ${A_{1}^{R}}$, b ${A_{2}^{T}}$ and ${A_{2}^{R}}$, and c ${A_{3}^{T}}$ and ${A_{3}^{R}}$

Fig. 9

TV Doppler spread (DS) $B_{ij}^{(2)}(0,t)$ observed between the antennas a ${A_{1}^{T}}$ and ${A_{1}^{R}}$, b ${A_{2}^{T}}$ and ${A_{2}^{R}}$, and c ${A_{3}^{T}}$ and ${A_{3}^{R}}$

6 Conclusion

This paper introduces a new 3D non-stationary indoor MIMO channel model, in which a single person is moving. The different segments making up the human body are modelled by a cluster of synchronized moving point scatterers. Taking advantage from the fact that an electric current is induced in the human body when it is exposed to low-frequency electromagnetic fields, we assume that the human body segments (moving scatterers) play the role of moving relays, which redirect the transmit radio signal. We present an expression for the TVCTF of the received RF signal with TV path gains and TV path delays. The TV path gain of each multipath component resulting from the moving person (moving scatterers) is modelled by means of the free-space path loss model. The impact of the TV distances between the moving person (scatterer) and the transmit and receive antenna elements on the spectrogram, the TV MDS, and the TV DS of the TVCTF is analyzed. It is concluded that this new expression for the TV path gains has two main advantages. First, it allows to detect the relative position of the person with respect to the transmit and receive antennas. Second, it gives insight into the range of the considered RF system. In fact, the closer the person is to the antennas, the higher is the power of the spectrogram. Moreover, when the person is out of range of the transmit/receive antennas (far away), the motion of the person cannot be clearly visualized in the spectrogram. The obtained analytical results have been illustrated by computer simulations.

$$ \begin{array}{@{}rcl@{}} c_{ijn}(t)&\approx& \frac{a_n C}{\left[D_{jn}^T(t_0)D_{in}^R(t_0)\right]^{\gamma}} \left\lbrace {\kern-0.05 cm}1{\kern-0.05 cm}-{\kern-0.05 cm} \frac{\gamma (t-t_0)}{2}\Bigg[{\kern-0.05 cm}\left( x_n(t)x_n^{\prime}(t_0)+y_n(t)y_n^{\prime}(t_0)+z_n(t)z_n^{\prime}(t_0)\right){\kern-0.07 cm}\left( {\kern-0.07 cm}\left( D_{jn}^T(t_0)\right)^{-2}{\kern-0.07 cm}+{\kern-0.07 cm}\left( D_{in}^R(t_0)\right)^{-2}\right) \right. \\ && \left. - \left( x_n^{\prime}(t_0)+y_n^{\prime}(t_0)+y_n^{\prime}(t_0) \right) \left( \frac{x_j^T+y_j^T+z_j^T}{\left( D_{jn}^T(t_0)\right)^2}+ \frac{x_i^R+y_i^R+z_i^R}{\left( D_{in}^R(t_0)\right)^2}\right){\kern-0.07 cm} \Bigg] {\kern-0.07 cm}\right\rbrace. \end{array} $$

(16)

Appendix

This appendix explains the mathematical manipulations leading to the approximate solutions to the TV path gains c_ijn(t) in (6). To do this, we apply a Taylor series expansion to the TV displacements x_n(t), y_n(t), and z_n(t) of the n th moving scatterer (modelling the n th body segment). Since the objective is to justify the assumption that the TV path gains c_ijn(t) can be assumed to be constant for short periods of time, the following mathematical calculations will be conducted using a first-order Taylor expansion.

In other words, for short time intervals centered at the time instant t₀ and of duration δt, the TV positions x_n(t), y_n(t), and z_n(t) can be written as

$$ \begin{array}{@{}rcl@{}} x_{n}(t)&\approx& x_{n}(t_{0})+(t-t_{0})x_{n}^{\prime}(t_{0}) \end{array} $$

(17)

$$ \begin{array}{@{}rcl@{}} y_{n}(t)&\approx& y_{n}(t_{0})+(t-t_{0})y_{n}^{\prime}(t_{0}) \end{array} $$

(18)

and

$$ \begin{array}{@{}rcl@{}} z_{n}(t)&\approx& z_{n}(t_{0})+(t-t_{0})z_{n}^{\prime}(t_{0}) \end{array} $$

(19)

for t ∈ [t₀ − δt/2,t₀ + δt/2], respectively. In Eqs. 17–19, $u^{\prime }(t)$ refers to the time derivative of the function u(t). Then, the TV distances $D_{jn}^{T}(t)$ and $D_{in}^{R}(t)$ can be approximated by

$$ \begin{array}{@{}rcl@{}} D_{jn}^{T}(t){\kern-0.1 cm}&\approx&{\kern-0.13 cm}\bigg[{\kern-0.1 cm}\left( {\kern-0.03 cm}D_{jn}^{T}(t_{0}){\kern-0.03 cm}\right)^{2}{\kern-0.05 cm}+{\kern-0.05 cm} (t{\kern-0.03 cm}-{\kern-0.03 cm}t_{0})\left( x_{n}(t)x_{n}^{\prime}(t_{0}){\kern-0.05 cm}+{\kern-0.05 cm}y_{n}(t)y_{n}^{\prime}(t_{0})\right. \\ &&{\kern-1.2 cm} \left. +{\kern-0.03 cm}z_{n}(t)z_{n}^{\prime}(t_{0}){\kern-0.05 cm}-{\kern-0.05 cm}x_{n}^{\prime}(t_{0}){x_{j}^{T}}{\kern-0.05 cm}-{\kern-0.05 cm}y_{n}^{\prime}(t_{0}){y_{j}^{T}}{\kern-0.05 cm}-{\kern-0.05 cm}y_{n}^{\prime}(t_{0}){y_{j}^{T}}\right){\kern-0.1 cm}\bigg]^{1/2} \end{array} $$

(20)

and

$$ \begin{array}{@{}rcl@{}} D_{in}^{R}(t)&\approx&\bigg[\left( D_{in}^{R}(t_{0})\right)^{2}+ (t-t_{0})\left( x_{n}(t)x_{n}^{\prime}(t_{0})+y_{n}(t)y_{n}^{\prime}(t_{0})\right.\\ &&\left.+z_{n}(t)z_{n}^{\prime}(t_{0}) -x_{n}^{\prime}(t_{0}){x_{i}^{R}}-y_{n}^{\prime}(t_{0}){y_{i}^{R}}-y_{n}^{\prime}(t_{0}){y_{i}^{R}}\right)\bigg]^{1/2} \end{array} $$

(21)

for t ∈ [t₀ − δt/2,t₀ + δt/2], respectively. By placing the transmit antennas ${A_{j}^{T}}$ and receive antennas ${A_{i}^{R}}$ in such way that the distances $D_{jn}^{T}(t_{0})$ and $D_{in}^{R}(t_{0})$ are larger than 1 (e.g., on the ceiling or in the corners of the room, which ensures maximum coverage) and using (1 + x)^α ≈ 1 + αx, the TV quantities $\left (D_{jn}^{T}(t)\right )^{-\gamma }$ and $\left (D_{in}^{R}(t)\right )^{-\gamma }$ can be obtained as

$$ \begin{array}{@{}rcl@{}} \left( {\kern-0.03 cm}D_{jn}^{T}(t){\kern-0.03 cm}\right)^{{\kern-0.05 cm}-\gamma}{\kern-.1 cm}&\approx&{\kern-0.1 cm} \left( {\kern-0.03 cm}D_{jn}^{T}(t_{0}){\kern-0.03 cm}\right)^{{\kern-0.05 cm}-\gamma}{\kern-0.1 cm}\bigg[1{\kern-0.05 cm}-{\kern-0.05 cm}\frac{\gamma (t-t_{0})}{2\left( D_{jn}^{T}(t_{0})\right)^{2}}\left( x_{n}(t)x_{n}^{\prime}(t_{0})\right.\\ &&{\kern-.1 cm}\left.+y_{n}(t)y_{n}^{\prime}(t_{0}){\kern-0.05 cm}+{\kern-0.05 cm}z_{n}(t)z_{n}^{\prime}(t_{0}){\kern-0.05 cm}-{\kern-0.05 cm}x_{n}^{\prime}(t_{0}){x_{j}^{T}}{\kern-0.05 cm}\right.\\ &&{\kern-.1 cm}\left.-y_{n}^{\prime}(t_{0}){y_{j}^{T}}-y_{n}^{\prime}(t_{0}){y_{j}^{T}}\right)\bigg] \end{array} $$

(22)

and

$$ \begin{array}{@{}rcl@{}} \left( D_{in}^{R}(t)\right)^{-\gamma}&\approx& \left( D_{in}^{R}(t_{0})\right)^{-\gamma}\bigg[1-\frac{\gamma (t-t_{0})}{2\left( D_{in}^{R}(t_{0})\right)^{2}}\left( x_{n}(t)x_{n}^{\prime}(t_{0})\right.\\ && \left.+y_{n}(t)y_{n}^{\prime}(t_{0})+z_{n}(t)z_{n}^{\prime}(t_{0})-x_{n}^{\prime}(t_{0}){x_{i}^{R}}\right.\\ && \left.-y_{n}^{\prime}(t_{0}){y_{i}^{R}}-y_{n}^{\prime}(t_{0}){y_{i}^{R}}\right)\bigg] \end{array} $$

(23)

for t ∈ [t₀ − δt/2,t₀ − δt/2], respectively. Inserting (22) and (23) in (6) yields the first-order approximate solution to the TV path gains c_ijn(t) in Eq. 16 [see the top of this page], for time instance t in the interval [t₀ − δt/2,t₀ − δt/2].

Profiting from the facts that |t − t₀| < δt, $D_{jn}^{R}(t_{0})\gg 1$, $D_{in}^{T}(t_{0})\gg 1$, and γ/2 ≤ 1, the second term of Eq. 16 can be neglected, which in turn results in the following expression for the TV path gains c_ijn(t) for short time intervals of duration δt

$$ \begin{array}{@{}rcl@{}} c_{ijn}(t)&\approx& a_{n} C \left( D_{jn}^{T}(t_{0})D_{in}^{R}(t_{0})\right)^{-\gamma}. \end{array} $$

(24)

For simplicity, we have considered a first-order Taylor expansion for the TV displacements to show that the TV path gains c_ijn(t) can be assumed to be constant for short time intervals of duration δt. In case of the higher-order Taylor expansion of x_n(t), y_n(t), and z_n(t), it can be similarly argued that higher-order terms can be neglected in the approximation of the TV path gains c_ijn(t).