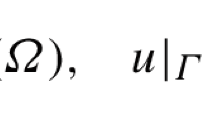Abstract
We study the geometry of domains in complete metric measure spaces equipped with a doubling measure supporting a 1-Poincaré inequality. We propose a notion of domain with boundary of positive mean curvature and prove that, for such domains, there is always a solution to the Dirichlet problem for least gradients with continuous boundary data. Here least gradient is defined as minimizing total variation (in the sense of BV functions), and boundary conditions are satisfied in the sense that the boundary trace of the solution exists and agrees with the given boundary data. This extends the result of Sternberg et al. (J Reine Angew Math 430:35–60, 1992) to the non-smooth setting. Via counterexamples, we also show that uniqueness of solutions and existence of continuous solutions can fail, even in the weighted Euclidean setting with Lipschitz weights.













Similar content being viewed by others
References
Ambrosio, L.: Fine properties of sets of finite perimeter in doubling metric measure spaces. Set Valued Anal. 10(2–3), 111–128 (2002)
Ambrosio, L., Di Marino, S.: Equivalent definitions of BV space and of total variation on metric measure spaces. J. Funct. Anal. 266(7), 4150–4188 (2014)
Ambrosio, L., Miranda Jr., M., Pallara, D.: Special functions of bounded variation in doubling metric measure spaces. In: Calculus of Variations: Topics from the Mathematical Heritage of E. De Giorgi, 1–45, Quad. Mat. 14 Department of Mathematics, Seconda Univ. Napoli, Caserta (2004)
Anzellotti, G., Giaquinta, M.: BV functions and traces. Rend. Sem. Mat. Univ. Padova 60, 1–21 (1978)
Barozzi, E., Massari, U.: Regularity of minimal boundaries with obstacles. Rend. Sem. Mat. Univ. Padova 66, 129–135 (1982)
Björn, A., Björn, J.: Nonlinear Potential Theory on Metric Spaces, EMS Tracts in Mathematics, 17. European Mathematical Society (EMS), Zürich (2011)
Bombieri, E., De Giorgi, E., Giusti, E.: Minimal cones and the Bernstein problem. Invent. Math. 7, 243–268 (1969)
Bouchitté, G., Dal Maso, E.: Integral representation and relaxation of convex local functionals on BV(\(\Omega \)). Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 20(4), 483–533 (1993)
Camfield, C.: Comparison of BV norms in weighted euclidean spaces and metric measure spaces Ph.D. thesis, University of Cincinnati (2008). http://rave.ohiolink.edu/etdc/view?acc_num=ucin1211551579
Giaquinta, M., Souček, J.: Esistenza per il problema dell’area e controesempio di Bernstein. Boll. Un. Mat. Ital. (4) 9, 807–817 (1974)
Giusti, E.: Boundary value problems for non-parametric surfaces of prescribed mean curvature. Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 3(3), 501–548 (1976)
Giusti, E.: Minimal Surfaces and Functions of Bounded Variation. Monographs in Mathematics, vol. 80, p. xii+240. Birkhäuser Verlag, Basel (1984)
Hajłasz, P., Koskela, P.: Sobolev met Poincaré. Memoirs AMS 145, No. 688 (2000)
Hakkarainen, H., Korte, R., Lahti, P., Shanmugalingam, N.: Stability and continuity of functions of least gradient. Anal. Geom. Metr. Spaces 3(1), 123–139 (2015)
Heinonen, J.: Lectures on analysis on metric spaces, Universitext, p. x+141. Springer, New York (2001)
Heinonen, J., Koskela, P., Shanmugalingam, N., Tyson, J.: Sobolev spaces on metric measure spaces: an approach based on upper gradients. In: New Mathematical Monographs, vol. 27. Cambridge University Press, Cambridge (2015)
Kinnunen, J., Korte, R., Lorent, A., Shanmugalingam, N.: Regularity of sets with quasiminimal boundary surfaces in metric spaces. J. Geom. Anal. 23, 1607–1640 (2013)
Korte, R., Lahti, P., Li, X., Shanmugalingam, N.: Notions of Dirichlet problem for functions of least gradient in metric measure spaces. To appear in Rev. Mat. Iberoam. https://arxiv.org/pdf/1612.06078.pdf
Lahti, P., Shanmugalingam, N.: Trace theorems for functions of bounded variation in metric spaces. J. Funct. Anal. 274(10), 2754–2791 (2018)
Malý, L., Shanmugalingam, N., Snipes, M.: Trace and extension theorems for functions of bounded variation. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 18(1), 313–341 (2018)
Mazón Ruiz, J.M., Rossi, J.D., de León, S.S.: Functions of least gradient and 1-harmonic functions. Indiana Univ. Math. J. 63, 1067–1084 (2014)
Miranda Jr., M.: Functions of bounded variation on “good” metric spaces. J. Math. Pures Appl. (9) 82(8), 975–1004 (2003)
Pinamonti, A., Serra Cassano, F., Treu, G., Vittone, D.: BV minimizers of the area functional in the Heisenberg group under the bounded slope condition. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 14(3), 907–935 (2015)
Rashed, R.: A pioneer in anaclastics: Ibn Sahl on burning mirrors and lenses. Isis 81(3), 464–491 (1990)
Spradlin, G.S., Tamasan, A.: Not all traces on the circle come from functions of least gradient in the disk. Indiana Univ. Math. J. 63(6), 1819–1837 (2014)
Sternberg, P., Williams, G., Ziemer, W.P.: Existence, uniqueness, and regularity for functions of least gradient. J. Reine Angew. Math. 430, 35–60 (1992)
Author information
Authors and Affiliations
Corresponding author
Additional information
The authors thank Estibalitz Durand-Cartagena, Marie Snipes and Manuel Ritoré for fruitful discussions about the subject of the paper. The research of N.S. was partially supported by the NSF Grant #DMS-1500440 (U.S.). The research of P.L. was supported by the Finnish Cultural Foundation. The research of L.M. was supported by the Knut and Alice Wallenberg Foundation (Sweden). Part of this research was conducted during the visit of N.S. and P.L. to Linköping University. The authors wish to thank this institution for its kind hospitality.
Rights and permissions
About this article
Cite this article
Lahti, P., Malý, L., Shanmugalingam, N. et al. Domains in Metric Measure Spaces with Boundary of Positive Mean Curvature, and the Dirichlet Problem for Functions of Least Gradient. J Geom Anal 29, 3176–3220 (2019). https://doi.org/10.1007/s12220-018-00108-9
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12220-018-00108-9