Skip to main content
SpringerLink
Log in

Study of co-dimension two bifurcation of a prey–predator model with prey refuge and non-linear harvesting on both species

  • Published:
Rendiconti del Circolo Matematico di Palermo Series 2 Aims and scope Submit manuscript

Abstract

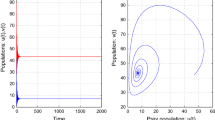
The dynamics of prey–predator system, when one or both the species are harvested non-linearly, has become a topic of intense study because of its wide applications in biological control and species conservation. In this paper we have discuss different bifurcation analysis of a two dimensional prey–predator model with Beddington–DeAngelis type functional response in the presence of prey refuge and non-linear harvesting of both species. We have studied the positivity and boundedness of the model system. All the biologically feasible equilibrium points are investigated and their local stability is analyzed in terms of model parameters. The global stability of coexistence equilibrium point has been discussed. Depending on the prey harvesting effort (\(E_1\)) and degree of competition among the boats, fishermen and other technology (\(l_1\)) used for prey harvesting, the number of axial and interior equilibrium points may change. The system experiences different type of co-dimension one bifurcations such as transcritical, Hopf, saddle-node bifurcation and co-dimension two Bogdanov–Takens bifurcation. The parameter values at the Bogdanov–Takens bifurcation point are highly sensitive in the sense that the nature of coexistence equilibrium point changes dramatically in the neighbourhood of this point. The feasible region of the bifurcation diagram in the \(l_1-E_1\) parametric plane divides into nine distinct sub-regions depending on the number and nature of equilibrium points. We carried out some numerical simulations using the Maple and MATLAB software to justify our theoretical findings and finally some conclusions are given.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Malthus, T.R.: An Essay on the Principle of Population (1978)

  2. Verhulst, P.H.: Notice sur la loi que la population poursuit dans son accroissement. Carrespondance mathematique et physique, vol. 10, pp. 113–121 (1838)

  3. Lotka, A.J.: Elements of Physical Biology. Williams and wilkins, Baltimore (1925)

    MATH  Google Scholar 

  4. Volterra, V.: Variations and fluctuations of a number of individuals in animal species living together [translation by Chapman R.N., 1931], Animal Ecology, pp. 409-448. Mcgraw Hill (1926)

  5. Chen, B.: The influence of commensalism on a Lotka-Volterra commensal symbiosis model with Michaelis-Menten type harvesting. Adv. Differ. Equ. 43 (2019)

  6. Gupta, R.P., Chandra, P., Banerjee, M.: Dynamical complexity of a prey-predator model with non-linear predator harvesting. Discret. Contin. Dyn. Syst. 20, 423–443 (2015)

    MATH  Google Scholar 

  7. Sen, M., Srinivasu, P.D.N., Banerjee, M.: Global dynamics of an additional food provided predator-prey system with constant harvest in predators. Appl. Math. Comput. 250, 193–211 (2015)

    MathSciNet  MATH  Google Scholar 

  8. Aguirre, P., González-Olivares, E., Sáez, E.: Two limit cycles in a Leslie-Gower predator-prey model with additive Allee effect. Nonlinear Anal. Real World Appl. 10, 1401–1416 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Kar, T.K., Das, U., Jana, S.: Dynamical behavior of delayed stage structured predator prey model with non monotonic functional response. Int. J. Dyn. Control (2014). https://doi.org/10.1007/s40435-014-0110-9

    Article  Google Scholar 

  10. Roy, B., Roy, S.K.: Analysis of prey-predator three species models with vertebral and invertebral predators. Int. J. Dyn. Control 3, 306–312 (2015)

    Article  MathSciNet  Google Scholar 

  11. Pal, D., Mahapatra, G.S., Samanta, G.P.: Bifurcation analysis of predator-prey model with time delay and harvesting efforts using interval parameter. Int. J. Dyn. Control (2014). https://doi.org/10.1007/s40435-014-0083-8

    Article  Google Scholar 

  12. Sen, M., Banerjee, M., Morozov, A.: Bifurcation analysis ratio-dependent prey-predator model with the Allee effect. Ecol. Complex. 11, 12–27 (2012)

    Article  Google Scholar 

  13. Molla, H., Rahman, M.S., Sarwardi, S.: Dynamical study of a prey-predator model incorporating nonlinear prey refuge and additive Allee effect acting on prey species. Model Earth Syst. Environ. (2020). https://doi.org/10.1007/s40808-020-01049-5

    Article  MATH  Google Scholar 

  14. Holling, C.S.: The functional response of prey-predator to prey density and its role mimicry and population regulation. Mem. Entomol. Soc. Can. 45, 3–60 (1965)

    Google Scholar 

  15. Holling, C.S.: The components of predation as revealed by a study of small-mammal predation of the European pine sawfly. Can. Entomol. 91, 293–320 (1959)

    Article  Google Scholar 

  16. Holling, C.S.: Some characteristics of simple types of predation and parasitism. Can. Entomol. 91, 385–398 (1959)

    Article  Google Scholar 

  17. Ardity, R., Ginzburg, L.R.: Coupling in predator-prey dynamics : ratio dependence. J. Theor. Biol. 139, 311–326 (1989)

    Article  Google Scholar 

  18. Beddington, J.R.: Mutual interference between parasites or predators and its effect on searching efficiency. J. Anim. Ecol. 44, 331–340 (1975)

    Article  Google Scholar 

  19. DeAngelis, D.L., Goldstein, R.A., ONeill, R.V.: A model for trophic interactions. Ecology 56, 881–892 (1975)

  20. Cantrell, R.S., Cosner, C.: On the dynamics of predator-prey models with the Beddington-DeAngelis functional response. J. Math. Anal. Appl. 257, 206–222 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  21. Pal, P.J., Mandal, P.K.: Bifurcation analysis of a modified Leslie-Gower predator-preymodel with Beddington-DeAngelis functional response and strong Allee effect. Math. Comput. Simul. 97, 123–146 (2014)

    Article  MATH  Google Scholar 

  22. Meng, X., Huo, H., Zhang, X.: The effect of harvesting and delay on predator-prey system with beddington-DeAngelis fubctional response. Int. J. Biomath. 5, 23 (2012)

    Article  MATH  Google Scholar 

  23. Hwang, T.W.: Global analysis of the predator-prey system with Beddington-DeAngelis functional response. J. Math. Anal. Appl. 281, 395–401 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  24. Damian, Y.V., Vidal, C., Olivares, E.G.: Dynamics and bifurcations of a modified Leslie-Gower-type model considering a Beddington-DeAngelis functional response. Math. Methods Appl. Sci. 42(9), 3179–3210 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  25. Khellaf, W., Hamri, N.: Boundedness and Global Stability for a predator-prey System with the Beddington-DeAngelis functional response. Differ. Equ. Nonlinear Mech. 24 (2010)

  26. Lata, K., Misra, A.K., Upadhyay, R.K.: A mathematical model for the conservation of forestry resources with two discrete time delays. Model. Earth Syst. Environ. 3, 1011–1027 (2017). https://doi.org/10.1007/s40808-017-0349-1

    Article  Google Scholar 

  27. Tripathi, J.P., Abbas, S., Thakur, M.: Dynamical analysis of a prey predator model with Beddington-DeAngelis type functional response incorporating a prey refuge. Nonlinear Dyn. 80, 177–196 (2015)

    Article  MATH  Google Scholar 

  28. Xin, Y., Meng, Y., Qian, W.: Bifurcation analysis in a singular Beddington-DeAngelis predator-prey model with two delays and non-linear predator harvesting. Math. Biosci. Eng. 16(4), 2668–2696 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  29. Lv, Y., Zhang, Z., Yuan, R., Pei, Y.: Effect of harvesting and prey refuge in a prey-predator system. J. Biol. Syst. 22, 133–150 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  30. Gan, S.Y., Azman, B.A.R., Yoshida, T., Majid, A.M., Toda, T., Takahashi, K., Othman, B.H.R.: Comparison of day and night mysid assembages traps, with key to species occurring at Pulau Tinggi. Coast. Mar. Sci. Malays. 34(1), 74–81 (2010)

    Google Scholar 

  31. Bullard, S.G., Hay, M.E.: Plankton tethering to assess spatial patterns of predation risk over a coral reef and seagrass bed. Mar. Ecol. Prog. Ser. 225, 17–28 (2002)

    Article  Google Scholar 

  32. Ma, Z., Li, W., Zhao, Y., Wang, W., Zhang, H., Li, Z.: Effects of prey refuges on a predator-prey model with a class of functional responses: the role of refuges. Math. Biosci. 218(2), 73–79 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  33. McNair, J.M.: The effects of refuges on predator-prey interactions: a reconsideration. Theor. Popul. Biol. 29, 38 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  34. Sih, A.: Prey refuges and predator-prey stability. Theor. Popul. Biol. 31, 1 (1987)

    Article  MathSciNet  Google Scholar 

  35. Chakraborty, K., Chakraborty, M., Kar, T.K.: Regulation of a prey-predator fishery incorporating prey refuge by taxation: a dynamic reaction model. J. Biol. Syst. 19(03), 417–445 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  36. Xiao, D., Jennings, L.S.: Bifurcations of a ratio-dependent predator-prey system with constant rate harvesting. SIAM J. Appl. Math. 65(3), 737–753 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  37. Chen, J., Huang, J., Ruan, S., Wang, J.: Bifurcations of invariant tori in predator-prey models with seasonal prey harvesting. SIAM J. Appl. Math. 73(5), 1876–1905 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  38. May, R.M., Beddington, J.R., Clark, C.W., Holt, S.J., Laws, R.M.: Management of multispecies fisheries. Science 205(4403), 267–277 (1979)

    Article  Google Scholar 

  39. Huang, J., Gong, Y., Ruan, S.: Bifurcation analysis in a predator-prey model with constant-yield predator harvesting. Discret. Contin. Dyn. Syst. Ser. B 18, 2101–2121 (2013)

    MathSciNet  MATH  Google Scholar 

  40. Huang, J., Gong, Y., Chen, J.: Multiple bifurcations in a predator-prey system of Holling and Leslie type with constant-yield prey harvesting. Int. J. Bifurc. Chaos 23(10), 1350164 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  41. Clark, C.W.: Aggregation and fishery dynamics: a theoretical study of schooling and the purse seine tuna fisheries. Fish. Bull. 77(2), 317–337 (1979)

    Google Scholar 

  42. Agnew, T.T.: Optimal exploitation of a fishery employing non-linear harvestingfunction. Ecol. Model. 6, 47–57 (1979)

    Article  Google Scholar 

  43. Kong, L., Zhu, C.: Bogdanov-Takens bifurcations of codimensions 2 and 3 in a Leslie-Gower predator-prey model with Michaelis-Menten-type prey harvesting. Math. Methods Appl Sci. 40(18), 6715–6731 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  44. Hu, D., Cao, H.: Stability and bifurcation analysis in a predator-prey system with Michaelis-Menten type predator harvesting. Nonlinear Anal. Real World Appl. 33, 58–82 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  45. Debnath, S., Majumdar, P., Sarkar, S., Ghosh, U.: Global dynamics of a prey-predator model with Holling type iii functional response in the presence of harvesting. J. Biol. Syst. 30(01), 225–260 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  46. Majumdar, P., Debnath, S., Mondal, B., Sarkar, S., Ghosh, U.: Complex dynamics of a prey-predator interaction model with Holling type-II functional response incorporating the effect of fear on prey and non-linear predator harvesting. Rend. Circ. Mat. Palermo Ser. 2, 1–32 (2022)

    MATH  Google Scholar 

  47. Santra, P., Mahapatra, G.S., Pal, D.: Prey-predator nonlinear harvesting model with functional response incorporating prey refuge. Int. J. Dyn. Control 4(3), 293–302 (2015)

    Article  MathSciNet  Google Scholar 

  48. Debnath, S., Ghosh, U., Sarkar, S.: Global dynamics of a tritrophic food chain model subject to the Allee effects in the prey population with sexually reproductive generalized-type top predator. Comput. Math. Methods (2019). https://doi.org/10.1002/cmm4.1079

    Article  Google Scholar 

  49. Perko, L.: Differential Equations and Dynamical Systems. Texts in Applied Mathematics, third ed., vol. 7, Springer-Verlag, New York (2001)

  50. La Salle, J.: The Stability of Dynamical Systems. SIAM (1976)

  51. Sotomayor, J.: Generic bifurcations of dynamical systems. In: Peixoto, M.M. (ed.) Dynamical Systems. Academic Press, New York (1973)

    MATH  Google Scholar 

  52. Ghosh, U., Majumdar, P., Ghosh, J.K.: Bifurcation analysis of a two-dimensional predator-prey model with Holling type IV functional response and nonlinear predator harvesting. J. Biol. Syst. 28(04), 839–864 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  53. Majumdar, P., Debnath, S., Sarkar, S., Ghosh, U.: The complex dynamical behavior of a prey-predator model with Holling type III functional response and non-linear predator harvesting. Int. J. Model. Simul. 42(2), 287–304 (2022)

    Article  Google Scholar 

  54. Kuznetsov, Y.A.: Elements of Applied Bifurcation Theory. Applied Mathematical Sciences, vol. 112. Springer, (1998) New York

Download references

Acknowledgements

The authors would like to thank the anonymous reviewers for their careful reading, useful comments and constructive suggestions for the improvement of the manuscript of the present research work.

Funding

This research does not receive any funding from government or private bodies.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, University of Calcutta, Kolkata, 700009, India

    Prahlad Majumdar, Uttam Ghosh, Susmita Sarkar & Surajit Debnath

Authors
  1. Prahlad Majumdar
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Uttam Ghosh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Susmita Sarkar
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Surajit Debnath
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Surajit Debnath.

Ethics declarations

Conflict of interest

There is no conflict of interest in this research work as declared by authors.

Ethical approval

This study does not contain any animal model/experiment and hence does not require any ethical approval.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendices

Appendix I

The coefficient of six degree polynomial equation (6) is given by MAPLE software in the following:

$$\begin{aligned} a_0{} & {} =abcmp_1^2p_2-abcp_1^2p_2,\\ a_1{} & {} =2E_1abc l_1mp_1p_2+a^2dm^2p_1^2p_2-2E_1abc l_1p_1p_2\\{} & {} +E_2b^2c l_2p_1^2-2a^2dmp_1^2p_2-2abcmp_1^2p_2\\{} & {} -acm^2p_1^2p_2+a^2dp_1^2p_2+2abcp_1^2p_2\\{} & {} +2acmp_1^2p_2-acp_1^2 p_2-bcp_1^2p_2,\\ a_2{} & {} =E_1^2abc l_1^2mp_2+2E_1a^2dl_1m^2p_1p_2-E_1^2abc l_1^2p_2\\{} & {} +2E_1E_2b^2c l-1 l_2p_1-4E_1a^2dl_1mp_1p_2\\{} & {} -4E_1abc l_1mp_1p_2-2E_1ac l_1m^2p_1p_2+E_2abd l_2mp_1^2\\{} & {} -a^2dm^2p_1^2p_2+2E_1a^2d l_1 p_1p_2\\{} & {} +4E_1abc l_1p_1p_2+2E_1abcmp_1p_2+4E_1ac l_1mp_1p_2-E_2abd l_2p_1^2\\{} & {} -2E_2b^2c l_2p_1^2-2E_2bc l_2mp_1^2\\{} & {} +2a^2dmp_1^2p_2+abcmp_1^2p_2+acm^2p_1^2p_2-2E_1abcp_1p_2\\{} & {} -2E_1ac l_1p_1p_2-2E_1bc l_1*p_1p_2+E_2abmp_1^2\\{} & {} +2E_2bc l_2p_1^2-a^2dp_1^2p_2-abcp_1^2p_2-2acmp_1^2p_2\\{} & {} -2admp_1^2p_2-E_2abp_1^2+acp_1^2p_2\\{} & {} +2adp_1^2p_2+2bcp_1^2p_2+cmp_1^2p_2-cp_1^2p_2,\\ a_3{} & {} =E_1^2a^2d l_1^2m^2p_2+E_1^2E_2b^2c l_1^2 l_2-2E_1^2a^2d l_1^2mp_2\\{} & {} -2E_1^2abcl_1^2mp_2-E_1^2ac l_1^2m^2p_2\\{} & {} +2E_1E_2abd l_1 l_2mp_1-2E_1a^2d l_1m^2p_1p_2+E_1^2a^2d l_1^2p_2\\{} & {} +2E_1^2abc l_1^2p_2+2E_1^2abc l_1mp_2\\{} & {} +2E_1^2ac l_1^2mp_2-2E_1E_2abd l_1 l_2p_1-4E_1E_2b^2c l_1 l_2p_1\\{} & {} -4E_1E_2bc l_1 l_2mp_1+4E_1a^2d l_1mp_1p_2\\{} & {} +E_1a^2dm^2p_1p_2+2E_1abc l_1mp_1p_2+2E_1ac l_1m^2p_1p_2-E_2abd l_2mp_1^2\\{} & {} -E_2ad l_2m^2p_1^2-2E_1^2abc l_1 p_2\\{} & {} -E_1^2ac l_1^2p_2-E_1^2bc l_1^2p_2+2E_1E_2abl_1mp_1+2E_1E_2b^2c l_2p_1\\{} & {} +4E_1E_2bc l_1 l_2 p_1-2E_1a^2d l_1p_1p_2\\{} & {} -2E_1a^2dmp_1p_2-2E_1abc l_1p_1p_2-2E_1abcmp_1p_2-4E_1acl_1mp_1p_2\\{} & {} -E_1acm^2p_1p_2-4E_1ad l_1mp_1p_2\\{} & {} +E_2abd l_2p_1^2+2E_2adl_2mp_1^2+E_2b^2cl_2p_1^2+2E_2bcl_2mp_1^2\\{} & {} +E_2c l_2m^2p_1^2-2E_1E_2ab l_1p_1+E_1a^2dp_1p_2\\{} & {} +2E_1abcp_1p_2+2E_1acl_1p_1p_2+2E_1acmp_1p_2+4E_1adl_1p_1p_2\\{} & {} +4E_1bcl_1p_1p_2+2E_1c l_1mp_1p_2-E_2abmp_1^2-E_2adl_2p_1^2\\{} & {} -E_2am^2p_1^2-2E_2bc l_2p_1^2-E_2bdl_2p_1^2-2E_2c l_2p_1^2+2admp_1^2p_2\\{} & {} -E_1acp_1p_2-2E_1bcp_1p_2-2E_1c l_1p_1p_2+E_2abp_1^2+2E_2amp_1^2\\{} & {} +E_2c l_2p_1^2-2adp_1^2p_2-bcp_1^2p_2-cmp_1^2p_2\\{} & {} -E_2ap_1^2-E_2bp_1^2+cp_1^2p_2+dp_1^2p_2, \\ a_4{} & {} =E_1^2E_2abd l_1^2 l_2 m-E_1^2a^2d l_1^2 m^2p_2\\{} & {} -E_1^2E_2abd l_1^2 l_2-2E_1^2E_2b^2c l_1^2 l_2\\{} & {} -2E_1^2E_2bc l_1^2 l_2 m+2E_1^2a^2d l_1^2 mp_2+E_1^2a^2d l_1 m^2p_2\\{} & {} +E_1^2abc l_1^2 mp_2+E_1^2ac l_1^2 m^2p_2\\{} & {} -2E_1E_2abd l_1 l_2 mp_1-2E_1E_2ad l1 l_2 m^2p_1+E_1^2E_2abl_1^2m\\{} & {} +2E_1^2E_2b^2c l_1 l_2+2E_1^2E_2bc l_1^2 l_2\\{} & {} -E_1^2a^2d l_1^2p_2-2E_1^2a^2d l_1 mp_2-E_1^2abc l_1^2p_2-2E_1^2abc l_1 mp_2\\{} & {} -2E_1^2ac l_1^2 mp_2-E_1^2ac l_1 m^2p_2\\{} & {} -2E_1^2ad l_1^2 mp_2+2E_1E_2abd l_1 l_2 p_1+E_1E_2abd l_2 mp_1\\{} & {} +4E_1E_2ad l_1 l_2 mp_1+2E_1E_2b^2c l_1 l_2 p_1+4E_1E_2bc l_1 l_2 mp_1\\{} & {} +2E_1E_2c l_1 l_2 m^2p_1-E_1^2E_2ab l_1^2+E_1^2a^2dl_1p_2+2E_1^2abc l_1 p_2\\{} & {} +E_1^2abcmp_2+E_1^2ac l_1^2 p_2+2E_1^2ac l_1mp_2\\{} & {} +2E_1^2ad l_1^2 p_2+2E_1^2 bc l_1^2p_2+E_1^2c l_1^2 mp_2\\{} & {} -E_1E_2abd l_2 p_1-2E_1E_2abl_1mp_1-2E_1E_2ad l_1 l_2p_1\\{} & {} -2E_1E_2a l_1m^2p_1-2E_1E_2b^2c l_2 p_1-4E_1E_2bc l_1 l_2 p_1\\{} & {} -2E_1E_2bc l_2 mp_1-2E_1E_2bd l_1 l_2p_1-4E_1E_2c l_1 l_2 mp_1\\{} & {} +4E_1ad l_1 mp_1p_2-E_1^2abcp_2-E_1^2ac l_1 p_2-2E_1^2bc l_1p_2\\{} & {} -E_1^2c l_1^2 p_2+2E_1E_2abl_1p_1+E_1E_2abmp_1+4E_1E_2a l_1 mp_1\\{} & {} +2E_1E_2bc l_2 p_1+2E_1E_2c l_1 l_2 p_1-4E_1adl_1p_1p_2-2E_1admp_1p_2\\{} & {} -2E_1bc l_1p_1p_2-2E_1cl_1mp_1p_2+E_2bd l_2 p_1^2\\{} & {} +E_2d l_2 mp_1^2-E_1E_2abp_1-2E_1E_2a l_1 p_1-2E_1E_2bl_1p_1+2E_1adp_1p_2\\{} & {} +2E_1bcp_1p_2+2E_1c l_1 p_1p_2+E_1cmp_1p2\\{} & {} +2E_1d l_1p_1p_2-E_2d l_2p_1^2-E_1cp_1p_2+E_2bp_1^2+E_2mp_1^2-dp_1^2p_2-E_2p_1^2, \\ a_5{} & {} =-E_1^2E_2abdl_1^2l_2m-E_1^2E_2adl_1^2l_2m^2+E_1^2E_2abdl_1^2l_2\\{} & {} +E_1^2E_2abd l_1 l_2 m+2E_1^2E_2ad l_1^2 l_2 m\\{} & {} +E_1^2 E_2b^2c l_1^2 l_2+2E_1^2 E_2bc l_1^2 l_2 m+E_1^2E_2c l_1^2 l_2m^2\\{} & {} -E_1^2E_2abd l_1 l_2-E_1^2E_2ab l_1^2 m\\{} & {} -E_1^2E_2 ad l_1^2 l_2-E_1^2E_2a l_1^2m^2-2E_1^2E_2b^2c l_1 l_2\\{} & {} -2E_1^2*E_2bc l_1^2 l_2-2E_1^2 E2bc l_1 l_2m-E_1^2E_2bd l_1^2 l_2\\{} & {} -2E_1^2E_2c l_1^2 l_2 m+2E_1^2ad l_1^2 mp_2+E_1^2E_2abl_1^2+E_1^2E_2ab l_1 m\\{} & {} +2E_1^2E_2a l_1^2 m+E_1^2E_2b^2c l_2+2E_1^2E_2bcl_1l_2\\{} & {} +E_1^2E_2c l_1^2l_2-2E_1^2adl_1^2p_2-2E_1^2adl_1mp_2-E_1^2bcl_1^2p_2\\{} & {} -E_1^2c l_1^2 mp_2+2E_1E_2bdl_1l_2p_1+2E_1E_2dl_1 l_2mp_1\\{} & {} -E_1^2E_2abl_1-E_1^2E_2a l_1^2-E_1^2E_2bl_1^2+2E_1^2ad l_1 p_2\\{} & {} +2E_1^2bcl_1p_2+E_1^2cl_1^2p_2+E_1^2c l_1mp_2+E_1^2dl_1^2p_2\\{} & {} -E_1E_2bd l_2 p_1-2E_1E_2 d l_1 l_2p_1-E_1^2bcp_2\\{} & {} -E_1^2cl_1p_2+2E_1 E_2 bl_1p_1+2E_1E_2 l_1 mp_1\\{} & {} -2E_1d l_1 p_1p_2-E_1E_2bp_1-2E_1E_2 l_1 p_1+E_1dp_1p_2, \\ a_6{} & {} =E_1^2E_2bd l_1^2 l_2+E_1^2E_2d l_1^2l_2 m-E_1^2E_2bd l_1 l_2\\{} & {} -E_1^2E_2 d l_1^2 l_2+E_1^2E_2b l_1^2+E_1^2E_2 l_1^2 m\\{} & {} -E_1^2d l_1^2p_2-E_1^2E_2bl_1-E_1^2E_2 l_1^2+E_1^2dl_1p_2.\\ \end{aligned}$$

Appendix II

$$\begin{aligned} h_1{} & {} =-2+\frac{2(1-m)^2y^*a}{(1+a(1-m)x^*+by^*)^2}-\frac{2(1-m)^3x^*y^*a^2}{(1+a(1-m)x^*+by^*)^3}\\{} & {} +\frac{2E_1p_1}{(E_1 l_1+p_1x^*)^2}-\frac{2E_1x^*p_1^2}{(E_1 l_1+p_1x^*)^3},\\ h_2{} & {} =-\frac{(1-m)}{(1+a(1-m)x^*+by^*)}+\frac{(1-m)y^*b}{(1+a(1-m)x^*+by^*)^2}\\{} & {} +\frac{(1-m)^2 x^*a}{(1+a(1-m)x^*+by^*)^2}-\frac{2(1-m)^2 x^*y^*ab}{(1+a(1-m)x^*+by^*)^3},\\ h_3{} & {} =\frac{(2(1-m))x^*b}{(1+a(1-m)x^*+by^*)^2}-\frac{(2(1-m))x^*y^*b^2}{(1+a(1-m)x^*+by^*)^3},\\ h_4{} & {} =-\frac{2c(1-m)^2y^*a}{(1+a(1-m)x^*+by^*)^2}+\frac{2c(1-m)^3 x^*y^*a^2}{(1+a(1-m)x^*+by^*)^3}, \\ h_5{} & {} =\frac{c(1-m)}{(1+a(1-m)x^*+by^*)}-\frac{c(1-m)y^*b}{(1+a(1-m)x^*+by^*)^2}\\{} & {} -\frac{c(1-m)^2 x^*a}{(1+a(1-m)x^*+by^*)^2}+\frac{2c(1-m)^2 x^*y^*ab}{(1+a(1-m)x^*+by^*)^3},\\ h_6{} & {} =-\frac{2c(1-m)x^*b}{(1+a(1-m)x^*+by^*)^2}+\frac{2c(1-m)x^*y^*b^2}{(1+a(1-m)x^*+by^*)^3}\\{} & {} +\frac{2E_2p_2}{(E_2l_2+p_2y^*)^2}-\frac{2E_2y^*p_2^2}{(E_2l_2+p_2y^*)^3}.\\ \end{aligned}$$

Appendix III

$$\begin{aligned} \gamma _{10}{} & {} =1-2x^*-\frac{(1-m)y^*(1+by^*)}{(1+a(1-m)x^*+by^*)^2}-\frac{E_1^2 l_1}{(l_1 E_1+p_1 x^*)^2}, \\ \gamma _{01}{} & {} =-\frac{(1-m)x^*[1+(1-m)ax^*]}{(1+a(1-m)x^*+by^*)^2},\\ \gamma _{20}{} & {} =-1+\frac{(-1+m)(-by^{*2}-y^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2(1+a(1-m)x^*+by^*)}\\{} & {} +\frac{E_1^2 l_1 p_1}{(E_1 l_1+x^*p_1)^3},\\ \gamma _{11}{} & {} =-\frac{(1-m-\frac{(-1+m)(amx^{*2}-ax^{*2}-x^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2}-\frac{(-1+m)(-by^{*2}-y^*)b}{(amx^*-ax^*-by^*-1)^2)}}{(1+a(1-m)x^*+by^*)}, \\ \gamma _{02}{} & {} =\frac{(-1+m)(amx^{*2}-ax^{*2}-x^*)b}{((amx^*-ax^*-by^*-1)^2(1+a(1-m)x^*+by^*))}, \\ \gamma _{30}{} & {} =\frac{(-1+m)(abmy^{*2}-aby^{*2}+amy^*-ay^*)a(1-m)}{((amx^*-ax^*-by^*-1)^3 (1+a(1-m)x^*+by^*))}-\frac{E_1^2 l_1 p_1^2}{(E_1 l_1+x^* p_1)^4},\\ \gamma _{21}{} & {} =\frac{\frac{((-1+m)(-2abmx^*y^*+2aby^*-amx^*+ax^*+by^*+1)a(1-m)}{(amx^*-ax^*-by^*-1)^3}+\frac{(-1+m)(abmy^{*2}-aby^{*2}+amy^*-ay^*)b}{(amx^*-ax^*-by^*-1)^3)}}{(1+a(1-m)x^*+by^*)}, \\ \gamma _{12}{} & {} =\frac{\frac{(-1+m)(abmx^{*2}-abx^{*2}-bx^*)a(1-m)}{(amx^*-ax^*-by^*-1)^3}+\frac{(-1+m)(-2abmx^*y^*+2abx^*y^*-amx^*+ax^*+by^*+1)b}{(amx^*-ax^*-by^*-1)^3}}{(1+a(1-m)x^*+by^*)}, \\ \gamma _{03}{} & {} =\frac{(-1+m)(abmx^{*2}-abx^{*2}-bx^*)b}{((amx^*-ax^*-by^*-1)^3(1+a(1-m)x^*+by^*))},\\ \beta _{10}{} & {} =A_{21}=\frac{c(1-m)y^*(1+by^*)}{(1+a(1-m)x^*+by^*)^2}, \beta _{01}\\{} & {} =-d+\frac{c(1-m)x^*[1+a(1-m)x^*]}{(1+a(1-m)x^*+by^*)^2}-\frac{E_2^2 l_2}{(E_2l_2+p_2y^*)^2},\\ \beta _{20}{} & {} =-\frac{(-1+m)c(-by^{*2}-y^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2 (1+a(1-m)x^*+by^*))}, \\ \beta _{11}{} & {} =\frac{\frac{(c(1-m)-(-1+m)c(amx^{*2}-ax^{*2}-x^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2}-\frac{(-1+m)c(-by^{*2}-y^*)b}{(amx^*-ax^*-by^*-1)^2)}}{(1+a(1-m)x^*+by^*)},\\ \beta _{02}{} & {} =-\frac{(-1+m)c(amx^{*2}-ax^{*2}-x^*)b}{((amx^*-ax^*-by^*-1)^2(1+a(1-m)x^*+by^*))}\\{} & {} +\frac{(E_2+\epsilon _2)^2 l_2 p_2}{((E_2 l_2+\epsilon _2 l_2+p_2y^*)^2(l_2 (E_2+\epsilon _2)+p_2 y^*))}, \\ \beta _{30}{} & {} =-\frac{(-1+m)c(abmy^{*2}-aby^{*2}+amy^*-ay^*)a(1-m)}{((amx^*-ax^*-by^*-1)^3(1+a(1-m)x^*+by^*))}, \\ \beta _{21}{} & {} =-\frac{\frac{(-1+m)c(-2abmx^*y^*+2abx^* y^*-amx^*+ax^*+by^*+1)a(1-m)}{(amx^*-ax^*-by^*-1)^3}+\frac{(-1+m)c(abmy^{*2}-aby^{*2}+amy^*-ay^*)b}{(amx^*-ax^*-by^*-1)^3}}{(1+a(1-m)x^*+by^*)}, \\ \beta _{12}{} & {} =-\frac{\frac{(-1+m)c(abmx^{*2}-abx^{*2}-bx^*)a(1-m)}{(amx^*-ax^*-by^*-1)^3}+\frac{(-1+m)c(-2abmx^*y^*+2abx^*y^*-amx^*+ax^*+by^*+1)b}{(amx^*-ax^*-by^*-1)^3}}{(1+a(1-m)x^*+by^*)}, \\ \beta _{03}{} & {} =-\frac{(-1+m)c(abmx^{*2}-abx^{*2}-bx^*)b}{((amx^*-ax^*-by^*-1)^3(1+a(1-m)x^*+by^*))}\\{} & {} -\frac{(E_2+\epsilon _2)^2 l_2 p_2^2}{((E_2 l_2+\epsilon _2 l_2+p_2y^*)^3(l_2(E_2+\epsilon _2)+p_2y^*))}.\\ \end{aligned}$$

Appendix IV

$$\begin{aligned} a(\epsilon ){} & {} =-2x^*+1-\frac{((1-m)y^*-\frac{(-1+m)x^*y^*a(1-m)}{(amx^*-ax^*-by^*-1))}}{(1+a(1-m)x^*+by^*)}\\{} & {} -\frac{((E_1+e_2)-\frac{(E_1+e_2)x^*p_1}{(E_1 e_1+E_1 l_1+e_1 e_2+e_2 l_1+x^*p_1))}}{((l_1+e_1)(E_1+e_2)+p_1 x^*)},\\ b(\epsilon ){} & {} =-\frac{((1-m)x^*-\frac{(-1+m)x^*y^*b}{(amx^*-ax^*-by^*-1))}}{(1+a(1-m)x^*+by^*)},\\ c(\epsilon ){} & {} =\frac{(c(1-m)y^*-\frac{c(-1+m)x^*y^*a(1-m)}{(amx^*-ax^*-by^*-1))}}{(1+a(1-m)x^*+by^*)},\\ d(\epsilon ){} & {} =-d+\frac{(c(1-m)x^*-\frac{c(-1+m)x^*y^*b}{(amx^*-ax^*-by^*-1))}}{(1+a(1-m)x^*+by^*)}\\{} & {} -\frac{(E_2-\frac{E_2y^*p_2}{(E_2 l_2+p_2y^*))}}{(E_2 l_2+p_2y^*)},\\ p_{00}(\epsilon ){} & {} =x^*(-x^*+1)-\frac{(1-m)x^*y^*}{(1+a(1-m)x^*+by^*)}-\frac{(E_1+e_2)x^*}{((l_1+e_1)(E_1+\epsilon _2)+p_1x^*)},\\ p_{20}(\epsilon ){} & {} =-1+\frac{(-1+m)(-by^{*2}-y^*)a(1-m)}{((amx^*-ax^*-by^*-1)^2 (1+a(1-m)x^*+by^*))}\\{} & {} +\frac{(E_1+e_2)(E_1e_1+E_1 l_1+e_1 e_2+e_2 l_1)p_1}{((E_1 e_1+E_1 l_1+e_1 e_2+e_2 l_1+x^*p_1)^2 ((l_1+e_1)(E_1+e_2)+p_1 x^*))},\\ p_{11}(\epsilon ){} & {} =-\frac{(1-m-\frac{(-1+m)(amx^{*2}-ax^{*2}-x^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2}-\frac{(-1+m)(-by^{*2}-y^*)b}{(amx^*-ax^*-by^*-1)^2)}}{(1+a(1-m)x^*+by^*)},\\ p_{02}(\epsilon ){} & {} =\frac{(-1+m)(amx^{*2}-ax^{*2}-x^*)b}{((amx^*-ax^*-by^*-1)^2 (1+a(1-m)x^*+by^*))},\\ q_{00}(\epsilon ){} & {} =-dy^*+\frac{c(1-m)x^*y^*}{(1+a(1-m)x^*+by^*)}-\frac{E_2y^*}{(E_2 l_2+p_2y^*)},\\ q_{20}(\epsilon ){} & {} =-\frac{(-1+m)c(-by^{*2}-y^*)a(1-m)}{((amx^*-ax^*-by^*-1)^2(1+a(1-m)x^*+by^*))},\\ q_{11}(\epsilon ){} & {} =\frac{(c(1-m)-\frac{(-1+m)c(amx^{*2}-ax^{*2}-x^*)a(1-m)}{(amx^*-ax^*-by^*-1)^2}-\frac{(-1+m)c(-by^{*2}-y^*)b}{(amx^*-ax^*-by^*-1)^2)}}{(1+a(1-m)x^*+by^*)},\\ q_{02}(\epsilon ){} & {} =-\frac{(-1+m)c(amx^{*2}-ax^{*2}-x^*)b}{((amx^*-ax^*-by^*-1)^2(1+a(1-m)x^*+by^*))}+\frac{E_2^2 l_2 p_2}{(E_2 l_2+p_2y^*)^3}.\\ \end{aligned}$$

Appendix V Expressions of \(k_{ij}\) and \(l_{ij}\)

$$\begin{aligned} k_{00}(\epsilon ){} & {} =\frac{p_{00}(\epsilon )}{\eta _{12}} -\eta _{11} \frac{p_{00}(\epsilon )}{\eta _{12}} -\eta _{00}(\epsilon ),\\ k_{10}(\epsilon ){} & {} =\frac{a(\epsilon ) \eta _{12} -b(\epsilon ) \eta _{11}}{\eta _{12}}-\eta _{11} \lbrace \frac{a(\epsilon ) \eta _{12} -b(\epsilon ) \eta _{11}}{\eta _{12}}+\frac{c(\epsilon ) \eta _{12} -d(\epsilon ) \eta _{11}}{\eta _{11}} \rbrace ,\\ k_{01}(\epsilon ){} & {} =\frac{a(\epsilon ) \eta _{12} +b(\epsilon ) (1-\eta _{11})}{\eta _{12}}-\eta _{11} \lbrace \\{} & {} \frac{a(\epsilon ) \eta _{12} +b(\epsilon )(1-\eta _{11})}{\eta _{12}}+\frac{c(\epsilon ) \eta _{12} +d(\epsilon ) (1-\eta _{11})}{\eta _{11}} \rbrace -1,\\ k_{20}(\epsilon ){} & {} =2\frac{p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{02}(\epsilon ) \eta _{11}^{2}}{\eta _{12}}\\{} & {} -2\eta _{11} \frac{p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{02}(\epsilon ) \eta _{11}^{2}}{\eta _{12}}+2q_{11}(\epsilon ) \eta _{12} \eta _{11}-2q_{02}(\epsilon ) \eta _{11}^{2},\\ k_{11}(\epsilon ){} & {} =\frac{2p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{11}(\epsilon ) \eta _{12} (1-\eta _{11})-2p_{02}(\epsilon ) \eta _{11} (1-\eta _{11})}{\eta _{12}} \\{} & {} -\eta _{11} \frac{2p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{11}(\epsilon ) \eta _{12} (1-\eta _{11})-2p_{02}(\epsilon ) \eta _{11} (1-\eta _{11})}{\eta _{12}}\\{} & {} -q_{11}(\epsilon ) \eta _{12} (1-\eta _{11})+q_{11}(\epsilon ) \eta _{11} \eta _{12}+2q_{02}(\epsilon ) \eta _{11} (1-\eta _{11}),\\ k_{02}(\epsilon ){} & {} =2\frac{p_{20}(\epsilon ) \eta _{12}^{2}+p_{11}(\epsilon ) \eta _{12} (1-\eta _{11})+p_{02}(\epsilon ) (1-\eta _{11})^{2}}{\eta _{12}}\\{} & {} -2\eta _{11} \frac{p_{20}(\epsilon ) \eta _{12}^{2}+p_{11}(\epsilon ) (1-\eta _{11})+p_{02}(\epsilon ) (1-\eta _{11})^{2}}{\eta _{12}}-2q_{11}(\epsilon ) \eta _{12} (1-\eta _{11})\\{} & {} -2q_{02}(\epsilon ) (1-\eta _{11})^{2},\\ l_{00}(\epsilon ){} & {} = \frac{ \eta _{11}p_{00}(\epsilon )}{\eta _{12}}+q_{00}(\epsilon ),\\ l_{10}(\epsilon ){} & {} =\eta _{11} \left\{ \frac{a(\epsilon ) \eta _{12} -b(\epsilon ) \eta _{11}}{\eta _{12}}+\frac{c(\epsilon ) \eta _{12} -d(\epsilon ) \eta _{11}}{\eta _{11}} \right\} ,\\ l_{01}(\epsilon ){} & {} =\eta _{11} \left\{ \frac{a(\epsilon ) \eta _{12} +b(\epsilon )(1-\eta _{11})}{\eta _{12}}+\frac{c(\epsilon ) \eta _{12} +d(\epsilon ) (1-\eta _{11})}{\eta _{11}} \right\} ,\\ l_{20}(\epsilon ){} & {} =2\eta _{11} \frac{p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{02}(\epsilon ) \eta _{11}^{2}}{\eta _{12}}-2q_{11}(\epsilon ) \eta _{12} \eta _{11}+2q_{02}(\epsilon ) \eta _{11}^{2},\\ l_{11}(\epsilon ){} & {} =\eta _{11} \frac{2p_{20}(\epsilon ) \eta _{12}^{2}-p_{11}(\epsilon ) \eta _{12} \eta _{11}+p_{11}(\epsilon ) \eta _{12} (1-\eta _{11})-2p_{02}(\epsilon ) \eta _{11} (1-\eta _{11})}{\eta _{12}}\\{} & {} +q_{11}(\epsilon ) \eta _{12} (1-\eta _{11})\\{} & {} -q_{11}(\eta ) \eta _{11} \eta _{12}-2q_{02}(\epsilon ) \eta _{11} (1-\eta _{11}),\\ l_{02}(\epsilon ){} & {} =2 \eta _{11} \frac{p_{20}(\epsilon ) \eta _{12}^{2}+p_{11}(\epsilon ) \eta _{12} (1-\eta _{11})+p_{02}(\epsilon ) (1-\eta _{11})^{2}}{\eta _{12}}\\{} & {} +2q_{11}(\epsilon ) \eta _{12} (1-\eta _{11})+2q_{02}(\epsilon ) (1-\eta _{11})^{2}.\\ \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Majumdar, P., Ghosh, U., Sarkar, S. et al. Study of co-dimension two bifurcation of a prey–predator model with prey refuge and non-linear harvesting on both species. Rend. Circ. Mat. Palermo, II. Ser 72, 4067–4100 (2023). https://doi.org/10.1007/s12215-023-00881-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12215-023-00881-9

Keywords

Mathematics Subject Classification

Search

Navigation