Mean reversion and long memory in African stock market prices

Abstract

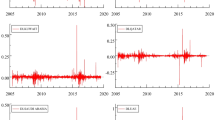
We examine the behavior of stock market prices in several African countries by means of fractionally integrated techniques. In doing so, we can test for mean reversion in these markets. Our results can be summarized as follows: we cannot find evidence of mean reversion in any single market, and evidence of long memory returns (i.e., orders of integration above 1 in the logged stock prices) is obtained in the cases of Egypt and Nigeria, and, in a lesser extent in Tunisia, Morocco and Kenya. Permitting the existence of a structural change, the break dates take place in the earlier 2000s in the majority of the cases, and evidence of mean reversion seems to have taken place in the periods before the breaks in most of the countries. If we focus on the absolute and squared returns, evidence of long memory is obtained in Nigeria and Egypt. Thus, for these two countries, a long memory model incorporating positive fractional degrees of integration in both the level and the volatility process should be considered.

Appendix

The Lagrange Multiplier (LM) test of Robinson (1994) for testing H_o: d = d _o, in the model given by the Eqs. 1 and 2 is

$$ \widehat{r} = \frac{{{T^{1/2}}}}{{{{\widehat{\sigma }}^2}}}{\widehat{A}^{ - 1/2}}\widehat{a}, $$

where T is the sample size and:

$$ \widehat{a} = \frac{{ - 2\pi }}{T}\sum\limits_{j = 1}^{T - 1} {\psi \left( {{\lambda_j}} \right)\,g{{\left( {{\lambda_j};\widehat{\tau }} \right)}^{ - 1}}\,I\left( {{\lambda_j}} \right);\quad {{\widehat{\sigma }}^2} = {\sigma^2}\left( {\hat{\tau }} \right) = \frac{{2\pi }}{T}\,\sum\limits_{j = 1}^{T - 1} {g{{\left( {{\lambda_j};\widehat{\tau }} \right)}^{ - 1}}\,I\left( {{\lambda_j}} \right);} \,} $$

$$ \widehat{A} = \frac{2}{T}\left( {\sum\limits_{j = 1}^{T - 1} {\psi {{\left( {{\lambda_j}} \right)}^2} - \sum\limits_{j = 1}^{T - 1} {\psi \left( {{\lambda_j}} \right)\widehat{\varepsilon }\left( {{\lambda_j}} \right)\prime \times {{\left( {\sum\limits_{j = 1}^{T - 1} {\widehat{\varepsilon }\left( {{\lambda_j}} \right)\widehat{\varepsilon }\left( {{\lambda_j}} \right)\prime } } \right)}^{ - 1}} \times \sum\limits_{j = 1}^{T - 1} {\widehat{\varepsilon }\left( {{\lambda_j}} \right)\psi \left( {{\lambda_j}} \right)} \,} } } \right) $$

$$ \psi \left( {{\lambda_j}} \right) = \log \,\left| {2\,\sin \,\frac{{{\lambda_j}}}{2}} \right|;\quad \widehat{\varepsilon }\left( {{\lambda_j}} \right) = \frac{\partial }{{\partial \,\tau }}\log g\left( {{\lambda_j};\widehat{\tau }} \right);\quad {\lambda_j} = \frac{{2\pi \;j}}{T};\quad \widehat{\tau } = \arg \min {\sigma^2}\left( \tau \right). $$

$\widehat{\hbox{a}}$ and $\widehat{\hbox{A}}$ in the above expressions are obtained through the first and second derivatives of the log-likelihood function with respect to d (see Robinson 1994, page 1,422, for further details). I(λ _j) is the periodogram of u _t evaluated under the null, i.e.:

$$ \widehat{u} = {\left( {1 - L} \right)}^{{d_{o} }} y_{t} - \widehat{\beta }\prime w_{t} ; \quad \widehat{\beta } = {\left( {{\sum\limits_{t = 1}^T {w_{t} w^{\prime }_{t} } }} \right)}^{{ - 1}} {\sum\limits_{t = 1}^T {w_{t} {\left( {1 - L} \right)}^{{d_{o} }} y_{t} ; \quad w_{t} = {\left( {1 - L} \right)}^{{d_{o} }} z_{t} ,} } $$

where z _t = (1, t)^T, and g is a known function related to the spectral density function of u _t:

$$ f\left( {\lambda; {\sigma^2};\tau } \right) = \frac{{{\sigma^2}}}{{2\,\pi }}g\left( {\lambda; \tau } \right),\quad - \pi < \lambda \leqslant \pi . $$