Skip to main content
SpringerLink
Log in

Numerical solution of a class of Caputo–Fabrizio derivative problem using Haar wavelet collocation method

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

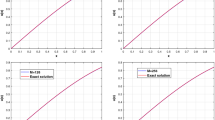
In this paper, we propose an efficient numerical method to solve a class of fractional differential equations with Caputo–Fabrizio fractional derivative. This method is based on Haar wavelet collocation method to convert the considered problem to an algebraic system of equations. The main advantage of our proposed method is its accuracy and exponential convergence which is proved by error analysis. Finally, illustative examples are provided to demontrate the efficiency of our method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Mehandiratta, V., Mehra, M., Leugering, G.: An approach based on Haar wavelet for the approximation of fractional calculus with application to initial and boundary value problems. Math. Meth. Appl. Sci. 44(4), 3195–3213 (2021). https://doi.org/10.1002/mma.6800

    Article  MathSciNet  MATH  Google Scholar 

  2. Liang, S., Wu, R., Chen, L.: Laplace transform of fractional order differential equations. Electron. J. Differ. Equ. 2015(139), 1–15 (2015)

    MathSciNet  MATH  Google Scholar 

  3. Priyadharsini, S.: Stability of fractional neutral and integrodifferential systems. J. Fract. Calc. Appl. 7(1), 87–102 (2016)

    MathSciNet  MATH  Google Scholar 

  4. Youbi, F., Momani, S., Hasan, S., Al-Smadi, M.: Effective numerical technique for nonlinear Caputo-Fabrizio systems of fractional Volterra integro-differential equations in Hilbert space. Alex. Eng. J. 61(3), 1778–1786 (2022). https://doi.org/10.1016/j.aej.2021.06.086

    Article  Google Scholar 

  5. Alharbi, F.M., Zidan, A.M., Naeem, M., Shah, R., Nonlaopon, K.: Numerical investigation of fractional-order differential equations via \(\varphi \)-Haar-wavelet method. J. Funct. Sp. 2021, 1–14 (2021). https://doi.org/10.1155/2021/3084110

    Article  MathSciNet  MATH  Google Scholar 

  6. Mboro Nchama, G.A., Lau Alfonso, L.D., León Mecías, A.M., Rodríguez Ricard, M.: Properties of the Caputo-Fabrizio fractional derivative. Int. J. Appl. Eng. Res. 16(1), 13–21 (2021)

    Google Scholar 

  7. Moumen Bekkouche, M., Mansouri, I., Ahmed, A.A.: Numerical solution of fractional boundary value problem with Caputo-Fabrizio and its fractional integral. J. Appl. Math. Comput. 68(6), 4305–4316 (2022). https://doi.org/10.1007/s12190-022-01708-z

    Article  MathSciNet  MATH  Google Scholar 

  8. Kumar, S., Nieto, J.J., Ahmad, B.: Chebyshev spectral method for solving fuzzy fractional Fredholm-Volterra integro-differential equation. Math. Comput. Simul. 192, 501–513 (2022). https://doi.org/10.1016/j.matcom.2021.09.017

    Article  MathSciNet  MATH  Google Scholar 

  9. Kumar, S., Zeidan, D.: An efficient Mittag-Leffler kernel approach for time-fractional advection-reaction-diffusion equation. Appl. Numer. Math. 170, 190–207 (2021). https://doi.org/10.1016/j.apnum.2021.07.025

    Article  MathSciNet  MATH  Google Scholar 

  10. Kumar, S.: Numerical solution of fuzzy fractional diffusion equation by Chebyshev spectral method. Numer. Meth. Part. Diff. Eq. 38(3), 490–508 (2022). https://doi.org/10.1002/num.22650

    Article  MathSciNet  Google Scholar 

  11. Kumar, S., Zeidan, D.: Numerical study of zika model as a mosquito borne virus with non singular fractional derivative. Int. J. Biomath. 15(5), 2250018 (2022). https://doi.org/10.1142/S1793524522500188

    Article  MathSciNet  MATH  Google Scholar 

  12. Kumar, S.: Fractional fuzzy model of advection-reaction-diffusion equation with application in porous media. J. Porous Med. 25(7), 15–33 (2022)

    Article  Google Scholar 

  13. Amin, R., Shah, K., Asif, M., Khan, I.: A computational algorithm for the numerical solution of fractional order delay differential equations. Appl. Math. Comput. 402, 125863 (2021). https://doi.org/10.1016/j.amc.2020.125863

    Article  MathSciNet  MATH  Google Scholar 

  14. Amin, R., Ahmadian, A., Alreshidi, N.A., Gao, L., Salimi, M.: Existence and computational results to Volterra-Fredholm integro-differential equations involving delay term. Comp. Appl. Math. 40, 1–18 (2021). https://doi.org/10.1007/s40314-021-01643-y

    Article  MathSciNet  MATH  Google Scholar 

  15. Alrabaiah, H., Ahmad, I., Amin, R., Shah, K.: A numerical method for fractional variable order pantograph differential equations based on Haar wavelet. Eng. Comput. 38, 2655–2668 (2022). https://doi.org/10.1007/s00366-020-01227-0

    Article  Google Scholar 

  16. Aziz, I., Amin, R.: Numerical solution of a class of delay differential and delay partial differential equations via Haar wavelet. Appl. Math. Model. 40(23–24), 10286–10299 (2016). https://doi.org/10.1016/j.apm.2016.07.018

    Article  MathSciNet  MATH  Google Scholar 

  17. Berwal, N., Panchal, D., Parihar, C.L.: Solution of differential equations based on Haar operational matrix. Palest. J. Math. 3(2), 281–288 (2014)

    MathSciNet  MATH  Google Scholar 

  18. Shiralashetti, S.C., Angadi, L.M., Kantli, M.H., Deshi, A.B.: Numerical solution of parabolic partial differential equations using adaptive gird Haar wavelet collocation method. Asian Eur. J. Math. 10(1), 1750026 (2017). https://doi.org/10.1142/S1793557117500267

    Article  MathSciNet  MATH  Google Scholar 

  19. Shesha, S.R., Savitha, S., Nargund, A.L.: Numerical solution of fredholm integral equations of second kind using Haar wavelets. Commun. Appl. Sci. 4(2), 49–66 (2016)

    Google Scholar 

  20. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. North Holland Mathematics Studies. Elsevier Science Publishers, Amsterdam (2006)

    Google Scholar 

  21. Shah, F.A., Abbas, R.: Haar wavelet operational matrix method for the numerical solution of fractional order differential equations. Nonlinear Eng. 4(4), 203–213 (2015)

    Article  Google Scholar 

  22. Babolian, E., Shahsavaran, A.: Numerical solution of nonlinear Fredholm integral equations of the second kind using Haar wavelets. J. Comput. Appl. Math. 225(1), 87–95 (2009). https://doi.org/10.1016/j.cam.2008.07.003

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors are highly grateful to the anonymous referee for his/her valuable comments and suggestions for the improvement of the paper.

Author information

Author notes

  1. Bachir Dehda, Abdelaziz Azeb Ahmed, Fares Yazid and Fatima Siham Djeradi have contributed equally to this work.

Authors and Affiliations

  1. Laboratory of Operator Theory and PDE: Foundations and Applications, University of El Oued, 39000, El Oued, Algeria

    Bachir Dehda & Abdelaziz Azeb Ahmed

  2. Laboratory of Pure and Applied Mathematics, Amar Telidji University, 03000, Laghouat, Algeria

    Fares Yazid & Fatima Siham Djeradi

Authors
  1. Bachir Dehda
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abdelaziz Azeb Ahmed
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Fares Yazid
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Fatima Siham Djeradi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Fares Yazid.

Ethics declarations

Conflict of interest

The authors declare that they have no conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dehda, B., Azeb Ahmed, A., Yazid, F. et al. Numerical solution of a class of Caputo–Fabrizio derivative problem using Haar wavelet collocation method. J. Appl. Math. Comput. 69, 2761–2774 (2023). https://doi.org/10.1007/s12190-023-01859-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-023-01859-7

Keywords

Mathematics Subject Classification

Search

Navigation