A hybrid numerical scheme for singular perturbation delay problems with integral boundary condition

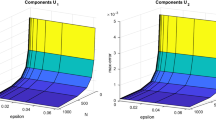
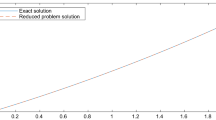
Abstract

In this article, a singular perturbation delay problem of convection–diffusion (C–D) type having an integral boundary condition is considered. The analytical solution of the considered problem has a weak interior layer in addition to the boundary layer at the right end of the domain. Some a priori estimates are given on the exact solution which are useful for the error analysis. The numerical approximation is composed of a hybrid finite difference scheme on a generalized Shishkin mesh. For the proposed scheme, almost second order $\varepsilon$-uniform convergence is established. Numerical experiments are conducted to corroborate the theoretical results. A comparison with the existing scheme (J Appl Math Comput 63:813–828) is also performed.

Appendices

Appendix

Some properties of the barrier function $\phi _{i}(m)$.

Lemma A.1

For $0< m < r^{*}/2$, the following inequalities hold

$$\begin{aligned} {\mathcal {L}}_\varepsilon ^{M}\phi _{i}(m) \ge {\left\{ \begin{array}{ll} \dfrac{C}{\varepsilon + m h_i}\phi _{i}(m), &{} i=1, \ldots , M/4, \, M/2+1, \ldots , 3M/4, \\ \dfrac{C}{\varepsilon }\phi _{i}(m), &{} i=M/4+1, \ldots , M/2, \, 3M/4+1, \ldots , M-1. \end{array}\right. } \end{aligned}$$

Proof

Using the barrier function defined in Eq. (4.3), we obtain

$$\begin{aligned} \phi _{i+1}(m)-\phi _{i}(m) = \dfrac{m h_{i+1}}{\varepsilon + m h_{i+1}}\phi _{i+1}(m). \end{aligned}$$

We now apply the operator ${\mathcal {L}}_\varepsilon ^M$ on $\phi _{i}(m)$ for $i=1,\ldots ,M/4$ to obtain

$$\begin{aligned} {\mathcal {L}}_\varepsilon ^{M}\phi _{i}(m)&=-\varepsilon \delta ^2 \phi _{i}(m) + r_{i-1/2} D^{-} \phi _{i}(m) + s_{i-1/2} \phi _{i-1/2}(m) \\&=\bigg [ \dfrac{m}{\varepsilon + m h_{i}}\bigg (r_{i-1/2}-\dfrac{2mh_{i}}{h_{i}+h_{i+1}}\bigg ) + \dfrac{s_{i-1/2}}{2}\bigg (\dfrac{\varepsilon }{\varepsilon +mh_{i}} + 1\bigg )\bigg ]\phi _{i}(m) \\&\ge \dfrac{C}{\varepsilon +mh_{i}} \phi _{i}(m). \end{aligned}$$

Again, for $i=M/4+1,\ldots ,M/2$, we have

$$\begin{aligned} {\mathcal {L}}_\varepsilon ^{M}\phi _{i}(m)&=-\varepsilon \delta ^2\phi _{i}(m) + r_{i} D^{0}\phi _{i}(m) + s_{i} \phi _{i}(m) \\&=\bigg [ \dfrac{m}{h_{i}+h_{i+1}}\bigg \{ \dfrac{h_{i}}{\varepsilon +mh_{i}}(r_i - 2m)+ \dfrac{h_{i+1} r_i}{\varepsilon }\bigg \} + s_{i}\bigg ]\phi _{i}(m) \\&\ge \dfrac{C}{\varepsilon } \phi _{i}(m) . \end{aligned}$$

It is easy to obtain that

$$\begin{aligned} \phi _{i-M/2}(m)=\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_1}\bigg )^{M/4}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_2}\bigg )^{M/4}\phi _{i}(m), \,\, for \,\, i=M/2+1, \ldots , M-1. \end{aligned}$$

Now, applying the discrete operator ${\mathcal {L}}_\varepsilon ^M$ on $\phi _{i}(m)$ for $i= M/2+1,\ldots ,3M/4$, we obtain

$$\begin{aligned}&{\mathcal {L}}_\varepsilon ^{M}\phi _{i}(m) \\&\quad =-\varepsilon \delta ^2 \phi _{i}(m) + r_{i-1/2} D^{-}\phi _{i}(m) + s_{i-1/2}\, \phi _{i-1/2}(m) + t_{i-1/2} \,\phi _{i-M/2-1/2}(m) \\&\quad =\Bigg [ \dfrac{m}{\varepsilon + m h_{i}}\bigg (r_{i-1/2}-\dfrac{2mh_{i}}{h_{i}+h_{i+1}}\bigg )+\dfrac{s_{i-1/2}}{2}\bigg (\dfrac{\varepsilon }{\varepsilon +mh_{i}} + 1\bigg )\\&\qquad \qquad \quad +\dfrac{t_{i-1/2}}{2}\bigg (\dfrac{\varepsilon }{\varepsilon +mh_{i}} + 1\bigg )\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_1}\bigg )^{M/4}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_2}\bigg )^{M/4}\Bigg ]\phi _{i}(m) \\&\quad =\Bigg [ \dfrac{m}{\varepsilon + m h_{i}}\bigg (r_{i-1/2}-\dfrac{2mh_{i}}{h_{i}+h_{i+1}}\bigg )+\bigg (\dfrac{s_{i-1/2}+t_{i-1/2}}{2}\bigg )\bigg (\dfrac{\varepsilon }{\varepsilon +mh_{i}} + 1\bigg )\\&\qquad \quad \quad +\dfrac{t_{i-1/2}}{2}\bigg (\dfrac{\varepsilon }{\varepsilon +mh_{i}} + 1\bigg )\bigg \{\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_1}\bigg )^{M/4}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_2}\bigg )^{M/4}-1\bigg \}\Bigg ]\phi _{i}(m) \\&\quad \ge \dfrac{C}{\varepsilon +mh_{i}} \phi _{i}(m) \quad (using \,\,\, m < r^{*}/2). \end{aligned}$$

Again, for $i=3M/4+1,\ldots ,M-1$, we have

$$\begin{aligned}&{\mathcal {L}}_\varepsilon ^{M}\phi _{i}(m) =-\varepsilon \delta ^2\phi _{i}(m) + r_{i} D^{0}\phi _{i}(m) + s_{i} \phi _{i}(m) + t_{i} \phi _{i-M/2}(m) \\&\quad =\Bigg [ \dfrac{m}{h_{i}+h_{i+1}}\bigg \{ \dfrac{h_{i}}{\varepsilon +mh_{i}}(r_i - 2m)+ \dfrac{h_{i+1} r_i}{\varepsilon }\bigg \} + s_{i} \\&\qquad +t_{i}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_1}\bigg )^{M/4}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_2}\bigg )^{M/4}\Bigg ]\phi _{i}(m) \\&\quad =\Bigg [ \dfrac{m}{h_{i}+h_{i+1}}\bigg \{ \dfrac{h_{i}}{\varepsilon +mh_{i}}(r_i - 2m)+ \dfrac{h_{i+1} r_i}{\varepsilon }\bigg \} + s_{i}+t_{i} \\&\qquad +t_{i}\bigg \{\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_1}\bigg )^{M/4}\bigg (\dfrac{\varepsilon }{\varepsilon + m {\tilde{h}}_2}\bigg )^{M/4}-1\bigg \}\Bigg ]\phi _{i}(m) \\&\quad \ge \dfrac{C}{\varepsilon } \phi _{i}(m). \end{aligned}$$

$\square$

Lemma A.2

For each i and $0<m<r^{*}/2$, the mesh function $\phi _{i}(m)$ satisfies

$$\begin{aligned} \exp \bigg (\dfrac{-m(1-y_i)}{\varepsilon }\bigg )\le \dfrac{\phi _i (m)}{\phi _{M/2} (m)},\quad 1\le i \le M/2-1, \quad \,\, \end{aligned}$$

(A.1)

and

$$\begin{aligned} \exp \bigg (\dfrac{-m(2-y_i)}{\varepsilon }\bigg )\le \dfrac{\phi _i (m)}{\phi _{M} (m)}, \quad M/2+1\le i \le M-1. \end{aligned}$$

(A.2)

Proof

We have,

$$\begin{aligned} \mathrm {e}^{-mh_j/\varepsilon }= (\mathrm {e}^{mh_j/\varepsilon })^{-1}\le \bigg (1+\dfrac{m h_j}{\varepsilon }\bigg )^{-1} \,\,\text {for each}\,\, j=1, \ldots , M. \end{aligned}$$

Multiplying the above inequalities for $j= i+1,\ldots , M/2$, we obtain

$$\begin{aligned} \mathrm {e}^{-m(h_{i+1}+\cdots +h_{M/2})/\varepsilon }\le \prod _{j=i+1}^{M/2} \bigg (1+\dfrac{m h_j}{\varepsilon }\bigg )^{-1}, \end{aligned}$$

or,

$$\begin{aligned} \mathrm {e}^{-m(1-y_i)/\varepsilon }\le \dfrac{\displaystyle \prod _{j=i+1}^{M}\bigg (1+\dfrac{m h_j}{\varepsilon }\bigg )^{-1}}{\displaystyle \prod _{j=M/2+1}^{M}\bigg (1+\dfrac{m h_j}{\varepsilon }\bigg )^{-1}}=\dfrac{\phi _i (m)}{\phi _{M/2} (m)}, \quad for \quad 1\le i \le M/2-1. \end{aligned}$$

$\square$

Lemma A.3

For $0<m<r^{*}/2$, the following inequalities hold true

$$\begin{aligned} \dfrac{\phi _{i} (m)}{\phi _{M/2} (m)}\le C L^{2m \tau _{0}(1-2i/M)} M^{-2m \tau _{0}(1-2i/M)}, \quad M/4\le i \le M/2, \end{aligned}$$

and

$$\begin{aligned} \dfrac{\phi _{i} (m)}{\phi _{M} (m)}\le C L^{2m \tau _{0}(2-2i/M)} M^{-2m \tau _{0}(2-2i/M)}, \quad 3M/4\le i \le M-1. \end{aligned}$$

Proof

For $M/4 \le i \le M/2$, we have

$$\begin{aligned} \dfrac{\phi _{i} (m)}{\phi _{M/2} (m)}&=\prod _{j=i+1}^{M/2}\bigg (1+\dfrac{m h_j}{\varepsilon }\bigg )^{-1}\\&\le \exp \bigg (\dfrac{-m(y_{M/2}-y_{i})}{\varepsilon +m {\tilde{h}}_2}\bigg ), \quad where \,\, {\tilde{h}}_2=4\tau _{0}\varepsilon M^{-1} L \\&=\exp \bigg (\dfrac{-m(1-y_{i})}{\varepsilon +m {\tilde{h}}_2}\bigg ) \\&=\exp \bigg (\dfrac{-m(M/2-i) {\tilde{h}}_2 }{\varepsilon +m {\tilde{h}}_2}\bigg )\\&=\exp \bigg (\dfrac{-2m\tau _{0}(1-2i/M) L }{1+4m\tau _{0} M^{-1} L}\bigg ). \end{aligned}$$

Using $e^{-L}\le L/M$, we get

$$\begin{aligned} \dfrac{\phi _{i} (m)}{\phi _{M/2} (m)} \le L^{\frac{2m\tau _{0}(1-2i/M)}{1+4m\tau _{0} M^{-1}\ln M}} M^{\frac{-2m\tau _{0}(1-2i/M)}{1+4m\tau _{0} M^{-1}\ln M}} \le C L^{2m \tau _{0}(1-2i/M)} M^{-2m \tau _{0}(1-2i/M)}. \end{aligned}$$

Using similar arguments, we get the desired inequality for $3M/4\le i \le M-1$. $\square$