Introduction

Socio-spatial polarisation is increasing in many large cities throughout Europe (Tammaru et al. 2016). Socio-spatial polarisation refers to the process where the gap between the rich and the poor is increasing, which is translated into spatial segregation along ethnic or socioeconomic lines. In the European context, this has resulted in distinctive spatial patterns in large cities where the rich are increasingly located in historic city centres, while the poor reside in the more disadvantaged outer-city neighbourhoods (cf. Van Eijk 2010). Despite substantial government investments to counteract such socio-spatial polarisation over the past few decades, this process seems to be persistent, though it varies over time and between places (Bailey 2012).

In most of the studies on socio-spatial polarisation the continuous dynamic character of neighbourhoods is neglected, reducing neighbourhood change to the difference between two points in time. However, neighbourhoods are constantly changing in their population composition as a result of residential mobility and demographic events, thereby changing the aggregate status of neighbourhoods. Many studies investigating neighbourhood change focus on exceptional cases of gentrifying or declining neighbourhoods (e.g. Bailey 2012; Jivraj 2013; Hochstenbach and Van Gent 2015). Although these studies have provided important insight in the drivers behind neighbourhood change, they are typically limited to time-specific case-studies in particular cities. As a result, we do not know if neighbourhoods with similar characteristics experience similar processes of change over time – or if processes of gentrification or downgrading are the exception to the rule. In addition, we have limited understanding of how processes of gentrification and downgrading affect other neighbourhoods. As neighbourhoods do not operate in a societal and policy vacuum, changes in one neighbourhood are likely to affect other neighbourhoods as well. It has, for example, been argued that processes of urban restructuring or gentrification are likely to lead to new concentrations of deprivation in other neighbourhoods through the displacement of low-income groups (Bolt et al. 2009). As such, the upgrading of one neighbourhood might go hand-in-hand with the deterioration of another neighbourhood (Musterd and Ostendorf 2005; Bråmå 2013).

In addition, many studies in this field rely on percentile shifts and point-in-time measures to analyse change, neglecting the possibility that development over time might be more non-linear than linear or need much more time to take effect (see also Van Ham and Manley 2012). Because the physical structure of neighbourhoods hardly changes, neighbourhoods can maintain their overall status for longer periods of time (Meen et al. 2013; Tunstall 2015). However, selective mobility and demographic events lead to a constantly changing population composition (Van Ham et al. 2013). In this paper we argue that to fully understand processes of neighbourhood change, the next step in neighbourhood research is to focus on detailed neighbourhood trajectories and to identify typologies of neighbourhood change over longer periods of time. Analysing interrelated neighbourhood trajectories and understanding why some neighbourhoods are more prone to change than others is therefore highly relevant to the debate on spatial manifestations of inequality and neighbourhood development.

In this paper, we present an approach for analysing neighbourhood change by focussing on long-term neighbourhood change combined with a detailed analysis of neighbourhood trajectories. Focussing on the trajectories of low-income neighbourhoods in the Netherlands over the period 1971–2013, we analyse the role of physical characteristics in neighbourhood change. In the Dutch context, neighbourhood and housing quality is often related to the debate on neighbourhood change, however, few empirical studies try to analyse to what extent physical characteristics are related to today’s spatial patterns. Different starting positions in terms of housing quality can have long-lasting effects on neighbourhood statuses through processes of path-dependency (Meen et al. 2013). In addition, because the Dutch government has invested heavily in urban restructuring by changing the share of owner-occupied and social rented dwellings in particular neighbourhoods, we analyse the effect of demolition and construction on the different neighbourhood trajectories. Changes in the housing stock generate mobility processes and may thus affect neighbourhoods in both direct and indirect ways.

To analyse neighbourhood trajectories we use a combination of methods. Sequence analysis allows for the analysis of complete pathways through time and is therefore a promising method for longitudinal neighbourhood research. Sequence analysis is gaining popularity in the social sciences and is increasingly used by researchers interested in patterns of socio-spatial inequalities (e.g. Coulter and Van Ham 2013; Van Ham et al. 2014; Hedman et al. 2015). However, sequence analysis is ultimately a descriptive method and its potential for explaining trajectories is limited. Researchers have therefore developed a methodological framework that combines sequence analysis and a tree-structured discrepancy analysis, allowing for the analysis of the relationship between covariates and sequences (Studer et al. 2011). As such, this framework can provide insight in how different covariates affect neighbourhood trajectories in different ways. To our knowledge, this paper offers the first empirical application of this combination in the field of urban research, constituting a new approach towards researching neighbourhood dynamics and a move towards the visualisation and analysis of complex trajectories. In this paper, we only highlight the most important aspects of the combination between sequence analysis and the tree-structured discrepancy analysis. Based on our presentation, researchers should be able to get a basic understanding of both methods (for a full understanding of sequence analysis researchers are referred to Gabadinho et al. 2011; for the tree-structured discrepancy analysis to Studer et al. 2011).

The remainder of this paper is organised as follows. We start with expounding our approach for analysing neighbourhood change. We then move to describe the combination of sequence analysis and the tree-structured discrepancy analysis in more detail. In the “Data and Methods” section, we elaborate on the structure of the dataset and the methodological choices made. We then discuss the substantive results and reflect on the applicability of the methods for neighbourhood research.

Longitudinal Neighbourhood Change

Time is an important dimension in neighbourhood research. There are generally two viewpoints on this: one emphasises the general stability of neighbourhood status over longer periods of time as a result of path-dependency (Dorling et al. 2007; Meen et al. 2013). Another viewpoint argues that neighbourhoods are highly dynamic and are constantly experiencing population change (Van Ham et al. 2013). These two views on neighbourhood change are however rather complimentary than competing. On the one hand, neighbourhoods are indeed very dynamic and are constantly changing in their population composition as a result of residential mobility and demographic events (Simpson & Finney, 2009; Van Ham et al. 2013). On the other hand, because the housing stock of neighbourhoods is rather static, the overall socio-economic status of neighbourhoods does not change much over time (Meen et al. 2013). In other words: because the physical spatial structure of neighbourhoods remains broadly unchanged, similar types of residents move in and out of these neighbourhoods, thereby maintaining the status quo.

This is not to say that there are no changes in neighbourhood status at all: neighbourhoods can experience processes of decline or gentrification over time because the population in-situ experiences changes in employment status (Bailey 2012), or because of selective out- and inflow of different income groups (Van Ham et al. 2013). However, extreme processes of decline or gentrification whereby neighbourhoods experience a complete transformation of their population composition and overall status are rare (Cortright and Mahmoudi 2014; Tunstall 2015). Moreover, when neighbourhoods experience processes of decline or gentrification, the effects of these processes on the urban mosaic are often only visible after longer periods of time (e.g. Hulchanski 2010).

When such extreme changes do occur, they can often be explained by the physical quality of the neighbourhood. Processes of gentrification have been related to the desirable location and high quality and architectural aesthetics of pre-war or other historic neighbourhoods (e.g. Zukin 1982, 2010; Bridge 2001). As higher income groups gradually move into these neighbourhoods, house values and prices go up, thereby pushing lower income households out. In a similar vein, many unattractive post-war neighbourhoods have experienced processes of extreme neighbourhood decline over the past few decades. Researchers have argued that this decline can be explained by the low quality of and technical problems with dwellings and neighbourhoods built after the Second World War (Prak and Priemus 1986; Van Beckhoven et al. 2009).

In the Netherlands, these extreme processes of neighbourhood decline in post-war neighbourhoods (built between 1945 and 1970) led to the development of large-scale urban restructuring programmes. These urban restructuring programmes were aimed at creating a social mix in these neighbourhoods by demolishing social housing and constructing more upmarket owner-occupied or rental dwellings (Kleinhans 2004). Researchers have argued that urban restructuring programmes have led to minor improvements in the socio-economic position of these neighbourhoods (Permentier et al. 2013; Kleinhans et al. 2014). This can be explained by the fact that while a large number of social rented dwellings has been demolished, the overall share of social housing remained high in most restructuring neighbourhoods (Dol and Kleinhans 2012). Urban restructuring is only effective in reducing socio-spatial segregation when a substantial part of the social housing stock in a neighbourhood is replaced by owner-occupied dwellings (Bolt et al. 2009). Quite often (part of) the original residents in restructuring neighbourhoods moved back in the newly constructed dwellings. This meant that while these neighbourhoods have experienced a physical upgrade; the socio-economic status of the population remained largely unchanged (see e.g. Kleinhans et al. 2014).

There are thus two important, yet related, gaps in the literature on neighbourhood change. First, many studies focus on exceptional cases of change involving gentrification, downgrading or urban restructuring in particular cities or neighbourhoods, failing to answer the question if neighbourhoods with similar characteristics experience similar changes over time. Second, few studies have analysed the role of path-dependency of the physical characteristics of neighbourhoods in processes of change for a large sample of neighbourhoods in different cities. As a result, we have little insight into which neighbourhoods are more prone to change than others. Analysing the effects of physical characteristics and/or physical changes on neighbourhood trajectories is important for our understanding of why some neighbourhoods experience change while others remain stable for longer periods of time and help to answer the question which neighbourhood characteristics are predictors of future processes of change.

However, research on neighbourhood change is complicated because neighbourhoods have different starting positions, may experience different paces and processes of change over time, and the effects of changes in context might be non-linear or might only be visible after longer periods of time (Van Ham and Manley 2012). To fully capture patterns of neighbourhood change, it is therefore necessary to adopt a twofold approach: 1) Change should be analysed over longer periods of time (20–40 years) to capture the effects of longer term processes; and 2) The focus should be on continuous change of neighbourhood trajectories instead of simply comparing two points in time. As such, a dual approach would contribute to the identification of neighbourhood change typologies providing insight in (the drivers of) different spatial dynamics.

Analysing Neighbourhood Trajectories

The methods for analysing trajectories are limited: the most common statistical methods treat time as another level (in multilevel models), as dummy variables (in regression models), or as growth curves (time-series models). While all of these models have their advantages and disadvantages for studying change over time, they generally do not easily allow for the identification of patterns of change. Sequence analysis, a method that originates from the biological sciences to map DNA patterns, however, allows for the study of patterns of change and is gaining increasing popularity within the social sciences because of its ability to show complete pathways. Social researchers are using sequence analysis to explore class careers (Halpin and Chan 1998), labour market patterns (Abbott and Hrycak 1990; Pollock et al. 2002; McVicar and Anyadike-Danes 2002; Brzinsky-Fay 2007), family histories (Elzinga and Liefbroer 2007), and life-course trajectories (Billari and Piccarreta 2005; Wiggins et al. 2007; Martin et al. 2008).

The main goal of sequence analysis is to explore trajectories of subjects (individuals, neighbourhoods, et cetera) over time and to identify groups of subjects that experience similar trajectories (see also Gabadinho et al. 2011). Sequences are comprised of different states that show the order and duration that the individual subject occupied in each state. Focussing on neighbourhood trajectories, a neighbourhood can, for example, be in the 6th socio-economic neighbourhood category in 1971, then move up to the 5th category in 1999, and the 4th category in 2000, to end up in the 3th category in 2013. The neighbourhood categories in this example represent the different states that a neighbourhood can move through. The sequence of this particular neighbourhood would then look like this: 6th category -5th category -4th category -3rd category. This is an example of the most straightforward state sequence format (STS), however, other sequence representations are also possible (for a detailed understanding of state sequence representations, see Gabadinho et al. 2011). All sequences together can then be visualised as a series of individual neighbourhood trajectories, which represent how each neighbourhood moves through the different states over time. There are different ways to visualise sequences depending on the objective of the researcher (see Gabadinho et al. 2011).

Many researchers are however interested in going a step further and explain variation in sequences. For that reason, sequence analysis is often combined with cluster analysis where similar sequences are clustered together. However, cluster analysis has several disadvantages. First of all, the clusters can be very arbitrary because different algorithms generate different clusters. In addition, cluster membership tends to be unstable and the optimal number of clusters is very difficult to assess (see also Studer 2013). Cluster analysis reduces sets of sequences to a number of standard trajectories which are a rather crude approximation that consider deviations from the standard as noise (Studer et al. 2011).

In a few recent papers Studer and colleagues (2010, 2011, 2012, 2013) indicate a tree-structured discrepancy analysis as a valuable alternative to cluster analysis. The advantage of this method over cluster analysis is that a tree-structured discrepancy analysis does not create a number of groups that is supposedly representative for the entire population, instead it shows the effect of different variables on the set of sequences in a stepwise approach. Discrepancy analysis is similar to the analysis of variance (ANOVA)-types of analyses and measures the variability between sequences (Studer et al. 2011). The researcher can select a number of explanatory variables which are hypothesised to be related to the different sequences. Based on these predictor variables, the tree-structured discrepancy analysis will group similar sequences together. This is done by using the pairwise dissimilarities between sequences to compute the discrepancy within groups (Studer et al. 2010, 2011). In practice, this means that two sequences are compared to determine to what extent they are different from one another. This level of mismatch is then quantified by the dissimilarity measure (Studer and Ritschard 2016). In this paper, we use Optimal Matching distances to quantify dissimilarity. Optimal Matching computes the distance between pairs of sequences using a chosen cost scheme. This cost scheme constitutes of (1) insertion and deletion costs (indel) which capture whether the same state occurs in two sequences, and (2) substitution costs that focus on the timing of states and whether the same state occurs at the same time point in two sequences (Aisenbrey and Fasang 2010). Here we have set the indel costs to one and we base the substitution costs on the inverse transition frequencies between different states, which is in line with previous studies (e.g. Aassve et al. 2007; Widmer and Ritschard 2009; Barban 2013; Kleinepier et al. 2015). This means that we are more focussed on distinct trajectories (i.e. a change from the 1st category to the 6nd category is considered to be more costly than a change from the 1st category to the 2nd category) than on timing (i.e. we place less importance on differences in neighbourhood states at different points in time). We have replicated our results using a different dissimilarity matrix to ensure robustness. We have used Optimal Matching with indel costs of 1 and substitution costs of 2, which is equivalent to the Longest Common Subsequence distance (Studer and Ritschard 2016). All of our results remain the same.

There are different ways to measure dissimilarity and the choice of dissimilarity algorithm has been subject to debate for many years (see Abbott and Tsay 2000; Aisenbrey and Fasang 2010; Gabadinho et al. 2011). Different dissimilarity measures focus on different aspects of the trajectories; researchers interested in change are advised to use one of the Optimal Matching algorithms; researchers focussed on timing should employ one of the Hamming algorithms; while researchers interested in duration are recommended to use algorithms such as the Longest Common Subsequence, Chi2 or Euclidian distances (for an excellent overview, see Studer and Ritschard 2016). Optimal Matching remains the most popular dissimilarity matrix used in the social sciences because of its flexibility and can generally be used to understand the ‘common narrative’ between trajectories (Elzinga and Studer 2015).

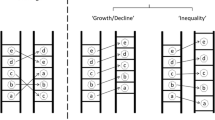
The tree-structured discrepancy analysis visualises the relationship between predictor variables and the sequences trajectories. The tree starts with all sequences in an initial group. The tree-structured discrepancy analysis then selects the most important (significant) predictor and its most important values to split the group into two distinctly different groups using the dissimilarity measure and a pseudo R2 and a pseudo F test. Significance is assessed through permutation tests (5000 permutations are sufficient to assess the results at the 1 % significance level, see Studer et al. 2011). Looking at, for example, the share of social housing, the model identifies the threshold value at which the sequences differ most, resulting in two significantly different groups of sequences that show different trajectories below and above the threshold value. In practice, this could mean that the model illustrates the trajectories for a group of neighbourhoods with low shares of social housing and a group of neighbourhoods with high shares of social housing. For each of the newly created groups, the discrepancy analysis splits the groups into two again, using the second most important predictor and its values (for that group) for which the highest pseudo R2 is found. Using our example, for the group of neighbourhoods with high shares of social housing, the model then shows the effect of a different variable, again creating two groups that show distinctly different trajectories. The process is repeated until a stopping criterion is reached or when a non-significant F for the selected split is encountered (Studer et al. 2010). The overall quality of the model can be assessed through the pseudo F test and the pseudo R2 that provide information on the statistical significance of the tree and the part of the total discrepancy explained, respectively (Studer et al. 2010).

A tree-structured discrepancy analysis can be seen as the next step in sequence analysis and contributes to the creation of meaningful groups of sequences (Studer et al. 2011). In this paper, we adopt an exploratory approach and use the tree-structured discrepancy analysis to understand how variation in neighbourhood sequences can be explained by the physical characteristics of neighbourhoods.

Data and Methods

Data and Measures

Research on neighbourhood change ideally requires individual-level georeferenced data at short-time intervals over a longer period of time. Unfortunately, in many countries, such longitudinal data are unavailable or inconsistent through time. Researchers are therefore confronted with a trade-off between data quality and data availability. This paper used longitudinal register data from the System of social Statistical Datasets (SSD) from Statistics Netherlands. For 1999 to 2013, we have data for the full Dutch population. Historic neighbourhood-level data from before the 1990s is extremely scare in the Netherlands due to the move from a census based system to a register based system. The last Dutch census was conducted in 1971, and the alternative country-wide individual-level registration system was installed by 1995. Data on neighbourhood income levels is however only available from 1999 onwards, hence our focus on 1999 to 2013. Combining the recent register data with the last census from 1971 allowed us to analyse long-term neighbourhood change, however, this meant that there was a 28-year time-gap in our dataset. Nevertheless, the inclusion of 1971 data provides a unique viewpoint on long-term neighbourhood change in the Dutch context.

Our definition of a neighbourhood is based on 500 by 500 m grids. The use of 500 by 500 m grids enabled the comparability of geographical units over time (as other administrative definitions of neighbourhoods have changed drastically over the last 40 years) and allowed for a detailed analysis on a relatively low level of aggregation. We focussed on the 31 largest cities of the Netherlands, resulting in a total of 8917 500 by 500 m grids (including newly constructed neighbourhoods in the period 1971–2013). The choice for the 31 largest cities in the Netherlands is related to the scale of urban restructuring programmes over the past few decades and can therefore be understood as a political construct. To ensure the stability of spatial boundaries over time, we used the city boundaries of 2013. Because of the high density in these cities, the average grid consists of 900 residents. For privacy reasons, grids with less than 10 residents have been excluded from the analyses. 

We analysed changes in the share of low-income households in neighbourhoods over time. Low-income households are defined as the bottom 20 %, which in 1971 included households with an income below 8000 guilders and in 2013 households with an income below 17167 euros. Neighbourhoods have been categorised according to their share of low-income households into deciles. Because there were few neighbourhoods with more than 50 % low-income households, the last four deciles have been grouped together.

To examine the role of the physical characteristics of neighbourhoods on their trajectories over time, we have included several control variables. We first included a dummy variable for the four largest cities in the Netherlands (Amsterdam, Rotterdam, Utrecht and the Hague) because we expected more dynamics in big cities. To analyse the path-dependency of neighbourhood quality, we included the share of social housing and the share of post-war housing in 1971. We included the change in the share of owner-occupied dwellings between 1971 and 2013 as an indicator for high-quality construction. To assess the effect of changes to the physical structure, we analysed the effect of the demolition, defined as the cumulative number of demolished post-war rental dwellings over the period 1999 to 2013. We have no information on demolition in 1971, however, as many post-war dwellings were still relatively new in 1971 and as large-scale urban restructuring of post-war areas started in the 1990s, it is highly unlikely that the demolition of post-war rental dwellings in 1971 was more than incidental. A summary of all the variables used in the analyses is presented in Table 1.

Table 1 Summary of the dataset
Full size table

Methods

To provide a detailed illustration of long-term neighbourhood change, we first zoomed in on Amsterdam and Rotterdam. We visualised how the spatial distribution of low-income neighbourhoods has changed in Amsterdam and Rotterdam between 1971 and 2013. Amsterdam and Rotterdam are the two largest cities in the Netherlands, but have experienced different neighbourhood trajectories over time. The economy of Amsterdam is characterised by a strong service sector, while Rotterdam’s economy remains tied to the harbour (Burgers and Musterd 2002; Hochstenbach and Van Gent 2015). The average income level of the population is therefore higher in Amsterdam (Hochstenbach and Van Gent 2015). Amsterdam has experienced strong gentrification in the past few decades, which is often ascribed to the historic architecture of inner-city neighbourhoods. Although some neighbourhoods in Rotterdam have also experienced processes of gentrification, the dominant process in Rotterdam has been neighbourhood downgrading since the 1970s (Hochstenbach and Van Gent 2015).

To come to a better understanding of patterns of neighbourhood change, we next focussed on neighbourhood trajectories of the 31 largest cities using a combination of sequence analysis and a tree-structured discrepancy analysis. We have first conducted a multifactor discrepancy analysis to assess the raw effects of the variables on the sequences trajectories (see Table 3). The multifactor approach offers insight in which covariates are significantly associated with the neighbourhood trajectories and provides information on the significance of the variables (using permutation tests) and the strength of the model using a pseudo F and a pseudo R2 (see also Studer et al. 2011).

We then combined sequence analysis and a tree-structured discrepancy analysis to analyse variation in neighbourhood trajectories. Sequence analysis is used for the visualisation of neighbourhood trajectories showing the neighbourhood status at each point in time using a colour scheme. Each neighbourhood category is assigned a different colour where the grey scheme represents the low to high neighbourhood status scale. There are different ways to visualise sequences (for an overview, see Gabadinho et al. 2011). In this paper, we used a sequence distribution plot showing the overall neighbourhood distribution instead of individual sequences. Importantly, this means that we are focussed on the general pattern of neighbourhood trajectories rather than individual neighbourhoods. The tree-structured discrepancy analysis then visualised how our control variables affect the trajectories in a tree-structured sequence plot (Studer et al. 2011). We have used the default stopping criteria of a p-value of 1 % for the F test (R = 5000), fixing the minimal group size at N = 446 (5 % of the total N = 8917), and allowing for the creation of five levels (see also Studer et al. 2011). The analyses were conducted in R version 3.2.1 “World-Famous Astronaut” (R Core Team 2015) using the TraMineR package (Gabadinho et al. 2011).

Results

We first zoom in on Amsterdam and Rotterdam in Figs. 1 and 2. Table 2 tabulates the neighbourhood categories in 1971 and 2013 for each city. Both illustrate a process of increasing poverty concentration these cities. Table 2 shows that the share of low-income neighbourhoods in the last two categories has remained stable over 40 years: the share of neighbourhoods with more than 40 % low-income households has not increased. However, the spatial distribution of these neighbourhoods is characterised by increasing spatial concentration as shown in Figs. 1 and 2. While both cities were characterised by a large share of high-income neighbourhoods (category 1) in 1971, they show more variation in the neighbourhood income distribution by 2013. 

Fig. 1
figure 1

Percentage low-income households in Amsterdam 1971 and 2013. Source: System of social Statistical Datasets, Statistics Netherlands, 2015

Full size image
Fig. 2
figure 2

Percentage low-income households in Rotterdam, 1971 and 2013. Source: System of social Statistical Datasets, Statistics Netherlands, 2015

Full size image
Table 2 Tabulation of the distribution of low-income households in neighbourhood category in Amsterdam and Rotterdam, 1971 and 2013
Full size table

The maps show the distribution of low-income households in 1971 and 2013. Figure 1 illustrates how inner-city neighbourhoods in Amsterdam have maintained their high status over time, while the post-war neighbourhoods at the outskirts of the city have experienced downgrading. Low-income neighbourhoods in Amsterdam are now increasingly concentrated outside the city centre (cf. Van Gent 2013). Figure 2 shows significant downgrading of large parts of Rotterdam over the last 40 years. Contrary to Amsterdam, Rotterdam’s inner city neighbourhoods have experienced downgrading, while the high-status neighbourhoods in the northern part of the city have maintained their status (cf. Hochstenbach and Van Gent 2015).

We are interested in the neighbourhood trajectories underlying the patterns described above and how these trajectories are related to a set of predictors. We are particularly interested in how the physical characteristics of neighbourhoods are associated with neighbourhood trajectories over time. As mentioned earlier, we have first conducted a multi-factor discrepancy analysis to assess the raw effect of our variables on the neighbourhood sequences. The results are shown in Table 3. The global statistics show that the model is significant (F = 43.584, R = 5000) with an R2 of 14.4 %, meaning that our set of variables provides overall significant information about the diversity of neighbourhood trajectories. All variables are significant at the 1 % level (assessed through 5000 permutations), with the exception of our dummy variable for the four largest cities. The share of social housing in 1971 and the number of demolished dwellings appear to be the most important predictors of neighbourhood trajectories between 1971 and 2013.

Table 3 Multifactor discrepancy analysis
Full size table

Figure 3 shows the tree-structured discrepancy analysis for the neighbourhood trajectories in the 31 largest Dutch cities. The initial node shows the distribution of neighbourhood states by year (box 1). Overall, the 31 largest cities are characterised by a more or less even distribution of neighbourhoods. Over time, the share of high-income neighbourhoods is decreasing while the share of low-income neighbourhoods is increasing.

Fig. 3
figure 3

Tree-structured discrepancy analysis of neighbourhood trajectories, 1971–2013. Source: System of social Statistical Datasets, Statistics Netherlands, 2015

Full size image

In the tree, the most significant variables and their most significant values are used in respective order. For each group, we see how the selected variable (and the threshold values of the variable) affects the neighbourhood trajectories, showing the group size, the within-discrepancy, and the R2 for that split. Our overall model has an R2 of 19.5 %, which is higher than the R2 from the multifactor discrepancy analysis, meaning that the tree has better explanatory power, which can be explained by the fact that the tree automatically accounts for interaction effects (Studer et al. 2011). Our neighbourhood characteristics explain 19.5 % of the variability in neighbourhood trajectories. We have forced the model to use the dummy variable for the four largest cities – Amsterdam, Rotterdam, the Hague and Utrecht – for its first split because we were interested to see how the trajectories of neighbourhoods in these large cities differ from the trajectories in the other cities. We find that neighbourhoods in the four largest cities (box 3) are characterised by more neighbourhood dynamics than the other cities (box 2). Since 1971, the four largest cities have experienced a substantial decrease in their share of high-income neighbourhoods and an increase in low-income neighbourhoods. The model shows that the share of social housing in 1971 is the most important indicator in explaining variance in neighbourhood trajectories in the four largest cities (box 6 and 7). The neighbourhoods with hardly any social housing in 1971 are characterised by high-income trajectories, while the neighbourhoods with higher shares of social housing show more downward trajectories. For this latter group, the number of demolished dwellings between 1999 and 2013 seems to matter (box 10 and 11). Demolition took place in neighbourhoods that were experiencing downgrading (box 11). These processes of decline were the reason for the Dutch government to target these neighbourhoods for urban renewal through the demolition of low-quality social rented dwellings (Kleinhans 2004).

The left side of the tree shows that changes in the share of owner-occupied dwellings between 1971 and 2013 is the most important predictor for neighbourhood trajectories in the other 27 cities (box 4 and 5). Box 4 consists almost solely of newly constructed neighbourhoods with high shares of owner-occupied dwellings since 1999. These neighbourhoods are characterised by more neighbourhood stability. Existing neighbourhoods that have seen increases in their share of owner-occupied dwellings are characterised by more downward trajectories (box 5). Here the share of owner-occupied dwellings interacts with the share of social housing in 1971. For those neighbourhoods that have seen an increase in the share of owner-occupied dwellings (box 5), the share of social housing seems to matter. Higher shares of social housing in 1971 are associated with more downward trajectories (box 9). For this latter group, higher increases in the share of owner-occupied dwellings are associated with more high-income trajectories (box 15). This interaction between the share of social housing in 1971 and changes in the share of owner-occupied dwellings captures the Dutch policy of social mixing by changing the tenure composition in neighbourhoods.

Discussion and Conclusion

Especially in the four largest Dutch cities, our results show an increase in the share of low-income neighbourhoods since 1971. Amsterdam and Rotterdam, in particular, have experienced increasing poverty concentrations in specific neighbourhoods. Most of these neighbourhoods were built after the Second World War and were characterised by concentrations of social housing. The Netherlands, historically, had a large social housing sector with relatively high-quality housing. Contrary to many other countries, social rented dwellings were inhabited by a mix of socioeconomic groups, not just low-income households (Van Kempen and Priemus 2002). In 1971, many post-war neighbourhoods were still relatively new and were considered to be high status neighbourhoods (Van Beckhoven et al. 2009). By 2013, these post-war neighbourhoods have experienced significant downgrading and are characterised by concentrations of poverty as is shown in Figs. 1 and 2. The downgrading of these neighbourhoods can be explained by their phsyical characteristics, in particular, the current low-quality housing and its multiple technical and physical problems. This, combined with relative downgrading due to new housing construction elsewhere, fuelled processes of neighbourhood decline (Prak and Priemus 1986; Kleinhans 2004). At the same time, this process led to the residualisation of the social housing stock in the Netherlands, where the social housing sector increasingly became the domain of low-income households (Van Kempen and Priemus 2002).

In the 1990s, the Dutch government launched large-scale urban renewal programmes to target the most disadvantaged neighbourhoods. In practice, this meant that many low-quality post-war social rented dwellings were demolished to make room for more expensive privately rented or owner-occupied dwellings (Kleinhans 2004). Fig. 3 captures this process very well: we see that demolition took place in downgrading neighbourhoods with relatively high shares of post-war rental dwellings in the four largest cities. At the same time, we see that the changes in the share of owner-occupied dwellings interacts with the share of social housing in 1971 in the other 27 cities. If we interpret a rising share of owner-occupied dwellings in these neighbourhoods as an indicator of the Dutch policy of mixing tenure, it then seems to be most effective in neighbourhoods that have experienced substantial increases in the share of owner-occupied dwellings, thereby contributing to more high-income trajectories (see also Bolt et al. 2009). The question however remains if such changes to the housing stock will lead to significant neighbourhood upgrading and to what extent these effects will be temporary or long-lasting (Van Ham and Manley 2012; Tunstall 2015; Zwiers et al. 2016).

Our analyses seem to indicate a high degree of path-dependency as the initial quality of dwellings and neighbourhoods was found to be associated with neighbourhood trajectories over time. While the four largest cities generally show a change towards a more equal neighbourhood distribution, there is some indication of increasing poverty concentration. Especially neighbourhoods with high shares of social housing in 1971 have experienced strong processes of neighbourhood decline. Zooming in on Amsterdam and Rotterdam in Table 2 and Figs. 1 and 2, we see that both cities were characterised by high shares of high-income neighbourhoods in 1971, but show more variation in neighbourhood income groups by 2013, albeit with more poverty concentration in many post-war neighbourhoods.

The main contribution of this paper is the introduction of a new method for exploring neighbourhood trajectories. Our empirical exercise confirms the need for an approach that incorporates both long-term neighbourhood changes and a more detailed analysis of neighbourhood trajectories, because neighbourhoods are extremely dynamic but the effects of downgrading and upgrading on neighbourhoods are only visible after longer periods of time. A focus on neighbourhood trajectories lends itself for the identification of different patterns of change over time. The combination of sequence analysis and a tree-structured discrepancy analysis contributes to an understanding of how changes in a particular group of neighbourhoods are related to the trajectories of other neighbourhoods. As such, these methods provide an integrated approach towards neighbourhood change, by focussing on trajectories and by identifying factors that contribute to changing trajectories over time. The analyses show how specific levels of change function as thresholds for a different direction of neighbourhood trajectories. It is however unclear to what extent these thresholds can be used as more than cut-off points. Future research should aim to explore the meaning of these thresholds for the identification of risk factors for neighbourhood change and its implications for spatial policy.

A tree-structured discrepancy analysis can be seen as the next step in sequence analysis, providing a new way of researching neighbourhood dynamics. The combination between sequence analysis and a tree-structured discrepancy analysis has proven to be a powerful tool to visualise and understand complex, contextualised patterns of change over time. These methods could contribute to an understanding of ‘when’, or ‘under what circumstances’, neighbourhood trajectories diverge in a particular direction, instead of ‘if’. Such research is necessary, because the time-period, frequency and composition of mechanisms that influence neighbourhood trajectories may be non-linear, can be temporary or long-lasting, may vary over time, and might be conditional on other factors (Galster 2012; Van Ham and Manley 2012).