Analytical solution of thermally developing heat transfer in circular and parallel plates microchannels

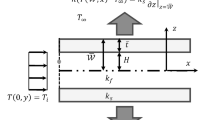
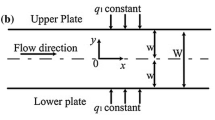
Abstract

The heat transfer characteristics of the thermally developing flow in the circular and parallel plates microchannels under the constant wall temperature and the constant heat flux are studied analytically. The energy equations are solved by using the separation of variables combined with the Gram–Schmidt orthogonalization. The effect of the number of eigenvalues on the calculation accuracy of the local Nusselt number is first determined. The temperature distribution and the heat transfer coefficient at the entrance region are calculated considering the effects of the rarefaction (0 < Kn < 0.1) and the axial heat conduction (Pe > 50). It is found that the axial heat conduction can dramatically improve the heat transfer of the thermally developing flow when the Peclet number is less than 250. But when the Peclet number is greater than 500, the effect of the axial heat conduction can be omitted. Enhancing the rarefaction would weaken the influence of the axial heat conduction on the heat transfer, and the difference of the local Nusselt number between the two boundary conditions decreases as increasing Kn. Enhancing fluid axial heat conduction would increase the thermal entrance length. The thermal entrance length of the microtube is 3–4 times that of the parallel plates microchannel, and the correlations of the thermal entrance lengths are developed, which may provide guidance for thermal design and optimization of microchannel heat sinks.

Appendix A: Steps of Gram-Schmidt orthogonalization to obtain C n

For the eigenfunctions sequence {ϕ_n}, assuming that the orthogonalized function sequences are {g_n}, the relationship between the above two is as follows

$$ g_{1} = \phi_{1} $$

(A-1)

$$ g_{2} = \phi_{2} - a_{21} g_{1} = \phi_{2} - a_{21} \phi_{1} $$

(A-2)

$$ \begin{gathered} g_{3} = \phi_{3} - a_{32} g_{2} - a_{31} g_{1} = \phi_{3} - a_{32} \left( {\phi_{2} - a_{21} \phi_{1} } \right) - a_{31} \phi_{1} \hfill \\ \, = \phi_{3} - a_{32} \phi_{2} + \left( {a_{32} a_{21} - a_{31} } \right)\phi_{1} \hfill \\ \end{gathered} $$

(A-3)

$$ g_{n} = \phi_{n} - \sum\limits_{m = 1}^{n - 1} {a_{nm} } g_{m} $$

(A-4)

For the orthogonal function sequences {g_n}, there is the following orthogonal property when i ≠ j

$$ \int_{0}^{1} {g_{i} g_{j} } {\text{d}}Y = 0 $$

(A-5)

Substituting (A-1) and (A-2) into (A-5) yields

$$ \int_{0}^{1} {g_{1} g_{2} } {\text{d}}Y = \int_{0}^{1} {\phi_{1} \left( {\phi_{2} - a_{21} g_{1} } \right)} {\text{d}}Y = \int_{0}^{1} {\phi_{1} \phi_{2} } {\text{d}}Y - a_{21} \int_{0}^{1} {\phi_{1} g_{1} } {\text{d}}Y = 0 $$

(A-6)

then

$$ a_{21} = \frac{{\int_{0}^{1} {\phi_{1} \phi_{2} } {\text{d}}Y}}{{\int_{0}^{1} {\phi_{1} g_{1} } {\text{d}}Y}} = \frac{{ < \phi_{1} ,\phi_{2} > }}{{ < \phi_{1} ,g_{1} > }} $$

(A-7)

More generally,

$$ a_{nm} = \frac{{ < \phi_{n} ,g_{m} > }}{{ < g_{m} ,g_{m} > }} $$

(A-8)

Based on the inlet boundary condition, setting the coefficient of the eigenfunctions after the orthogonalization is B_n, namely

$$ \sum\limits_{n = 1}^{\infty } {C_{n} \phi_{n} (Y)} = \sum\limits_{n = 1}^{\infty } {B_{n} g_{n} (Y)} $$

(A-9)

and

$$ \sum\limits_{n = 1}^{\infty } {B_{n} g_{n} (Y)} = \theta (0,Y) $$

(A-10)

The coefficient B_n can be obtained based on the characteristics of the orthogonal function:

$$ B_{n} = \frac{{\int_{0}^{1/A} {\theta (0,Y)g_{n} {\text{d}}Y} }}{{\int_{0}^{1/A} {g_{n}^{2} {\text{d}}Y} }} $$

(A-11)

According to (A-1)–(A-4), we have

$$ \begin{aligned} \sum\limits_{{n = 1}}^{\infty } {B_{n} g_{n} (Y)} & = B_{1} \phi _{1} + B_{2} \left( {\phi _{2} - a_{{21}} \phi _{1} } \right) + B_{3} \left( {\phi _{3} - a_{{32}} \phi _{2} } \right. \\ & \quad \left. { + a_{{32}} a_{{21}} \phi _{1} - a_{{31}} \phi _{1} } \right) + B_{4} \left( {\phi _{4} - a_{{43}} \phi _{3} + a_{{43}} a_{{32}} \phi _{2} } \right. \\ & \quad \left. { - a_{{43}} a_{{32}} a_{{21}} \phi _{1} + a_{{43}} a_{{31}} \phi _{1} - a_{{42}} \phi _{2} + a_{{42}} a_{{21}} \phi _{1} - a_{{41}} \phi _{1} } \right) \\ & \quad + \cdots B_{n} \left( {\phi _{n} - \sum\limits_{{m = 1}}^{{m - 1}} {a_{{nm}} } g_{m} } \right) \\ \end{aligned} $$

(A-12)

After determining B_n, C_n can be calculated according to (A-9), that is

$$ \begin{aligned} & C_{1} = B_{1} - B_{2} k_{1,2} - \cdots - B_{N} k_{1,N} = B_{1} - \sum\limits_{i = n + 1}^{N} {B_{i} k_{1,i} } \\ & \vdots \\ & C_{n} = B_{n} - B_{n + 1} k_{n,n + 1} - \cdots - B_{N} k_{n,N} = B_{n} - \sum\limits_{i = n + 1}^{N} {B_{i} k_{n,i} } \\ & \vdots \\ & C_{N} = B_{N} \\ \end{aligned} $$

(A-13)

where

$$ \begin{aligned} k_{n,m} &= a_{m,n} k_{n,n} - a_{m,n + 1} k_{n,n + 1} - \cdots - a_{m,m - 1} k_{n,m - 1} = a_{m,n}\\&\quad - \sum\limits_{i = n + 1}^{m - 1} {a_{m,i} k_{n,i} } \\ k_{n,n} &= 1 \\ \end{aligned} $$

(A-14)