Optimization of imperfect economic manufacturing models with a power demand rate dependent production rate

Abstract

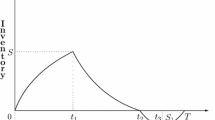
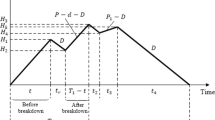
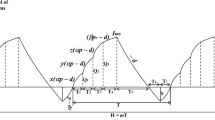
The constant demand rate is the most common assumption of the basic economic production quantity model, which is not very frequent in practice. In real world situations, demand usually varies with time. With regard to the widespread necessity of power demand pattern, demand is supposed to follow a power law. Another unrealistic assumption is perfect quality of all items. This paper presents a production system with defective items to determine the optimal replenishment quantity, cycle length and backordered size with a power demand rate dependent production rate. We assume that a manufacturer may be faced with three different cases regarding to the date that defective items are drawn from inventory. The set-up, backordering, inspection, and production costs, as well as holding cost of both perfect and imperfect items are accounted in the inventory system. An algorithm is offered to optimize total inventory cost and then numerical analyses are presented to demonstrate the applicability of the proposed models. Finally, some sensitivity analyses and managerial insights are provided.

Appendix A

Proposition 1

Equal $\left( {1 - x} \right)^{n} - \frac{{x^{n} }}{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)^{n} }} - \frac{{C_{b} }}{{C_{b} + C_{h} }} = 0$ has a unique solution $x^{*}$on $\left( {0,\frac{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)}}{{\left( {1 -\uplambda} \right)\alpha }}} \right)$.

Proof

Suppose that f(x) is a real function on [0,1] defined by:

$$ f\left( x \right) = \left( {1 - x} \right)^{n} - \frac{{x^{n} }}{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)^{n} }} - \frac{{C_{b} }}{{C_{b} + C_{h} }} $$

(a)

f(x) is continuous, strictly decreasing differentiable on the interval (0,1) because (notice that according to assumption 9, $\left( {1 - \lambda } \right)\alpha - 1 > 0$):

$$ f^{\prime}\left( x \right) = - n\left( {1 - x} \right)^{n - 1} - \frac{{nx^{n - 1} }}{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)^{n} }} < 0 $$

(b)

Also, we have $f\left( 0 \right) = \frac{{C_{h} }}{{C_{b} + C_{h} }} > 0$ and $f\left( {\frac{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)}}{{\left( {1 -\uplambda} \right)\alpha }}} \right) = - \frac{{C_{b} }}{{C_{b} + C_{h} }} < 0$. So, using the intermediate value theory, a point $x^{*}$ exists in the interval $\left( {0,\frac{{\left( {\left( {1 -\uplambda} \right)\alpha - 1} \right)}}{{\left( {1 -\uplambda} \right)\alpha }}} \right)$, where $y(x^{*} ) = 0$. Finally, because of that the function is decreasing on (0,1), the point $x^{*}$ is unique.

Proposition 2

The total cost function $TC_{I} \left( {s,T} \right)$ is strictly convex.

Proof

Using the second order derivatives of $TC_{I} \left( {s,T} \right)$ respect to decision variables, we have:

$$ \frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{{\partial s^{2} }} = \left( {C_{h} + C_{b} } \right)\left[ {\frac{{n\left( {s + rT} \right)^{n - 1} }}{{r^{n} T^{n} }} + \frac{{n\left( { - s} \right)^{n - 1} }}{{\left( {\left( {1 - \lambda } \right)\alpha - 1} \right)^{n} r^{n} T^{n} }}} \right] $$

(c)

$$ \frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{{\partial T^{2} }} = \left( {C_{h} + C_{b} } \right)\left[ {\frac{{ns^{2} \left( {s + rT} \right)^{n - 1} }}{{r^{n} T^{n + 2} }} + \frac{{n\left( { - s} \right)^{n + 1} }}{{\left( {\left( {1 - \lambda } \right)\alpha - 1} \right)^{n} r^{n} T^{n + 2} }}} \right] + \frac{{2C_{o} }}{{T^{3} }} $$

(d)

$$ \frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{\partial s\partial T} = \left( {C_{h} + C_{b} } \right)\left[ {\frac{{n\left( { - s} \right)\left( {s + rT} \right)^{n - 1} }}{{r^{n} T^{n + 1} }} + \frac{{n\left( { - s} \right)^{n} }}{{\left( {\left( {1 - \lambda } \right)\alpha - 1} \right)^{n} r^{n} T^{n + 1} }}} \right] $$

(f)

And the Hessian of the function $TC_{I} \left( {s,T} \right)$ is given by:

$$ \begin{aligned} H\left( {s,T} \right) & = \left[ {\frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{{\partial s^{2} }}} \right]\left[ {\frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{{\partial T^{2} }}} \right] - \left[ {\frac{{\partial^{2} TC_{I} \left( {s,T} \right)}}{\partial s\partial T}} \right]^{2} \\ & = \left( {C_{h} + C_{b} } \right)\left[ {\frac{{n\left( {s + rT} \right)^{n - 1} }}{{r^{n} T^{n} }} + \frac{{n\left( { - s} \right)^{n - 1} }}{{\left( {\left( {1 - \lambda } \right)\alpha - 1} \right)^{n} r^{n} T^{n} }}} \right]\left[ {\frac{{2C_{o} }}{{T^{3} }}} \right] > 0 \\ \end{aligned} $$

(g)

Eqs. (c) to (g) are positive, because in the region $\frac{{ - \left( {\left( {1 - \lambda } \right)\alpha - 1} \right)}}{{\left( {1 - \lambda } \right)\alpha }}rT \le s \le 0$, we always have $s + rT > 0$. Therefore, the function $TC_{I} \left( {s,T} \right)$ is strictly convex.