Skip to main content
SpringerLink
Log in

Lie symmetry analysis, abundant exact solutions and dynamics of multisolitons to the \((2+1)\)-dimensional KP-BBM equation

  • Research paper
  • Published:
Pramana Aims and scope Submit manuscript

Abstract

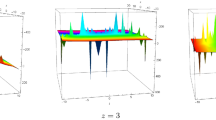
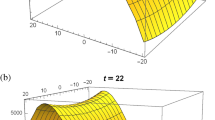
In the present article, our main aim is to construct abundant exact solutions for the \((2+1)\)-dimensional Kadomtsev–Petviashvili-Benjamin–Bona–Mahony (KP-BBM) equation by using two powerful techniques, the Lie symmetry method and the generalised exponential rational function (GERF) method with the help of symbolic computations via Mathematica. Firstly, we have derived infinitesimals, geometric vector fields, commutation relations and optimal system. Therefore, the KP-BBM equation is reduced into several nonlinear ODEs under two stages of symmetry reductions. Furthermore, abundant solutions are obtained in different shapes of single solitons, solitary wave solutions, quasiperiodic wave solitons, elastic multisolitons, dark solitons and bright solitons, which are more relevant, meaningful and useful to describe physical phenomena due to the existence of free parameters and constants. All these generated exact soliton solutions are new and completely different from the previous findings. Moreover, the dynamical behaviour of the obtained exact closed-form solutions is analysed graphically by their 3D, 2D-wave profiles and the corresponding density plots by using the mathematical software, which will be comprehensively used to explain complex physical phenomena in the fields of nonlinear physics, plasma physics, optical physics, mathematical physics, nonlinear dynamics, etc.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. V O Vakhnenko, E J Parkes and A J Morrison, Chaos Solitons Fractals 17(4), 683 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  2. X Lü. Nonlinear Dyn. 76(1), 161 (2014)

    Article  Google Scholar 

  3. W Malfliet and W Hereman, Phys. Scr. 54(6), 563 (1996)

    Article  ADS  Google Scholar 

  4. A M Wazwaz, Chaos Solitons Fractals 38(5), 1505 (2008)

    Article  ADS  MathSciNet  Google Scholar 

  5. A M Wazwaz, Appl. Math. Comput. 169(1), 700 (2005)

    MathSciNet  Google Scholar 

  6. M Song, C Yang and B Zhang, Appl. Math. Comput. 217(4), 1334 (2010)

    MathSciNet  Google Scholar 

  7. H Tam, W X Ma, X B Hu and D L Wang, J. Phys. Soc. Japan 69(1), 45 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  8. L Q Kong and C Q Dai, Nonlinear Dyn. 81(3), 1553 (2015)

    Article  Google Scholar 

  9. S Kumar, D Kumar and A M Wazwaz, Phys. Scr. 94, 065204 (2019)

    Article  ADS  Google Scholar 

  10. S Kumar, A Kumar and H Kharbanda, Phys. Scr. 133, 142 (2018)

    Google Scholar 

  11. S Kumar and A Kumar, Nonlinear Dyn. 98, 1891 (2019)

    Article  Google Scholar 

  12. D Kumar and S Kumar, Eur. Phys. J. Plus 135, 162 (2020)

    Article  Google Scholar 

  13. M Senthilvelan, Appl. Math. Comput. 123(3), 381 (2001)

    MathSciNet  Google Scholar 

  14. B Ghanbari and M Inc, Eur. Phys. J. Plus 133, 14 (2018)

    Article  Google Scholar 

  15. B Ghanbari, H Gnerhan, O A lhan and H M Baskonus, Phys. Scr. 95, 7 (2020)

    Google Scholar 

  16. L V Ovsiannikov, Group analysis of differential equations (Academic Press, Cambridge, 1982)

    MATH  Google Scholar 

  17. P J Olver, Applications of Lie groups to differential equations (Springer, Berlin, 1986)

    Book  Google Scholar 

  18. S Kumar, M Kumar and D Kumar, Pramana – J. Phys. 94: 28 (2020)

    Article  ADS  Google Scholar 

  19. S Kumar and S Rani, Pramana – J. Phys. 94: 116 (2020)

    Article  ADS  Google Scholar 

  20. G W Bluman and S Kumei, Symmetries and differential equations (Springer, Berlin, 1989)

    Book  Google Scholar 

  21. G W Bluman and J D Cole, Similarity methods for differential equations, Applied mathematical sciences (Springer-Verlag, New York Inc., 1974) vol. 13

  22. T B Benjamin, J L Bona and J J Mahony, Philos. Trans. Roy. Soc. London Ser. A 272, 47 (1972)

    Article  ADS  MathSciNet  Google Scholar 

  23. B B Kadomtsev and V I Petviashvili, Dokl. Akad. Nauk SSSR 192(4), 753 (1970)

    Google Scholar 

  24. M A Abdou, Appl. Math. Comput. 190(1), 988 (2007)

    MathSciNet  Google Scholar 

  25. J Manafian, M A S Murad, A Alizadeh and S Jafarmadar, Eur. Phys. J. Plus 135, 167 (2020)

    Article  Google Scholar 

  26. D V Tanwar and A M Wazwaz, Phys. Scr. 95(6), 065220 (2020)

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Mathematical Sciences, University of Delhi, Delhi, 110 007, India

    Sachin Kumar & Harsha Kharbanda

  2. Department of Mathematics, SGTB Khalsa College, University of Delhi, Delhi, 110 007, India

    Dharmendra Kumar

Authors
  1. Sachin Kumar
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dharmendra Kumar
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Harsha Kharbanda
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sachin Kumar.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kumar, S., Kumar, D. & Kharbanda, H. Lie symmetry analysis, abundant exact solutions and dynamics of multisolitons to the \((2+1)\)-dimensional KP-BBM equation. Pramana - J Phys 95, 33 (2021). https://doi.org/10.1007/s12043-020-02057-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12043-020-02057-x

Keywords

PACS Nos

Search

Navigation