Least number of n-periodic points of self-maps of $PSU(2)\times PSU(2)$

Abstract

Let $f:M\rightarrow M$ be a self-map of a compact manifold and $n\in {\mathbb {N}}$. In general, the least number of n-periodic points in the smooth homotopy class of f may be much bigger than in the continuous homotopy class. For a class of spaces, including compact Lie groups, a necessary condition for the equality of the above two numbers, for each iteration $f^n$, appears. Here we give the explicit form of the graph of orbits of Reidemeister classes $\mathcal {GOR}(f^*)$ for self-maps of projective unitary group PSU(2) and of $PSU(2)\times PSU(2)$ satisfying the necessary condition. The structure of the graphs implies that for self-maps of the above spaces the necessary condition is also sufficient for the smooth minimal realization of n-periodic points for all iterations.

1 Introduction

We consider a map $f:M\rightarrow M$ where M is a compact manifold, $\mathrm{dim}M\ge 3$ and we ask about the least number of n-periodic points in the homotopy class of f : $\displaystyle \min _{g} \{\#\mathrm{Fix}(g^n):g\sim f\}$. It turns out that the minimum depends on which homotopy class we consider: continuous or smooth. The difference was noticed in 1974 by Shub and Sullivan [19]. The reason of the gap between the minimal numbers of periodic points, in continuous and smooth category, is the following. Each integer sequence satisfying Dold congruences (see Sect. 2) can be obtained as $\mathrm{ind}(f^n;0)$ for a continuous self-map $f: {\mathbb {R}}^m\rightarrow {\mathbb {R}}^m$ ,in the isolated periodic point 0 and $n\ge 3$ . On the other hand if f is smooth then the sequence $\mathrm{ind}(f^n;0)$ must be periodic. The smooth map near a periodic point is close to its differential linear map and is not so flexible as the continuous map can be. Thus, to realize the necessary indices of Nielsen classes, we usually need more periodic points in the smooth case.

In [3], the restrictions on the sequence $\mathrm{ind}(f^n;0)$ for a smooth self-map f of ${\mathbb {R}}^m$ were given with the suggestion that they are the only restrictions. The last was confirmed by Thm.2.2 in [10] which gave rise to a generalization of the Nielsen periodic number $NF_n(f)$, introduced by Boju Jiang [18], to the smooth category and to an algebraic method of determining the least number of n-periodic points in the smooth homotopy class [8, 9]. In general, the gap between the numbers $NF_n(f)\le NJD_n(f)$ can be very large. The growth of the sequence $L(f^n)$ plays a role. When the growth is exponential, then the gap may be arbitrarily large. This happens for each self-map $f:S^m \rightarrow S^m$, $|\mathrm{deg}(f)|\ge 2$ , $m\ge 3$ [8].

In [13], we started to study the problem when the equality $NF_n(f)= NJD_n(f)$ holds. It was noticed by Cheng Ye You, much earlier in a geometric way, that the equality holds for each self-maps of a torus [20]. This result was generalized to self-maps of nilmanifolds and solvmanifolds in [17]. In general, the progress in the computation in Nielsen periodic theory was done mainly, or rather only, under the assumption that the map f is essentially reducibe and has the Jiang property [11, 12]. If we make these assumptions and we add the assumption of Haibao Duan that the Lefschetz numbers $L(f^k)= det (I-A^k),$ for an integer matrix A, then the necessary condition for the equality $NF_n(f)= NJD_n(f)$ appears.

The aim of this paper is to show that the above necessary condition is also sufficient for self-map of PSU(2) (the only quotient of SU(2)) and of $PSU(2)\times PSU(2)$. This follows from the detailed description of the graph of orbits of Reidemeister classes $\mathcal {GOR}(f^n)$ which captures all necessary data: splitting to Nielsen classes, fixed point indices of the classes and the homomorphisms between the sets of (orbits of) Reidemeister classes.

Remark 1.1

We avoid recalling here the definitions of $NF_n(f)$ and $NJD_n(f)$ which are very technical [16]. Instead we will use Theorem 3.5 which gives an equivalent condition for the equality of the two numbers.

Remark 1.2

The equality $NF_n(f)=NJD_n(f)$ guaranties a smooth deformation of f to a map with the minimal set of n-periodic points (see Theorem 4.4 in [9]). But we do not claim that the equality for all $n\in {\mathbb {N}}$ gives a map with the minimal number of periodic points for all iterations. Theorem 4.4 in [9] implies a deformation with the least number of periodic points only for a finite, arbitrarily prescribed, number of iterations.

2 Indices of iterations of a smooth map

We enlist basic information from Nielsen fixed and periodic point theory. For details see [15, 16, 18].

Let f be a $C^1$ self-map of ${\mathbb {R}}^m$ where 0 is an isolated fixed and periodic point for each iteration. We will also assume that f is smooth unless other stated. By smooth we mean $C^1$.

Albrecht Dold [4] noticed that a sequence of fixed point indices $A_k=\mathrm{ind}(f^k;x_0)$, where f is a continuous self-map of a Euclidean space ${\mathbb {R}}^m$ and $x_0$ is an isolated fixed point for each $f^k$, must satisfy the following congruences:

$$\begin{aligned} \sum _{k|n} \mu (n/k)\cdot \mathrm{ind}(f^k;x_0) \equiv 0 (modulo\; n) \end{aligned}$$

where $\mu$ denotes the $\hbox {M}\ddot{o}\hbox {bius}$ function.

Moreover, each sequence of integers $(A_k)$ satisfying Dold congruences can be realized as $A_k=\mathrm{ind}(f^k;x_0)$, for a continuous self-map of ${\mathbb {R}}^m$ for $m\ge 3$ [1]. In other words, Dold congruences are the only restrictions for the sequence of the fixed point index of a continuous map.

The basic Dold sequence is given by the formula $\mathrm{reg}_k(n)= {\left\{ \begin{array}{ll} k &{} \text {if} \;\; k \mid n, \\ 0 &{} \text {if}\;\; k \not \mid n. \end{array}\right. }$

Surprisingly, it turned out that there are much more restrictions on sequences $A_k=\mathrm{ind}(f^k;x_0)$ when f is smooth [3, 19].

Each integer sequence $(A_n)$ can be written down uniquely in the following form of a periodic expansion: $A_n= \sum _{k=1}^{\infty } a_k \mathrm{reg}_k(n),$ where $a_n=\frac{1}{n} \sum _{k|n} \mu (\frac{n}{k})\; A_k$. Then all coefficients $a_k$ are integers if and only if the sequence $(A_n)$ satisfies Dold congruences.

We will see that in the smooth case, there are much more restrictions for the sequence $\mathrm{ind}(f^k;x_0)$.

Definition 2.1

A sequence of integers $A_n$ will be called smoothly realizable in ${\mathbb {R}}^m$ (or in dimension m) if there exists a smooth self-map $f: {\mathbb {R}}^m \rightarrow {\mathbb {R}}^m$ and an isolated fixed point $x_0\in \mathrm{Fix}(f)$, which is also an isolated fixed point of each iteration $f^n$, so that $A_k=\mathrm{ind}(f^k;x_0)$.

We will use the following notation. For a finite subset $A\subset {\mathbb {N}}$, we denote by $\mathrm{lcm}(A)$ the least common multiple. Moreover we will define $\mathrm{LCM}(A)=\{\mathrm{lcm}(B); B\subset A\}$ and $\mathrm{LCM}_2(A)=\mathrm{LCM}(A\cup \{2\})$.

Theorem 2.2

Thm. 2.5 in [14] A sequence $(D_n)$ is smoothly realizable in dimension m if and only if there exist natural numbers $d_1,\ldots ,d_s$ ($d_i\ge 3$ ,$2s\le m$) so that

$$\begin{aligned} D_n= \sum _{k} \alpha _k\mathrm{reg}_k(n) \end{aligned}$$

(1)

where the summation runs through the set $\text { LCM }_2(\{d_1,\ldots ,d_s\}$ and the coefficients $\alpha _k$ are integers. If moreover $m\le 2s+2$ then the following restrictions hold

• [1] if $m=2s$ then $\alpha _1=1$ and LCM. Here LCM is the shorthand for : the restriction of summation (1) must run through $\text { LCM }(\{d_1,\ldots ,d_s\})$ (without this restriction the summation may run through $\text { LCM }_2(\{d_1,\ldots ,d_s\})$).

• [2] if $m=2s+1$ then ($|\alpha _1|\le 1$ and LCM) or ($\alpha _1=1$ and ($\alpha _2=0$ or 1))

• [3] if $m=2s+2$ then $|\alpha _1|\le 1$ or LCM.

$\square$

Remark 2.3

It is easy to notice that if $f:M\rightarrow M$ is a self-map of a compact manifold homotopic to a smooth map g and $\mathrm{Fix(g^n)}$ is a point then the sequence $L(f^k)_{k|n}$ is smoothly realizable in ${\mathbb {R}}^m$ where $m = \mathrm{dim}M$. It turns out that if moreover M is simply-connected and $\mathrm{dim}(M) \ge 3$ then the inverse implication is also true [8]; if the sequence $L(f^k)_{k|n}$ is smoothly realizable in ${\mathbb {R}}^m$ then f is homotopic to a smooth map g such that $\mathrm{Fix}(f^n)$ is a point.

3 Nielsen fixed point theory

In this Section, we enlist the basic information about the Nielsen fixed point theory. The most important information of this section to the rest of the paper are the graph of orbits Reidemeister classes (briefly, Reidemeister graph) and Theorem 3.5 which gives an algebraic, necessary and sufficient, condition for smooth realizability in a continuous homotopy class.

We consider a self-map of a compact connected polyhedron $f:X\rightarrow X$ and its fixed point set $\mathrm{Fix}(f)$. We define the Nielsen relation on this set by :

$x\sim y$ if there is a path $\omega$ joining x with y so that $f\omega$ and $\omega$ are fixed end point homotopic

This relation splits $\mathrm{Fix}(f)$ into Nielsen classes. Their set will be denoted by ${\mathcal {N}}(f)$. We say that a Nielsen class A is ${ essential}$ if its fixed point index is nonzero : $\mathrm{ind}(f;A)\ne 0$. The number of essential Nielsen classes is called Nielsen number and denoted N(f). This is a homotopy invariant and moreover it is the lower bound of the number of fixed points in the (continuous) homotopy class: $N(f)\le \min _{h\sim f} \# \mathrm{Fix}(h)$ [2, 16, 18].

On the other hand, we define the set of Reidemeister classes of the map f as the quotient set of the action of the fundamental group $\pi _1M$ on itself given by $\omega * \alpha = \omega \cdot \alpha \cdot (f_{\#}\omega )^{-1}$. We denote the quotient space by ${\mathcal {R}}(f)$. There is a natural injection from the set of the Nielsen classes to the set of Reidemeister classes ${\mathcal {N}}(f)\subset {\mathcal {R}}(f)$ defined as follows. We choose a point x in the given Nielsen class A and a path $\omega$ from the base point $x_0$ to x. Then the loop $\omega *(f\omega )^{-1}$ represents the corresponding Reidemeister class.

Now we consider the iterations of the map f. For fixed natural numbers l|k, there is a natural inclusion $\mathrm{Fix}(f^l)\subset \mathrm{Fix}(f^k)$ which induces the map ${\mathcal {N}}(f^l)\rightarrow {\mathcal {N}}(f^k)$ (which may be not injective). This map extends to $i_{kl}: {\mathcal {R}}(f^l) \rightarrow {\mathcal {R}}(f^k)$ and the last is given by

$$\begin{aligned} i_{kl}[x]=[x\cdot f^l(x)\cdot f^{2l}(x)\cdots f^{k-l}(x)] \end{aligned}$$

The functorial equalities are satisfied: $i_{kl}i_{lm}=i_{km}$ , $i_{kk}=\mathrm{id}$ and moreover the diagram

commutes.

The group ${\mathbb {Z}}_k$ acts on $\mathrm{Fix}(f^k)$ by

$$\begin{aligned} \mathrm{Fix}(f^k)\ni [x] \rightarrow [f(x)]\in \mathrm{Fix}(f^k) \end{aligned}$$

and on ${\mathcal {R}}(f^k)$ by

$$\begin{aligned} {\mathcal {R}}(f^k) \ni [a]\rightarrow [f_{\#}(a)]\in {\mathcal {R}}(f^k) \end{aligned}$$

Then the diagram

commutes. We denote by ${{\mathcal {OR}}}(f^k)$ the set of orbits of the above action (orbits of Reidemeister classes).

Consider Reidemeister classes $A\in {\mathcal {R}}(f^k)$, $B\in {\mathcal {R}}(f^l)$ , l|k , satisfying $i_{kl}(B)=A$. Then we say that A reduces to B , or B preceeds A and we write $B \preceq A$. The class is called reducible if $B \preceq A$ for a $B\ne A$. A similar definition works for orbits.

Let ${\mathcal {ER}}(f^k)$ , ${\mathcal {IR}}(f^k)$ denote sets of essential and irreducible classes, respectively.

A map f is called essentially reducible if each class $A\in {\mathcal {R}}(f^l)$ preceding an essential class is essential.

We will denote by $\mathcal {IEOR}(f)$, or simply $\mathcal {IEOR}$, the set of irreducible essential orbits of Reidemeister classes of f.

Corollary 3.1

Lie groups are essentially reducible [18]. $\square$

It is convenient to put all Reidemeister data into a directed graph $\Gamma$.

• Vertices are elements of the union $\mathrm{Vert}(\Gamma )= \bigcup _{k\in {\mathbb {N}}} {\mathcal {OR}}(f^k)$.

• There is a unique directed edge from $A\in {\mathcal {OR}}(f^l)$ to $B\in {\mathcal {OR}}(f^k)$ if $i_{kl}(A)=B$.

Moreover, to each vertex $A\in {\mathcal {OR}}(f^k)$, an integer $\mathrm{ind}(f^k;A)$ is defined, and the following Dold Congruences are satisfied

Lemma 3.2

(Lemma 3.3 in [9]) For each $B\in {\mathcal {OR}}(f^b)$ :

$$\begin{aligned} \sum _{C \preceq B} \mu (b/c)\cdot \mathrm{ind}(f^c:C) \equiv 0 (modulo\; b) \end{aligned}$$

where $\mu$ denotes the Mobius function and $C\in {\mathcal {OR}}(f^c)$. $\square$

Definition 3.3

• 1. For a fixed $A\in {\mathcal {OR}}(f^k)$ and a number $r\in {\mathbb {N}}$ we define $\mathrm{Reg}_A^r:\mathrm{Vert}(f) \rightarrow {\mathbb {Z}}$ by the formula

  $$\begin{aligned} \mathrm{Reg}_A^r(B)= {\left\{ \begin{array}{ll} k\cdot r &{} \text { if } i_{rk,k}(A) \preceq B \\ 0 &{} \text { otherwise } \end{array}\right. } \end{aligned}$$

• 2. More generally for each Dold sequence $d_k =\sum _l a_l\mathrm{reg}_l(k)$ and an orbit $A\in {\mathcal {OR}}(f^r)$ we define a function $C:\mathrm{Vert}(f^k)\rightarrow {\mathbb {Z}}$ as $C(B) =\sum _l a_l\mathrm{Reg}_A^l(B)$. We say then that the sequence $\sum a_l\mathrm{reg}_l$ is attached to the orbit A or that $D: \mathrm{Vert}(f^k)\rightarrow {\mathbb {Z}}$ comes from $\sum a_l\mathrm{reg}_l$.

Corollary 3.4

(Corollary 7.2 in [13]). A continuous essentially reducible map $f:M\rightarrow M$ realizes the least number of n-periodic points $\iff$ for each orbit $A\in \mathcal {IEOR}(f^k)$ (k|n), the corresponding orbit of Nielsen classes (also denoted by A) contains exactly one k-orbit of points ($A=\{a_1,\ldots ,a_k\}$) and all other orbits are empty. $\square$

Now we are in a position to make precise when the map in the above Corollary may be smooth.

Theorem 3.5

(Theorem (7.3) in [13]). An essentially reducible map $f:M\rightarrow M$ is homotopic to a smooth map g realizing the least number of n-periodic points $\iff$ one can attach to each orbit $A\in \mathcal {IEOR}(f^k)$ (k|n) an expression $C_A$, realizable in ${\mathbb {R}}^m$, so that

$$\begin{aligned} \mathrm{ind}(f^k;B)= \sum _{A\in {\mathcal {IEOR}}} C_A(B) \end{aligned}$$

for each $B\in {\mathcal {OR}}(f^k)$ , k|n. Here $C_A: \mathrm{Vert}(f)\rightarrow {\mathbb {Z}}$ is the function C given by Definition 3.3$\square$

The above Theorem and Theorem 2.2 allow to determine whether the least number of n-periodic point can be realized by a smooth map.

Definition 3.6

If the right-hand side in Theorem 3.5 holds then we say that the Reidemeister graph $\mathcal {GOR}(f)$ is smoothly realizable in dimension dim(M) for k|n. By Theorem 3.5 this is equivalent to a smooth minimization of ${ Fix}(f^n)$

We end the section by a remark which guaranties the partial realization of the index function.

Remark 3.7

(Remark 3.7 in [15]) We fix a map $f:M\rightarrow M$ and a number $d\in {\mathbb {N}}$ so that each sum $\sum _{l|d}a_l\cdot l$ is smoothly realizable in dimension $m=\mathrm{dim}M$ for k|d. We, moreover, assume that f is essentially reducible for k|d. Then the Reidemeister graph $\mathcal {GOR}(f)$ is smoothly realizable for k|d. $\square$

4 Duan’s cohomology space

We recall the class of rational exterior spaces introduced by H. Duan [5]. The basic property of such a space X is the existence of a finite dimensional vector space A(X) such that: for each self map $f:X\rightarrow X$ there is homomorphism $A(f):A(M)\rightarrow A(M)$ such that

$$\begin{aligned} L(f^k)=\mathrm{det}(I-A(f)^k) \end{aligned}$$

We are given a topological space X and we consider the rational cohomology $H^*(X;{\mathbb {Q}})$. An $x\in H^r(X;{\mathbb {Q}})$, is called decomposable if $x=\sum _i x_i\cup y_i$ for some $x_i\in H^{p_i}(X;{\mathbb {Q}})$ , $y_i\in H^{q_i}(X;{\mathbb {Q}})$ , $p_i+q_i=r$ , $p_i\ge 1, q_i\ge 1$. Let $D^r(X)\subset H^r(X;{\mathbb {Q}})$ denote the space over ${\mathbb {Q}}$ consisting of all decomposable elements. Then the quotient $A^r(X)=H^r(X;{\mathbb {Q}})/D^r(X)$ is a vector space over ${\mathbb {Q}}$. A continuous map $f:X\rightarrow Y$ induces $f^*_r: H^r(Y; {\mathbb {Q}}) \rightarrow H^r(X; {\mathbb {Q}})$ and $A^r(f):A^r(Y) \rightarrow A^r(X)$ for each $r\ge 0$. Let $A(X)=\oplus _{r=1}^{\infty }A^r(X)$ and $A(f):A(Y)\rightarrow A(X)$ be the induced homomorphism.

Example 4.1

If $H^*(M;{\mathbb {Q}})=\Lambda (a_{1},\ldots ,a_{s};{\mathbb {Q}})$ is the exterior algebra then $A^*(M)= {\mathbb {Q}}(a_{1},\ldots ,a_{s})$ is the free ${\mathbb {Q}}$-linear space and $A^r(M)$ is the subspace spanned by the above generators of degree r.

Definition 4.2

[5] A connected topological space X is called rational exterior if some homogeneous elements $\omega _i\in H^{odd}(X; {\mathbb {Q}})$, $i=1,\cdots , k$, can be chosen so that the inclusions $\omega _i\rightarrow H^*(X; {\mathbb {Q}})$ give rise to a ring isomorphism $\Lambda (\omega _1,\ldots ,\omega _k;{\mathbb {Q}})= H^*(X;{\mathbb {Q}})$. $\square$

Theorem 4.3

[5] (Duan) Let f be a self-map of a rational exterior space. Then $L(f^n)= det(I-(A(f))^n)$ for all $n \in {\mathbb {N}}$. $\square$

Let us recall that the least simple Lie group SU(2) is diffeomorphic to the sphere $S^3$ and that the center of SU(2) consists of two elements, hence SU(2) has a unique nontrivial quotient which we will denote PSU(2) and this group is diffeomorphic to $\mathbb {RP}^3$. Moreover $H^*(PSU(2);{\mathbb {Q}})=\Lambda ^*_{{\mathbb {Q}}}(a_3)$ , $A(PSU(2))= {\mathbb {Q}}$ and $\pi _1(PSU(2))= {\mathbb {Z}}_2$. Let $M=PSU(2)\times \cdots \times PSU(2)$ (n times). Then $H^*(M;{\mathbb {Q}})=\Lambda (a_3^1,\ldots ,a_3^n;{\mathbb {Q}})$ which yields the natural isomorphism $A(M)={\mathbb {Q}}\oplus \cdots \oplus {\mathbb {Q}}=H^3(M;{\mathbb {Q}})$. Moreover $\pi _1M={\mathbb {Z}}_2\oplus \cdots \oplus {\mathbb {Z}}_2$ (n times). A self-map $f:M\rightarrow M$ induces homomorphisms $f_3^*: H^3(M;{\mathbb {Q}}) \rightarrow H^3(M;{\mathbb {Q}})$ and $f_{\#}: \pi _1M\rightarrow \pi _1M$. Let the matrices $A(f)\in {\mathcal {M}}_{2\oplus 2}({\mathbb {Z}})$ and $B(f)\in {\mathcal {M}}_{2\oplus 2}({\mathbb {Z}}_2)$ represent $f^*$ and $f_{\#}$ respectively (in canonical bases coming from the decomposition $M=PSU(2)\times \cdots \times PSU(2)$).

Lemma 4.4

Under the above assumptions $B(f)\equiv (A(f))^T \mathrm{modulo}\; 2$. Here $^T$ denotes the transpose.

Proof

Let $n=1$. Then the map $f:PSU(2)\rightarrow PSU(2)$ of degree d induces the homomorphism $f_3^*$ of $H^3(PSU(2);{\mathbb {Q}})={\mathbb {Q}}$ given by $f^*_3(z)=d\cdot z$. On the other hand, the homomorphism $f_{\#}$ of $\pi _1PSU(2)={\mathbb {Z}}_2$ satisfies $f_{3}(\alpha )=0$ for d even and $f_{3}(\alpha )=1$ for d odd. Now $\alpha \equiv d\; \mathrm{modulo\;} 2$.

Let us fix an $n\in {\mathbb {N}}$. Let us denote $A(f)=[a_{ij}]$ and $B(f)=[b_{ij}]$. Now $a_{ij}= \mathrm{deg}(r_jfs_i)$ where $s_i(x)=(0,\ldots ,x,0,\ldots ,0)$ and $r_j(x_1,\ldots ,x_n)=x_j$. This implies $[a_{ij}]^T=[\mathrm{deg}(r_ifs_j)]$. On the other hand $b_{ij}= \alpha$ where $\alpha =0$ for $\mathrm{deg}(r_ifs_j)$ even and $\alpha =1$ for $\mathrm{deg}(r_ifs_j)$ odd. Thus $b_{ij}\equiv a_{ji} \; \mathrm{modulo} \; 2$. $\square$

5 Self-map of PSU(2)

Fig. 1

$\mathcal {GOR}$ for self-maps of PSU(2)

In the next sections, we will consider self-maps of PSU(2) and of $PSU(2)\times PSU(2)$. We will à priori assume that $+1$ is not an eigen-value A(f), since otherwise the Lefschetz numbers of all iterations disappear, and we also assume that all eigenvalues of A(f) have moduli $\le 1$. We will describe their Graph of Reidemeister classes. In particular, we will see that each iteration $f^k$ is homotopic to a smooth $g_k$ such that $\#\mathrm{Fix}(g_k^k)$ is minimal in the continuous homotopy class of f.

This section and each subsection of the next section end with the decomposition of the index function as the sum of smoothly realizable expressions. Now the smooth realizability of the map f follows from Theorem 3.5.

We consider $M=PSU(2)=S^3/antipodism= {\mathbb {R}}P^3$ and its self-map $f:M\rightarrow M$. Now $H^*(M;{\mathbb {Q}})=\Lambda ^*(a_3;{\mathbb {Q}})$ and $\pi _1 M={\mathbb {Z}}_2$. If $f^*(a_3)=ra_3$ then the necessary condition gives $r=0$ or $-1$ (+1 is excluded). If $r=0$ then $L(f^k)=1-0^k=1$ for all k so by Jiang property there is a single Reidemeister class in each ${\mathcal {OR}}(f^k)$. Now $\mathrm{}ind(f^k,*)=\mathrm{reg}_1(k)$ gives the desired decomposition and the graph is smoothly realizable in dimension 1.

Now we assume that $r=-1$. Since r is odd number, $f_{\#}=\mathrm{id}$. Since $A(PSU(2))= {\mathbb {Q}}=H^3(PSU(2);{\mathbb {Q}})$, $A(f)=f_3^*$ is given by $f^*_3(a)=\mathrm{deg}(f) a$. But by the assumption $\mathrm{deg}(f)=-1$ ($+1$ and 0 are excluded) so by Lemma 4.4$f_{\#}:\pi _1(PSU(2))\rightarrow \pi _1(PSU(2))= {\mathbb {Z}}_2$ is the identity.

Lemma 5.1

Let $f: PSU(2) \rightarrow PSU(2)$ satisfy $f_{\#}=\mathrm{id}$ and $f^*_3(a_3)=-a_3$ then

• 1. ${\mathcal {R}}(f^k)={\mathbb {Z}}_2$ for each $k\in {\mathbb {N}}$

• 2. $i_{kl}= {\left\{ \begin{array}{ll} id &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$

• 3. Each orbit is a single class.

• 4.
  $$\begin{aligned} L(f^k)=1-(-1)^k= {\left\{ \begin{array}{ll} 2 &{} \text {if} \;\; k \text { is odd}, \\ 0 &{} \text {if} \;\; k \text { is even}, \\ \end{array}\right. } \end{aligned}$$

  which implies the expansion $L(f^k)=2\mathrm{reg}_1(k)-\mathrm{reg}_2(k)$

• 5. The set of essential and irreducible classes $\mathcal {IEOR}(f)=\{1^0,1^1\}$ (two Reidemeister classes in ${\mathcal {R}}(f^1)$).

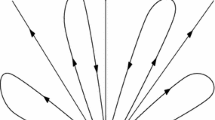
• 6. $\mathrm{ind}(f^k;B)=\left( \mathrm{Reg}_{1^0}^1(B)-\mathrm{Reg}_{1^0}^2(B)\right) +\mathrm{Reg}_{1^1}^1(B)$ for each orbit (here a class) $B\in {\mathcal {OR}}(f^k)$. The graph $\mathcal {GOR}(f)$ is smoothly realizable in ${\mathbb {R}}^2$. See Fig. 1.

Proof

We denote the elements of the group ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2=\{k^{0},k^{1}\}$. We put elements of ${\mathcal {R}}(f^1)$ ,${\mathcal {R}}(f^2)$,...vertically as columns. To avoid to draw too many arrows, we use the following convention. For two symbols $l^t$ , $k^t$ standing in the same horizontal line, and satisfying l|k, we assume implicitly that there is an (invisible) arrow from $l^t$ to $k^t$ unless another skew arrow (shown on the Picture) starts from $l^{t}$ an goes to a $k'^{t'}$ where $k'|k$. In other words, there is an arrow from $l^t$ to $k^s$ if one of the cases occures

• 1. there is an arrow in the Picture between these orbits

• 2. l|k and $t=s$ and no skew arrow starts at $l^t$

• 3. a concatenation of arrows defined above starts from $l^t$ and ends at $k^s$

Moreover we identify the compositions starting and ending at the same orbits.

Ad 1. ${\mathcal {R}}(f^k)={\mathbb {Z}}_2/(id-id)({\mathbb {Z}}_2) = {\mathbb {Z}}_2$ for all $k\in {\mathbb {N}}$.

Ad 2. $i_{kl}(x)=(x+f_{\#}^l(x)+f_{\#}^{2l}(x)+\cdots + f_{\#}^{k-l}(x))=(x+\cdots +x) =k/l\cdot x$. Now $\pi _1 M={\mathbb {Z}}_2$ implies the formula.

Ad 3. This follows from $f_{\#}=\mathrm{id}$.

Ad 4. Obvious

Ad 5. The classes in ${\mathcal {OR}}^b(B)$ for even b are inessential. On the other hand $i_{k1}$ is a bijection for odd k.

Ad 6. We check that the equality $\mathrm{ind}(f^k;B)=((\mathrm{Reg}_{1^0}^1-\mathrm{Reg}_{1^0}^2)+\mathrm{Reg}_{1^1}^1))(B)$ holds for $k=1$ and $k=2$ and we recall that the indices are the same for odd and the same for even multiplicities respectively. The summands $\mathrm{Reg}_{1^0}^1-\mathrm{Reg}_{1^0}^2$ and $\mathrm{Reg}_{1^1}^1$ are lifts of $\mathrm{reg}_1 - \mathrm{reg}_2$ and $\mathrm{reg}_1$, respectively, and the last expressions are smoothly realizable in ${\mathbb {R}}^2$ hence in the dimension less than $\mathrm{dim}M=3$. $\square$

6 Self-maps of $PSU(2)\times PSU(2)$

We pass to the case $M=PSU(2)\times PSU(2)$. Now $H^*(M;{\mathbb {Q}})=H^*(PSU(2)\times PSU(2);{\mathbb {Q}})=\Lambda _{{\mathbb {Q}}}(a_3,a'_3)$ and $\pi _1M=\pi _1(PSU(2)\times PSU(2))= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$. In the consequence, $A(M)=H^3(M;{\mathbb {Q}})={\mathbb {Q}}\oplus {\mathbb {Q}}$. A self-map $f:M\rightarrow M$ induces homomorphisms $f^*: H^3(M;{\mathbb {Q}}) \rightarrow H^3(M;{\mathbb {Q}})$ and $f_{\#}: \pi _1M\rightarrow \pi _1M$ given by matrices $A(f)\in {\mathcal {M}}_{2\times 2}({\mathbb {Z}})$ and $B(f)\in {\mathcal {M}}_{2\times 2}({\mathbb {Z}}_2)$ respectively.

First, we assume that $f_{\#}$ is not an isomorphism. If, moreover, $f_{\#}\equiv 0$ the Reidemeister graph reduces to natural numbers so the smooth realizability of $L(f^n)$ gives the result. Now we assume that $im(f_{\#})$ is 1-dimensional ${{\mathbb {Z}}_2}$-space. Then A(f) can be represented by the diagonal matrix $\mathrm{diag}(1,0)\in {\mathcal {M}}_{2\times 2}({\mathbb {Z}}_2)$ so $\mathcal {GOR}(f^*)$ reduces to the graph discussed in Sect. 5. Since the eigen-values of A(f) and $f_{\#}$ are congruent modulo 2, A(f) must have as eigen-values $-1$ and 0 (+1 is excluded). But now the Lefschetz numbers $L(f^k)$ coincide with those in Sect. 5 and the Reidemeister graph is realizable in dimension 2 as before.

From now on we assume that $f_{\#}$ is an isomorphism.

Lemma 6.1

There are six invertible matrices in ${\mathcal {M}}_{2 \times 2}({\mathbb {Z}}_2)$

$$\begin{aligned} \left( \begin{array}{cc} 1 &{} 0\\ 0 &{} 1\\ \end{array} \right) \;\; ;\;\; \left( \begin{array}{cc} 0 &{} 1 \\ 1 &{} 0 \\ \end{array} \right) , \left( \begin{array}{cc} 1 &{} 1 \\ 0&{} 1 \\ \end{array} \right) , \left( \begin{array}{cc} 1 &{} 0 \\ 1&{} 1 \\ \end{array} \right) \;\; ;\;\; \left( \begin{array}{cc} 0 &{} 1 \\ 1&{} 1 \\ \end{array} \right) , \left( \begin{array}{cc} 1 &{} 1 \\ 1&{} 0\\ \end{array} \right) \end{aligned}$$

Moreover, they split to three conjugacy classes (over ${\mathbb {Z}}_2$) as shown above.

Proof

The identity matrix is conjugate only to itself. We notice that $\left( \begin{array}{cc} 1 &{} 0 \\ 1&{} 1 \\ \end{array} \right) \left( \begin{array}{cc} 1 &{} 1 \\ 0&{} 1 \\ \end{array} \right) \left( \begin{array}{cc} 1 &{} 0 \\ 1&{} 1 \\ \end{array} \right) = \left( \begin{array}{cc} 0 &{} 1 \\ 1&{} 0 \\ \end{array} \right) = \left( \begin{array}{cc} 1 &{} 1 \\ 0&{} 1 \\ \end{array} \right) \left( \begin{array}{cc} 1 &{} 0 \\ 1&{} 1 \\ \end{array} \right) \left( \begin{array}{cc} 1 &{} 1 \\ 0&{} 1 \\ \end{array} \right)$. To get a conjugation between two last matrices, we use the matrix $\left( \begin{array}{cc} 0 &{} 1 \\ 1&{} 0 \\ \end{array} \right)$. Finally we notice that two last matrices enlisted above have nonzero trace $1\in {\mathbb {Z}}_2$ while the trace of each of the first 4 matrices equals $0\in {\mathbb {Z}}_2$; hence, there is no other conjugation. $\square$

Remark 6.2

Let us remark that exchanging the variables $(x,y)\in PSU(2)\times PSU(2)$ the matrix representing $f_{\#}$ becomes its transpose matrix. Thus each $f_{\#}$ can be represented by one of the matrices

$$\begin{aligned} B_1= \left( \begin{array}{cc} 1 &{} 0\\ 0 &{} 1\\ \end{array} \right) \;\; ;\;\; B_2= \left( \begin{array}{cc} 0 &{} 1 \\ 1 &{} 0 \\ \end{array} \right) \;\; \;\; \;\; ;\;\; B'_2= \left( \begin{array}{cc} 1 &{} 0 \\ 1 &{} 1 \\ \end{array} \right) \;\; ;\;\; B_3= \left( \begin{array}{cc} 0 &{} 1 \\ 1&{} 1 \\ \end{array} \right) \end{aligned}$$

Then $(B_i)^i=I$ for each $i=1,2,3$ and also $(B'_2)^2=I$ . Let us note that $B_2,B'_2$ are conjugate. $\square$

Lemma 6.3

Each matrix $A\in {\mathcal {M}}_{2\times 2}({\mathbb {Z}})$ whose all eigen-values are roots of unity different than $+1$ is conjugate (over real numbers) to one of

$$\begin{aligned} A_2= \left( \begin{array}{cc} -1 &{} \lambda \\ 0 &{} -1 \\ \end{array} \right) \text { where } \lambda \in {\mathbb {Z}} \;\; \text { or } \;\; A_k= \left( \begin{array}{cc} \cos (2\pi /k) &{} -\sin (2\pi /k) \\ \sin (2\pi /k) &{} \cos (2\pi /k) \\ \end{array} \right) \text { for } k=3,4,6 \end{aligned}$$

Proof

An integer nonsingular $2\times 2$ matrix whose eigen-values are only roots of unity has in its spectrum only roots of unity of degree 1, 2, 3, 4 or 6. Here we assume that $+1$ is not an eigen-value, hence the degree 1 is excluded. Now either $-1$ is the double eigen-value which gives $A_2$ or there is a unique complex eigen-value of degree 3,4 or 6 giving matrices $A_3,A_4$ or $A_6$ respectively. $\square$

We will denote $A_2= \left( \begin{array}{cc} -1 &{} \lambda \\ 0 &{} -1 \\ \end{array} \right)$ as above and moreover by $A'_2$, $A''_2$ we will mean the matrix $A_2$ with odd or even $\lambda$, respectively.

Let $f:M\rightarrow M$ be a self-map of $M=PSU(2)\times PSU(2)$ where A(f) has in its spectrum only roots of unity different from 1 and $f_{\#}$ is an isomorphism. Then by the above A(f) is given by a matrix conjugate (over ${\mathbb {R}}$) to $A_2,A_3,A_4$ or $A_6$, and $f_{\#}$ is represented by a matrix $B_1, B_2$, $B'_2$ or $B_3$. The next lemma makes precise which pairs of matrices may occur for a given f.

Lemma 6.4

Under the above assumptions, f gives a pair from $(A''_2,B_1)$ , $(A'_2,B'_2)$ , $(A_4,B_2)$ , $(A_3,B_3)$ , $(A_6,B_3)$.

Proof

Let us notice that only matrices $A_3$ and $A_6$ have odd trace, hence they must correspond to the matrix $B_3$. By Lemma 4.4: $A''_2$ determines $B_1$ , $A'_2$ determines $B'_2$ and $A_4$ determines $B_2$. $\square$

Now we will present all these cases. We will use the notation which we explain on examples. We consider $\pi _1 M={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ and a map $f:M\rightarrow M$.

If ${\mathcal {R}}(f^k)={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ then we write ${\mathcal {R}}(f^k)=\{k^{00},k^{01},k^{10},k^{11}\}$. If, for example, the action of $f_{\#}$ permutes $k^{01}$ with $k^{10}$ and keeps $k^{00}$ and $k^{11}$ fixed then we write ${\mathcal {OR}}(f^k)=\{k^{00};k^{01},k^{10};k^{11}\}$ i.e. semicolons separate the orbits.

If ${\mathcal {R}}(f^k)={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/({\mathbb {Z}}_2\oplus 0)=0\oplus {\mathbb {Z}}_2$ then we write ${\mathcal {R}}(f^k)=\{k^{*0},k^{*1}\}$ where $k^{*j}= \{k^{0j},k^{1,j}\}$.

If both Reidemeister classes belong to the same orbit, we write ${\mathcal {OR}}(f^k)=\{k^{*0},k^{*1}\}$ and if each of them is a single orbit then ${\mathcal {OR}}(f^k)=\{k^{*0};k^{*1}\}$.

If ${\mathcal {R}}(f^k)={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ and $f_{\#}$ permutes all nontrivial elements then we write ${\mathcal {OR}}(f^k)=\{k^{00};k^{nt}\}$ (here nt means all notrivial elements).

6.1 Case($A''_2$ , $B_1$)

Fig. 2

$\mathcal {GOR}$ of the map given by $(A''_2,B_1)$

The Reidemeister graph is given by Fig. 2. and its structure is explained by the next Lemma.

Lemma 6.5

If $A_2=\left( \begin{array}{cc} -1 &{} \lambda \\ 0&{} -1 \\ \end{array} \right)$ , $B_1=\left( \begin{array}{cc} 1 &{} 0 \\ 0&{} 1 \\ \end{array} \right)$ and $\lambda$ is even

• 1. ${\mathcal {R}}(f^k)={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$

• 2. $i_{kl}={\left\{ \begin{array}{ll} id &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$

• 3. Each orbit in ${\mathcal {OR}}(f^b)$ reduces to a single class.

• 4. $L(f^k)={\left\{ \begin{array}{ll} 4 &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$

  hence $L(f^k)=4\mathrm{reg}_1(k)-2\mathrm{reg}_2(k)$ is smoothly realizable in ${\mathbb {R}}^3$.

• 5. $\mathcal {IEOR}(f)= \{1^{00} , 1^{01} , 1^{10} , 1^{11}\}={\mathcal {OR}}(f^1)$.

• 6.
  $$\begin{aligned} \mathrm{ind}(f^b;B)=(\mathrm{Reg}_{1^{0,0}}^1-2\mathrm{Reg}_{1^{0,0}}^2)(B) + \mathrm{Reg}_{1^{1,0}}^1(B)+ \mathrm{Reg}_{1^{0,1}}^1(B)+ \mathrm{Reg}_{1^{1,1}}^1(B) \end{aligned}$$

  so the graph is smoothly realizable in dimension 2. See Fig. 2.

Proof

• Ad 1 Since $f_{\#}^k=B_1^k=id$ , ${\mathcal {R}}(f^k)= ({\mathbb {Z}}_2\oplus {\mathbb {Z}}_2)/(im(id-id))={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ for each k.

• Ad 2 $i_{kl}=I+B_1^{l}+B_1^{2l}+\cdots + +B_1^{k-l}= I+I+\cdots +I=k/l\cdot I$.

  Now $i_{kl}=id$ for k/l odd and 0 for k/l even.

• Ad 3 This follows from $f_{\#}=id$.

• Ad 4
  $$\begin{aligned} L(f^k)= \mathrm{det}(I-A_2^k)= \mathrm{det}\left( \begin{array}{cc} 1- (-1)^k &{} 0 \\ 0 &{} 1- (-1)^k \\ \end{array} \right) = {\left\{ \begin{array}{ll} 4 &{} \text {if} \;\; k \text { is odd}, \\ 0 &{} \text {if} \;\; k \text { is even}, \\ \end{array}\right. } \end{aligned}$$

• Ad 5 The classes in ${\mathcal {OR}}^b(B)$ for even b are inessential. On the other hand, $i_{b1}$ is a bijection for odd b.

• Ad 6 It is enough to check that the equality holds for $b=1,2$. Moreover we notice that each of the four summands, each attached at a different orbit, is smoothly realizable in dimension 2.

$\square$

6.2 Case $(A'_2,B'_2)$

Fig. 3

$\mathcal {GOR}$ of a map given by $(A'_2,B'_2)$

The Reidemeister graph is given by Fig. 3. and its structure is explained by the next Lemma. Here we drop the arrows starting at $k^{**}$, for k even, since all such orbits are inessential.

Lemma 6.6

If $\lambda$ is odd and $A'_2=\left( \begin{array}{cc} -1 &{} \lambda \\ 0&{} -1 \\ \end{array} \right)$ , $B'_2=\left( \begin{array}{cc} 1 &{} 0 \\ 1 &{} 1 \\ \end{array} \right)$ then:

• 1. ${\mathcal {R}}(f^k)={\left\{ \begin{array}{ll} {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2 &{} \text {if} \;\; k \text { is even}, \\ {\mathbb {Z}}_2 \oplus 0&{} \text {if} \;\; k \text { is odd}, \\ \end{array}\right. }$

• 2. $i_{kl}={\left\{ \begin{array}{ll} \text {isomorphism} &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$ as the map $i_{kl}:{\mathcal {R}}(f^l)\rightarrow {\mathcal {R}}(f^k)$

  More precisely $i_{kl}:\pi _1 M \rightarrow \pi _1 M$ is given by:

  If l is even then $i_{kl}={\left\{ \begin{array}{ll} {id} &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$

  If l is odd then $i_{kl}={\left\{ \begin{array}{ll} \text {id} &{} \text {if} \;\; k/l\equiv 1 \text { modulo } 4, \\ \left( \begin{array}{cc} 0 &{} 0 \\ 1 &{} 0 \\ \end{array} \right) &{} \text {if} \;\; k/l\equiv 2 \text { modulo } 4, \\ \left( \begin{array}{cc} 1 &{} 0 \\ 1 &{} 1 \\ \end{array} \right) &{} \text {if} \;\; k/l\equiv 3 \text { modulo } 4, \\ 0 &{} \text {if} \;\; k/l \equiv 0 \text { modulo } 4, \\ \end{array}\right. }$

• 3. Each orbit contains a single Reidemeister class.

• 4. $L(f^k)={\left\{ \begin{array}{ll} 4 &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even}, \\ \end{array}\right. }$

  hence $L(f^k)=4\mathrm{reg}_1(k)-2\mathrm{reg}_2(k)$

• 5. $\mathcal {IEOR}={\mathcal {OR}}(f^1)=\{ 1^{0*} ; 1^{1*} \}$.

• 6.
  $$\begin{aligned} \mathrm{ind}(f^b;B)=(\mathrm{Reg}_{1^{0*}}^1+2\mathrm{Reg}_{1^{1*}}^1)(B)-2\mathrm{Reg}_{2^{00}}^1(B) \end{aligned}$$

  for each $B\in {\mathcal {OR}}(f^b)$, $b\in {\mathbb {N}}$. $\mathcal {GOR}(f)$ is smoothly realizable in dimension 2.

Proof

• Ad 1. We notice that $(B'_2)^k = {\left\{ \begin{array}{ll} B'_2 &{} \text {if} \;\; k \text { is odd} \\ I &{} \text {if} \;\; k \text { is even}\\ \end{array}\right. }$.

  Now, for odd k, ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/\mathrm{im}(I-B'_2)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/\mathrm{im}\left( \begin{array}{cc} 0 &{} 0 \\ 1 &{} 0 \\ \end{array} \right) = {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/0\oplus {\mathbb {Z}}_2 = {\mathbb {Z}}_2 \oplus 0$.

  On the other hand ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ for even k, since then $(B'_2)^k=id$.

• Ad 2 It is obvious for l even, since then $i_{kl}=I+B_2'^l+B_2'^{2l}+\cdots + B_2'^{k-l}=I+I+\cdots +I=k/l\cdot I$.

  For l odd: $i_{ll}=I$ , $i_{2l,l}=I+B_2^l=I+B'_2$ , $i_{3l,l}=I+B_2'^l+B_2'^{2l}=I+B'_2+I=B'_2$ , $i_{4l,l}=0$ and then cyclically.

  We notice that $i_{2l,l}(1,*)=(I+B'_2)(1,*)= \left( \begin{array}{cc} 0 &{} 0 \\ 1 &{} 0 \\ \end{array} \right) \left( \begin{array}{c} 1 \\ * \\ \end{array} \right) = \left( \begin{array}{c} 0 \\ *\\ \end{array} \right) = \left( \begin{array}{c} 0 \\ 0 \end{array} \right) \in {\mathbb {Z}}_2 \oplus 0$

  Now $i_{ll}=I$ , $i_{3l,l}=B'_2$ is an isomorphism and $i_{2l,l}$ , $i_{4l,l}$ are zero maps of ${\mathcal {R}}(f^{*l})$.

• Ad 3 If k is odd, then the action of ${\mathbb {Z}}_k$ induced by $f_{\#}$ on $\pi _1(M\times M)={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ is trivial.

  If k is even then $(B'_2)^k=id$.

• Ad 4 As in the previous subsection, since $\lambda$ gives no contribution to the determinant.

• Ad 5 For even b, all classes in ${\mathcal {OR}}^b(B)$ are inessential. On the other hand, $i_{b1}$ is a bijection for odd b.

• Ad 6 We check the equality for $B\in {\mathcal {OR}}(b^b))$ , $b=1,2$. The equality for other b follows from the above remark that for even b all classes in ${\mathcal {OR}}^b(B)$ are inessential and $i_{b1}$ is a bijection for odd b. $\square$

6.3 Case $A_4$ , $B_2$

Fig. 4

$\mathcal {GOR}$ of a self-map given by $(A_3,B_3)$

We consider matrices $A_4=\left( \begin{array}{cc} 0&{} -1 \\ 1 &{} 0 \\ \end{array}\right)$ and $B_2= \left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 0 \\ \end{array}\right)$. We will show that then

Lemma 6.7

Under the above assumptions

• 1. ${\mathcal {R}}(f^k)={\left\{ \begin{array}{ll} {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2 &{} \text {if} \;\; k/l \text { is even}, \\ {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/\Delta &{} \text {if} \;\; k/l \text { is odd}, \\ \end{array}\right. }$

  here $\Delta =\{(x,x);x\in {\mathbb {Z}}_2 \}$.

• 2. $i_{kl}=k/l\cdot I$ if l is even. If l is odd $i_{l,l}=\left( \begin{array}{cc} 1&{} 0 \\ 0 &{} 1 \\ \end{array}\right)$ $i_{2l,l}=\left( \begin{array}{cc} 1&{} 1 \\ 1 &{} 1 \\ \end{array}\right)$ , $i_{3l,l}= \left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 0 \\ \end{array}\right)$, $i_{4l,l}=\left( \begin{array}{cc} 0&{} 0 \\ 0 &{} 0 \\ \end{array}\right)$ and then cyclically.

• 3. For odd k , ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/ \Delta$ so we have two Reidemeister classes $\Delta =\{(0,0) , (1,1)\}$ and $\Delta '=\{(1,0) , (0,1)\}$.

  For even k , ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ contains two orbits each consisting of a single element $k^{00}$, $k^{11}$ , and one 2-orbit $\Delta '=\{k^{01}, k^{10}\}$. Each orbit contains a single class.

• 4. $L(f^1)=2$ , $L(f^2)=4$ , $L(f^3)=2$ , $L(f^4)=0$ , and then cyclically.

  Now $L(f^k)=2\mathrm{reg}_1(k)+\mathrm{reg}_2(k)-\mathrm{reg}_4(k)$ is smoothly realizable in ${\mathbb {R}}^4$.

• 5. $\mathcal {IEOR}(f)=\{1^{\Delta }; 1^{\Delta '};2^{\Delta '}\}$.

• 6. $\mathrm{ind}(f^b:B)=(\mathrm{Reg}^1_{1^{\Delta }}- \mathrm{Reg}^4_{1^{\Delta }})(B) + \mathrm{Reg}^1_{1^{\Delta '}}(B)+ \mathrm{Reg}^1_{2^{\Delta '}}(B)$ for each $B\in {\mathcal {OR}}(f^b)$, $b\in {\mathbb {N}}$. $\mathcal {GOR}(f)$ is smoothly realizable in dimension 2. See Fig. 4.

Proof

• Ad 1 We consider the matrix $B_2=\left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 0 \\ \end{array} \right)$. Then $B_2^k=I$ for k even and $B_2^k=B_2$ for k odd. Now ${\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ for k even and

  $$\begin{aligned} {\mathcal {R}}(f^k)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/ im(I-B_2)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/ im \left( \begin{array}{cc} 1&{} 1 \\ 1 &{} 1 \\ \end{array}\right) = {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/ \Delta =\{\Delta ,\Delta '\} \end{aligned}$$

  for k odd.

• Ad 2 If l is even then $i_{kl}=I+B_2^l+B_2^{2l}+\cdots +B_2^{k-l}=I+\cdots +I= k/l\cdot I$. On the other hand for odd l

  $$\begin{aligned} i_{21}=I+B_2=\left( \begin{array}{cc} 1&{} 1 \\ 1 &{} 1 \\ \end{array}\right) \end{aligned}$$
  $$\begin{aligned} i_{31}=I+B_2+B_2^2= I+B_2+I=B_2= \left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 0 \\ \end{array}\right) \end{aligned}$$
  $$\begin{aligned} i_{41}=I+B_2+B_2^2+B_2^3= I+B_2+I+B_2= \left( \begin{array}{cc} 0&{} 0 \\ 0 &{} 0 \\ \end{array}\right) \end{aligned}$$

  The above can be rewritten to get cyclic formulae (for each odd l):

  $i_{2l,l}=\left( \begin{array}{cc} 1&{} 1 \\ 1 &{} 1 \\ \end{array}\right)$ , $i_{3l,l}= \left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 0 \\ \end{array}\right)$, $i_{4l,l}=\left( \begin{array}{cc} 0&{} 0 \\ 0 &{} 0 \\ \end{array}\right)$,....

• Ad 3 If k is odd then we have two Reidemeister classes $\Delta$ and $\Delta '$. Moreover, the action of $B_2$ preserves these classes, hence each class is an orbit. On the other hand for k even we have four Reidemeister classes and $B_2$ permutes elements (1, 0) and (0, 1) which gives one 2-orbit $\Delta =\{k^{01}, k^{10}\}$ but two other orbits consist each of a single element $\{k^{00}\}$ and $\{k^{11}\}$.

• Ad 4
  $$\begin{aligned} L(f^1)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 0&{} -1 \\ 1 &{} 0 \\ \end{array} \right) \right) =\mathrm{det} \left( \begin{array}{cc} 1&{} 1 \\ -1 &{} 1 \\ \end{array} \right) =2 \end{aligned}$$
  $$\begin{aligned} L(f^2)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} -1&{} 0 \\ 0 &{} -1 \\ \end{array} \right) \right) =\mathrm{det} \left( \begin{array}{cc} 2&{} 0 \\ 0 &{} 2 \\ \end{array} \right) =4 \end{aligned}$$
  $$\begin{aligned} L(f^3)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 0&{} 1 \\ -1 &{} 0 \\ \end{array} \right) \right) =\mathrm{det} \left( \begin{array}{cc} 1&{} -1 \\ 1 &{} 1 \\ \end{array} \right) =2 \end{aligned}$$
  $$\begin{aligned} L(f^4)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 1&{} 0 \\ 0 &{} 1 \\ \end{array} \right) \right) =\mathrm{det} \left( \begin{array}{cc} 0&{} 0 \\ 0 &{} 0 \\ \end{array} \right) =0 \end{aligned}$$

  and then we notice that $A^4=I$ implies $A^{4k+l}=A^l$.

  This implies $L(f^k)=2\mathrm{reg}_1(k)+\mathrm{\mathrm{reg}}_2(k)-\mathrm{\mathrm{reg}}_4(k)= (\mathrm{reg}_1(k)+\mathrm{\mathrm{reg}}_2(k)) \;\; +\;\; (\mathrm{reg}_1(k)-\mathrm{\mathrm{reg}}_4(k))$.

• Ad 5 If k/l is odd then $i_{kl}$ is the bijection. Now each orbit $B\in {\mathcal {OR}}(f^b)$, where 4 does not divide b, reduces to a class in ${\mathcal {OR}}(f^1)$ or ${\mathcal {OR}}(f^2)$. If 4 divides k then $L(f^b)=0$ so B is inessential.

• Ad 6 We check that the equality holds for $i=1,2,3,4$. If b is odd then $i_{1b}$ is a bijection and $L(f^b)=L(f^1)=2$ . If b is even but 4 does not divide b then then $i_{2b}$ is a bijection and $L(f^b)=L(f^2)=4$ . If 4 divides b then all classes are inessential, $b^{11}$ is irreducible and $\mathrm{Reg}(b^{00})=0$. $\square$

6.4 Case $A_3$ , $B_3$

Fig. 5

$\mathcal {GOR}$ of a self map given by $(A'_2,B'_2)$

Now we consider the case $(A_3 , B_3)$. The Reidemeister graph turns out to be simpler than the previous ones. We will see that the essential part reduces to the graph of natural numbers and then the smooth realizability is implied by the sequence $L(f^n)$. From the Lemma given below it follows that ${\mathcal {OR}}(f^k)$ reduces to a point if k does not divide 3 (see Figure 5) and $L(f^k)=0$ when 3 divides k. Now each essential class reduces to the unique element in ${\mathcal {OR}}(f^1)$. Then the Dold decomposition of $L(f^k)$ attached at ${\mathcal {OR}}(f^1)$ gives the distribution of the index function $\mathrm{ind}(f^b;B)$ and proves the smooth realizability of the graph.

Lemma 6.8

• 1. ${\mathcal {R}}(f^k)={\left\{ \begin{array}{ll} {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2&{} \text {if} \;\; 3 \text { divides } k, \\ 0 &{} \text { otherwise }\\ \end{array}\right. }$

• 2. $i_{3k,3l}= {\left\{ \begin{array}{ll} I &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even} \\ \end{array}\right. }$.

  If 3 does not divide l then the homomorphism $i_{kl}$ is trivial.

• 3. ${\mathcal {R}}(f^{3k})= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ and nontrivial classes form a 3-orbit.

• 4. $L(f^k) = {\left\{ \begin{array}{ll} 0 &{} \text {if} \;\; 3 \text { divides } k \\ 3 &{} \text {if} \;\; 3 \text { otherwise } \\ \end{array}\right. }$

  hence $L(f^k)=3\mathrm{reg}_1(k)-\mathrm{reg}_3(k)$.

• 5. $\mathcal {IEOR}(f)=\{1^{**}\}={\mathcal {OR}}(f^1)$

• 6. $\mathrm{ind}(f^b;B)=(3\mathrm{Reg}_{1^{**}}^1 - \mathrm{Reg}_{1^{**}}^3)(B)$ . The Reidemeister graph is smoothly realizable in dimension 4.

Proof

• Ad 1 . We consider the matrix $B_3= \left( \begin{array}{cc} 0&{} 1 \\ 1 &{} 1\\ \end{array}\right)$. Then $B_3^2= \left( \begin{array}{cc} 1&{} 1 \\ 1 &{} 0\\ \end{array}\right)$ , $B_3^3= \left( \begin{array}{cc} 1&{} 0 \\ 0 &{} 1\\ \end{array}\right)$. This implies

  $$\begin{aligned} {\mathcal {R}}(f)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/im(I-B_3)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/im \left( \begin{array}{cc} 1&{} 1\\ 1&{}0 \end{array}\right) =0 \end{aligned}$$
  $$\begin{aligned} {\mathcal {R}}(f^2)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/im(I-B_3^2)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2/im \left( \begin{array}{cc} 0&{} 1\\ 1&{}1 \end{array}\right) =0 \end{aligned}$$
  $$\begin{aligned} {\mathcal {R}}(f^3)= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2 \end{aligned}$$

  and then cyclically.

• Ad 2 . $i_{3k,3l}=I+B_3^{3l}+ B_3^{3l}+ \cdots +B_3^{3(k-l)}=I+\cdots +I=k/l\cdot I$, hence $i_{3k,3l}= {\left\{ \begin{array}{ll} I &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even} \\ \end{array}\right. }$.

  If 3 does not divide l then ${\mathcal {R}}(f^l)=0$

• Ad 3 . $B_3$ permutes all three nonzero elements in ${\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ so ${\mathcal {R}}(f^{3k})$ contains one 1-orbit $(3k)^{00}$ and one 3-orbit $(3k)^{nt}$ (here nt set of all nontrivial elements in ${\mathcal {R}}(f^{3k})={\mathbb {Z}}_2\oplus {\mathbb {Z}}_2)$.

• Ad 4 . To find the Lefschetz numbers we choose a matrix $A= \left( \begin{array}{cc} -1&{} 1 \\ -1 &{} 0\\ \end{array}\right)$ which is conjugate to $A_3$, since the two matrices have the same trace and determinant. Now

  $$\begin{aligned} L(f^1)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} -1&{} 1 \\ -1 &{} 0 \\ \end{array} \right) \right) =\mathrm{det} \left( \begin{array}{cc} 2&{} -1 \\ 1 &{} 1 \\ \end{array} \right) =3 \end{aligned}$$
  $$\begin{aligned} L(f^2)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} -1&{} 1 \\ -1 &{} 0 \\ \end{array} \right) ^2\right) = \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 0&{} -1 \\ 1 &{} -1 \\ \end{array} \right) \right) = \mathrm{det} \left( \begin{array}{cc} 1&{} 1 \\ -1&{} 2 \\ \end{array} \right) =3 \end{aligned}$$
  $$\begin{aligned} L(f^3)= \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 1&{} 1 \\ -1 &{} 0 \\ \end{array} \right) ^3\right) = \mathrm{det}\left( \left( \begin{array}{cc} 1&{} 0 \\ 0&{} 1 \\ \end{array} \right) - \left( \begin{array}{cc} 1&{} 0 \\ 0 &{} 1 \\ \end{array} \right) \right) = 0 \end{aligned}$$

  and then cyclically. Now $L(f^k)=3\mathrm{reg}_1(k)-\mathrm{reg}_3(k)$

• Ad 5 . All essential classes are on the level 0, hence they reduce to the class represented by $1^{00}$.

• Ad 6 . $\mathrm{ind}(f^b:B)=(3\mathrm{Reg}^1_{1^{**}}- \mathrm{Reg}^3_{1^{**}})(B)$ and this expression is smoothly realizable in dimension 4.

$\square$

6.5 Case $A_6,B_3$

Lemma 6.9

Under the above assumptions

• 1. ${\mathcal {R}}(f^k)={\left\{ \begin{array}{ll} {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2&{} \text {if} \;\; k/l \text { divides } 3, \\ 0 &{} \text {if} \;\; k/l \text { otherwise }\\ \end{array}\right. }$

• 2. $i_{3k,3l}= {\left\{ \begin{array}{ll} I &{} \text {if} \;\; k/l \text { is odd}, \\ 0 &{} \text {if} \;\; k/l \text { is even} \\ \end{array}\right. }$.

  If 3 does not divide l then the homomorphism $i_{kl}$ is trivial.

• 3. ${\mathcal {R}}(f^{3k})= {\mathbb {Z}}_2\oplus {\mathbb {Z}}_2$ and nontrivial classes form a 3-orbit.

• 4. $L(f^1)=1$ , $L(f^2)=3$ , $L(f^3)=4$ , $L(f^4)=3$ ,$L(f^5)=1$, $L(f^6)=0$ and the cyclically.

  Now $L(f^k)=(\mathrm{reg}_1+\mathrm{reg}_2 +\mathrm{reg}_3-\mathrm{reg}_6)(k)$

• 5. $\mathcal {IEOR}(f)=\{1^{**} \}$

• 6.
  $$\begin{aligned} \mathrm{ind}(f^b:B)=((\mathrm{Reg}^1_{1^{**}}+\mathrm{Reg}^2_{1^{**}})+(\mathrm{Reg}^1_{3^{nt}}-\mathrm{Reg}^2_{3^{nt}})(B) \end{aligned}$$

  for each $B\in {\mathcal {OR}}(f^b)$, $b\in {\mathbb {N}}$. $\mathcal {GOR}(f)$ is smoothly realizable in dimension 2.

Proof

Let us remark that here we use the same matrix $B_3$ as in the previous case. Thus the vertices and arrows in the Reidemeister graphs coincide with those in Figure 5. and the proof of 1. ,2. , 3. reduces to that of Lemma 6.8.

• Ad 4 We consider the matrix $A_6= \left( \begin{array}{cc} 1&{} -1 \\ 1 &{} 0 \\ \end{array} \right)$ satisfying $A^6=I$ and $A^k\ne I$ for $k\le 5$.

  In fact $A_6^2= \left( \begin{array}{cc} 0&{} -1 \\ 1 &{} -1 \\ \end{array} \right)$ , $A_6^3= \left( \begin{array}{cc} -1&{} 0 \\ 0 &{} -1 \\ \end{array} \right)$, $A_6^4=\left( \begin{array}{cc} -1&{} 1 \\ -1 &{} 0 \\ \end{array} \right)$ , $A_6^5= \left( \begin{array}{cc} 0&{} 1 \\ -1 &{} 1 \\ \end{array} \right)$ , $A_6^6=I$

  In the consequence

  $$\begin{aligned} L(f)=\mathrm{det}(I-A)=\mathrm{det} \left( \begin{array}{cc} 0&{} 1 \\ -1 &{} 1 \\ \end{array} \right) = 1 \end{aligned}$$
  $$\begin{aligned} L(f^2)=\mathrm{det}(I-A^2)=\mathrm{det} \left( \begin{array}{cc} 1&{} 1 \\ -1 &{} 2 \\ \end{array} \right) =3 \end{aligned}$$
  $$\begin{aligned} L(f^3)=\mathrm{det}(I-A^3)=\mathrm{det} \left( \begin{array}{cc} 2&{} 0 \\ 0 &{} 2\\ \end{array} \right) =4 \end{aligned}$$
  $$\begin{aligned} L(f^4)=\mathrm{det}(I-A^4)=\mathrm{det} \left( \begin{array}{cc} 2&{} -1 \\ 1 &{} 1 \\ \end{array} \right) =3 \end{aligned}$$
  $$\begin{aligned} L(f^5)=\mathrm{det}(I-A)=\mathrm{det} \left( \begin{array}{cc} 1&{} -1 \\ 1 &{} 0 \\ \end{array} \right) =1 \end{aligned}$$
  $$\begin{aligned} L(f^6)=\mathrm{det}(I-A^6)=\mathrm{det} \left( \begin{array}{cc} 0&{} 0 \\ 0 &{} 0 \\ \end{array} \right) =0 \end{aligned}$$

  This implies $L(f^k)= (\mathrm{reg}_1+\mathrm{reg}_2+\mathrm{reg}_3-\mathrm{reg}_6)(k)$.

• Ad 5 . All elements represented by $k^{00}$ reduce to $1^{00}$. On the other hand elements in ${\mathcal {OR}}(f^{3k})$ are inessential.

• Ad 6 We check that both sides of the equality agree if $b=1,\ldots ,6$. $\square$

Remark 6.10

All cases discussed above can occur. Let us fix a matrix $A=[a_{ij}]\in {\mathcal {M}}_{2\times 2}({\mathbb {Z}})$. For each $i,j\in 1,2$ we fix a map $f_{ij}:PSU(2)\rightarrow PSU(2)$ of degree $a_{ij}$ and we define a self-map of $PSU(2)\times PSU(2)$ by $f(x,y)=(f_{11}(x)*f_{12}(y), f_{21}(x)*f_{22}(y))$ where $*$ denotes the multiplication in the group PSU(2). Then the cohomology homomorphism $f_3^*$, of $H^3(PSU(2)\times PSU(2))=A(PSU(2)\times PSU(2)) = {\mathbb {Q}} \oplus {\mathbb {Q}}$, is given by the matrix

$$\begin{aligned} A(f)= \left( \begin{array}{cc} \mathrm{deg} f_{11}&{} \mathrm{deg} f_{12} \\ \mathrm{deg} f_{21} &{} \mathrm{deg} f_{22} \\ \end{array} \right) \end{aligned}$$

which coincides with the given A.