Invasibility of Nectarless Flowers in Plant–Pollinator Systems

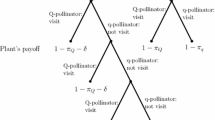
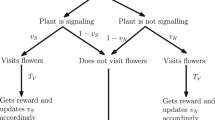
Abstract

This paper considers plant–pollinator systems in which plants are divided into two categories: The plants that secret a substantial volume of nectar in their flowers are called secretors, while those without secreting nectar are called nonsecretors (cheaters). The interaction between pollinators and secretors is mutualistic, while the interaction between pollinators and nonsecretors is parasitic. Both interactions can be described by Beddington–DeAngelis functional responses. Using dynamical systems theory, we show global dynamics of a pollinator–secretor–cheater model and demonstrate mechanisms by which nectarless flowers/nonsecretors can invade the pollinator–secretor system and by which the three species could coexist. We define a threshold in the nonsecretors’ efficiency in translating pollinator–cheater interaction into fitness, which is determined by parameters (factors) in the systems. When their efficiency is above the threshold, non-secretors can invade the pollinator–secretor system. While the nonsecretors’ invasion often leads to their persistence in pollinator–secretor systems, the model demonstrates situations in which the non-secretors’ invasion can drive secretors into extinction, which consequently leads to extinction of the nonsecretors themselves.

Appendices

Appendix A: Proof of Theorem 4.3

Since $\mu_{v}^{K} >0$, P _uv is globally asymptotically stable in the interior of the (u,v)-plane by Proposition 3.1(i). Since $\mu_{w}^{K} <0$, P _K is globally asymptotically stable in the interior of the (u,w)-plane by Proposition 3.2(i).

(i):

Since $\mu_{w}^{+}>0$, the boundary equilibria P ₀,P ₁ and P _uv are hyperbolic saddle points and cannot form a heteroclinic cycle. Thus hypotheses of (H-1) to (H-4) derived by Butler et al. (1986) are satisfied. As a result of Butler et al. (1986), system (3) is uniformly persistent.

(ii):

From $\mu_{w}^{+}<0$, we have −D+Eu ⁺/(1+Bu ⁺)<0. Since the function u/(1+Bu) is monotonically increasing, there are constants ξ>0,η>0 such that when 0≤u<ξ+u ⁺, we have

$$-D + \frac{Eu}{1+Bu} < -\eta < 0. $$

Let (u(t),v(t),w(t)) be a solution of (3) with u(0)>0,v(0)>0,w(0)>0. Let $(\bar{u}(t),\bar{v}(t))$ be a solution of (1) with $\bar{u}(0)=u(0), \bar{v}(0)=v(0)$. By (3), the solution (u(t),v(t),w(t)) satisfies

From the comparison theorem (Cosner 1996), we obtain $u(t)\le \bar{u}(t)$ as t>0.

Since P _uv is globally asymptotically stable in the interior of the (u,v)-plane, there is T>0 such that when t>T, $\bar{u}(t) <\xi+u^{+}$. Then u(t)<ξ+u ⁺ as t>T. Hence, dw/dt<−ηw as t>T. By the Lyapunov theorem, we have lim _t→+∞ w(t)=0. Then systems (1) and (3) have the same asymptotic dynamics as a result of Thieme (1992). Thus, the result in (ii) is proved.

Appendix B: Proof of Theorems 4.4–4.5

Proof of Theorem 4.4

Since $\mu_{v}^{K} >0$, P _uv is globally asymptotically stable in the interior of the (u,v)-plane by Proposition 3.1(i). From $\mu_{w}^{K} >0$ and u ⁺>K, we have $\mu_{w}^{+}>\mu_{w}^{K} >0$ by the monotonicity of function u/(1+Bu). Since $\mu_{w}^{K} >0$ and $\operatorname{tr}J(E_{uw})<0$, P _uw is globally asymptotically stable in the interior of the (u,w)-plane by Proposition 3.2(ii).

(i):

By $\mu_{v}^{\#}>0$ and $\mu_{w}^{+}>0$, the boundary equilibria P ₀,P ₁,P _uv and P _uw are hyperbolic saddle points and cannot form a heteroclinic cycle. Thus, hypotheses of (H-1) to (H-4) derived by Butler et al. (1986) are satisfied. As a result of Butler et al. (1986), system (3) is uniformly persistent.

(ii):

Since $\mu_{v}^{\#}<0$ and a<a ₀, there is no interior equilibrium of (3) by Lemma 4.1. Since $\mu_{w}^{K}>0$ and $\operatorname{tr}J(E_{uw})<0$, P _uw is globally asymptotically stable in the interior of the (u,w)-plane and is locally asymptotically stable in $R_{+}^{3}$. Let Ω ^# be the basin of attraction of P _uw in $R_{+}^{3}$. Then Ω ^# is open and forward invariant and $R_{+}^{3} - {\varOmega}^{\#}$ is closed and forward invariant in $R_{+}^{3}$. Suppose the interior of $R_{+}^{3} - {\varOmega}^{\#}$ is not empty, then orbits of (3) in $\operatorname{int}(R_{+}^{3} - {\varOmega}^{\#})$ will not converge to P _uw since they are not in the basin of attraction of P _uw . Let (u(t),v(t),w(t)) be a solution of (3) with $(u(0), v(0), w(0))\in \operatorname{int}(R_{+}^{3} - {\varOmega}^{\#})$, then we have lim sup _t→∞ u(t)>0,lim sup _t→∞ v(t)>0,lim sup _t→∞ w(t)>0. Indeed, suppose lim _t→∞ v(t)=0, then the ω-limit set of the orbit lies on the (u,w)-plane. On the (u,w)-plane, P _uw is globally asymptotically stable while $\tilde{O}$ and $\tilde{E}_{1}$ are hyperbolic saddle points. We apply a result of Thieme (1992) and conclude that this orbit converges to P _uw , which is a contradiction. Similar discussions could show that lim sup _t→∞ u(t)>0,lim sup _t→∞ w(t)>0. Hence system (3) is weakly persistent on $R_{+}^{3} - {\varOmega}^{\#}$. Since the boundary equilibria O,P ₁,P _uv and P _uw are hyperbolic saddle points and can not form a heteroclinic cycle, hypotheses of (H-1) to (H-4) derived by Butler et al. (1986) are satisfied on $R_{+}^{3}-{\varOmega}^{\#}$. Thus, system (3) restricted on $R_{+}^{3}-{\varOmega}^{\#}$ is uniformly persistent and has an interior equilibrium P ^∗ as a result of Butler et al. (1986). This forms a contradiction since there is no interior equilibrium. Thus, $\operatorname{int}(R_{+}^{3}-{\varOmega}^{\#})$ is empty and the result (ii) is proved.

(iii):

Since $\mu_{v}^{\#}<0$ and a>a ₀, there are two interior equilibrium of (3) by Lemma 4.1. Thus, P _uw cannot be globally asymptotically stable in int$R_{+}^{3}$. Let Ω ^# be the basin of attraction of P _uw in $R_{+}^{3}$. Similar to the proof of Theorem 4.4(ii), system (3) restricted on $R_{+}^{3}-{\varOmega}^{\#}$ is uniformly persistent.  □

Proof of Theorem 4.5

Since $\mu_{v}^{K} >0$, P _uv is globally asymptotically stable in the interior of the (u,v)-plane by Proposition 3.1(i). From $\mu_{w}^{K} >0$ and u ⁺>K, we have $\mu_{w}^{+}>\mu_{w}^{K} >0$ by the monotonicity of function u/(1+Bu). Since $\mu_{w}^{K} >0$ and trJ(E _uw )>0, P _ϕ is asymptotically stable in the interior of the (u,w)-plane by Proposition 3.2(iii).

(i):

Since $\mu_{v}^{\#}>0$, P _uw is unstable in the v-axis direction. Since $\mu_{v}^{\phi}>0$, P _ϕ is unstable in the v-axis direction. Thus, the boundary periodic orbit and equilibria are hyperbolic and cannot form a heteroclinic cycle. Similar to the proof of Theorem 4.4(i), system (3) is uniformly persistent.

(ii):

By $\mu_{v}^{\#}<0$ and $\mu_{v}^{\phi}<0$, the set P _uw ∪P _ϕ is locally asymptotically stable in $R_{+}^{3}$ with a basin of attraction Ω _ϕ . Since a<a ₀, there is no interior equilibrium of (3) by Lemma 4.1. Similar to the proof of Theorem 4.4(ii), we conclude that $\operatorname{int}(R_{+}^{3} - {\varOmega}_{\phi})$ is empty. Thus the result (ii) is proved.

(iii):

Since $\mu_{v}^{\#}<0$ and a>a ₀, there are two interior equilibrium of (3) by Lemma 4.1. Thus P _uw ∪P _ϕ cannot be globally asymptotically stable in $\operatorname{int}R_{+}^{3}$. Let Ω _ϕ be the basin of attraction of P _uw ∪P _ϕ in $R_{+}^{3}$. Similar to the proof of Theorem 4.4(ii), system (3) restricted on $R_{+}^{3}-{\varOmega}_{\phi}$ is uniformly persistent.

(iv):

If $\mu_{v}^{\#} >0$ and $\mu_{v}^{\phi}<0$, then P _ϕ is locally asymptotically stable in $R_{+}^{3}$. If $\mu_{v}^{\#} <0$ and $\mu_{v}^{\phi}>0$, then P _uw is asymptotically stable in the v-axis direction, which implies that there is a solution of (3) that converges to P _uw . Thus system (3) is not persistent.  □

Appendix C: Proof of Theorem 4.6

Since $\mu_{v}^{K}<0$, P _K is locally asymptotically stable in the interior of the (u,v)-plane. By Proposition 3.1, there are at most two interior equilibria of system (1). Let (u(t),v(t),w(t)) be a solution of (1) with u(0)>0,v(0)>0,w(0)>0.

(i):

Since $\bar{B}>0$ or $\bar{{\varDelta}}<0$, there is no interior equilibrium of (1) by Proposition 3.1(iii). Then P _K is globally asymptotically stable in the interior of the (u,v)-plane. Thus for the solution $(\bar{u}(t),\bar{v}(t))$ of (1) with $\bar{u}(0) = u(0), \bar{v}(0) = v(0)$, we obtain $\lim_{t \to \infty} \bar{v}(t)=0$. Similar to the proof of Theorem 4.3(ii), we have $v(t) \le \bar{v}(t)$. Thus, lim _t→+∞ v(t)=0.

(ii)–(iii):

Since $\bar{B}<0, \bar{{\varDelta}}>0$, there are two interior equilibria of system (1) by Proposition 3.1(ii). The separatrice of equilibrium $E_{uv}^{-}(u^{-},v^{-})$ divide the (u,v)-plane into two regions: one is the basin of attraction of E _K , which is denoted by Π _K ; the other is that of E _uv , which is denoted by Π _uv . Then for any solution $(\bar{u}(t),\bar{v}(t))$ of (1) with $(\bar{u}(0),\bar{v}(0)) \in {\varPi}_{K}$, we have $\lim_{t \to \infty} \bar{v}(t)=0$. Similar to the proof above, we have lim _t→∞ v(t)=0 for any solution of (3) with (u(0),v(0))∈Π _K and w(0)>0. Thus, the set P _uw or P _uw ∪P _ϕ (if P _ϕ exists) is locally asymptotically stable in $R_{+}^{3}$ with a basin of attraction Ω. When a<a ₀, there is no interior equilibrium of (3) by Lemma 4.1. Similar to the proof of Theorem 4.4(ii), we conclude that $\operatorname{int}(R_{+}^{3} - {\varOmega})$ is empty. Thus, the result (ii) is proved. When a>a ₀, there are two interior equilibrium of (3) by Lemma 4.1. Let Ω be the basin of attraction of P _uw ∪P _ϕ in $R_{+}^{3}$. Similar to the proof of Theorem 4.4(iii), system (3) restricted on $R_{+}^{3} - {\varOmega}$ is uniformly persistent. Thus, the result (iii) is proved.

Appendix D: Proof of Theorem 4.7

By $\mu_{w}^{K}<0$ and Proposition 3.2(i), P _K is globally asymptotically stable in the interior of the (u,w)-plane.

(i):

Since $\bar{B}>0$ or $\bar{{\varDelta}} <0$, it follows from Proposition 3.1(iii) that P _K is globally asymptotically stable in the interior of the (u,v)-plane. Similar to the proof of Theorem 4.3(ii), we obtain lim _t→∞ v(t)=lim _t→∞ w(t)=0.

(ii):

Since $\bar{B}<0$ and $\bar{{\varDelta}}>0$, there exist equilibria $P_{12}^{-}(x_{1}^{-},x_{2}^{-},0)$ and $P_{12}( x_{1}^{+},x_{2}^{+},0)$ on the (u,v)-plane.

• (a) From $\mu_{w}^{+}<0$ and the monotonicity of function u/(1+bu), we have $\mu_{w}^{-}<\mu_{w}^{+}<0$. Then $P_{uv}^{-}$ is a saddle point and has a two-dimensional stable manifold $S_{uv}^{-}$, and P _uv is locally asymptotically stable. By Proposition 3.1 and the comparison theorem, solutions of (3) with u(0)>0 satisfy either lim sup _t→∞ u(t)≤K,lim sup _t→∞ v(t)≤0, or lim sup _t→∞ u(t)≤u ⁺, lim sup _t→∞ v(t)≤v ⁺. For the former case, we have lim _t→∞ v(t)=0 and then lim _t→∞ w(t)=0. For the latter case, similar to the proof of Theorem 4.3(ii), we have lim _t→∞ w(t)=0. Because M _K and M _uv are forward invariant, the result (a) is proved by Proposition 3.1(ii).

• (b) Since $S_{uv}^{-}$ is two-dimensional, solutions of (3) with $(u(0),v(0),w(0))\in S_{uv}^{-}$ converge to $P_{uv}^{-}(u^{-},v^{-}, 0 )$, which implies that system (3) is not persistent.

• (c) Since $\mu_{w}^{-}>0$ and A>A ₀, P _K is locally asymptotically stable and there is no interior equilibrium of (3). Similar to the proof of Theorem 4.4(ii), P _K is globally asymptotically stable.

• (d) Since $\mu_{w}^{-}>0$ and A<A ₀, P _K is locally asymptotically stable and there are two interior equilibria of (3). Similar to the proof of Theorem 4.4(iii), the result (d) is proved.