Abstract
In thermal-related engineering such as thermal energy structures and nuclear waste disposal, it is essential to well understand volume change and excess pore water pressure buildup of soils under thermal cycles. However, most existing thermo-mechanical models can merely simulate one heating–cooling cycle and fail in capturing accumulation phenomenon due to multiple thermal cycles. In this study, a two-surface elasto-plastic model considering thermal cyclic behavior is proposed. This model is based on the bounding surface plasticity and progressive plasticity by introducing two yield surfaces and two loading yield limits. A dependency law is proposed by linking two loading yield limits with a thermal accumulation parameter nc, allowing the thermal cyclic behavior to be taken into account. Parameter nc controls the evolution rate of the inner loading yield limit approaching the loading yield limit following a thermal loading path. By extending the thermo-hydro-mechanical equations into the elastic–plastic state, the excess pore water pressure buildup of soil due to thermal cycles is also accounted. Then, thermal cycle tests on four fine-grained soils (natural Boom clay, Geneva clay, Bonny silt, and reconstituted Pontida clay) under different OCRs and stresses are simulated and compared. The results show that the proposed model can well describe both strain accumulation phenomenon and excess pore water pressure buildup of fine-grained soils under the effect of thermal cycles.













Similar content being viewed by others
Abbreviations
- α 0 :
-
Parameter controlling thermal plastic behavior
- α 1 :
-
Parameter controlling thermal elastic behavior
- β :
-
The size parameter of potential plastic surface
- λ, κ :
-
Slope of isotropic loading, reloading line, respectively
- υ, ν :
-
Current void ratio, Poisson’s ratio, respectively
- A d :
-
Parameter controlling the contribution of shear plastic strain
- dε, dσ, dT :
-
Total strain, stress, temperature increment respectively
- \({\text{d}}{\varvec{\upvarepsilon}}_{\sigma }^{\text{e}}\), \({\text{d}}{\varvec{\upvarepsilon}}_{\text{T}}^{\text{e}}\) :
-
Stress, thermal-induced elastic strain increment, respectively
- \({\text{d}}\varepsilon_{\text{v}}\), \({\text{d}}\varepsilon_{\text{v}}^{\text{e}}\), \({\text{d}}\varepsilon_{\text{s}}^{\text{e}}\) :
-
Total volumetric, elastic volumetric, shear strain increment, respectively
- \({\text{d}}{\varvec{\upvarepsilon}}^{p}\), \({\text{d}}\varepsilon_{\text{v}}^{\text{p}}\), \({\text{d}}\varepsilon_{\text{s}}^{\text{p}}\) :
-
Plastic, plastic volumetric, plastic shear strain increment, respectively
- \(\varLambda\) :
-
Plastic multiplier
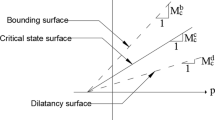
- fI, fY :
-
Loading surface, yield surface, respectively
- G, K, h :
-
Elastic shear, elastic bulk, plastic modulus, respectively
- kf, kg :
-
Parameter controlling the shape of yield surface, plastic potential surface, respectively
- M f :
-
Stress ratio at the apex of yield surface
- Mg, Mg0 :
-
Critical stress ratio at current, reference temperature, respectively
- \(p^{{\prime }}\), \(\bar{p}^{{\prime }}\) :
-
Current, mapping mean effective stress, respectively
- \(p_{\text{cT}}^{{\prime }}\), \(p_{{{\text{c}}0}}^{{\prime }}\) :
-
Pre-consolidation pressure in loading surface at current, reference temperature, respectively
- \(\bar{p}_{\text{cT}}^{{\prime }}\), \(\bar{p}_{\text{c0}}^{{\prime }}\) :
-
Pre-consolidation pressure in yield surface at current, reference temperature, respectively
- \(q,\bar{q}\) :
-
Current, mapping deviator stress, respectively
- r, r0 :
-
Reverse of OCR at current, reference temperature, respectively
- s :
-
Parameter controlling the rate of evolution of the slope of the stress–strain relation
- T, T0 :
-
Current, reference temperature, respectively
- De, Dep, Det :
-
Elastic, elasto-plastic, temperature stiffness matrix, respectively
- m :
-
Unit vector
References
Abuel-Naga HM, Bergado DT, Bouazza A, Ramana GV (2007) Volume change behaviour of saturated clays under drained heating conditions: experimental results and constitutive modeling. Can Geotech J 44:942–956
Amatya BL, Soga K, Bourne-Webb PJ, Amis T, Laloui L (2012) Thermo-mechanical behaviour of energy piles. Geotechnique 62:503–519
Baldi G, Hueckel T, Peano A, Pellegrini R (1991) Developments in modelling of thermohydro-geomechanical behaviour of Boom clay and clay-based buffer materials, vol 2. Commission of the European Communities
Borja RI, Tamagnini C, Amorosi A (1997) Coupling plasticity and energy-conserving elasticity models for clays. J Geotech Geoenviron Eng 123(10):948–957
Bourne-Webb PJ, Amatya B, Soga K, Amis T, Davidson C, Payne P (2009) Energy pile test at Lambeth College, London: geotechnical and thermodynamic aspects of pile response to heat cycles. Géotechnique 59:237–248
Campanella RG, Mitchell JK (1968) Influence of temperature variations on soil behavior. J Soil Mech Found Div 94(3):609–734
Collins IF, Houlsby GT (1997) Application of thermomechanical principles to the modelling of geotechnical materials. Proc R Soc A Math Phys Eng Sci 453(1964):1975–2001
Coussy O (2004) Poromechanics. Wiley, New York
Cui YJ, Sultan N, Delage P (2000) A thermomechanical model for saturated clays. Can Geotech J 37(3):607–620
Delage P, Sultan N, Cui YJ (2000) On the thermal consolidation of Boom clay. Can Geotech J 37(2):343–354
Demars KR, Charles RD (2011) Soil volume changes induced by temperature cycling. Can Geotech J 19(2):188–194
Di Donna A, Laloui L (2015) Response of soil subjected to thermal cyclic loading: experimental and constitutive study. Eng Geol 190:65–76
Eriksson LG (1989) Temperature effects on consolidation properties of sulphide clays. In: International conference on soil mechanics and foundation engineering, Balkema Publishers, AA/Taylor & Francis The Netherlands, pp 2087–2090
Gens A, Sanchez M, Guimaraes LD, Alonso EE, Lloret A, Olivella S et al (2009) A full-scale in situ heating test for high-level nuclear waste disposal: observations, analysis and interpretation. Geotechnique 59:377–399
Graham J, Tanaka N, Crilly T, Alfaro M (2001) Modified Cam-Clay modelling of temperature effects in clays. Can Geotech J 38:608–621
Hong PY, Pereira JM, Tang AM, Cui YJ (2013) On some advanced thermo-mechanical models for saturated clays. Int J Numer Anal Meth Geomech 37:2952–2971
Hong PY, Pereira JM, Cui YJ, Tang AM (2016) A two-surface thermomechanical model for saturated clays. Int J Numer Anal Meth Geomech 40:1059–1080
Hueckel T, Baldi G (1990) Thermoplasticity of saturated clays - experimental constitutive study. J Geotech Eng ASCE 116:1778–1796
Hueckel T, Borsetto M (1990) Thermoplasticity of saturated soils and shales: constitutive equations. J Geotech Eng ASCE 116:1765–1777
Hueckel T, Pellegrini R (1991) Thermoplastic modeling of undrained failure of saturated clay due to heating. Soils Found 31(3):1–16
Laloui L, François B (2009) ACMEG-T: soil thermoplasticity model. J Eng Mech 135(9):932–944
Lê T (2008) Comportement thermo-hydro-mécanique de l’argile de boom. PhD Thesis, École Nationale des Ponts et Chaussées, France
Lebon G, Jou D, Casas-Vázquez J (2008) Understanding non-equilibrium thermodynamics, vol 295. Springer, Berlin
Liu XC, Xiao YM, Inthavong K, Tu JY (2014) A fast and simple numerical model for a deeply buried underground tunnel in heating and cooling applications. Appl Therm Eng 62:545–552
Ma QJ, Ng CWW, Masin D, Zhou C (2017) An approach for modelling volume change of fine-grained soil subjected to thermal cycles. Can Geotech J 54:896–901
McDowell GR, Hau KW (2004) A generalised modified Cam clay model for clay and sand incorporating kinematic hardening and bounding surface plasticity. Granul Matter 6:11–16
Mimouni T, Laloui L (2015) Behaviour of a group of energy piles. Can Geotech J 52:1913–1929
Ng CWW, Shi C, Gunawan A, Laloui L (2014) Centrifuge modelling of energy piles subjected to heating and cooling cycles in clay. Géotechn Lett 4:310–316
Ng CWW, Shi C, Gunawan A, Laloui L, Liu HL (2015) Centrifuge modelling of heating effects on energy pile performance in saturated sand. Can Geotech J 52:1045–1057
Pastén C, Castillo E, Chong SH (2019) Thermo-mechanical ratcheting in soil–structure interfaces. Acta Geotech 14:1561–1569
Plum RL, Esrig MI (1969) Some temperature effects on soil compressibility and pore water pressure. Spec Report Highw Res Board 103:231
Robinet JC, Rahbaoui A, Plas F, Lebon P (1996) A constitutive thermomechanical model for saturated clays. Eng Geol 41:145–169
Spang B (2002) Excel add-in for properties of water and steam in si-units. Available from http://www.cheresources.com/iapwsif97.shtml
Vega A, McCartney JS (2015) Cyclic heating effects on thermal volume change of silt. Environ Geotech 2:257–268
Yao YP, Zhou AN (2013) Non-isothermal unified hardening model: a thermo-elasto-plastic model for clays. Géotechnique 63:1328–1345
Yao YP, Sun DA, Luo T (2004) A critical state model for sands dependent on stress and density. Int J Numer Anal Meth Geomech 28(4):323–337
Yao YP, Sun DA, Matsuoka H (2008) A unified constitutive model for both clay and sand with hardening parameter independent on stress path. Comput Geotech 35(2):210–222
Yavari N, Tang AM, Pereira JM, Hassen G (2016) Mechanical behaviour of a small-scale energy pile in saturated clay. Géotechnique 66:878–887
Zhang S, Leng W, Zhang F et al (2012) A simple thermo-elastoplastic model for geomaterials. Int J Plast 34:93–113
Zhou C, Fong KY, Ng CWW (2017) A new bounding surface model for thermal cyclic behaviour. Int J Numer Anal Meth Geomech 41:1656–1666
Zymnis DM, Whittle AJ, Cheng X (2019) Simulation of long-term thermo-mechanical response of clay using an advanced constitutive model. Acta Geotech 14:295–311
Acknowledgements
The present work is carried out with the support of National Natural Science Foundation of China (51608188, 758201011). The authors also wish to acknowledge the support of the European Commission by the Marie Skłodowska–Curie Actions HERCULES- Toward Geohazards Resilient Infrastructure Under Changing Climates (H2020-MSCA-RISE-2017, 778360).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1
The consistency condition of loading surface in ACC2-T model is adopted herein:
The plastic multiplier \(\varLambda\) and the plastic modulus h are obtained in non-isothermal condition:
The total strain increment is made up of mechanical elastic strain increment, thermal elastic strain increment and plastic strain increment.
The thermal elastic strain increment \({\text{d}}{\varvec{\upvarepsilon}}_{\text{T}}^{\text{e}}\) is defined as:
where m is the column vector with 1 at normal stress entries and 0 at shear stress entries.
By substituting Eq. (23) into Eq. (22), the general incremental stress–strain relation can be expressed:
where De is the mechanical elastic matrix.
By substituting the plastic multiplier into Eq. (24), the differential stress–strain equations can be obtained:
where Dep, Det are the mechanical elasto-plastic matrix and the thermal elasto-plastic matrix, respectively.
Appendix 2
Assume the soil is loaded and heated from Point A to Point B under two different loading paths I (\(A \to C \to B\)) and II(\(A \to D \to B\)) in Fig. 5. The soil is at the initial stress state (\(p^{\prime}_{0} ,r_{0} ,p^{\prime}_{c0} ,T_{1}\)) which automatically satisfies the yield condition:
Under loading path I, the soil element is isotropically loaded to stress point \(C\)(\(p^{\prime}_{0} + \Delta p^{\prime}_{1} ,r_{1} ,p^{\prime}_{{{\text{c}}1}} ,T_{1}\)) at constant temperature \(T_{1}\).
Then the soil element is heated up to stress point \(B\) (\(p^{\prime}_{0} + \Delta p^{\prime}_{1} ,r_{2} ,p^{\prime}_{{{\text{c}}2}} ,T_{1} + \Delta T_{1}\)) at constant mean effective stress \(p^{\prime}_{0} + \Delta p^{\prime}_{1}\).
Under loading path II, the soil element is firstly heated up to stress point \(D\)(\(p^{\prime}_{0} ,r_{3} ,p^{\prime}_{{{\text{c}}3}} ,T_{1} + \Delta T_{1}\)) at constant mean effective stress \(p^{\prime}_{0}\).
Then, the soil element is isotropically loaded to stress point \(B\)(\(p^{\prime}_{0} + \Delta p^{\prime}_{1} ,r_{ 4} ,p^{\prime}_{\text{c4}} ,T_{1} + \Delta T_{1}\)) at constant temperature \(T_{1} + \Delta T_{1}\).
Compare Eqs. (32)–(34) and Eqs. (38)–(40), it appears clearly that the two different loading paths would reach the same final stress state, namely:
No matter Path I or Path II is followed, the plastic volumetric strain will be equal, which means that plastic volumetric strain in ACC2-T model is also loading path independent.
Rights and permissions
About this article
Cite this article
Cheng, W., Chen, Rp., Hong, Py. et al. A two-surface thermomechanical plasticity model considering thermal cyclic behavior. Acta Geotech. 15, 2741–2755 (2020). https://doi.org/10.1007/s11440-020-00999-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11440-020-00999-5