Skip to main content
SpringerLink
Log in

Non-Markovian discrete qubit dynamics

  • Article
  • Physics & Astronomy
  • Published:
Science Bulletin

Abstract

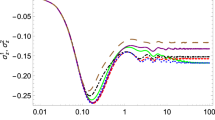
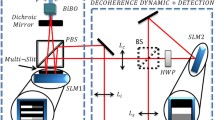
The study of open quantum systems is important for fundamental issues of quantum physics as well as for technological applications such as quantum information processing. The interaction of a quantum system with its environment is usually detrimental for the quantum properties of the system and leads to decoherence. However, sometimes a quantum control can lead to a coherent partial exchange of information between the system and the dynamics of the open system might become non-Markovian. In this article, we study experimentally discrete non-Markovian open quantum system dynamics. We implement a local control protocol using linear optics for controlling the information flow between the open system and the environment. We show how the transition from Markovian to non-Markovian dynamics can be controlled using only local operations for the open system.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Breuer HP, Petruccione F (2007) Theory of open quantum systems. Oxford University Press, Oxford

    Book  Google Scholar 

  2. Alicki R, Lendi K (1987) Lecture notes in physics. In: Quantum dynamical semigroups and applications. Springer, Berlin

  3. Gorini V, Kossakowski A, Sudarshan ECG (1976) Completely positive dynamical semigroups of \(N\)-level systems. J Math Phys 17:821–825

    Article  Google Scholar 

  4. Lindblad G (1976) On the generators of quantum dynamical semigroups. Comm Math Phys 48:119–130

    Article  Google Scholar 

  5. Gardiner CW, Zoller P (1999) Quantum noise. Springer series in synergetics. Springer, Berlin

    Google Scholar 

  6. Breuer HP, Laine EM, Piilo J et al (2015) Non-Markovian dynamics in open quantum systems. arXiv:1505.01385

  7. Wolf MM, Cirac JI (2008) Dividing quantum channels. Comm Math Phys 279:147–168

    Article  Google Scholar 

  8. Wolf MM, Eisert J, Cubitt TS et al (2008) Assessing non-Markovian quantum dynamics. Phys Rev Lett 101:150402

    Article  Google Scholar 

  9. Breuer HP, Laine EM, Piilo J (2009) Measure for the degree of non-Markovian behavior of quantum processes in open systems. Phys Rev Lett 103:210401

    Article  Google Scholar 

  10. Rivas Á, Huelga SF, Plenio MB (2010) Entanglement and non-Markovianity of quantum evolutions. Phys Rev Lett 105:050403

    Article  Google Scholar 

  11. Luo S, Fu S, Song H (2012) Quantifying non-Markovianity via correlations. Phys Rev A 86:044101

    Article  Google Scholar 

  12. Lorenzo S, Plastina F, Paternostro M (2013) Geometrical characterization of non-Markovianity. Phys Rev A 88:020102

    Article  Google Scholar 

  13. Chruściński D, Maniscalco S (2014) Degree of non-Markovianity of quantum evolution. Phys Rev Lett 112:120404

    Article  Google Scholar 

  14. Bylicka B, Chruściński D, Maniscalco S (2014) Non-Markovianity and reservoir memory of quantum channels: a quantum information theory perspective. Sci Rep 4:5720

    Article  Google Scholar 

  15. Pollock FA, Rodríguez-Rosario C, Frauenheim T et al (2015) Complete framework for efficient characterisation of non-Markovian processes. arXiv:1512.00589

  16. Liu BH, Li L, Huang YF et al (2011) Experimental control of the transition from Markovian to non-Markovian dynamics of open quantum systems. Nat Phys 7:931–934

    Article  Google Scholar 

  17. Smirne A, Brivio D, Cialdi S et al (2011) Experimental investigation of initial system-environment correlations via trace-distance evolution. Phys Rev A 84:032112

    Article  Google Scholar 

  18. Chiuri A, Greganti C, Mazzola L et al (2012) Linear optics simulation of quantum non-Markovian dynamics. Sci Rep 2:968

    Article  Google Scholar 

  19. Bernardes NK, Cuevas A, Orieux A et al (2015) Experimental observation of weak non-Markovianity. Sci Rep 5:17520

    Article  Google Scholar 

  20. Jirari H, Pötz W (2006) Quantum optimal control theory and dynamic coupling in the spin-boson model. Phys Rev A 74:022306

    Article  Google Scholar 

  21. Rebentrost P, Serban I, Schulte-Herbrüggen T et al (2009) Optimal control of a qubit coupled to a non-Markovian environment. Phys Rev Lett 102:090401

    Article  Google Scholar 

  22. Lapert M, Zhang Y, Braun M et al (2010) Singular extremals for the time-optimal control of dissipative spin \(\frac{1}{2}\) particles. Phys Rev Lett 104:83001

    Article  Google Scholar 

  23. Schmidt R, Negretti A, Ankerhold J et al (2011) Optimal control of open quantum systems: cooperative effects of driving and dissipation. Phys Rev Lett 107:130404

    Article  Google Scholar 

  24. Hwang B, Goan HS (2012) Optimal control for non-Markovian open quantum systems. Phys Rev A 85:032321

    Article  Google Scholar 

  25. Tai JS, Lin KT, Goan HS (2014) Optimal control of quantum gates in an exactly solvable non-Markovian open quantum bit system. Phys Rev A 89:062310

    Article  Google Scholar 

  26. D’Arrigo A, Franco RL, Beneti G et al (2014) Recovering entantlement by local operations. Ann Phys 350:211–224

    Article  Google Scholar 

  27. Orieux A, D’Arrigo A, Ferranti G et al (2015) Experimental on-demand recovery of entanglement by local operations within non-Markovian dynamics. Sci Rep 5:8575

    Article  Google Scholar 

  28. Dong WB, Wu RB, Yuan XH et al (2015) The modelling of quantum control systems. Sci Bull 60:1493–1508

    Article  Google Scholar 

  29. Jing J, Wu LA (2015) Overview of quantum memory protection and adiabaticity induction by fast signal control. Sci Bull 60:328–335

    Article  Google Scholar 

  30. Jin J, Giovannetti V, Fazio R et al (2015) All-optical non-Markovian stroboscopic quantum simulator. Phys Rev A 91:012122

    Article  Google Scholar 

  31. Viola L, Knill E, Lloyd S (1999) Dynamical decoupling of open quantum systems. Phys Rev Lett 82:2417

    Article  Google Scholar 

  32. Uhrig GS (2007) Keeping a quantum bit alive by optimized \(\pi \)-pulse sequences. Phys Rev Lett 98:100504

    Article  Google Scholar 

  33. Lidar DA (2014) Quantum information and computation for chemistry. Wiley, New York

    Google Scholar 

  34. Lo Franco R, D’Arrigo A, Falci G et al (2014) Preserving entanglement and nonlocality in solid-state qubits by dynamical decoupling. Phys Rev B 90:054304

    Article  Google Scholar 

  35. Nielsen M, Chuang I (2000) Quantum computation and quantum information. Cambridge University Press, Cambridge

    Google Scholar 

  36. Luoma K, Piilo J (2015) Discrete dynamics and non-Markovianity. J Phys B. arXiv:1509.04231

Download references

Acknowledgments

This work was supported by the Magnus Ehrnrooth Foundation and Academy of Finland (287750), National Natural Science Foundation of China (61327901, 11274289 and 11325419), the Strategic Priority Research Program (B) of the Chinese Academy of Sciences (XDB01030300). C.F.L. and J.P. acknowledge financial support from the EU Collaborative project QuProCS (Grant Agreement 641277).

Author information

Authors and Affiliations

  1. Key Laboratory of Quantum Information, University of Science and Technology of China, Chinese Academy of Sciences, Hefei, 230026, China

    Jun Sun, Yong-Nan Sun, Chuan-Feng Li & Guang-Can Guo

  2. Synergetic Innovation Center of Quantum Information and Quantum Physics, University of Science and Technology of China, Hefei, 230026, China

    Jun Sun, Yong-Nan Sun, Chuan-Feng Li & Guang-Can Guo

  3. Institut für Theoretische Physik, Technische Universität Dresden, 01062, Dresden, Germany

    Kimmo Luoma

  4. Department of Physics and Astronomy, Turku Centre for Quantum Physics, University of Turku, 20014, Turun Yliopisto, Finland

    Jyrki Piilo

Authors
  1. Jun Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yong-Nan Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Chuan-Feng Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Guang-Can Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Kimmo Luoma
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Jyrki Piilo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Chuan-Feng Li, Kimmo Luoma or Jyrki Piilo.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sun, J., Sun, YN., Li, CF. et al. Non-Markovian discrete qubit dynamics. Sci. Bull. 61, 1031–1036 (2016). https://doi.org/10.1007/s11434-016-1089-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11434-016-1089-8

Keywords

Search

Navigation