Credit diversification and banking systemic risk

Abstract

Banks generally enhance their competitiveness through credit diversification. However, credit diversification may trigger serious systemic risk through the effect of fire sales. Using data from the Chinese banking market, we quantify the fire sales of credits and provide empirical evidence for the impact of credit diversification on systemic risk. The results reveal that an increased level of credit diversification promotes systemic risk and is more pronounced among small banks. Both the credit loss and network complexity of individual banks contribute to the impact of credit diversification on systemic risk. Declining economic prospects will also promote credit diversification to cause more systemic risk. However, tight macroprudential regulation helps to mitigate the promotion of credit diversification. These findings provide regulatory insights for systemic risk prevention.

Appendices

Appendix A. Market value of credit

As the credit of banks cannot be traded directly in the market, we determine the market values based on the option pricing approach proposed by Merton (1974). Treating credit as credit default bonds, the present market values of credit are equal to the risk-free present value of credit minus the present value of expected default losses on credit under risk-neutral conditions, that is, the European put option pricing based on credit.

Suppose that D_j represents the total credit to sector j and ${\text{MV}}_{j}^{t}$ represents the market value of sector j at time t, which is characterized by a geometric Brownian motion as follows:

$${\text{MV}}_{j}^{T} = {\text{MV}}_{j}^{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right) + \sigma \sqrt {T - t} z}}$$

(25)

where T is the maturity of credit, r is the risk-free interest rate, $\sigma_{V}$ is the volatility of sector j, and z is a standard normal distribution.

If the market value of sector j moves below its total credit, the repayment of credit will be affected. Therefore, the expected default loss of credit in sector j is expressed as the difference between the total credit and its market value. This is equivalent to the payoff at maturity for a put option p_t whose underlying is the market value of sector j, which is expressed as

$$E\left[ {p_{T} } \right] = E\left[ {\max \left\{ {D_{j} - {\text{MV}}_{j}^{T} ,0} \right\}} \right]$$

(26)

This equation is further expressed as a function of z by substituting Eq. (1) into it, that is,

$$E\left[ {p_{T} } \right] = \int_{ - \infty }^{ + \infty } {\max \left\{ {D_{j} - {\text{MV}}_{j}^{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right) + \sigma_{V} \sqrt {T - t} z}} ,0} \right\}} g\left( z \right){\text{d}}z$$

(27)

By simplifying the maximum function that $D_{j} - {\text{MV}}_{j}^{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right) + \sigma_{V} \sqrt {T - t} z}} > 0$, we have $z < - d_{2}$, where

$$d_{2} = \frac{{\ln \left( {{{{\text{MV}}_{j}^{t} } \mathord{\left/ {\vphantom {{{\text{MV}}_{j}^{t} } {D_{j} }}} \right. \kern-0pt} {D_{j} }}} \right) + \left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right)}}{{\sigma_{V} \sqrt {T - t} }}$$

(28)

Accordingly, the integration interval of Eq. (3) is adjusted as

$$\begin{gathered} E\left[ {p_{T} } \right] = \int_{ - \infty }^{{ - d_{2} }} {\left[ {D_{j} - {\text{MV}}_{j}^{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right) + \sigma_{V} \sqrt {T - t} z}} } \right]g\left( z \right){\text{d}}z} \hfill \\ \, = D_{j} \int_{ - \infty }^{{ - d_{2} }} {g\left( z \right){\text{d}}z - {\text{MV}}_{j}^{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right)}} \int_{ - \infty }^{{ - d_{2} }} {e^{{\sigma_{V} \sqrt {T - t} z}} g\left( z \right){\text{d}}z} } \hfill \\ \end{gathered}$$

(29)

Given that $\int_{ - \infty }^{{ - d_{2} }} {e^{{\sigma_{V} \sqrt {T - t} z}} g\left( z \right){\text{d}}z} = \frac{{e^{{{{\sigma_{V}^{2} \left( {T - t} \right)} \mathord{\left/ {\vphantom {{\sigma_{V}^{2} \left( {T - t} \right)} 2}} \right. \kern-0pt} 2}}} }}{{\sqrt {2\pi } }}\int_{ - \infty }^{{ - d_{2} }} {e^{{{{ - \left( {z - \sigma_{V} \sqrt {T - t} } \right)^{2} } \mathord{\left/ {\vphantom {{ - \left( {z - \sigma_{V} \sqrt {T - t} } \right)^{2} } 2}} \right. \kern-0pt} 2}}} {\text{d}}z}$, we have

$$\begin{gathered} E\left[ {p_{T} } \right] = D_{j} N\left( { - d_{2} } \right) - {\text{MV}}_{t} e^{{\left( {r - {{\sigma_{V}^{2} } \mathord{\left/ {\vphantom {{\sigma_{V}^{2} } 2}} \right. \kern-0pt} 2}} \right)\left( {T - t} \right)}} e^{{{{\sigma_{V}^{2} \left( {T - t} \right)} \mathord{\left/ {\vphantom {{\sigma_{V}^{2} \left( {T - t} \right)} 2}} \right. \kern-0pt} 2}}} \int_{ - \infty }^{{ - d_{1} }} {\frac{1}{{\sqrt {2\pi } }}e^{{{{ - \omega^{2} } \mathord{\left/ {\vphantom {{ - \omega^{2} } 2}} \right. \kern-0pt} 2}}} {\text{d}}\omega } \hfill \\ \, = D_{j} N\left( { - d_{2} } \right) - {\text{MV}}_{j}^{t} e^{{r\left( {T - t} \right)}} N\left( { - d_{1} } \right) \hfill \\ \end{gathered}$$

(30)

where $N\left( \cdot \right)$ represents the cumulative probability density function of a standard normal distribution and $d_{1} = d_{2} + \sigma_{V} \sqrt {T - t}$.

Therefore, the present value of the expected default loss of credit in sector j is

$$p_{t} = e^{{ - r\left( {T - t} \right)}} E\left[ {p_{T} } \right] = D_{j} e^{{ - r\left( {T - t} \right)}} N\left( { - d_{2} } \right) - {\text{MV}}_{j}^{t} N\left( { - d_{1} } \right)$$

(31)

From this, the market value of credit, defined as the difference between the risk-free discounted value of credit and the present value of the expected default losses, is determined by:

$$\begin{gathered} V_{j}^{t} = D_{j} e^{{ - r\left( {T - t} \right)}} - D_{j} e^{{ - r\left( {T - t} \right)}} N\left( { - d_{2} } \right) + {\text{MV}}_{j}^{t} N\left( { - d_{1} } \right) \hfill \\ \, = D_{j} e^{{ - r\left( {T - t} \right)}} N\left( {d_{2} } \right) + {\text{MV}}_{j}^{t} N\left( { - d_{1} } \right) \hfill \\ \end{gathered}$$

(32)

Define $g_{i} = {{\left( {{\text{MV}}_{j}^{t} - D_{j} } \right)} \mathord{\left/ {\vphantom {{\left( {{\text{MV}}_{j}^{t} - D_{j} } \right)} {{\text{MV}}_{j}^{t} }}} \right. \kern-0pt} {{\text{MV}}_{j}^{t} }}$ as the initial leverage ratio of sector j and t = 0; then,

$$V_{j} = D_{j} e^{ - rT} N\left( {d_{2} } \right) + D_{j} \left( {1 - g_{j} } \right)^{ - 1} N\left( { - d_{1} } \right)$$

(33)

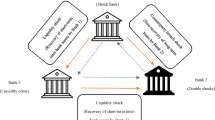
Appendix B. Dynamic updates of the equilibrium

We consider the contagion process of systemic risk arising from the interaction between interbank loans and credit markets in this paper. As there is a cascade of insolvency events, both interbank defaults and credit depreciations in the banking market are dynamically updated until equilibrium is reached when no more insolvency events occur. The insolvent banks in equilibrium are determined by the following algorithm: The variables $L_{i}^{0} { = }b_{i}^{0}$ and $f_{j}^{0} = 1$ are defined at the initial time t = 0, where $b_{i}^{0} = \sum\nolimits_{k = 1}^{N} {L_{ki} }$. The following processes are repeated until convergence. All insolvent banks are in the set $F^{t}$ when the algorithm ends.

• I.t=t+1.

• II.Determine the set of insolvent banks

  $$F^{t} { = }\left\{ {i \in \left[ {1,N} \right]|SR_{i}^{t} = w_{i}^{0} } \right\}$$

  (34)

  where $SR_{i}^{t}$ is the measured systemic risk contribution of bank i at time t.

• III.Calculate the market value of credits based on fire sales

  $$f_{j}^{t} = \exp \left( { - \beta_{j} \sum\limits_{i \in F}^{N} {s_{ij} } } \right)$$

  (35)

  where $s_{ij}$ denotes the present value of liquidated credits from insolvent bank i to sector j.

• IV.Determine interbank repayments

  $$L_{i}^{t} = \min \left\{ {b_{i}^{0} ,w_{i}^{0} + b_{i}^{0} + \sum\limits_{j = 1}^{M} {V_{ij}^{t} } - \sum\limits_{j = 1}^{M} {V_{ij}^{0} } + \sum\limits_{k = 1}^{N} {\pi_{is} b_{k}^{t} } - L_{i}^{0} } \right\}$$

  (36)

  where $V_{ij}^{t}$ denotes the market value of credit at time t from bank i to sector j. $b_{k}^{t} = \sum\nolimits_{i = 1}^{N} {L_{ik}^{t} }$ indicates that it is a fixed-point iteration problem.

• V.The algorithm ends if $t \ge 2$ and $F^{t} = F^{t - 1}$.

Appendix C. DebtRank method

For systemic risk measurement based on network models, the systemic cost function is generally used. The systemic cost function measures the situation in which an insolvent bank leads to the failure of other banks through the product of the number and probability of failures. However, if the affected banks have sufficient capital to cover the shocks, systemic risk will be underestimated by the systemic cost of failures. This problem is solved by a DebtRank method (Battiston et al. 2012), where the net worth losses caused by counterparties for surviving banks are contained in systemic risk.

DebtRank uses cumulative net worth losses for systemic risk measurement. Therefore, the systemic risk contribution of bank i at time t is represented by the relative net worth losses, that is,

$${\text{DR}}_{i}^{t} = \min \left\{ {1,\frac{{w_{i}^{0} - w_{i}^{t} }}{{w_{i}^{0} }}} \right\}$$

(37)

The bank goes bankrupt when the loss is greater than its net worth, which is expressed as ${\text{DR}}_{i}^{t} = 1$.

The net worth loss is mainly contributed by interbank defaults and credit fire sales in this study. Therefore, the net worth loss of bank i is expanded as

$${\text{DR}}_{i}^{t} = \min \left\{ {1,\sum\limits_{k = 1}^{N} {\frac{{L_{ik}^{0} - L_{ik}^{t} }}{{w_{i}^{0} }}} + \sum\limits_{j = 1}^{M} {\frac{{V_{ij}^{0} - V_{ij}^{t} }}{{w_{i}^{0} }}} } \right\}$$

(38)

The real payment of interbank loans received by a creditor depends on the failure of its counterparties in DebtRank. Therefore, the real value of interbank lending from bank i to bank k at time t is determined by its initial value and the default probability of bank k as follows:

$$L_{ik}^{t} = L_{ik}^{0} \left( {1 - {\text{DR}}_{k}^{t - 1} } \right)$$

(39)

This means that the changes in net worth lead to a dynamic default probability. Accordingly, the relative net worth loss of bank i is expressed as

$${\text{DR}}_{i}^{t} = \min \left\{ {1,\;\sum\limits_{k = 1}^{N} {\frac{{L_{ik}^{0} }}{{w_{i}^{0} }}{\text{DR}}_{k}^{t - 1} } + \sum\limits_{j = 1}^{M} {\frac{{V_{ij}^{0} - V_{ij}^{t} }}{{w_{i}^{0} }}} } \right\}$$

(40)

We further take the size of banks into account in DebtRank and multiply ${\text{DR}}_{i}^{t}$ by the net worth of bank i. Then, systemic risk measurement is constructed as follows:

$${\text{SR}}_{i}^{t} = \min \left\{ {w_{i}^{0} ,\;\sum\limits_{k = 1}^{N} {L_{ik}^{0} {\text{SR}}_{k}^{t - 1} } + \sum\limits_{j = 1}^{M} {\left( {V_{ij}^{0} - V_{ij}^{t} } \right)} } \right\}$$

(41)