Energy efficiency and energy rebound, intensity, and output effects in transport sector of Pakistan

Abstract

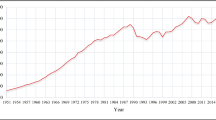
According to Jevon’s paradox, energy efficiency leads to more energy consumption instead of low. So, calculating the size of energy rebound effect is need of the time to devise sustainable environmental and energy policies. This paper aims to analyze the impact of energy efficiency on energy consumption of transport sector in Pakistan by using time series data from 1980 to 2018. This incremental energy consumption channelizes through intensity and output effects. The study uses both Cobb–Douglas (C-D) and constant elasticity of substitution (CES) aggregate production functions to find the magnitudes of energy rebound effect. As the analysis of energy rebound effect is sensitive to the selection of data, model, and methodology. C-D production function deals with energy rebound effect, while CES provides extra information in the form of energy intensity and output effects along with energy rebound effect. The C-D function is estimated with linear estimation technique, while the CES function is estimated through nonlinear optimization method. The results indicate relatively low magnitudes of energy rebound effect in case of C-D function, e.g., 2% in the short run and about 36% in the long run while about 70% energy rebound, 63% energy intensity, and 7% output effect in the transport sector of Pakistan in the long run by using CES function. As anticipated, energy efficiency is less effective in terms of energy savings in transport sector due to energy rebound effect. Therefore, policy makers should incorporate energy rebound effect to achieve sustainable environmental goals along with economic growth path.

Appendices

Appendix 1. Cobb–Douglas production function

$${Y}_{t}=A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}({\tau }_{t}{{F}_{t})}^{{\beta }_{3}}$$

In above equation, $A$ is total factor productivity. ${\beta }_{1}$, ${\beta }_{2},$ and ${\beta }_{3}$ are shares of capital, labor, and energy, respectively.

$${Y}_{t}=A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}{{F}_{t}}^{{\beta }_{3}}$$

(14)

The partial derivative of output with respect to energy services of above equation will give us the marginal product of energy. By assuming perfect competition in economy, the marginal product of energy is equal to price of energy.

$$\frac{\partial {Y}_{t}}{\partial {F}_{t}}={\beta }_{3}A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}{{F}_{t}}^{{\beta }_{3}-1}$$

(15)

$$\begin{array}{c}{P}_{F}={\beta }_{3}A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}{{F}_{t}}^{{\beta }_{3}-1}\\ =\frac{{\beta }_{3}}{{F}_{t}}A{K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}{{F}_{t}}^{{\beta }_{3}}\\ {P}_{F}=\frac{{\beta }_{3}}{{F}_{t}}{Y}_{t}\end{array}$$

(16)

$${F}_{t}=\frac{{\beta }_{3}}{{P}_{F}}{Y}_{t}$$

(17)

Put above value into the production function.

$${Y}_{t}=A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}{\left(\frac{{\beta }_{3}}{{P}_{F}}{Y}_{t}\right)}^{{\beta }_{3}}$$

(18)

Now solve above the equation for ${(Y}_{t}$) and now output is function of energy efficiency instead of energy consumption.

$${Y}_{t}={\left(\frac{{\beta }_{3}}{{P}_{F}}\right)}^{{~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.}{\left(A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}\right)}^{{~}^{1}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.} {{\tau }_{t}}^{{~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.}$$

(19)

Now get the output effect of energy efficiency by taking partial derivative of Eq. (19) with respect to energy efficiency.

$$\begin{array}{c}\frac{\partial {Y}_{t}}{\partial {\tau }_{t}}=\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right){\left(\frac{{\beta }_{3}}{{P}_{F}}\right)}^{{~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.}{\left(A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}\right)}^{{~}^{1}\!\left/ \!{~}_{{\beta }_{1}+ {\beta }_{2}}\right.} {{\tau }_{t}}^{\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right) -1}\\ =\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right)\frac{{Y}_{t}}{{\tau }_{t}}\\ \frac{\partial {Y}_{t}}{\partial {\tau }_{t}}\frac{{\tau }_{t}}{{Y}_{t}}=\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right)\end{array}$$

(20)

Equation (20) is elasticity of output with respect to energy efficiency. Put Eq. (19) into Eq. (17).

$${F}_{t}=\frac{{\beta }_{3}}{{P}_{F}}{\left(\frac{{\beta }_{3}}{{P}_{F}}\right)}^{{~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.}{\left(A {K}_{t}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}\right)}^{{~}^{1}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.} {{\tau }_{t}}^{{~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.}$$

(21)

From Eq. (21), energy rebound effect is calculated by taking partial derivative of energy consumption with respect to energy efficiency.

$$\begin{array}{c}\frac{\partial {F}_{t}}{\partial {\tau }_{t}}=\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right) \frac{{F}_{t}}{{\tau }_{t}}\\ \frac{\partial {F}_{t}}{\partial {\tau }_{t}}\frac{{\tau }_{t}}{{F}_{t}}=\left({~}^{{\beta }_{3}}\!\left/ \!{~}_{{\beta }_{1}+{\beta }_{2}}\right.\right)\end{array}$$

(22)

Short-run energy rebound effect

The short-run energy rebound effect is identical to output effect that is given in Eq. (20). In the long run, the marginal product of capital is equal to rent of capital by assuming perfect competition in an economy.

$${r}_{t}={\beta }_{1}(\frac{{Y}_{t}}{{K}_{t}})$$

(23)

Here, share of capital $({\beta }_{1})$ and average product of capital are determining the real rent of capital.

$${K}_{t}={ \beta }_{1}(\frac{{Y}_{t}}{{r}_{t}})$$

(24)

By putting (24) and (17) into production function.

$$\begin{array}{c}{Y}_{t}=\mathrm{A}{({ \beta }_{1}\frac{{Y}_{t}}{{r}_{t}})}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}({\frac{{\beta }_{3}}{{P}_{F}}{Y}_{t}) }^{{\beta }_{3}}\\ {Y}_{t}^{{\beta }_{2}}=A {(\frac{{ \beta }_{1}}{{r}_{t}})}^{{\beta }_{1}}{L}_{t}^{{\beta }_{2}}{{\tau }_{t}}^{{\beta }_{3}}({\frac{{\beta }_{3}}{{P}_{F}}) }^{{\beta }_{3}}\\ \begin{array}{c}{Y}_{t}=\left({A}^{1/{\beta }_{2}} {(\frac{{ \beta }_{1}}{{r}_{t}})}^{{\beta }_{1/}{\beta }_{2}}{L}_{t}{{\tau }_{t}}^{({\beta }_{3})/{\beta }_{2}}({\frac{{\beta }_{3}}{{P}_{F}}) }^{({\beta }_{3})/{\beta }_{2}}\right) \\ \frac{\partial {Y}_{t}}{\partial {\tau }_{t}}= \frac{{\beta }_{3}}{{\beta }_{2}}\left({A}^{1/{\beta }_{2}} {(\frac{{ \beta }_{1}}{{r}_{t}})}^{{\beta }_{1/}{\beta }_{2}}{L}_{t}{{\tau }_{t}}^{({\beta }_{3})/{\beta }_{2}}({\frac{{\beta }_{3}}{{P}_{F}})}^{({\beta }_{3})/{\beta }_{2}}\right)\frac{1}{{\tau }_{t}}\\ \frac{\partial {Y}_{t}}{\partial {\tau }_{t}}= \frac{{\beta }_{3}}{{ \beta }_{2}}\frac{{Y}_{t}}{{\tau }_{t}}\end{array}\end{array}$$

(25)

$$\frac{\partial {Y}_{t}}{\partial {\tau }_{t}}\frac{{\tau }_{t}}{{Y}_{t}}= \frac{{\beta }_{3}}{{ \beta }_{2}}$$

(26)

$${F}_{t}=\frac{{\beta }_{3}}{{P}_{F}}\left({A}^{1/{\beta }_{2}} {(\frac{{ \beta }_{1}}{{r}_{t}})}^{{\beta }_{1/}{\beta }_{2}}{L}_{t}{{\tau }_{t}}^{({\beta }_{3})/{\beta }_{2}}\right)$$

(27)

$$\begin{array}{c}\frac{\partial {F}_{t}}{\partial {\tau }_{t}}=\left(\frac{{\beta }_{3}}{{ \beta }_{2}}\right) \frac{{F}_{t}}{{\tau }_{t}}\\ \frac{\partial {F}_{t}}{\partial {\tau }_{t}}\frac{{\tau }_{t}}{{F}_{t}}=\left(\frac{{\beta }_{3}}{{ \beta }_{2}}\right)\end{array}$$

(28)

Appendix 2. CES and long run energy rebound effect

This part of derivation is partially published by (Ullah et al. 2022) (with different topic, data, and analysis).

Taking the partial derivative of Eq. (24) with respect to fuel (F) and for the sake of simplicity, let pause the time subscript.

$$\begin{array}{c}\frac{\partial \mathrm{Y}}{\partial \mathrm{F}}=\frac{-1}{\rho }[\gamma \{b(\alpha {K}^{-\rho }+(1-\alpha ){L}^{-\rho }{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{-\rho }{\}}^{- \frac{1}{\rho }-1}](-\rho )(1-b)(\tau F{)}^{-\rho -1})\tau \\ =[\gamma \{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{\rho }{\}}^{\frac{-1}{\rho }-1}](1-b)(F{)}^{-\rho -1}{\tau }^{-\rho }\\ \begin{array}{c}=(1-b){\tau }^{-\rho }[{Y}^{1+\rho }](F{)}^{-\rho -1}\\ =(1-b){\tau }^{-\rho }(Y/F{)}^{1+\rho }\\ {\mathrm{P}}_{\mathrm{F}}=(1-b){\tau }^{-\rho }(Y/F{)}^{1+\rho }\end{array}\end{array}$$

(29)

The left-hand side is marginal product of energy that is equal to real price of energy ($\frac{\partial \mathrm{Y}}{\partial \mathrm{F}}={\mathrm{P}}_{\mathrm{F}}$). If multiply by ($\frac{Y}{F}$) on both sides.

$$\begin{array}{c}\left(F/Y\right)\frac{\partial Y}{\partial F}=\left(1-b\right){\tau }^{-\rho }{\left[Y/F\right]}^{1+\rho }\left(F/Y\right)\\ {\eta }_{y,F}=\left(1-b\right){\tau }^{-\rho }{\left[Y/F\right]}^{\rho }\end{array}$$

(30)

where ${\eta }_{y,F}$ is elasticity of output with respect to energy consumption. Now, we take the partial derivative of Eq. (28) with respect to energy efficiency (τ).

$$\begin{array}{c}\frac{\partial \mathrm{y}}{\partial\uptau }=- \frac{1}{\rho }[\gamma \{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+\left(1-b\right)\left(\tau F{)}^{-\rho }{\}}^{- \frac{1}{\rho }-1}\right](-\rho )(1-b)(\tau F{)}^{-\rho -1}\\ =[A\{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{\rho }{\}}^{\frac{-1}{\rho }-1}](1-b)(\tau {)}^{-\rho -1}{F}^{-\rho }\\ \begin{array}{c}=[A\{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{\rho }{\}}^{\frac{-(1+\rho )}{\rho }}](1-b)(\tau {)}^{-\rho -1}{F}^{-\rho }\\ =[A\{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{\rho }{\}}^{\frac{-1}{\rho }}{]}^{(1+\rho )}(1-b)(\tau {)}^{-\rho -1}{F}^{-\rho }\\ =(1-b){F}^{-\rho }{Y}^{(1+\rho )}{\tau }^{-\rho -1}\end{array}\end{array}$$

(31)

$$\partial \mathrm{Y}/\partial\uptau =(1-b){F}^{-\rho }(Y/\tau {)}^{(1+\rho )}$$

(32)

To obtain the energy rebound effect, study uses an implicit function theorem (Brockway et al. 2017a; Ullah et al. 2022). Study needs only two Eqs. (33 and 34) to capture the energy efficiency impact on economy via two channels (output and intensity) in the short run, while study solves the following three equations with the help of implicit function theorem for the long-run macro energy rebound effect.

Long-run analysis

$${\upmu }_{1}=g(Y, f( K,L,\tau F)$$

(33)

$${\upmu }_{2}=h({S}_{F}, \frac{\partial f}{\partial F}\left( K,L,\tau F\right)\frac{F}{Y})$$

(34)

$${\upmu }_{3}=k({S}_{k}, \frac{\partial f}{\partial k}\left( K,L,\tau F\right)\frac{K}{Y})$$

(35)

Let revisit Eq. (29)

$$\begin{array}{c}\begin{array}{c}\left(F/Y\right)\frac{\partial \mathrm{Y}}{\partial \mathrm{F}}=\left(1-b\right){\tau }^{-\rho }{\left[Y/F\right]}^{1+\rho }\left(F/Y\right)\\ {S}_{F}=\left(1-b\right){\tau }^{-\rho }{\left[Y/F\right]}^{\rho }\end{array}\\ \frac{\partial \mathrm{Y}}{\partial \mathrm{F}} (F/Y)={\mathrm{S}}_{\mathrm{F}}\\ {P}_{F}(F/Y)={\mathrm{S}}_{\mathrm{F}}\end{array}$$

(36)

$$\begin{array}{cc}{P}_{F}F={\mathrm{S}}_{\mathrm{F}} \mathrm{Y}& \mathrm{Y}-\frac{{P}_{F}F}{{S}_{F}}=0\end{array}$$

(37)

Study derives the responses of endogenous variables (Y and F) due to a exogenous shock of energy efficiency (τ). Jacobian matrix is utilized to complete this task.

$$\left[\begin{array}{c}\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\\ \frac{\partial K}{\partial \tau }\end{array}\right]= {-J}^{-1}\left[\begin{array}{c}\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\\ \frac{\partial {\mu }_{3}}{\partial \tau }\end{array}\right]$$

(38)

The above column vector (38) is also called efficiency gain vector. Then Jacobian matrix is like this.

$$J=\left[\begin{array}{ccc}\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial Y}\end{array}& \frac{\partial {\mu }_{1}}{\partial F}& \begin{array}{c}\frac{\partial {\mu }_{1}}{\partial K}\end{array}\\ \frac{\partial {\mu }_{2}}{\partial Y}& \frac{\partial {\mu }_{2}}{\partial F}& \begin{array}{c}\frac{\partial {\mu }_{2}}{\partial K}\end{array}\\ \frac{\partial {\mu }_{3}}{\partial Y}& \frac{\partial {\mu }_{3}}{\partial F}& \begin{array}{c}\frac{\partial {\mu }_{3}}{\partial K}\end{array}\end{array}\right]$$

The first row of the Jacobian matrix can be calculated by taking the partial derivatives of Eq. (33). On the same token, the second and third row can be calculated by taking partial derivatives of Eqs. (34) and (35).

$$J=\left[\begin{array}{ccc}1& {P}_{F}& {P}_{K}\\ 1& -\frac{{P}_{F}}{{S}_{F}}& 0\\ 1& 0& -\frac{{P}_{K}}{{S}_{K}}\end{array}\right]$$

(39)

$$\left[\begin{array}{c}\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\\ \frac{\partial K}{\partial \tau }\end{array}\right]= {-J}^{-1}\left[\begin{array}{c}\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\\ \frac{\partial {\mu }_{3}}{\partial \tau }\end{array}\right]={-J}^{-1}\left[\begin{array}{c}\begin{array}{c}\frac{{-P}_{F}F}{\tau }\\ -\frac{\rho }{1+\rho }(\frac{{P}_{F}}{{S}_{F}} ) \frac{F}{\tau }\end{array}\\ 0\end{array}\right]$$

(40)

Let, modify the CES function (41) to calculate the energy efficiency gain vector (38).

$${Y}_{t}=\gamma {e}^{{\lambda }_{t}}\{b {(\alpha {{K}_{t}}^{-\rho }+(1-\alpha ){{L}_{t}}^{-\rho })}^{\frac{\rho }{\rho 1}}+(1-b){({\tau }_{t}{F}_{t})}^{-\rho }{\}}^{- \frac{1}{ \rho }}$$

(41)

$$\mathrm{Let }U=b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{-\rho }$$

(42)

$$V=(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}})$$

(43)

$$\mathrm{S}=\tau F$$

(44)

$$U=b{V}^{{~}^{\rho }\!\left/ \!{~}_{\rho 1}\right.}+(1-b){S}^{-\rho }$$

(45)

Substitute (42), (43), (44), and (45) into above production function (41).

$$Y=A[{(U\left(V\left(\tau \right)\right)]}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}$$

(46)

Now, easily calculate the first element of energy efficiency gain vector.

Replace with Eq. (33).

$${\upmu }_{1}=\{Y-A[{\left(U\left(V\left(\tau \right)\right)\right]}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}\}$$

$$\frac{\partial f(k,L,\tau F)}{\partial \tau }=\frac{\partial y}{\partial U}\frac{\partial U}{\partial S}\frac{\partial S}{\partial \tau }$$

By taking the partial derivatives of Eqs. (46), (45), and (44) according to above requirements and simplifying it.

$$\begin{array}{c}\frac{\partial {\upmu }_{1}}{\partial \tau }=-\frac{{P}_{F}}{\tau }F\\ \frac{\partial {\upmu }_{1}}{\partial \tau }=-\frac{{S}_{F}}{\tau }Y\end{array}$$

(47)

Now move towards second Eq. (34) to obtain the second element of energy efficiency gain vector (38).

$${\upmu }_{2}=Y-\frac{{P}_{F}}{{S}_{F}}F=0$$

(48)

The above equation is solution of (29).

$$\begin{array}{c}P_F=\left(1-b\right)\tau^{-\rho}\left\lfloor\frac YF\right\rfloor^{1+\rho}\\\left\lceil\frac{P_F}{\left(1-b\right)\tau^{-\rho}}\right\rceil^\frac1{1+\rho}=\left\lfloor\frac YF\right\rfloor\end{array}$$

(49)

$$\begin{array}{c}Y=F{\lceil\frac{{P}_{F}}{\left(1-b\right){\tau }^{-\rho }}\rceil}^{\frac{1}{1+\rho }}\\ Y={\lceil\frac{{P}_{F}}{\left(1-b\right)}\rceil}^{\frac{1}{1+\rho }}{\tau }^{\frac{\rho }{1+\rho }} F\end{array}$$

(50)

By putting the value of (50) into (48)

$${\upmu }_{2}=Y-{\lceil\frac{{P}_{F}}{\left(1-b\right)}\rceil}^{\frac{1}{1+\rho }}{\tau }^{\frac{\rho }{1+\rho }} F=0$$

(51)

$$\begin{array}{c}\frac{\partial {\mu }_{2}}{\partial\uptau }=-\frac{\rho }{1+\rho }{\lceil\frac{{P}_{F}}{\left(1-b\right)}\rceil}^{\frac{1}{1+\rho }}{\frac{\left(\tau \right)}{\tau }}^{\frac{\rho }{1+\rho }} F\\ \frac{\partial {\mu }_{2}}{\partial\uptau }=-\frac{\rho }{1+\rho }{\lceil\frac{{P}_{F}}{\left(1-b\right) {\tau }^{-\rho }}\rceil}^{\frac{1}{1+\rho }} \frac{F}{\tau }\end{array}$$

(52)

From Eq. (36),

$$\begin{array}{cc}{P}_{F}(F/Y)={\mathrm{S}}_{\mathrm{F}}& (Y/F)= \frac{{P}_{F}}{{S}_{F}}\end{array}$$

(53)

So, Eq. (49) can be written as follows.

$${\lceil\frac{{P}_{F}}{\left(1-b\right){\tau }^{-\rho }}\rceil}^{\frac{1}{1+\rho }}=\frac{{P}_{F}}{{S}_{F}}$$

Therefore, Eq. (52) can be modified in following way.

$$\frac{\partial {\mu }_{2}}{\partial\uptau }=-\frac{\rho }{1+\rho }(\frac{{P}_{F}}{{S}_{F}} ) \frac{F}{\tau }$$

(54)

Put the value of S_F from (36).

$$\begin{array}{c}\frac{\partial {\mu }_{2}}{\partial\uptau }=-\frac{\rho }{1+\rho }\left(\frac{{P}_{F}}{{S}_{F}} \right)\frac{F}{\tau }=-\frac{\rho }{1+\rho }\left(\frac{{P}_{F}}{{P}_{F}}(Y/F) \right)\frac{F}{\tau }\\ \frac{\partial \mu 2}{\partial \tau }=-\frac{\rho }{1+\rho }\frac{Y}{\tau }\end{array}$$

(55)

Now turn to Eq. (35) for third element of energy efficiency gain vector. Again, modify the CES function like in Eq. (46) for simplicity purpose only.

$$\begin{array}{c}Y=A\{b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{-\rho }{\}}^{\frac{-1}{\rho }}\\ \mathrm{Let }U=b(\alpha {K}^{-{\rho }_{1}}+(1-\alpha ){L}^{-{\rho }_{1}}{)}^{\frac{\rho }{{\rho }_{1}}}+(1-b)(\tau F{)}^{-\rho }\end{array}$$

(56)

$$V=\left(\alpha {K}^{-{\rho }_{1}}+\left(1-\alpha \right){L}^{-{\rho }_{1}}\right)\mathrm{and S}=\tau F$$

(57)

$$U=b{V}^{{~}^{\rho }\!\left/ \!{~}_{\rho 1}\right.}+(1-b){S}^{-\rho }$$

(58)

$$Y=A[{(U\left(V\left(K\right)\right)]}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}$$

(59)

$$\frac{\partial f(k,L,\tau F)}{\partial K}=\frac{\partial Y}{\partial U}\frac{\partial U}{\partial V}\frac{\partial V}{\partial K}$$

(60)

Taking partial derivatives of (59), (58), and (57).

$$\frac{\partial Y}{\partial U}= -\frac{1}{\rho }A[{\left(U\left(V\left(K\right)\right)\right]}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.-1}\mathrm{V}(\mathrm{K})$$

(61)

$$= -\frac{1}{\rho }A[\frac{{U}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}}{U}]$$

(62)

From production function and Eq. (56).

$$\begin{array}{c}U=\frac{{Y}^{-\rho }}{A}\\ {U}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}=({\frac{{Y}^{-\rho }}{A})}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}\\ \begin{array}{c}\frac{U}{U}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}=\frac{({\frac{1}{A})}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}Y}{\frac{{Y}^{-\rho }}{A}}\\ \frac{U}{U}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}={({\frac{1}{A})}^{{~}_{-1}\!\left/ \!{~}_{\rho }\right.}AY}^{1+\rho }\\ \frac{U}{U}^{{~}^{-1}\!\left/ \!{~}_{\rho }\right.}={({\frac{1}{A})}^{{~}^{1-\rho }\!\left/ \!{~}_{\rho }\right.}Y}^{1+\rho }\end{array}\end{array}$$

(63)

Put the value of (63) into (62)

$$\frac{\partial Y}{\partial U}= -\frac{1}{\rho }{(A)}^{{~}^{1}\!\left/ \!{~}_{\rho }\right.}{Y}^{1+\rho }$$

(64)

$$\frac{\partial U}{\partial V}= b\frac{\rho }{{\rho }_{1}}\frac{V}{V}^{{~}^{\rho }\!\left/ \!{~}_{{\rho }_{1}}\right.}$$

(65)

$$\frac{\partial V}{\partial K}= -\mathrm{\alpha }{\rho }_{1}\frac{K}{K}^{-\rho 1}$$

(66)

Combing (64), (65), and (66) to get the value of (50).

$$\begin{array}{c}\frac{\partial f(k,L,\tau F)}{\partial K}=(-\frac{1}{\rho }{\left(A\right)}^{{~}^{1}\!\left/ \!{~}_{\rho }\right.}{Y}^{1+\rho })(b\frac{\rho }{{\rho }_{1}}\frac{V}{V}^{{~}^{\rho }\!\left/ \!{~}_{{\rho }_{1}}\right.})( -\left(\mathrm{\alpha }\right){\rho }_{1}\frac{K}{K}^{-\rho 1})\\ {P}_{K}= {\left(A\right)}^{{~}^{1}\!\left/ \!{~}_{\rho }\right.}b\left(\mathrm{\alpha }\right){V}^{\frac{\rho -{\rho }_{1}}{{\rho }_{1}}}{K}^{-{\rho }_{1}-1}{Y}^{1+\rho }\\ {P}_{K}= {\left(A\right)}^{{~}^{1}\!\left/ \!{~}_{\rho }\right.}b\left(\mathrm{\alpha }\right){V}^{\frac{\rho -{\rho }_{1}}{{\rho }_{1}}}\frac{Y}{K}\frac{{Y}^{\rho }}{{K}^{{\rho }_{1}}}\end{array}$$

(67)

Write the above equation in terms of Y.

$$Y=({P}_{K} \frac{{K}^{{\rho }_{1}}K}{{A}^{-\rho }b\mathrm{\alpha }{V}^{\frac{\rho -{\rho }_{1}}{{\rho }_{1}}}}{)}^{\frac{1}{\rho +1}}$$

(68)

Put the values of Eq. (67) and (68) into (33) and get,

$${\upmu }_{3}=k\left(Y, {\left(A\right)}^{{~}^{1}\!\left/ \!{~}_{\rho }\right.}b\left(\mathrm{\alpha }\right){V}^{\frac{\rho -{\rho }_{1}}{{\rho }_{1}}}\frac{Y}{K}\frac{{Y}^{\rho }}{{K}^{{\rho }_{1}}}\right)$$

(69)

$$\frac{\partial {\mu }_{3}}{\partial\uptau }=0$$

(70)

So, for study completes the elements of energy efficiency gain matrix (38) with values of (47), (55), and (70).

Study has the following matrix J (39), while for above Eq. (41) inverts the Jacobian matrix.

$$\begin{array}{c}\begin{array}{cc}J=\left[\begin{array}{ccc}1& {P}_{F}& {P}_{K}\\ 1& -\frac{{P}_{F}}{{S}_{F}}& 0\\ 1& 0& -\frac{{P}_{K}}{{S}_{K}}\end{array}\right]& {J}^{-1}=\frac{Adj (J)}{\left|J\right|}\end{array}\\ \left|J\right|=\Delta ={P}_{F}{P}_{K}\left(\frac{1+{S}_{F}+{S}_{K}}{{S}_{F}{S}_{K}}\right)\\ \begin{array}{c}Adj (J)=\left[\begin{array}{ccc}\frac{{P}_{F}{P}_{K}}{{S}_{F}{S}_{K}}& \frac{{P}_{F}{P}_{K}}{{S}_{K}}& \frac{{P}_{F}{P}_{K}}{{S}_{F}}\\ \frac{{P}_{K}}{{S}_{K}}& -\frac{{P}_{K}}{{S}_{K}}\left(1+{S}_{K}\right)& {P}_{K}\\ \frac{{P}_{F}}{{S}_{F}}& {P}_{F}& -\frac{{P}_{E}}{{S}_{E}}\left(1+{S}_{E}\right)\end{array}\right]\\ \begin{array}{cc}\left[\begin{array}{c}\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\\ \frac{\partial K}{\partial \tau }\end{array}\right]= {-J}^{-1}\left[\begin{array}{c}\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\\ \frac{\partial {\mu }_{3}}{\partial \tau }\end{array}\right]=-\frac{1}{\Delta }& \left[\begin{array}{ccc}\frac{{P}_{F}{P}_{K}}{{S}_{F}{S}_{K}}& \frac{{P}_{F}{P}_{K}}{{S}_{K}}& \frac{{P}_{F}{P}_{K}}{{S}_{F}}\\ \frac{{P}_{K}}{{S}_{K}}& -\frac{{P}_{K}}{{S}_{K}}\left(1+{S}_{K}\right)& {P}_{K}\\ \frac{{P}_{F}}{{S}_{F}}& {P}_{F}& -\frac{{P}_{E}}{{S}_{E}}\left(1+{S}_{E}\right)\end{array}\right]\left[\begin{array}{c}\begin{array}{c}\frac{{-P}_{F}F}{\tau }\\ -\frac{\rho }{1+\rho }(\frac{{P}_{F}}{{S}_{F}} ) \frac{F}{\tau }\end{array}\\ 0\end{array}\right]\end{array}\end{array}\end{array}$$

(71)

Now find the impact of energy efficiency on output, total energy consumption, and capital from Eq. (71). In next step is to simplify the following equation for the long-run energy rebound effect.

$${\eta }^{F}=\frac{\tau }{F}\frac{\partial F}{\partial \tau }=\frac{{S}_{F}+ \rho ({S}_{F}-{S}_{K}-1)}{{(1+{S}_{K}+S}_{F})(1+\rho )}$$

(72)

$RE=1+\eta^F=1+\frac{S_F+\rho\left(S_F-S_K-1\right)}{\left(1+S_K+S_F\right)\left(1+\rho\right)}$ (See 09 and 10)

$$\begin{array}{c}=\frac{\left(1+S_K+S_F\right)\left(1+\rho\right)+S_F+\rho\left(S_F-S_K-1\right)}{\left(1+S_K+S_F\right)\left(1+\rho\right)}\\RE=\frac{\left(1+S_K+S_F\right)+S_F\left(1+2\rho\right)}{\left(1+S_K+S_F\right)\left(1+\rho\right)}\end{array}$$

(73)

Long-run energy rebound effect is determined through the shares of energy and capital (S_F, S_K) and parameter of elasticity of substitution between labor-capital and energy (ρ).

$$\begin{array}{c}\frac{\partial y}{\partial \tau }\frac{\tau }{Y}=\left(\frac{{S}_{F}}{{1+S}_{F}+{S}_{K}}\right)\left(\frac{1+\rho +\rho }{1+\rho }\right)\\ {\eta }_{\tau }^{Output}=\frac{\partial y}{\partial \tau }\frac{\tau }{Y}=\left(\frac{{S}_{F}}{{1+S}_{F}+{S}_{K}}\right)\left(\frac{1+2\rho }{1+\rho }\right)\end{array}$$

(74)

Now derive energy intensity effect from above Eqs. (73) and (74).

$$\begin{array}{c}{\eta }_{\tau }^{Intensity}=\frac{\partial F}{\partial \tau }\frac{\tau }{F}- \frac{\partial y}{\partial \tau }\frac{\tau }{Y}\\ {\eta }_{\tau }^{Intensity}=\left(\frac{1}{1+\rho }\right)\end{array}$$

(75)

Short-run analysis

So, our concern equations are (33) and (34).

$$\left[\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\right]= -{J}^{-1}\left[\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\right]$$

$$\left[\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\right]= -{J}^{-1}\left[\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\right]=-{J}^{-1}\left[\begin{array}{c}\frac{{-S}_{F}Y}{\tau }\\ -\frac{\rho }{1+\rho }\frac{Y}{\tau }\end{array}\right]=-{J}^{-1}\left[\begin{array}{c}\frac{{-P}_{F}F}{\tau }\\ -\frac{\rho }{1+\rho }\left(\frac{{P}_{F}}{{S}_{F}} \right)\frac{F}{\tau }\end{array}\right]$$

Finally, move towards inverting the Jacobian matrix.

$$J=\left[\begin{array}{cc}1& {P}_{F}\\ 1& -\frac{{P}_{F}}{{S}_{F}}\end{array}\right]$$

The determinant of Jacobian matrix is

$$\begin{array}{c}\Delta =-\frac{{P}_{F}}{{S}_{F}}-{P}_{F}\\ \Delta ={-P}_{F}\left(\frac{1+{S}_{F}}{{S}_{F}}\right)\\ \begin{array}{c}\left[\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\right]= -{J}^{-1}\left[\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\right]=-\frac{1}{\Delta }\left[\begin{array}{cc}-\frac{{P}_{F}}{{S}_{F}}& -{P}_{F}\\ -1& 1\end{array}\right]\left[\begin{array}{c}\frac{{-S}_{F}Y}{\tau }\\ -\frac{\rho }{1+\rho }\frac{Y}{\tau }\end{array}\right]\\ \frac{\partial y}{\partial \tau }=\frac{1}{{P}_{F}}\left(\frac{{S}_{F}}{{1+S}_{F}}\right)\left(\frac{{P}_{F}Y}{\tau }+{P}_{F}\frac{\rho }{1+\rho }\frac{Y}{\tau }\right) \\ \begin{array}{c}\frac{\partial y}{\partial \tau }=\left(\frac{{S}_{F}}{{1+S}_{F}}\right)\left(\frac{Y}{\tau }+\frac{\rho }{1+\rho }\frac{Y}{\tau }\right)\\ \frac{\partial y}{\partial \tau }=\left(\frac{{S}_{F}}{{1+S}_{F}}\right)\left(1+\frac{\rho }{1+\rho }\right) \frac{Y}{\tau }\\ {\eta }_{\tau }^{Output}=\frac{\partial y}{\partial \tau }\frac{\tau }{Y}=\left(\frac{{S}_{F}}{{1+S}_{F}}\right)\left(\frac{1+2\rho }{1+\rho }\right)\end{array}\end{array}\end{array}$$

(76)

$$\begin{array}{c}\left[\begin{array}{c}\frac{\partial y}{\partial \tau }\\ \frac{\partial F}{\partial \tau }\end{array}\right]= -{J}^{-1}\left[\begin{array}{c}\frac{\partial {\mu }_{1}}{\partial \tau }\\ \frac{\partial {\mu }_{2}}{\partial \tau }\end{array}\right]=\frac{-1}{\Delta }\left[\begin{array}{cc}-\frac{{P}_{F}}{{S}_{F}}& -{P}_{F}\\ -1& 1\end{array}\right]\left[\begin{array}{c}\frac{{-P}_{F}F}{\tau }\\ -\frac{\rho }{1+\rho }\frac{{P}_{F}}{{S}_{F}}\frac{F}{\tau }\end{array}\right]\\ \frac{\partial F}{\partial \tau }=\frac{1}{{P}_{F}}\left(\frac{{S}_{F}}{{1+S}_{F}}\right)\left(\frac{{P}_{F}F}{\tau }-\frac{\rho }{1+\rho }\frac{{P}_{F}}{{S}_{F}}\frac{F}{\tau }\right)\\ \begin{array}{c}\frac{\partial F}{\partial \tau }=\frac{{S}_{F}}{{1+S}_{F}}\frac{F}{\tau }-\frac{1}{{1+S}_{F}}\frac{\rho }{1+\rho }\frac{F}{\tau }\\ \frac{\tau }{F}\frac{\partial F}{\partial \tau }=\left(\frac{{S}_{F}}{{1+S}_{F}}-\frac{\rho }{1+\rho }\frac{1}{{1+S}_{F}}\right)\\ \begin{array}{c}\frac{\tau }{F}\frac{\partial F}{\partial \tau }=\frac{1}{{1+S}_{F}}\left(\frac{{S}_{F}+\rho ({S}_{F}-1)}{1+\rho }\right)\\ \frac{\tau }{F}\frac{\partial F}{\partial \tau }=\frac{{S}_{F}+\rho ({S}_{F}-1)}{{(1+S}_{F})(1+\rho )}\end{array}\end{array}\end{array}$$

(77)

The above Eq. (77) is the key part of short-run energy rebound effect.

$$\begin{array}{c}R=\left(\tau/F\right)\left(\partial F/\partial\tau\right)+1\\R=\frac{1+2S_F\left(1+\rho\right)}{\left(1+S_F\right)\left(1+\rho\right)}\end{array}$$

(78)

The above equation is short-run energy rebound effect. Now, get the energy intensity effect for the short run with the help of Eqs. (77) and (78).

$$\begin{array}{c}{\eta }_{\tau }^{Intensity}=\frac{\partial F}{\partial \tau }\frac{\tau }{F}- \frac{\partial y}{\partial \tau }\frac{\tau }{Y}\\ {\eta }_{\tau }^{Intensity}=\left(\frac{1}{1+\rho }\right)\end{array}$$

(79)

Above three (77, 78, and 79) equations are energy rebound, intensity, and output effect for the short run.