Downlink Performance Analysis in D2D-Enabled Cellular Networks with Clustered Users

Abstract

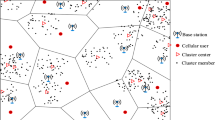
This paper studies the performance of device-to-device (D2D)-enabled cellular network with D2D users reusing downlink resources of cellular links. The cellular users and macro BSs are modeled as an independent Poisson point process (PPP), while the locations of D2D users are modeled as a Thomas cluster process. We analyze the performance of this D2D network for two cases: (1) the serving devices is selected uniformly at random from the same cluster, and (2) the serving devices is the closest devices to a typical device in the same cluster. We derive the theoretical expressions for the coverage probability, area spectral efficiency (ASE) and average rate of the whole network using stochastic geometry and analyze the performance trends with the number of simultaneously active D2D links per cluster, which shows that the closest choice case is better than the uniform choice case. The optimal number of simultaneously active D2D links per cluster can be obtained by analyzing ASE.

Appendices

Appendix

Proof of (10)

Laplace transform of the interference at the typical D2D user from intra-cluster interfering D2D users, $L_{{I_{{{\text{d2d}}}}^{{{\text{intra}}}} }} \left( {s|v_{0} } \right)$ is

$$\begin{gathered} {\text{ E}}_{{I_{{{\text{d2d}}}}^{{{\text{intra}}}} }} \left[ {\exp \left( { - sI_{{{\text{d2d}}}}^{{{\text{intra}}}} } \right)} \right] \hfill \\ = {\text{E}}\left[ {\exp \left( { - s\sum\limits_{{y \in A^{{x_{0} }} \backslash y_{0} }} {P_{d} h\left\| {x_{0} + y} \right\|^{ - \alpha } } } \right)} \right] \hfill \\ = {\text{E}}_{{A^{{x_{0} }} }} \left[ {\prod\limits_{{y \in A^{{x_{0} }} \backslash y_{0} }} {\left[ {{\text{E}}_{h} \left[ {\exp \left( { - sP_{d} h\left\| {x_{0} + y} \right\|^{ - \alpha } } \right)} \right]} \right]} } \right] \hfill \\ \mathop = \limits^{\left( a \right)} {\text{E}}_{{A^{{x_{0} }} }} \left[ {\prod\limits_{{y \in A^{{x_{0} }} \backslash y_{0} }} {\int_{0}^{\infty } {\exp \left( { - sP_{d} h\left\| {x_{0} + y} \right\|^{ - \alpha } } \right)\mu e^{ - \mu h} } } dh} \right] \hfill \\ = {\text{E}}_{{A^{{x_{0} }} }} \left[ {\prod\limits_{{y \in A^{{x_{0} }} \backslash y_{0} }} {E_{Y} \left[ {\frac{\mu }{{\mu + sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}} \right]} } \right] \hfill \\ \end{gathered}$$

$$\begin{gathered} \mathop = \limits^{\left( b \right)} \sum\limits_{k = 0}^{M - 1} {\left( {\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right)}^{k} P\left( {K = k|K < M - 1} \right) \hfill \\ = \sum\limits_{k = 0}^{M - 1} {\left( {\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right)}^{k} \frac{{\left( {m - 1} \right)^{k} }}{k!}\frac{{e^{{ - \left( {m - 1} \right)}} }}{\xi },\xi = \sum\limits_{j = 0}^{M - 1} {\frac{{\left( {m - 1} \right)^{j} e^{{ - \left( {m - 1} \right)}} }}{j!}} \hfill \\ \mathop = \limits^{\left( c \right)} \exp \left( {\left( {m - 1} \right)\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} + \left( { - \left( {m - 1} \right)} \right)} \right) \hfill \\ = \exp \left( { - \left( {m - 1} \right)\int\limits_{{R^{2} }} {\frac{{sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}{{\mu + sp_{d} \left\| {x_{0} + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right) \hfill \\ \mathop = \limits^{\left( d \right)} \exp \left( { - \left( {m - 1} \right)\int_{0}^{\infty } {\frac{{sp_{d} r_{d1}^{ - \alpha } }}{{\mu + sp_{d} r_{d1}^{ - \alpha } }}f_{{R_{d1} }} \left( {r_{d1} /v_{0} } \right)dr_{d1} } } \right) \hfill \\ \end{gathered}$$

where (a) represents the expectation of h∼exp(μ) and (b) represents the expectation of the number of interfering devices in each cluster, (c) under the assumption $M > > m$, the formula is simplified based on the Taylor formula, and (d) follows from the change of variable $\left\| {x_{0} + y} \right\| = r_{d1}$.

Proof of (12)

Laplace transform of the interference at the typical D2D user from inter-cluster interfering D2D users, $L_{{I_{{{\text{d2d}}}}^{{{\text{inter}}}} }} \left( s \right)$ is

$$\begin{gathered} {\text{ E}}_{{I_{{{\text{d2d}}}}^{{{\text{inter}}}} }} \left[ {\exp \left( { - sI_{{{\text{d2d}}}}^{{{\text{inter}}}} } \right)} \right] \hfill \\ = {\text{E}}\left[ {\exp \left( { - s\sum\limits_{{x \in \Phi_{C} }} {\sum\limits_{{y \in A^{X} }} {P_{d} h\left\| {x + y} \right\|^{ - \alpha } } } } \right)} \right] \hfill \\ = {\text{E}}_{{\Phi_{C} }} \left[ {\prod\limits_{{x \in \Phi_{C} }} {E_{{A^{x} }} \left[ {\prod\limits_{{y \in A^{X} }} {\left[ {E_{h} \left[ {\exp \left( { - sP_{d} h\left\| {x + y} \right\|^{ - \alpha } } \right)} \right]} \right]} } \right]} } \right] \hfill \\ \mathop = \limits^{\left( a \right)} {\text{E}}_{{\Phi_{C} }} \left[ {\prod\limits_{{x \in \Phi_{C} }} {E_{{A^{x} }} \left[ {\prod\limits_{{y \in A^{X} }} {E_{Y} \left[ {\frac{\mu }{{\mu + sp_{d} \left\| {x + y} \right\|^{ - \alpha } }}} \right]} } \right]} } \right] \hfill \\ = {\text{E}}_{{\Phi_{C} }} \left[ {\prod\limits_{{x \in \Phi_{C} }} {E_{{A^{x} }} \left[ {\prod\limits_{{y \in A^{X} }} {\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } } \right]} } \right] \hfill \\ \mathop = \limits^{\left( b \right)} {\text{E}}_{{\Phi_{C} }} \left[ {\prod\limits_{{x \in \Phi_{C} }} {\sum\limits_{k = 0}^{M} {\left( {\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right)}^{k} P\left( {K = k|K < M} \right)} } \right] \hfill \\ \end{gathered}$$

$$\begin{gathered} \mathop = \limits^{\left( c \right)} \exp \left( { - \lambda_{c} \int\limits_{{R^{2} }} {\left( {1 - \sum\limits_{k = 0}^{M} {\left( {\int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right)}^{k} \frac{{m^{k} }}{k!}\frac{{e^{ - m} }}{{\sum\limits_{j = 0}^{M} {\frac{{m^{j} e^{ - m} }}{j!}} }}} \right) \cdot 2\pi vdv} } \right) \\ \mathop = \limits^{\left( d \right)} \exp \left( { - 2\pi \lambda_{c} \int_{0}^{\infty } {1 - \exp \left( { - m\left( {1 - \int\limits_{{R^{2} }} {\frac{\mu }{{\mu + sp_{d} \left\| {x + y} \right\|^{ - \alpha } }}f_{Y} \left( y \right)dy} } \right)} \right) \cdot vdv} } \right) \\ \mathop = \limits^{\left( e \right)} \exp \left( { - 2\pi \lambda_{c} \int_{0}^{\infty } {1 - \exp \left( { - m\left( {1 - \int_{0}^{\infty } {\frac{\mu }{{\mu + sp_{d} r_{d2}^{ - \alpha } }}f_{{R_{d2} }} \left( {r_{d2} |v} \right)dr_{d2} } } \right)} \right) \cdot vdv} } \right) \\ \mathop \ge \limits^{\left( f \right)} \exp \left( { - 2\pi \lambda_{c} \int_{0}^{\infty } {m\int_{0}^{\infty } {\frac{{sp_{d} r_{d2}^{ - \alpha } }}{{\mu + sp_{d} r_{d2}^{ - \alpha } }} \cdot f_{{R_{d2} }} \left( {r_{d2} |v} \right) \cdot vdr_{d2} } dv} } \right) \\ \mathop = \limits^{\left( g \right)} \exp \left( { - 2\pi \lambda_{c} m\int_{0}^{\infty } {\frac{{sp_{d} r_{d2}^{ - \alpha } }}{{\mu + sp_{d} r_{d2}^{ - \alpha } }} \cdot r_{d2} dr_{d2} } } \right) \\ = \exp \left( { - \pi \lambda_{c} m\left( {sp_{d} } \right)^{{\frac{2}{\alpha }}} \cdot \frac{2\pi /\alpha }{{\sin \left( {2\pi /\alpha } \right)}}} \right) \\ \end{gathered}$$

where (a) represents the expectation of h∼exp(μ) and (b) represents the expectation of the number of interfering devices in each cluster, (c) follows from the probability generating functional (PGFL) of PPP [14], (d) under the assumption $M > > m$, the formula is simplified based on the Taylor formula, (e) follows from the change of variable $\left\| {x + y} \right\| = r_{d2}$, (f) according to Taylor, the approximate $1{\text{ - exp}}\left( { - ax} \right) \le a$ simplification formula is developed, and (g) follows by converting from Cartesian to polar coordinates where $\int_{0}^{\infty } {\frac{1}{{1 + su^{ - \alpha } }}} f_{U} (u|v)du$.

Proof of (21)

Average rate of a typical D2D user for the uniform choice case, $R_{{}}^{{\left( d \right)\left( {{\text{uniform}}} \right)}}$ is

$$\begin{gathered} E\left[ {\ln \left( {1 + SIR} \right)} \right] \\ = \int_{0}^{\infty } P \left[ {\ln \left( {1 + SIR} \right) > t} \right]dt \\ = \int_{0}^{\infty } {\int_{0}^{\infty } P \left[ {\ln \left( {1 + \frac{{P_{d} hr_{d}^{ - \alpha } }}{{I_{{{\text{b2d}}}} + I_{{{\text{d2d}}}}^{{{\text{inter}}}} + I_{{{\text{d2d}}}}^{{{\text{intra}}}} }}} \right) > t|v_{0} } \right]f_{{R_{d} }} \left( {r_{d} |v_{0} } \right)dr_{d} dt} \\ = \int_{0}^{\infty } {\int_{0}^{\infty } P \left[ {h > \left( {e^{t} - 1} \right)\left( {I_{{{\text{b2d}}}} + I_{{{\text{d2d}}}}^{{{\text{inter}}}} + I_{{{\text{d2d}}}}^{{{\text{intra}}}} } \right)P_{d}^{ - 1} r_{d}^{\alpha } |v_{0} } \right]f_{{R_{d} }} \left( {r_{d} |v_{0} } \right)dr_{d} dt} \\ \mathop = \limits^{\left( a \right)} \int_{0}^{\infty } {\int_{0}^{\infty } {\int_{0}^{\infty } {E_{I} \left[ {e^{{ - \mu \left( {e^{t} - 1} \right)P_{d}^{ - 1} r_{d}^{\alpha } \left( {I_{{{\text{b2d}}}} + I_{{{\text{d2d}}}}^{{{\text{inter}}}} + I_{{{\text{d2d}}}}^{{{\text{intra}}}} } \right)}} } \right]f_{{R_{d} }} \left( {r_{d} |v_{0} } \right)f_{{V_{0} }} \left( {v_{0} } \right)dr_{d} dv_{0} dt} } } \\ \mathop = \limits^{\left( b \right)} \int_{0}^{\infty } {\int_{0}^{\infty } {\int_{0}^{\infty } {L_{{I_{{{\text{b2d}}}} }} \left( s \right)L_{{I_{{{\text{d2d}}}}^{{{\text{inter}}}} }} \left( s \right)L_{{I_{{{\text{d2d}}}}^{{{\text{intra}}}} }} \left( {s|v_{0} } \right)f_{{R_{d} }} \left( {r_{d} |v_{0} } \right)f_{{V_{0} }} \left( {v_{0} } \right)} } } dr_{d} dv_{0} dt \\ \end{gathered}$$

where (a) represents the expectation of h∼exp(μ) and (b) follows from the change of variable $s = \mu \left( {e^{t} - 1} \right) \, r_{c}^{\alpha } P_{d}^{ - 1}$.