A User Grouping Based Opportunistic Network Coding Scheme for Multi-user Multi-relay Uplink Transmissions

Abstract

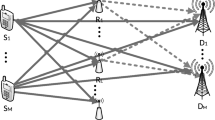
In this paper, we study a User Grouping based Opportunistic Network Coding (UG-ONC) scheme for multi-user multi-relay uplink transmission scenario. In traditional opportunistic network coding (ONC) schemes, the use of network coding is often determined by the state of relays, i.e., buffer, packets waiting time, packets’ successful decoding. However, whether the use of network coding is beneficial to the users is not considered. In our proposed UG-ONC scheme, whether network coding is adopted is not only determined by the state of relays, but also by the link quality between users and base station, that is, determined by the state of users. We consider two information-theoretic metrics, i.e., outage probability and throughput, to evaluate the validity and reliability of the UG-ONC scheme. And the approximate expressions of outage probability and throughput are derived. To further examine the performance of the scheme, we compare UG-ONC scheme with an existent network coding scheme. Monte Carlo simulations are presented to validate our analysis. The results show that our proposed UG-ONC scheme achieves considerable gains over the traditional network coding scheme. Our proposed UG-ONC scheme gives a new thought for designing opportunistic network coding.

Appendices

Appendix 1

Proof of Theorem 1

Recall from (23), we could denote the expression $\Pr \left( x_1 \ge \frac{2^{r}-1}{\rho },\ldots , x_K \ge \frac{2^{r}-1}{\rho }\right)$ on the right hand side as $\Pr \left( \sum \nolimits _{i\in S} x_i\ge \frac{2^{\Vert S\Vert r}-1}{\rho }, \ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \ \Vert S\Vert =1 \right)$, where $\Vert S\Vert$ denotes the number of element in S. We can prove it by using mathematical induction. We first prove that

$$ \begin{aligned}&\Pr \left( \sum \limits _{k\in S} x_k\ge \frac{2^{|S|r}-1}{\rho }, \ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert =1 \right) \\&\quad \approx \Pr \left( \sum \limits _{k\in S} x_k\ge \frac{2^{|S|r}-1}{\rho }, \ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert =1,2 \right) \end{aligned} $$

(37)

When $\Vert S\Vert =1$, there are K inequalities, and when $\Vert S\Vert =1,2$, the total number of inequalities is $K+\left( {\begin{array}{c}K\\ 2\\ \end{array}} \right) , \left( {\begin{array}{c}K\\ 2\\ \end{array}} \right)$ more inequalities than the probability expression when $|S|=1$. And these $\left( {\begin{array}{c}K\\ 2\\ \end{array}} \right)$ inequalities responds to the inequalities that the sum of arbitrary two norm should be larger than $\frac{2^{2r}-1}{\rho }$. Thus, we could artificially construct $\left( {\begin{array}{c}K\\ 2\\ \end{array}} \right) -1$ probability expression between $\Vert S\Vert =1$ and $\Vert S\Vert =2$ with different numbers of inequalities when $\Vert S\Vert =2$. Thus, (37) can be proved by proving that these $\left( {\begin{array}{c}K\\ 2\\ \end{array}} \right) +1$ are all approximately equal, on the base of including all K inequalities when $|S|=1$. We give demonstration below that

$$ \begin{aligned}&\Pr \left( \underbrace{x_1\ge \frac{2^{r}-1}{\rho },\ldots , x_K\ge \frac{2^{r}-1}{\rho }}_{\buildrel \varDelta \over ={\mathcal {D}}_1}\right) \approx \Pr \left( {\mathcal {D}}_1, x_1+x_2\ge \frac{2^{2r}-1}{\rho }\right) \end{aligned}$$

(38)

${\mathcal {D}}_1$ is used here for concision, whose subscript refers to the value of $\Vert S\Vert$. The difference between the two probabilities can be shown as

$$ \begin{aligned} \Delta _1&=\Pr \left( {\mathcal {D}}_1\right) -\Pr \left( {\mathcal {D}}_1, x_1+x_2\ge \frac{2^{2r}-1}{\rho }\right) \\&=\Pr \left( {\mathcal {D}}_1, x_1+x_2<\frac{2^{2r}-1}{\rho }\right) \\&<\Pr \left( x_1\ge \frac{2^{r}-1}{\rho }, x_2\ge \frac{2^{r}-1}{\rho }, x_1+x_2<\frac{2^{2r}-1}{\rho }\right) \\&=\int _{\frac{2^{r}-1}{\rho }}^{\frac{2^{2r}-2^r}{\rho }} \left( \int _{\frac{2^{r}-1}{\rho }}^{\frac{2^{2r}-1}{\rho }-x_1} \frac{1}{\lambda }e^{-\frac{x_2}{\lambda }}dx_2 \right) \frac{1}{\lambda }e^{-\frac{x_1}{\lambda }}dx_1\\&=e^{-\frac{2^{2r}-1}{\lambda \rho }}\left( e^{\frac{(2^r-1)^2}{\lambda \rho }}-1-\frac{(2^r-1)^2}{ \lambda \rho }\right) \end{aligned}$$

(39)

When $\rho \rightarrow \infty , e^{\frac{(2^r-1)^2}{\lambda \rho }}\approx 1+\frac{(2^r-1)^2}{\lambda \rho }+\frac{(2^r-1)^4}{2\lambda ^2\rho ^2}$. Thus it’s obviously that $\varDelta _1$ is decreasing to zero at high SNR. In the same way, it can be proved that

$$ \begin{aligned}&\Pr \left( {\mathcal {D}}_1, x_1+x_2\ge \frac{2^{2r}-1}{\rho }\right) \\& \quad \approx \Pr \left( {\mathcal {D}}_1, x_1+x_2\ge \frac{2^{2r}-1}{\rho },x_1+x_3\ge \frac{2^{2r}-1}{\rho }\right) \\& \quad \approx \Pr \left( {\mathcal {D}}_1,\sum \limits _{k\in S} x_k\ge \frac{2^{|S|r}-1}{\rho },\ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert =2 \right) \\& \quad = \Pr \left( \sum \limits _{k\in S} x_k\ge \frac{2^{\Vert S\Vert r}-1}{\rho }, \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert =1,2 \right) \end{aligned}$$

(40)

In this way, (37) can been proved. We then prove that

$$ \begin{aligned}&\Pr \left( \underbrace{\sum \nolimits _{k\in S} x_k\ge \frac{2^{\Vert S\Vert r}-1}{\rho },\ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert \le q-1}_{\buildrel \varDelta \over ={\mathcal {D}}_{q-1}} \right) \\&\quad \approx \Pr \left( \sum \limits _{k\in S} x_k\ge \frac{2^{|S|r}-1}{\rho },\ \forall \ S\subseteq \llbracket {{\mathcal {T}}_2} \rrbracket , \Vert S\Vert \le q \right) \end{aligned}$$

(41)

Just like the way we prove (37). We could prove it by proving that the probability of inequalities with different number of inequalities when $\Vert S\Vert =q$ are all approximately equal. This is equivalent to prove that

$$ \begin{aligned}&\Pr \left( {\mathcal {D}}_{q-1} \right) \approx \Pr \left( {\mathcal {D}}_{q-1}, \ \sum _{i=1}^{q} x_i\ge \frac{2^{qr}-1}{\rho } \right) \end{aligned}$$

(42)

The difference of the two probabilities can be shown as

$$ \begin{aligned}&\Delta _{q-1}=\Pr \left( {\mathcal {D}}_{q-1} \right) -\Pr \left( {\mathcal {D}}_{q-1}, \sum _{i=1}^{q} x_i\ge \frac{2^{qr}-1}{\rho } \right) \\&=\Pr \left( {\mathcal {D}}_{q-1}, \sum _{i=1}^{q} x_i< \frac{2^{(q)r}-1}{\rho } \right) \\&<\Pr \left( x_1>\frac{2^r-1}{\rho },{\sum _{i=2}^{q} x_i}\ge \frac{2^{(q-1)r}-1}{\rho }\sum _{i=1}^{q} x_i< \frac{2^{qr}-1}{\rho } \right) \\&=\Pr \left( x_1>\frac{2^r-1}{\rho },x_1+y\ge \frac{2^{(q-1)r}-1}{\rho }, x_1+y<\frac{2^{qr}-1}{\rho } \right) \\&=\int _{\frac{{2^r-1}}{\rho }}^{\frac{{2^{qr}-2^{\left( {q-1}\right) r}}}{\rho }} \left[ {\int _{\frac{{2^{\left( {q-1} \right) r}-1}}{\rho }}^{\frac{{2^{qr}-1}}{\rho }-x_1}{d\left( {1- \sum \limits _{i=0}^{q-2}{\frac{{\left( {\frac{y}{\lambda }}\right) ^i}}{{i!}}e^{-\frac{y}{\lambda }}}}\right) }}\right] de^{-\frac{{x_1}}{\lambda }}\\&=\sum \limits _{i = 0}^{q - 2} {\frac{{\left( {\frac{{2^{\left( {q - 1} \right) r} - 1}}{{\lambda \rho }}} \right) ^i }}{{i!}}e^{ - \frac{{2^{\left( {q - 1} \right) r} - 1}}{{\rho \lambda }}} } \cdot \left( {e^{ - \frac{{2^r - 1}}{{\lambda \rho }}} - e^{ - \frac{{\left( {2^r - 1} \right) 2^{\left( {q - 1} \right) r} }}{{\lambda \rho }}} } \right) \\&\quad +e^{ - \frac{{2^{qr} - 1}}{{\lambda \rho }}} \sum \limits _{i = 0}^{q - 2} {\left( {\frac{{\left( {\frac{{2^{\left( {q - 1} \right) r} - 1}}{{\lambda \rho }}} \right) ^{i + 1} }}{{\left( {i + 1} \right) !}} - \frac{{\left( {\frac{{2^{qr} - 2^r }}{{\lambda \rho }}} \right) ^{i + 1} }}{{\left( {i + 1} \right) !}}} \right) } \end{aligned}$$

(43)

It is obviously that when $\rho \rightarrow \infty , \varDelta _{q-1}=0$. Thus, (42) is proved. As a result, the theorem is proved. $\square$

Appendix 2

Proof of Theorem 2

To minimize the outage probability, it is equivalent to maximize the probability of ${\mathcal {Q}}$.

According to (18), we treat all packets as unknown except for arbitrary ones, and get the mutual information between users that own these packets and the base station. Different from traditional multiple access channel that all users send pure packets in the same time slot, there are K mixture of packets received in K time slots at the base station, like MIMO [25]. These mixtures are weighted and summed. For arbitrary packet set ${\mathcal {A}}_*$ when $|{\mathcal {A}}_*|=i$, the combined SNR can be given as below

$$ \begin{aligned} SNR_{{\mathcal {A}}_*}=\frac{ \sum \nolimits _{ j\in \llbracket {\mathcal {A}}_*\rrbracket } \left( {\sum \nolimits _{n=1}^{K} {\gamma _{{\hat{R}}_nj}h_{{\hat{R}}_nD}a_{n i}}}\right) ^2}{\sum \nolimits _{n=1}^K{a_{ni}}^2} \end{aligned}$$

(44)

where $\llbracket {\mathcal {A}}_*\rrbracket$ denotes the number set of subscript of packets in ${\mathcal {A}}_*$ and $a_{ni}$ denotes the combination coefficient of mixture from the relay ${\hat{R}}_n$ when all except i packets are treated unknown. Applying MRC [25], $SNR_{{\mathcal {A}}_*}$ achieves its maximum when

$$\begin{aligned} a_{ni}=h_{{\hat{R}}_nD} \end{aligned}$$

(45)

When $|{\mathcal {A}}_*|=i$, there are $\left( {\begin{array}{c} K\\ i \end{array}}\right)$ candidate sets for ${\mathcal {A}}_*$. We denote the set that contains all candidate sets as ${\mathcal {A}}_*^i$. Applying (6), (44) and (45) we have

$$ \begin{aligned}&\sum \limits _{{A}_*\in {\mathcal {A}}_*^i} SNR_{{\mathcal {A}}_*}\\&=\sum \limits _{{A}_*\in {\mathcal {A}}_*^i}\frac{ \sum \nolimits _{ j\in \llbracket {\mathcal {A}}_*\rrbracket } \left( {\sum \nolimits _{n=1}^{K} {\gamma _{{\hat{R}}_nj} |h_{{\hat{R}}_nD}|^2}}\right) ^2}{\sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^2}\\&= \left( {\begin{array}{c} K-1\\ i-1 \end{array}}\right) \frac{ \sum \nolimits _{j=1}^K \left( {\sum \nolimits _{n=1}^{K} {\gamma _{{\hat{R}}_nj} |h_{{\hat{R}}_nD}|^2}}\right) ^2}{\sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^2}\\&=\left( {\begin{array}{c} K-1\\ i-1 \end{array}}\right) \left[\mathop{\sum} \nolimits _{ \mathop{(p,q)}\limits_{{p,q} \in \llbracket {{\mathcal {T}}_2} \rrbracket}}\left( \sum \nolimits _{j=1}^K 2{\gamma _{{\hat{R}}_p,j}}{\gamma _{{\hat{R}}_q,j}}\right) \right. \\&\left. \qquad |h_{{\hat{R}}_pD}|^2|h_{{\hat{R}}_qD}|^2+\sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^4 \right] \Bigg / \sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^2\\&\le \left( {\begin{array}{c} K-1\\ i-1 \end{array}}\right) \left[\mathop{\sum} \nolimits _{ \mathop{(p,q)}\limits_{{p,q} \in \llbracket {{\mathcal {T}}_2} \rrbracket}} \sum \nolimits _{j=1}^K \left( {\gamma _{{\hat{R}}_p,j}}^2+{\gamma _{{\hat{R}}_q,j}}^2\right) \right. \\&\left. \qquad |h_{{\hat{R}}_pD}|^2|h_{{\hat{R}}_qD}|^2+\sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^4 \right] \Bigg / \sum \nolimits _{n=1}^K |h_{{\hat{R}}_nD}|^2\\&=\left( {\begin{array}{c} K-1\\ i-1 \end{array}}\right) \sum \limits _{n=1}^K |h_{{\hat{R}}_nD}|^2 \end{aligned}$$

(46)

When ${\gamma _{{\hat{R}}_p,n}}={\gamma _{{\hat{R}}_q,n}}$, the equality could be achieved. Accordingly,

$$\begin{aligned} \mathop {\arg \max }\limits _{{\mathcal {A}}_* \in {\mathcal {A}}_*^i}[{ SNR_{{\mathcal {A}}_*}}] \le \frac{i}{K} \sum \limits _{k=1}^K |h_{{\hat{R}}_kD}|^2 \end{aligned}$$

(47)

Recall from (21), we have

$$\begin{aligned} {\mathcal {Q}}_i\cong \left\{ \mathop {\arg \min }\limits _{{\mathcal {A}}_* \in {\mathcal {A}}_*^i} [{ SNR_{{\mathcal {A}}_*}}] \ge 2^{ir}-1 \right\} \end{aligned}$$

(48)

It is obviously that when $\gamma _{{\hat{R}}_kj}=\frac{1}{\sqrt{K}}$

$$\begin{aligned} \mathop {\arg \min }\limits _{{\mathcal {A}}_* \in {\mathcal {A}}_*^i} [{ SNR_{{\mathcal {A}}_*}}]= \mathop {\arg \max }\limits _{{\mathcal {A}}_* \in {\mathcal {A}}_*^i} [{ SNR_{{\mathcal {A}}_*}}]=\frac{i}{K} \sum \limits _{k=1}^K |h_{{\hat{R}}_kD}|^2 \end{aligned}$$

(49)

That means the probability of ${\mathcal {Q}}_i$ ($1\le i \le K$) achieves their maximum at the same time. Thus $\Pr ({\mathcal {Q}})$ is maximized. According to (26), the minimum of the outage probability is achieved. The theorem is proved. $\square$