1 Introduction

The evolution of the contact of rough surfaces is an important problem in contact mechanics [1] and tribology. Contact starts at the summits of the highest asperities, i.e., the real contact area is likely to be only a small fraction of the nominal contact area. The number of contacting asperities, as well as the real contact area, increases with increasing normal load. Greenwood and Williamson (GW) presented a theoretical model for contact of nominally flat rough surfaces [2]. They assumed that displacement occurs only at the contacting asperities, i.e., bulk displacement is absent. In addition, they assumed that the asperities displacement is purely elastic. Greenwood and Williamson also recognized that with increasing roughness, asperities plastic deformation may occur. To take account of this case, they introduced a dimensionless parameter, the plasticity index ψ, to determine the conditions for which their elastic model becomes invalid.

Different from nominally flat surfaces, in a rough spherical contact, both the contacting asperities and the bulk material of the sphere deform under normal load. Hence, the total displacement includes contributions of both the asperities and bulk material. Several studies dealing with contact of rough spherical surfaces can be found in the literature. Greenwood and Tripp [3] presented a model for the elastic contact of rough spheres. Using an iterative solution, they calculated the displacement of both the asperities and the sphere. They found that for light loads the total displacement at the center of the contact area is mainly due to the displacement of asperities, for high loads the total displacement is mainly due to bulk displacement of the sphere. Kagami et al. [4] analyzed the contact of a smooth sphere loaded against a rough flat. They presented a “mixed asperity contact” model, in which they assumed elastic or plastic deformation of asperities below or above a critical deformation, respectively. Experimental results for the contact radii and the total displacement are also presented in [4] as a function of normal load for rough and smooth copper and steel plates indented by a smooth steel sphere. They found that a rough spherical contact at low normal loads produces a lower contact pressure distribution and a larger contact radius compared to the Hertz solution for a smooth spherical contact. At high normal loads the roughness effect diminishes.

Greenwood et al. [5] examined the influence of surface roughness and investigated the contact pressure and contact area between a sphere and a plane. They proposed that the influence of surface roughness is governed by a single dimensionless parameter α defined by

$$ \alpha = {\frac{\sigma R}{{a^{2} }}}, $$
(1)

where σ is the combined roughness of the two contacting surfaces, R is the radius of the sphere, and a is the contact radius for contact of smooth surfaces given by the Hertz theory. It should be noted that in purely elastic contact the parameter α is in fact the ratio σ/ω, where ω is the displacement of the smooth sphere. According to Greenwood et al. [5], the effect of roughness is negligible if α is < 0.05.

Bahrami et al. [6] presented a model in which an elastic half space covered by plastically deformed asperities is indented by a rigid sphere. They observed good correlation between their theoretical results and the experimental results of Refs. [4] and [5] for the contact radius and compliance. Wang et al. [7] presented a multilevel contact model for a rough spherical contact and investigated the surface roughness effect on the real contact area. In their model, the total deformation was composed of the displacement of the bulk and asperities. Jamari and Schipper [8] evaluated the displacement behavior of the contact between a real rough flat surface and a smooth ball. They allowed three displacement responses: plastic deformation of the asperities only, plastic deformation of the bulk only, and combined plastic deformation of both the asperities and the bulk. They measured the contact area of aluminum and brass flats that were indented by a harder steel sphere and found good agreement between experimental results and theoretical predictions.

In Refs. [3–7], the total compliance of the rough spherical contact is provided without separating the individual contributions of bulk and asperities. Only in Ref. [8] such separation is made but as in Refs. [3–7] the bulk deformation of the sphere is assumed to be purely elastic. Furthermore, the displacement of the asperities in Refs. [3–8] is assumed to be purely elastic and/or ideal plastic.

Recently, Cohen et al. [9] and Li et al. [10] analyzed the contact of a rough sphere loaded by a rigid flat and relaxed previous simplifying assumptions regarding bulk and asperity deformation. They assumed that the sphere and the asperities on the sphere can have any combination of elastic, elastic–plastic, and fully plastic deformation. The main goal in those two papers was the prediction of real contact area and static friction and hence, only flattening of the asperities was reported.

The main goals of the present work are (a) to investigate the contact between a rough sphere and a rigid flat and determine the individual contributions of the asperities and the bulk of the sphere to the total displacement and (b) to find a dimensionless parameter similar to α in Eq. 1 that will not be limited to elastic displacement only.

2 Theoretical Analysis

As indicated in [3], the total displacement of a rough spherical contact is composed of displacements of both the asperities and the bulk of the sphere. Hence, the total displacement γ can be defined as

$$ \gamma = \omega + h_{1} , $$
(2)

where ω and h 1 are the displacement of the sphere bulk and the asperities on the sphere, respectively.

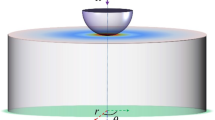
A model of the rough spherical contact is presented in Fig. 1. As shown in Refs. [9] and [10], the roughness of the sphere can be transferred to the flat without affecting the original contact problem. The contacting asperities and the sphere deform to provide a real contact area that supports the normal load P. The deformation of the asperities and the sphere can be elastic, elastic–plastic, fully plastic or any combination of the three types of deformation. The peak of the sphere is flattened, due to its displacement ω, forming a circular contact area with radius a n over which a uniform separation h 0 is assumed between the sphere and the mean of the surface heights of the rough flat. It should be noted here that representing the rough circular contact area as a flat is only a simplified approximation. The entire nominal contact area, in the presence of roughness, may be curved and its total size may be larger than the flat portion shown in Fig. 1. This larger size is accounted for in the present model by considering also asperity contact with the curved surface of the sphere outside the flattened area of radius a n. In the following, parameters that have dimensions of length in the radial direction are normalized by \( \sqrt {R\sigma } , \) parameters with dimensions of length in the normal direction are normalized by σ and are denoted with * [9].

Fig. 1
figure 1

Contact model for a rough sphere and a rigid flat showing an equivalent rough flat and a smooth sphere

Full size image

As shown in Ref. [9], the separation h* outside the flattened region has the form

$$ h^{*} \left( {r^{*} } \right) = \left\{ {\begin{array}{*{20}l} {{\frac{1}{{\pi \psi^{2} \rho /R}}}P^{*2/3} f^{*} \left( {r^{*} } \right) + h_{0}^{*} } & {0 \le P^{*} \le 1} \\ {{\frac{0.951}{{\pi \psi^{2} \rho /R}}}\left( {{\frac{{P^{*} }}{1.03}}} \right)^{0.809} f^{*} \left( {r^{*} } \right) + h_{0}^{*} ,} & {1 \le P^{*} \le 13.3} \\ {{\frac{0.457}{{\pi \psi^{2} \rho /R}}}\left( {{\frac{{P^{*} }}{1.40}}} \right)^{1.249} f^{*} \left( {r^{*} } \right) + h_{0}^{*} ,} & {13.3 \le P^{*} \le 530} \\ \end{array} } \right., $$
(3)

where ρ is the asperity summit radius and f*(r*)is given by (see [10])

$$ f^{*} (r^{*} ) = a_{\text{n}}^{*} \sqrt {r^{*2} - a_{\text{n}}^{*2} } - \left( {2a_{\text{n}}^{*2} - r^{*2} } \right)\tan^{ - 1} \left( {{\frac{{r^{*2} }}{{a_{\text{n}}^{*2} }}} - 1} \right)^{1/2} $$
(4)

The dimensionless normal load P* between the two contacting surfaces under full stick contact condition is obtained from [10] as

$$ \begin{aligned} P^{*} = {\frac{P}{{L_{\text{c}} }}} = & {\frac{{2\pi \beta \psi^{3} }}{{\left( {\bar{\delta }_{\text{c}} } \right)^{1.5} }}}{\frac{\rho }{R}}\int\limits_{0}^{{R^{*} }} {r^{*} } \left[ {\int\limits_{{h^{*} - y_{\text{s}}^{*} }}^{{h^{*} - y_{\text{s}}^{*} + \delta_{\text{c}}^{*} }} {\left( {z^{*} - h^{*} - y_{\text{s}}^{*} } \right)^{3/2} \phi^{*} \left( {z^{*} } \right)} {\text{d}}z^{*} } \right. \\ + \int\limits_{{h^{*} - y_{\text{s}}^{*} + \delta_{\text{c}}^{*} }}^{{h^{*} - y_{\text{s}}^{*} + 110\delta_{\text{c}}^{*} }} {\left( {z^{*} - h^{*} - y_{\text{s}}^{*} } \right)^{3/2} \left( {1 - I^{\alpha } } \right)\phi^{*} \left( {z^{*} } \right)} {\text{d}}z^{*} \\ \left. { + 4.6{\frac{{\bar{\delta }_{\text{c}}^{1/2} }}{\psi }}\int\limits_{{h^{*} - y_{\text{s}}^{*} + 110\delta_{\text{c}}^{*} }}^{\infty } {\left( {z^{*} - h^{*} - y_{\text{s}}^{*} } \right)\phi^{*} \left( {z^{*} } \right)} {\text{d}}z^{*} } \right]{\text{d}}r^{*} , \\ \end{aligned} $$
(5)

where the integrand I α is given by (see [9])

$$ I^{\alpha } = \exp \left[ {\left( {1 - \left( {\left( {z^{*} - h^{*} - y_{\text{s}}^{*} } \right){\frac{{\psi^{2} (\sigma /\sigma_{\text{s}} )}}{{\bar{\delta }_{\text{c}} }}}} \right)^{\alpha } } \right)^{ - 1} } \right] $$
(6)

In Eqs. 5 and 6, \( \bar{\delta }_{\text{c}} \) is the ratio δ c/ω c of the critical interference in full stick over that in perfect slip [11], \( \phi^{*} \) is the distribution function of asperity heights, and y s is the distance between the mean of the asperity heights and the mean of the surface heights (see Fig. 1). In this work, a Gaussian distribution is used. Additional details can be found in Ref. [10].

When the dimensionless separation \( h_{0}^{*} > 3 \) the number of asperities in contact is very small [12] and the surfaces are nearly separated. Hence, assuming that contact begins at \( h_{0}^{*} = 3 \) when both the normal load and the displacement of the asperities are zero, one can obtain the displacement of the asperities in the form

$$ h_{1}^{*} = 3 - h_{0}^{*} $$
(7)

The dimensionless displacement of the bulk of the sphere under slip contact condition is given by the following expression (see Ref. [13])

$$ {\frac{\omega }{{\omega_{\text{c}} }}} = \left\{ {\begin{array}{*{20}l} {P^{*2/3} ,} & {0 \le P^{*} \le 1} \\ {\left( {{\frac{{P^{*} }}{1.03}}} \right)^{0.702} ,} & {1 \le P^{*} \le 13.3} \\ {\left( {{\frac{{P^{*} }}{1.40}}} \right)^{0.801} ,} & {13.3 \le P^{*} \le 530} \\ \end{array} } \right., $$
(8)

where ω c is the critical interference of the bulk of the sphere at yield inception under slip contact condition and P* is the load P normalized by P c. It should be noted that for \( \nu > 0.25 \) the critical interference and critical load in slip and in stick contact condition are almost the same [11]. Hence, in the following we shall replace L c by P c to express P*.

Using Eq. 8, one can express the dimensionless displacement of the bulk of the sphere ω/σ in the form

$$ \omega^{*} = {\frac{\omega }{{\omega_{\text{c}} }}}\quad \omega_{\text{c}}^{*} = \left\{ {\begin{array}{*{20}l} {\omega_{\text{c}}^{*} P^{*2/3} ,} & {0 \le P^{*} \le 1} \\ {\omega_{\text{c}}^{*} \left( {{\frac{{P^{*} }}{1.03}}} \right)^{0.702} ,} & {1 \le P^{*} \le 13.3} \\ {\omega_{\text{c}}^{*} \left( {{\frac{{P^{*} }}{1.40}}} \right)^{0.801} ,} & {13.3 \le P^{*} \le 530} \\ \end{array} } \right. $$
(9)

The total dimensionless displacement γ* = γ/σ is the sum of the individual contributions of the asperities and the bulk displacements (see Eq. 2) and hence, it depends on the dimensionless normal load P*, the plasticity index ψ, and the parameter ω *c .

$$ \gamma^{*} = \omega^{*} + h_{1}^{*} $$
(10)

3 Results and Discussion

In this investigation, we use the same values of Poisson’s ratio (ν = 0.3) and surface roughness parameter (β = 0.04) as used in Refs. [9, 10]. An iterative solution (see Ref. [9]) was implemented to obtain the dimensionless normal load P* for varying separation h *0 (and, hence, h *1 , see Eq. 7) as a function of the plasticity index ψ. Then, ω* was obtained from Eq. 9 for the calculated P* values at various values of ω *c . Note that for σ ≈ σ s it is reasonable to express ω *c in the form (see List of symbols):

$$ \omega_{\text{c}}^{*} = {\frac{R}{\rho }}\psi^{ - 2} $$
(11)

This approximation may be valid even when σ is up to 10% smaller than σ s since the error in ψ (see List of symbols), which is related to the square root of the error in σ s, will be no more than 5%. Substituting the ratio ρ/R from Eq. 11 in Eqs. 3 and 5, we observe that Eq. 5 is independent of ρ/R. Hence, the parameters for the problem at hand are ψ and ω *c . Furthermore, as will be seen later, the results obtained from Eq. 5 are independent of ω *c . Hence, ψ affects the displacement of the asperities and ω *c affects the displacement of the sphere bulk.

Figure 2 shows the effect of the critical interference ω *c on the relation between the dimensionless normal load P* and the individual displacements h *1 and ω* of the asperities and the bulk of the sphere, respectively, along with their sum (the total displacement) γ*. The results are presented for a typical plasticity index ψ = 2 and three different values of ω *c  = 1, 2, and 5. The combination of the displacement of the bulk of the sphere and asperities can be described as a system of two nonlinear springs in series (see, e.g., [14]). Initially, at small loads, the stiffness of the asperities is much smaller than that of the bulk and hence, the contribution of the asperities to the total displacement is dominant. As the load P* increases the asperities are flattened, their stiffness increases rapidly and becomes larger than that of the bulk. Eventually, at a dimensionless transition load P *t the bulk displacement equals the displacement of the asperities. At P* > P *t the additional displacement of the stiffer asperities contributes very little to the increase of the total displacement and the bulk contribution becomes the dominant one. For ω *c  = 1, shown in Fig. 2a, the transition load is about P *t  = 16. As ω *c increases (see Fig. 2b, c) the dimensionless transition load P *t decreases. Rearranging Eq. 9 to express P* as a function of ω* it can be seen that the dimensionless stiffness of the bulk \( \partial P^{*} /\partial \omega^{*} \) decreases with increasing ω *c . A lower dimensionless stiffness of the bulk material increases the displacement at a given load and hence, reduces the dimensionless transition load P *t with increasing ω *c .

Fig. 2
figure 2

Dimensionless normal load P* versus dimensionless displacement of asperities h *1 , sphere bulk ω*, and the total displacement γ* for ψ = 2 and dimensionless critical interference: a ω *c  = 1, b ω *c  = 2, and c ω *c  = 5

Full size image

In Figs. 2a and 3, the effect of the plasticity index ψ on the relation between the normal load P* and the displacement components is presented. The results are shown for a typical dimensionless critical interference ω *c  = 1 and three values of the plasticity index ψ = 2, 5, and 8. As can be seen from these figures, as ψ increases, a higher load P* is required for a given displacement h *1 of the asperities. This is due to increased dimensionless stiffness of the asperities with increasing ψ [12]. Increased stiffness of the asperities affects the transition load in the same way as decreased stiffness of the bulk. Therefore, the dimensionless transition load P *t decreases with increasing ψ.

Fig. 3
figure 3

Dimensionless normal load P* versus dimensionless displacement for asperities h *1 , sphere bulk ω*, and the total displacement γ* for ω *c  = 2 and plasticity index: a ψ = 5 and b ψ = 8

Full size image

Figure 4 shows the combined effect of the controlling parameters ω *c and ψ on the dimensionless transition load P *t . As observed from the figure, for small values of both ω *c and ψ, the transition load P *t is very large, i.e., the contribution of the asperities to the total displacement is dominant. On the other hand, large ω *c or large ψ reduces P *t to very small values and hence, the contribution of the bulk to the total displacement becomes dominant. The results shown in Fig. 4 were curve fitted yielding the following empirical expression (with R 2 value above 0.99)

$$ P_{\text{t}}^{*} = \left( {75.8(\omega_{\text{c}}^{*} )^{ - 1.7} + 4.7} \right)\psi^{{0.01\omega_{\text{c}}^{*} - 0.16}} - \left( {51.3(\omega_{\text{c}}^{*} )^{ - 1.7} + 4.7} \right) $$
(12)

The dimensionless parameter P *t can be looked upon as a criterion for the importance of the effect of roughness on the total displacement of a rough spherical contact. If P* < P *t , the effect of the roughness on the total displacement must be considered. On the other hand, if P* is much larger than P *t , the contribution of the asperities can be neglected. A similar roughness effect on static friction coefficient and junction growth of a rough spherical contact was recently observed experimentally by Ovcharenko et al. [15, 16], and predicted theoretically by Cohen et al. [9]. In all these studies, the effect of surface roughness was found to diminish with an increase in the normal load above a threshold value. Interestingly, a somewhat similar transition load was observed in a nano-spherical contact [17] where the effect of an adsorbed layer on junction growth is investigated. Apparently, the effect of splaying out of adsorbed layers is similar to that of flattening asperities.

Fig. 4
figure 4

Dimensionless transition load \( P_{\text{t}}^{*} \) as functions of the plasticity index ψ and \( \omega_{\text{c}}^{*} \)

Full size image

It may be argued that some of the assumptions made in this study are oversimplification of an actual rough spherical contact. For example, a central flat contact area (see Fig. 1), which is typical for a smooth spherical contact, may not be adequate in the presence of roughness. Also, assuming Gaussian distribution of asperity heights requires an adequate statistical sample of asperity contacts. This can be reasonably met for many contact problems of nominally flat surfaces but may be questionable in concentrated contact problems, especially under low load with corresponding small contact area typical of elastic spherical contact (see Ref. [3]). A discussion of the real area of contact and real contact pressure is provided in Ref. [3]. It reveals that the variation of the mean real pressure with load in elastic rough spheres is very similar to that in elastic rough flat surfaces. This finding supports the present assumption of central flat contact area.

Another issue is that contact at nano-micro scales can be influenced by effects specific for these scales including effects of size-dependent plasticity (see, e.g., Ref. [18]), local anisotropy of contacting asperities and adhesion. The best way to clarify such issues and test the validity of the model is through experimental verification, as described in the following section.

4 Comparison with Experimental Results

Some preliminary experiments were performed in order to test the validity of the proposed contact model. A stainless steel (SST304) spherical specimen with a radius of R = 200 μm (see Fig. 5) was loaded against a rigid smooth sapphire flat using a modified nano-indenter test rig (Hysitron, Inc., USA). The spherical specimen has a yield strength Y = 1.68 GPa, Young’s modulus E = 181 GPa, and Poisson’s ratio ν = 0.31. With these material properties, the critical interference and critical load of the sphere are (see List of symbols) ω c = 186 nm and P c = 212 mN, respectively. The surface roughness of the spherical specimen was measured by an atomic force microscope (AFM, Pacific Nanotechnology, USA) using a scan size of 10 × 10 μm2 and a sampling interval of 0.69 μm. The roughness parameters, calculated from these measurements, were ρ = 8.30 μm and σ ≈ σ s = 90 nm. The sapphire flat has a negligible roughness σ of only 4 nm, Y = 2.95 GPa, Young’s modulus E = 435 GPa, and Poisson’s ratio ν = 0.27. Using the above material properties and roughness parameters the resulting value of the plasticity index is ψ = 3, the dimensionless critical interference is ω *c  = 2, and the dimensionless transition load (see Eq. 12) is P *t  = 3.03, which for P c = 212 mN corresponds to a dimensional value of P t = 642 mN. The loading range of the nano-indenter is no more than 10 mN, much below the transition load. Hence, according to the model prediction, a dominant contribution of the asperities to the total displacement should be expected.

Fig. 5
figure 5

Top view of the spherical specimen

Full size image

Figure 6 shows a comparison between the measured total displacement versus normal load (solid line) and the theoretical prediction (dashed lines). As can be seen from the figure, the theoretical total displacement, predicted by the contact model, correlates well with the experimental results. Also, the contribution of the asperities to the total displacement is dominant as predicted by the model for loads much below the transition load.

Fig. 6
figure 6

Comparison of experimental results (solid line) and theoretical predictions (dashed lines) for normal load versus displacement of a rough spherical sample having ψ = 3 and ω *c  = 2

Full size image

Finally, Fig. 7 shows an AFM image of the rough sphere after unloading from a 9 mN normal load corresponding to P* = 0.04. A certain portion of the contact area zone is shown encircled. It clearly demonstrates the substantial flattening of contacting asperities compared to all the other original asperities, which were outside the contact area. The very small normal load of 9 mN (P* = 0.04) caused very small elastic deformation of the sphere bulk but the asperities have large plastic deformation. It can also be seen from the figure that there was an adequate statistical sample of asperity contacts to justify the assumption of Gaussian distribution of asperity heights. Hence, these preliminary experimental results verify the contact model and justify its simplifying assumptions.

Fig. 7
figure 7

AFM image of surface roughness of a rough sphere after 200 load–unload cycles, showing the flattened asperities within the encircled portion of the contact area

Full size image

5 Conclusion

The effect of critical interference and plasticity index on the contributions of asperities and sphere bulk to the total displacement of a rough spherical contact was investigated. A dimensionless transition load P *t was found as a function of the bulk critical interference ω *c and the plasticity index ψ. This transition load decreases with an increase in ω *c and ψ. At loads much smaller than the dimensionless transition load the stiffness of the asperities is smaller than that of the bulk of the sphere and the asperity displacement is dominant. At loads above the transition load the contribution of the bulk to the total displacement becomes larger than the contribution of the asperities. At loads much larger than the dimensionless transition load, the roughness effect is negligible. Preliminary experimental results verified the theoretical predictions and validate the contact model.