Study of Dispersion in Porous Media by Pulsed Field Gradient NMR: Influence of the Fluid Rheology

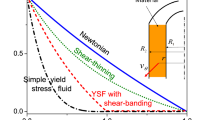
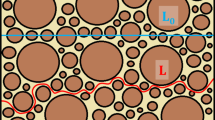
Abstract

Pulsed field gradient nuclear magnetic resonance (PFG-NMR) is used to measure the molecular displacements for the flow of a fluid through a capillary tube and a packed bed made of monodisperse PMMA beads. The molecules average displacement is studied using both the formalism of propagators and the cumulant method. In the Poiseuille case, the dispersion coefficients determined by the cumulant method compare satisfactorily with the theoretical values obtained. The technique is then extended to study the flow through a porous medium. We thus analyze Newtonian (water) and non-Newtonian (Xanthan) flows and put a particular emphasis on comparing the dispersion mechanisms between Newtonian and non-Newtonian fluids.

A Appendix

1.1 A.1 Homogenization analysis of the Taylor’s tube

The transport equation in a Taylor’s tube of radius R is written at the microscopic scale:

$$\begin{aligned} \displaystyle \frac{\partial c}{\partial t} + u(r) \displaystyle \frac{\partial c}{\partial z} = \mathscr {D}_{0} \left[ \displaystyle \frac{1}{r} \displaystyle \frac{\partial }{\partial r} \left( r \displaystyle \frac{\partial c}{\partial r}\right) + \displaystyle \frac{\partial ^{2}c}{\partial z^{2}}\right] \end{aligned}$$

(20)

with the following boundary conditions:

$$\begin{aligned} r = R , \quad \displaystyle \frac{\partial c}{\partial r} = 0 \end{aligned}$$

(21)

This equation is put in dimensionless form with $r^{*}= r/R$, $z^{*}= z/L$, $\epsilon = R /L$, $t^{*} = u_{m} \, t / L$, $u^{*} = u / u_{m}$ where $u_{m}$ is the average flow. All unknowns without dimension are of order $\mathscr {O}(1)$ except $\epsilon$ which is a small parameter. The important choice is $t^{*}$: the characteristic time at the macroscopic scale of the process is the convective time $L/u_{m}$. Therefore:

$$\begin{aligned} \displaystyle \frac{\partial c}{\partial t^{*}} + u^{*}(r^{*}) \displaystyle \frac{\partial c}{\partial z^{*}} = \displaystyle \frac{1}{\mathrm{Pe}} \left[ \epsilon \, \displaystyle \frac{\partial ^{2}c}{\partial {z^{*}}^{2}} + \displaystyle \frac{1}{\epsilon } \ \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial c}{\partial r^{*}}\right) \right] \end{aligned}$$

(22)

The order of magnitude of the Péclet number ${\mathrm{Pe}} = u_{m} R / \mathscr {D}_{0}$ appearing in Eq. (22) has to be given. In the case of the Taylor dispersion, we assume that at the microscopic scale, the diffusion transport time ($\simeq R^{2}/\mathscr {D}_{0}$) and convective transport time ($\simeq R / u_{m}$) are of the same order of magnitude and therefore ${\mathrm{Pe}} = \mathscr {O}(1)$.

We then search the concentration c in the form of a power series expansion of the small parameter $\epsilon$:

$$\begin{aligned} c = \sum _{m=0}^{+\infty } c^{(m)} \left( t^{*}, r^{*}, z^{*} \right) \ \epsilon ^{m} \end{aligned}$$

(23)

At the order $\mathscr {O}(\epsilon ^{-1})$, the problem is written as:

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial c^{(0)}}{\partial r^{*}}\right) = 0\\ r^{*} = 1 \quad \displaystyle \frac{\partial c^{(0)}}{\partial r^{*}} = 0 \end{array}\right. \end{aligned}$$

(24)

The concentration $c^{(0)}$ does not depend on r: $c^{(0)} (t^{*}, r^{*}, z^{*}) = c^{(0)} (t^{*}, z^{*})$. At the next order $\mathscr {O}(\epsilon ^{0})$, the problem is written as:

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle \frac{\partial c^{(0)}}{\partial t^{*}} + u^{*} \displaystyle \frac{\partial c^{(0)}}{\partial z^{*}} = \displaystyle \frac{1}{\mathrm{Pe}} \ \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial c^{(1)}}{\partial r^{*}}\right) \\ r^{*}=1 \qquad \displaystyle \frac{\partial c^{(1)}}{\partial r^{*}} = 0 \end{array}\right. \end{aligned}$$

(25)

Averaging Eq. (25) on the tube section leads to

$$\begin{aligned} \displaystyle \frac{\partial c^{(0)}}{\partial t^{*}} + \displaystyle \frac{\partial c^{(0)}}{\partial z^{*}} = 0 \end{aligned}$$

(26)

The transport of $c^{(0)}$ at the first order of approximation is thus purely convective with the average velocity $u_{m}$. Equation (25) could then be written using (26) to express the time derivative:

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle \frac{1}{\mathrm{Pe}} \ \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial c^{(1)}}{\partial r^{*}}\right) = \left( u^{*}-1\right) \displaystyle \frac{\partial c^{(0)}}{\partial z^{*}}\\ r^{*}=1 \quad \displaystyle \frac{\partial c^{(1)}}{\partial r^{*}}= 0 \end{array}\right. \end{aligned}$$

(27)

The only non-homogeneous term of Eq. (27) in $c^{(1)}$ is the term in $\displaystyle \frac{\partial c^{(0)}}{\partial z^{*}}$. $c^{(1)}$ is therefore searched in the form $c^{(1)}(t^{*}, r^{*}, z^{*}) = f(r^{*}) \, \displaystyle \frac{\partial c^{(0)}}{\partial z^{*}}(t^{*}, z^{*}) + \widehat{c}^{\, (1)}(t^{*}, z^{*})$ within an integration constant $\widehat{c}^{\, (1)}$ function of $t^{*}$ and $z^{*}$. f is solution of:

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial f}{\partial r^{*}}\right) = {\mathrm{Pe}} \left( u^{*} - 1 \right) \\ r^{*}=1 \quad \displaystyle \frac{\partial f}{\partial r^{*}} = 0 \end{array} \right. \end{aligned}$$

(28)

where for a circular tube and a power-law fluid

$$\begin{aligned} u^{*} = \displaystyle \frac{3n+1}{n+1} \left( 1-{r^{*}}^{1+{\frac{1}{n}}}\right) \end{aligned}$$

(29)

Integration of Eq. (28) leads to (the remaining integration constant is calculated requiring that the average value of f over the section is null):

$$\begin{aligned} f(r^{*}) = {\mathrm{Pe}} \left[ - \displaystyle \frac{n(7n+1)}{4(3n+1)(5n+1)} + \displaystyle \frac{n^{2}}{2(n+1)} {r^{*}}^{2} - \displaystyle \frac{n^{2}}{(n+1)(3n+1)} {r^{*}}^{3+{\frac{1}{n}}} \right] \end{aligned}$$

(30)

such as (with $\langle (-) \rangle = 1/S \int _{S} (-) {~\mathrm d} S$

$$\begin{aligned} c^{(1)} = \langle c^{(1)} \rangle (t^{*}, z^{*}) + f(r^{*}) \displaystyle \frac{\partial c^{(0)}}{\partial z^{*}} (t^{*}, z^{*}) \end{aligned}$$

(31)

At the order $\mathscr {O}(\epsilon )$, the equation is written as:

$$\begin{aligned} \left\{ \begin{array}{l} \displaystyle \frac{\partial c^{(1)}}{\partial t^{*}} + u^{*} \displaystyle \frac{\partial c^{(1)}}{\partial z^{*}} = \displaystyle \frac{1}{\mathrm{Pe}} \left[ \, \displaystyle \frac{\partial ^{2}c^{(0)}}{\partial {z^{*}}^{2}} + \displaystyle \frac{1}{r^{*}} \displaystyle \frac{\partial }{\partial r^{*}} \left( r^{*} \displaystyle \frac{\partial c^{(2)}}{\partial r^{*}}\right) \right] \\ r^{*}=1 \quad \displaystyle \frac{\partial c^{(2)}}{\partial r^{*}} = 0 \end{array}\right. \end{aligned}$$

(32)

Averaging in r leads to:

$$\begin{aligned} \displaystyle \frac{\partial \langle c^{(1)}\rangle }{\partial t^{*}} + \left\langle u^{*} \displaystyle \frac{\partial c^{(1)}}{\partial z^{*}}\right\rangle = \displaystyle \frac{1}{\mathrm{Pe}} \, \displaystyle \frac{\partial ^{2}c^{(0)}}{\partial {z^{*}}^{2}} \end{aligned}$$

(33)

Adding Eqs. (26) and (33) using relation (31) leads to

$$\begin{aligned} \displaystyle \frac{\partial }{\partial t^{*}} \left( c^{(0)} + \langle c^{(1)}\rangle \right) + \displaystyle \frac{\partial }{\partial z^{*}} \left( c^{(0)} + \langle c^{(1)}\rangle \right)= & {} \left[ \displaystyle \frac{1}{\mathrm{Pe}} - \left\langle u^{*} \, f \right\rangle \right] \displaystyle \frac{\partial ^{2}c^{(0)}}{\partial {z^{*}}^{2}} \end{aligned}$$

(34)

$$\begin{aligned} \text{ As }\quad - \left\langle u^{*} \, f \right\rangle = -2 \int _{r^{*}=0}^{1} u^{*} f(r^{*}) \, r^{*} \, \mathrm{d} r^{*}= & {} \displaystyle \frac{n^{2}}{2(3n+1)(5n+1)} \ {\mathrm{Pe}} \end{aligned}$$

(35)

the average equation is finally written for $\langle c\rangle = c^{(0)} + \langle c^{(1)} \rangle$

$$\begin{aligned} \displaystyle \frac{\partial \langle c\rangle }{\partial t} + u_{m} \displaystyle \frac{\partial \langle c\rangle }{\partial z}= & {} D_T \displaystyle \frac{\partial ^{2} \langle c\rangle }{\partial z^{2}} + \mathscr {O}\left( \epsilon ^{2} \displaystyle \frac{u_{m} \, \langle c \rangle }{L}\right) \end{aligned}$$

(36)

$$\begin{aligned} \text{ with } \displaystyle \frac{D_T}{\mathscr {D}_{0}}= & {} 1 + \displaystyle \frac{n^{2}}{2(3n+1)(5n+1)} \mathrm{Pe^{2}} \end{aligned}$$

(37)

The same result can be obtained by other methods (see for example Vartuli et al. 1995). The method presented above has the advantage of an easy extension to the general case of a porous medium (Auriault and Adler 1995). Note that in the Newtonian case, $n=1$, the classical Taylor result $D_T/\mathscr {D}_{0} = 1 + \mathrm{Pe}^{2}/48$ is recovered.