Skip to main content
SpringerLink
Log in

Onset of Double-Diffusive Reaction–Convection in an Anisotropic Rotating Porous Layer

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

Abstract

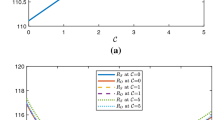
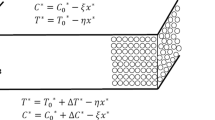
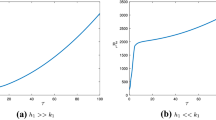
The linear and non-linear stability of a rotating double-diffusive reaction–convection in a horizontal anisotropic porous layer subjected to chemical equilibrium on the boundaries is investigated considering a Darcy model that includes the Coriolis term. The effect of Taylor number, mechanical, and thermal anisotropy parameters, reaction rate, solute Rayleigh number, Lewis number, and normalized porosity on the stability of the system is investigated. We find that the Taylor number has a stabilizing effect, chemical reaction may be stabilizing or destabilizing and that the anisotropic parameters have significant influence on the stability criterion. The effect of various parameters on the stationary, oscillatory, and finite-amplitude convection is shown graphically. A weak nonlinear theory based on the truncated representation of Fourier series method is used to find the finite amplitude Rayleigh number and heat and mass transfer.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Abbreviations

\(a\) :

Wavenumber

\(d\) :

Height of the porous layer [m]

\(\mathbf{g}\) :

Gravitational acceleration, (0, 0,-g) [m s\(^{-2}\)]

K :

Inverse anisotropic permeability tensor, \(K_x^{-1} \mathbf{ii}+K_y^{-1} \mathbf{jj}+K_z^{-1} \mathbf{kk}\)

\(k\) :

Lumped effective reaction rate

Le :

Lewis number, \({\kappa _{\mathrm{T}z} }/{\kappa _\mathrm{S} }\)

\(l, m\) :

Horizontal wavenumbers

Nu :

Nusselt number

\(p\) :

Pressure [kg m\(^{-1}\) s\(^{-2}\)]

\(\mathbf{q}\) :

Velocity vector (u,v,w) [m s\(^{-1}\)]

\(Ra_T \) :

Darcy–Rayleigh number, \({\rho _{0} \beta _\mathrm{T} g\Delta TdK_z }/{ \mu \varepsilon \kappa _{\mathrm{T}z} }\)

\(Ra_\mathrm{S} \) :

Solute Rayleigh number, \({\rho _{0} \beta _\mathrm{S}g\Delta SdK_z }/{\varepsilon \mu \kappa _{\mathrm{T}z} }\)

\(S\) :

Solute concentration

\(S_\mathrm{eq} (T)\) :

Equilibrium concentration of the solute at a given temperature

Sh :

Sherwood number

\(\Delta S\) :

Salinity difference between the walls

\(t\) :

Time [s]

\(T\) :

Temperature [K]

\(Ta\) :

Darcy Taylor number \(({{2\Omega K_z }/\mu })^{2}\)

\(\Delta T\) :

Temperature difference between the walls [K]

\(V_{z}\) :

\(z\) component of vorticity vector \(\mathbf{V}=\nabla \times \mathbf{q} \; [{\text{ m} \text{ s}}^{-1}]\)

\(x, y, z\) :

Space coordinates [d]

\(\beta _\mathrm{T}\) :

Thermal expansion coefficient

\(\beta _\mathrm{S}\) :

Solute expansion coefficient

\(\varepsilon \) :

Porosity

\(\varvec{\Omega }\) :

Angular velocity [radians s\(^{-1}\)] (\({0,0,\Omega }\))

\(\eta \) :

Thermal anisotropy parameter, \({\kappa _{\mathrm{T}x} }/{\kappa _{\mathrm{T}z} }\)

\(\varvec{\kappa }_\mathrm{T}\) :

Anisotropic thermal diffusion tensor, \(\kappa _{\mathrm{T}x} \mathbf{ii}+\kappa _{\mathrm{T}y} \mathbf{jj}+\kappa _{\mathrm{T}z} \mathbf{kk}\)

\(\kappa _\mathrm{S} \) :

Solute diffusivity

\(\lambda \) :

Normalized porosity parameter,\(\varepsilon \frac{({\rho c})_\mathrm{f} }{({\rho c})_\mathrm{m }}\)

\(\chi \) :

Damkohler number, \({kd^{2}}/{\varepsilon \kappa _{\mathrm{T}z} }\)

\(\mu \) :

Dynamic viscosity [N s m\(^{-2}\)]

\(\nu \) :

Kinematic viscosity [m\(^{2}\) s\(^{-1}\)]

\(\rho \) :

Density [kg m\(^{-3}\)]

\(\rho c\) :

Volumetric heat capacity

\(\sigma \) :

Growth rate

\(\xi \) :

Mechanical anisotropy parameter, \({K_x }/{K_z }\)

\(\psi \) :

Stream function

\(\nabla _1^2 \) :

\(\frac{\partial ^{2}}{\partial x^{2}}+\frac{\partial ^{2}}{\partial y^{2}}\)

\(\nabla ^{2} \) :

\(\nabla _1^2 +\frac{\partial ^{2}}{\partial z^{2}}\)

b:

Basic state

c:

Critical

f:

Fluid

m:

Porous medium

0:

Reference value

s:

Solid

i:

Imaginary part

*:

Dimensionless quantity

’:

Perturbed quantity

F:

Finite amplitude

Osc:

Oscillatory state

St:

Stationary state

References

  • Chakrabarti, A., Gupta, A.S.: Nonlinear thermohaline convection in a rotating porous medium. Mech. Res. Commun. 8(1), 9–22 (1981)

    Google Scholar 

  • Gaikwad, S.N., Malashetty, M.S., Prasad, K.R.: An analytical study of linear and nonlinear double diffusive convection in a fluid saturated anisotropic porous layer with Soret effect. Appl. Math. Model. 33, 3617 (2009a)

    Article  Google Scholar 

  • Gaikwad, S.N., Malashetty, M.S., Prasad, K.R.: Linear and nonlinear double diffusive convection in a fluid saturated anisotropic porous layer with cross diffusion effects. Transp. Porous Media 80, 537 (2009b)

    Article  Google Scholar 

  • Gatica, J.E., Viljoen, H.J., Hlavacek, V.: Interaction between chemical reaction and natural convection in porous media. Chem. Eng. Sci. 44(9), 1853 (1989)

    Article  Google Scholar 

  • Guo, J., Kaloni, P.N.: Nonlinear stability problem of a rotating doubly diffusive porous layer. J. Math. Anal. Appl. 190, 37–390 (1995)

    Article  Google Scholar 

  • Horton, C.W., Rogers, F.T.: Convection currents in a porous medium. J. Appl. Phys. 16, 367–370 (1945)

    Article  Google Scholar 

  • Ingham, D.B., Pop, I.: Transport Phenomena in Porous Media. Pergamon Press, Oxford (1998)

    Google Scholar 

  • Ingham, D.B., Pop, I.: Transport Phenomena in Porous Media, vol. 3. Elsevier, Oxford (2005)

    Google Scholar 

  • Jupp, T.E., Woods, A.W.: Thermally-driven reaction fronts in porous media. J. Fluid Mech. 484, 329–346 (2003)

    Google Scholar 

  • Lapwood, E.R.: Convection of a fluid in a porous medium. Proc. Camb. Phil. Soc. 44, 508–521 (1948)

    Article  Google Scholar 

  • Lombardo, S., Mulone, G.: Necessary and sufficient conditions of global nonlinear stability for rotating double diffusive convection in a porous medium. Continuum Mech. Thermodyn. 14(16), 527–540 (2002)

    Article  Google Scholar 

  • Malashetty, M.S., Bharati, Biradar S.: The onset of double diffusive reaction-convection in an anisotropic porous layer. Phys. Fluids 23, 064102 (2011). doi:10.1063/1.3598469

    Article  Google Scholar 

  • Malashetty, M.S., Gaikwad, S.N.: Onset of convective instabilities in a binary liquid mixtures with fast chemical reactions in a porous medium. Heat Mass Transf. 39, 415 (2003)

    Google Scholar 

  • Malashetty, M.S., Heera, R.: The effect of rotation on the onset of double diffusive convection in a horizontal anisotropic porous layer. Transp. Porous Media 74, 105 (2008)

    Article  Google Scholar 

  • Malashetty, M.S., Swamy, M.: The onset of convection in a binary fluid saturated anisotropic porous layer. Int. J. Therm. Sci 49, 867 (2010)

    Article  Google Scholar 

  • McKibbin, R.: Thermal convection in layered and anisotropic porous media, a review. In: Wooding, R.A., White, I. (eds.) Convective Flows in Porous Media, pp. 113–127. Department of Scientific and Industrial Research, Wellington (1985)

    Google Scholar 

  • Nield, D.A., Bejan, A.: Convection in Porous Media, 3rd edn. Springer, New York (2006)

    Google Scholar 

  • Phillips, O.M.: Geological Fluid Dynamics. Cambridge University Press, New York (2009)

    Book  Google Scholar 

  • Pritchard, D., Richardson, C.N.: The effect of temperature-dependent solubility on the onset of thermosolutal convection in a horizontal porous layer. J. Fluid Mech. 9, 571 (2007)

    Google Scholar 

  • Rudraiah, N., Shivakumara, I.S., Friedrich, R.: The effect of rotation on linear and nonlinear double diffusive convection in a sparsely packed porous medium. Int. J. Heat Mass Transf. 29, 1301–1317 (1986)

    Article  Google Scholar 

  • Steinberg, V., Brand, H.: Amplitude equations for the onset of convection in a reactive mixture in a porous medium. J. Chem. Phys. 80(1), 431 (1984)

    Article  Google Scholar 

  • Steinberg, V., Brand, H.: Convective instabilities of binary mixtures with fast chemical reaction in a porous medium. J. Chem. Phys. 78(5), 2655 (1983)

    Article  Google Scholar 

  • Storesletten, L., et al.: Effects of anisotropy on convection in horizontal and inclined porous layers. In: Ingham, D.B. (ed.) Emerging Technologies and Techniques in Porous Media, pp. 285–306. Kluwer Academic Publishers, Dordrecht (2004)

    Chapter  Google Scholar 

  • Storesletten, L.: Effects of anisotropy on convective flow through porous media. In: Ingham, D.B., Pop, I. (eds.) Transport Phenomena in Porous Media, pp. 261–283. Pergamon Press, Oxford (1998)

    Chapter  Google Scholar 

  • Tyvand, P.A.: Thermohaline instability in anisotropic porous media. Water Resour. Res. 16, 325 (1980)

    Article  Google Scholar 

  • Vadas, : Emerging Topics in Heat and Mass Transfer in Porous Media. Springer, New York (2008)

    Book  Google Scholar 

  • Vafai, : Handbook of Porous Media. Marcel Dekker, New York (2000)

    Book  Google Scholar 

  • Vafai, K.: Handbook of Porous Media. Taylor & Francis/CRC Press, London/Boca Raton (2005)

    Book  Google Scholar 

  • Viljoen, H.J., Gatica, J.E., Hlavacek, V.: Bifurcation analysis of chemically driven convection. Chem. Eng. Sci. 45(2), 503 (1990)

    Article  Google Scholar 

Download references

Acknowledgments

This study was supported by University Grants Commission, New Delhi, under Maulana Azad National Scholarship to Minority Students and partially under Major Research Project F. No. 37-174/2009 (SR) dated 12-01-2010. One of the authors (Irfana Begum) thanks UGC for awarding the Scholarship. The authors thank the reviewers for their useful suggestions.

Author information

Authors and Affiliations

  1. Department of Mathematics, Gulbarga University, Jnana Ganga Campus, Gulbarga, 585106, India

    S. N. Gaikwad & Irfana Begum

Authors
  1. S. N. Gaikwad
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Irfana Begum
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. N. Gaikwad.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Gaikwad, S.N., Begum, I. Onset of Double-Diffusive Reaction–Convection in an Anisotropic Rotating Porous Layer. Transp Porous Med 98, 239–257 (2013). https://doi.org/10.1007/s11242-013-0143-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11242-013-0143-0

Keywords

Search

Navigation